How To Solve Trigonometric Equations With Multiple Angles - Trigonometry
TLDRThis educational video script discusses solving trigonometric equations with multiple angles. It begins with finding the values of x for sin(2x) = 1/2 within the range of 0 to 2π, identifying two possible solutions: π/12 and 5π/12. The script then illustrates how to find solutions for tan(3x) = -1, resulting in six solutions due to the triple angle. It explains the pattern of increasing solutions with higher powers of trigonometric functions and concludes with an example of cos(x/2) = -1/2, which yields a single solution within the specified range. The script effectively demonstrates trigonometric problem-solving techniques and the relationship between angles and their solutions.
Takeaways
- 📚 The script discusses solving trigonometric equations involving multiple angles for specific values of x within the range of 0 to 2π.
- 🔍 It explains how to find the values of x for the equation sine(2x) = 1/2 by identifying angles whose sine is 1/2 and considering the periodic nature of the sine function.
- 📉 The solution process involves using the general form of the sine function, 2x = π/6 + 2πn or 2x = 5π/6 + 2πn, and solving for x.
- 📝 The script identifies that there are two solutions for sine(2x) = 1/2 within the given range: x = π/12 and x = 5π/12 when n=0, and additional solutions for different values of n.
- 🔢 For the tangent function, the script shows how to find solutions for tangent(3x) = -1 by considering the angles in quadrants where tangent is negative.
- 📐 It explains that tangent(3x) = -1 has solutions when 3x equals 3π/4 + πm or 7π/4 + πm, and then solves for x by dividing by 3.
- 📈 The script demonstrates that the number of solutions for a trigonometric function increases with the multiple of the angle, e.g., tangent x has 2 solutions, tangent 3x has 6 solutions.
- 📉 For cosine(x/2) = -1/2, the script finds the solution by setting x/2 equal to angles where cosine is negative and then solving for x.
- 🔍 The solution for cosine(x/2) = -1/2 is x = 4π/3, as it is the only value that satisfies the equation within the specified range.
- 📝 The script emphasizes the importance of considering the range of possible solutions and the periodicity of trigonometric functions when solving such equations.
- 📚 The process of finding solutions involves understanding the properties of trigonometric functions and their behavior in different quadrants.
Q & A
If sine(2x) = 1/2, what are the possible values of x between 0 and 2π?
-The possible values of x are π/12, 5π/12, 13π/12, and 17π/12. These are derived from setting 2x equal to π/6 + 2πn and 5π/6 + 2πn, then dividing by 2.
Why are only the solutions between 0 and 2π considered for the sine(2x) = 1/2 equation?
-The restriction to solutions between 0 and 2π is due to the periodic nature of the sine function and the desire to find principal values of the angle x.
How does the sign of the sine function relate to the quadrants in the context of the sine(2x) = 1/2 problem?
-The sine function is positive in the first and second quadrants. Since sine(2x) = 1/2 and 1/2 is positive, the solutions are found in the first quadrant (π/6) and the second quadrant (5π/6).
What is the significance of the value n in the equations 2x = π/6 + 2πn and 2x = 5π/6 + 2πn?
-The value n represents the periodic increments of the sine function, allowing for the calculation of additional solutions within the specified range by accounting for the sine function's periodicity.
How does the number of solutions for the equation sine(2x) = 1/2 compare to the number of solutions for sine(x) = 1/2?
-For sine(x) = 1/2, there are two solutions within the range of 0 to 2π: π/6 and 5π/6. However, for sine(2x) = 1/2, the number of solutions doubles to four, due to the multiple angles involved.
What are the general solutions for the tangent(3x) = -1 equation within the interval from 0 to 2π?
-The general solutions are x = π/4 + π/3n and x = 7π/4 + π/3n, where n is an integer. These are derived from setting 3x equal to 3π/4 + πm and 7π/4 + πm, then dividing by 3.
Why are the solutions for tangent(3x) = -1 tripled compared to tangent(x) = -1?
-The number of solutions triples because the angle is tripled (from x to 3x), which means for every solution of tangent(x), there will be three corresponding solutions for tangent(3x) within the given range.
In which quadrants is the tangent function negative, and how does this relate to the tangent(3x) = -1 problem?
-The tangent function is negative in the second and fourth quadrants. This is relevant for the tangent(3x) = -1 problem because the solutions are found in these quadrants at angles 3π/4 and 7π/4.
How can you determine the number of solutions for an equation involving a trigonometric function of x raised to a power?
-The number of solutions can be determined by considering the number of solutions for the basic trigonometric function (e.g., tangent(x)) and multiplying by the power of x (e.g., for tangent(3x), there would be three times as many solutions).
What is the only solution for the equation cosine(x/2) = -1/2 within the range of 0 to 2π?
-The only solution is x = 4π/3. This is because x/2 must be equal to 2π/3 + 2πn or 4π/3 + 2πn, and when solving for x, only x = 4π/3 falls within the specified range.
Why is there only one solution for the equation cosine(x/2) = -1/2, unlike the two solutions for cosine(x) = -1/2?
-There is only one solution because halving the angle (x/2) reduces the number of solutions within the 0 to 2π range from two to one, as the reference angle of 60° (π/3) leads to only one valid angle when doubled.
Outlines
📚 Solving Trigonometric Equations with Multiple Angles
This paragraph introduces the process of solving trigonometric equations where the angle is multiplied, such as sin(2x) = 1/2. The solution involves identifying the angles whose sine is 1/2, which are 30° or π/6 and 150° or 5π/6. The equation 2x is set equal to these angles plus 2πn, where n is an integer, to account for the periodic nature of the sine function. The solution then involves dividing by 2 to solve for x, yielding two sets of solutions: x = π/12 + πn and x = 5π/12 + πn. The paragraph explores the solutions for different values of n, keeping them within the range of 0 to 2π, and explains that the number of solutions doubles when dealing with 2x compared to a single x.
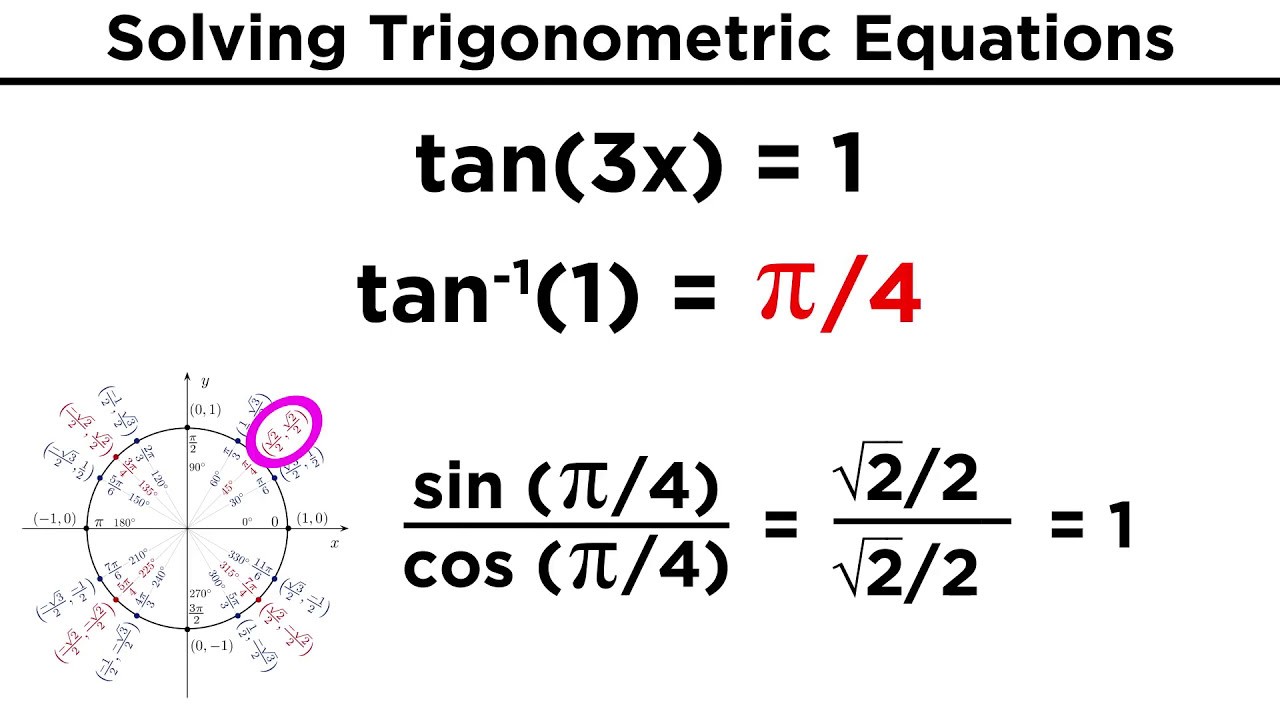
🔍 Finding Solutions for Tangent of Tripled Angle
This section discusses solving for the tangent of a tripled angle, specifically tan(3x) = -1. The tangent function is negative in the second and fourth quadrants, corresponding to angles of 3π/4 and 7π/4. The equation 3x is set equal to these angles plus πm, where m is an integer. By dividing by 3, the solutions for x within the range of 0 to 2π are found to be x = π/4 + π/3n and x = 7π/12 + 4π/12n. The paragraph explains that the number of solutions increases with the multiple of the angle, tripling from two to six when going from tan(x) to tan(3x), and provides the solutions for n = 0, 1, and 2, stopping at 23π/12 as it exceeds the 2π limit.
📉 Cosine of Halved Angle and Solution Limitations
The final paragraph addresses the cosine of a halved angle, starting with the equation cos(x/2) = -1/2. The cosine function is negative in the second and third quadrants, with reference angles of 60° or π/3, leading to solutions of 2π/3 and 4π/3. The equation x/2 is set equal to these angles plus 2πn, and by multiplying by 2, the solutions for x are found to be x = 4π/3 + 2πn and x = 8π/3 + 4πn. The paragraph then evaluates these solutions for different values of n, concluding that only x = 4π/3 is a valid solution within the 0 to 2π range. It also explains that the number of solutions for cosine equations increases with the halving of the angle, but in this case, there is only one valid solution.
Mindmap
Keywords
💡Trigonometric Equation
💡Sine Function
💡Quadrant
💡Period
💡Tangent Function
💡Reference Angle
💡Solution Set
💡Multiple Angles
💡Cosine Function
💡Range
💡Common Denominator
Highlights
Solving trigonometric equations with multiple angles requires identifying the sine values that equal one-half within the range of zero to two pi.
Sine of 30 degrees (pi/6) and sine of 150 degrees (5pi/6) are both equal to one-half, indicating the angles where sine is positive in quadrant two.
The general solution for 2x when sine(2x) equals one-half is 2x = pi/6 + 2pin or 2x = 5pi/6 + 2pin, where n is an integer.
Dividing the general solution by two gives x = pi/12 + pin and x = 5pi/12 + pin, providing specific values for x.
For n=0, x can be pi/12 or 5pi/12, which are the initial solutions for the sine equation.
When n=1, x can be 13pi/12, obtained by adding pi to pi/12, and 17pi/12 by adding pi to 5pi/12.
For n=2, the solution 25pi/12 exceeds the range of 0 to 2pi and is therefore not included.
The number of solutions for the equation with 2x doubles compared to x, resulting in four solutions for the given range.
Finding solutions for tangent(3x) = -1 involves identifying angles in quadrants 2 and 4 where tangent is negative.
Tangent of 135 degrees (3pi/4) and tangent of 315 degrees (7pi/4) are both equal to -1.
The general solution for 3x when tangent(3x) equals -1 is 3x = 3pi/4 + pi*m, simplifying the process.
Solving for x gives x = pi/4 + pi/3*n, with n being an integer.
For n=0, the first solution is x = pi/4; for n=1, x = 7pi/12.
Incrementing n by 1 and solving for x yields subsequent solutions such as 11pi/12 and 15pi/12.
The solution set for tangent(3x) = -1 stops at 23pi/12, as further increments exceed the 0 to 2pi range.
The number of solutions for tangent equations increases with the multiple of x, tripling from tangent x to tangent 3x.
For cosine(x/2) = -1/2, there is only one solution within the 0 to 2pi range, which is 4pi/3.
The general solution for x/2 when cosine(x/2) equals -1/2 is x/2 = 2pi/3 + 2pin or x/2 = 4pi/3 + 2pin.
Multiplying the general solution by two isolates x, yielding x = 4pi/3 + 4pin as the only valid solution.
Transcripts
Browse More Related Video
Solving a trigonometric equation by factoring
Solving Trigonometric Equations By Finding All Solutions
Verifying Trigonometric Identities With Double Angle Formulas

How to solve trigonometric equations with negative angles
Trigonometry - Quadrant rule : Solving Sin θ = a positive value : ExamSolutions
Solving Trigonometric Equations
5.0 / 5 (0 votes)
Thanks for rating: