Graphing Quadratic Functions in Vertex & Standard Form - Axis of Symmetry - Word Problems

The Organic Chemistry Tutor

26 Sept 201647:00

EducationalLearning

32 Likes 10 Comments

TLDRThis video focuses on graphing quadratic functions, including vertex and standard forms. It covers finding the maximum and minimum values, axis of symmetry, and vertex. The video also demonstrates how to graph functions, use tables for plotting points, and solve word problems involving maximum height, time, range, and impact time. Practical examples illustrate how to determine the domain and range, find x and y-intercepts, and write equations in both vertex and standard forms. Techniques for solving quadratic equations and understanding graph behavior are thoroughly explained.

Takeaways

📈 Understanding Quadratic Functions: The video focuses on graphing quadratic functions in both vertex and standard forms, and identifying their key characteristics.
📚 Vertex and Standard Forms: It explains the difference between vertex form (y = a(x-h)² + k) and standard form (y = ax² + bx + c) of quadratic functions, and how to graph them.
🔍 Finding Maximum and Minimum Values: The video teaches how to determine whether a parabola has a maximum or minimum value based on the direction it opens and locate these values.
📐 Axis of Symmetry: It discusses how to find the axis of symmetry (x = h), which is a vertical line that passes through the vertex of the parabola.
🔑 Vertex Identification: The vertex (h, k) of a parabola is identified as the point where the maximum or minimum value occurs, and its x-coordinate is the axis of symmetry.
📉 Positive and Negative x²: The script explains that a positive x² term results in a parabola opening upwards with a minimum value, while a negative x² term results in a parabola opening downwards with a maximum value.
📝 Graphing Techniques: Several techniques for graphing quadratic functions are presented, including using the vertex and plotting points based on the distance from the vertex.
📋 Table Method: An alternative method for graphing is introduced, which involves creating a table of values centered around the vertex and using symmetry to find corresponding points.
🔢 Domain and Range: The video clarifies that the domain of a quadratic function is all real numbers, while the range varies depending on whether the parabola opens upwards or downwards.
⏱ Word Problems: Practical applications of quadratic functions are explored, such as calculating the maximum height of an object thrown into the air and the time it takes to reach the ground.
🔄 Converting Forms: The script shows how to convert a quadratic equation from standard form to vertex form using the method of completing the square, which is useful for graphing and analyzing the function.

Q & A

What is the main focus of the video?
-The video focuses on graphing quadratic functions, including how to graph them in vertex and standard form, finding maximum and minimum values, axis of symmetry, vertex, and solving word problems related to maximum height, time, and range of an object.
How does the direction of the parabola opening affect its vertex value?
-A parabola that opens upward has a minimum value at the vertex, while a parabola that opens downward has a maximum value at the vertex.
What is the vertex form of a quadratic function?
-The vertex form of a quadratic function is given by y = a(x - h)² + k, where (h, k) is the vertex of the parabola.
What is the standard form of a quadratic equation?
-The standard form of a quadratic equation is ax² + bx + c, where a, b, and c are constants, and a is not zero.
How can you find the axis of symmetry for a quadratic function?
-The axis of symmetry is a vertical line that passes through the x-coordinate of the vertex, and it can be found using the equation x = h, where h is the x-coordinate of the vertex.
What is the domain of a quadratic function?
-The domain of a quadratic function is all real numbers, represented as negative infinity to infinity, since there are no restrictions on the x-values.
How can you determine if a quadratic function has a maximum or minimum value?
-A quadratic function has a maximum value if it opens downward (negative coefficient for x²) and a minimum value if it opens upward (positive coefficient for x²).
What is the range of a quadratic function that opens upward?
-For a quadratic function that opens upward, the range starts from the minimum y-value (which is the y-coordinate of the vertex) and goes to positive infinity.
How can you find the vertex of a quadratic function given in vertex form?
-The vertex of a quadratic function given in vertex form y = a(x - h)² + k is directly given by the coordinates (h, k).
What is the method to find the vertex of a quadratic function given in standard form?
-To find the vertex of a quadratic function in standard form, use the formula x = -b / 2a, where a is the coefficient of x² and b is the coefficient of x.
How can you find the x-intercepts of a quadratic function?
-To find the x-intercepts of a quadratic function, set y to zero and solve the resulting equation for x. The solutions are the x-intercepts.
What is the process to find the y-intercept of a quadratic function?
-To find the y-intercept, set x to zero in the quadratic equation and solve for y. The resulting value is the y-intercept.
How can you convert a quadratic equation from vertex form to standard form?
-To convert a quadratic equation from vertex form y = a(x - h)² + k to standard form, you need to expand the equation by squaring the binomial and simplifying.
What is the significance of the vertex in solving word problems related to quadratic functions?
-The vertex is significant in word problems as it can represent key points such as maximum or minimum values, which can be used to solve for things like the maximum height an object reaches or the time it takes to reach a certain point.
How can you find the time it takes for an object to reach its maximum height in a quadratic word problem?
-In a quadratic word problem, the time it takes for an object to reach its maximum height can be found by determining the x-coordinate of the vertex, which represents time in such contexts.
What is the method to find the maximum height of an object thrown upwards from a quadratic equation?
-To find the maximum height, plug the time value (x-coordinate of the vertex) into the quadratic height equation to get the corresponding y-coordinate, which represents the maximum height.
How can you determine the time it takes for an object to hit the ground using a quadratic equation?
-To determine the time it takes for an object to hit the ground, set the height (y-value) of the quadratic equation to zero and solve for the time (x-value).
What is the process to write the equation of a quadratic function if you are given its graph?
-If given the graph of a quadratic function, you can write the equation by identifying two points (such as the vertex and y-intercept or x-intercepts) and using them to determine the coefficients in the vertex form or standard form of the equation.
How can you complete the square to convert a quadratic equation from standard form to vertex form?
-To convert a quadratic equation from standard form to vertex form by completing the square, you need to focus on the x terms, factor out the coefficient of x², add and subtract the square of half the coefficient of x, and then adjust the equation to maintain equality.

Outlines

00:00

📚 Introduction to Graphing Quadratic Functions

This paragraph introduces the topic of graphing quadratic functions, focusing on how to graph them in vertex and standard forms. It explains the concepts of maximum and minimum values, axis of symmetry, and vertex identification. The importance of understanding the difference between positive and negative x-squared terms is highlighted, with examples provided to illustrate how a parabola opens upward or downward. The vertex form and standard form of quadratic functions are defined, and a step-by-step method for graphing a quadratic function by identifying the vertex and using it to find additional points on the graph is discussed.

05:01

📈 Graphing Quadratics and Finding Domain & Range

The second paragraph delves into the specifics of graphing quadratic functions, emphasizing the process of finding the domain and range of these functions. It explains how the domain of a quadratic function is always all real numbers, while the range varies depending on whether the parabola opens upward (minimum value) or downward (maximum value). The paragraph provides examples of graphing quadratic functions with vertex form and standard form equations, demonstrating how to find the vertex, axis of symmetry, and how to use the vertex to determine the minimum or maximum value. It also discusses how to calculate the x and y-intercepts and how to represent the domain and range correctly.

10:01

📉 Understanding Vertex Form and Graphing Examples

This paragraph continues the discussion on graphing quadratic functions, specifically focusing on the vertex form. It explains how to identify the vertex and use it to graph the function, even when the function is not in vertex form. The paragraph provides a detailed example of graphing a quadratic function that has been shifted to the left and down, demonstrating how to find the vertex, axis of symmetry, and how to determine the direction in which the parabola opens. It also covers how to find x and y-intercepts and the range of the function, reinforcing the concepts introduced earlier.

15:10

🔍 Advanced Graphing Techniques and Word Problems

The fourth paragraph introduces advanced graphing techniques for quadratic functions, including the use of the vertex form to find the axis of symmetry and the minimum or maximum values. It also presents a word problem involving a ball thrown upward, which requires understanding the maximum height reached and the time taken to get there. The paragraph explains how to manipulate the quadratic equation to find the vertex and solve for the time it takes for the ball to hit the ground, emphasizing the practical application of graphing quadratic functions.

20:12

📘 Graphing Quadratics from Standard Form

This paragraph discusses how to graph quadratic functions when given in standard form. It explains the process of converting the standard form equation to vertex form by completing the square, which allows for easier graphing. The paragraph provides a step-by-step example of finding the vertex, x-intercepts, and y-intercept of a quadratic function from its standard form equation. It also demonstrates how to organize the data into a table and use it to graph the function, highlighting the minimum or maximum value and the domain and range of the function.

25:14

📝 Solving for Vertex and Intercepts in Quadratics

The sixth paragraph focuses on the methods for finding the vertex, x-intercepts, and y-intercept of a quadratic function. It explains how to use the midpoint between the x-intercepts to find the x-coordinate of the vertex and how to use the equation x = -b/2a to find the vertex's x-coordinate. The paragraph also describes an alternative method for finding the vertex by completing the square and converting the equation from standard form to vertex form. It provides examples of organizing the data into a table and graphing the function, including determining the axis of symmetry, domain, and range.

30:14

🚀 Applying Quadratics to Real-World Problems

This paragraph presents a real-world problem involving a ball thrown upward from a cliff, demonstrating how to apply quadratic functions to solve it. It explains how to find the height function, determine the time it takes for the ball to reach its maximum height, and calculate the maximum height itself. The paragraph also discusses how to find the time it takes for the ball to hit the ground after being thrown, using the quadratic equation. It provides a detailed explanation of the steps involved in solving these problems, emphasizing the practical use of quadratic functions.

35:17

📐 Writing Quadratic Equations from a Graph

The eighth paragraph explains how to write the equation of a quadratic function when given its graph and certain points. It describes the process of using the vertex form of the equation and the additional point, which could be an x-intercept or y-intercept, to find the value of 'a' in the equation. The paragraph demonstrates how to convert the vertex form to standard form by expanding the squared term and combining like terms. It also provides an example of writing the equation when given x-intercepts and a y-intercept, showing how to factor the equation and complete the square to find the vertex form.

40:18

🎯 Mastering Quadratic Functions and Solving Word Problems

The final paragraph wraps up the video by summarizing the key points covered on quadratic functions. It highlights the ability to find domain and range, x and y intercepts, axis of symmetry, maximum and minimum values, and to solve word problems related to quadratic functions. The paragraph emphasizes that the viewer has now mastered these concepts, thanks to the comprehensive explanation provided throughout the video, and it concludes with a positive note, encouraging the viewer to continue exploring these mathematical topics.

Mindmap

Keywords

💡Graphing Quadratic Functions

Graphing quadratic functions is the process of visually representing the mathematical relationship described by a quadratic equation on a coordinate plane. The video focuses on this concept, teaching viewers how to plot and analyze the shape, orientation, and key features of parabolas. It is central to understanding the behavior of quadratic equations, as it helps visualize the maximum or minimum values these equations can attain.

💡Vertex Form

Vertex form is a way of expressing a quadratic function where the equation is written in terms of the vertex of the parabola. The script mentions that the vertex form is 'y = a(x - h)^2 + k', where (h, k) represents the vertex coordinates. This form is particularly useful for quickly identifying the axis of symmetry and the vertex of the parabola, which are key to graphing and analyzing quadratic functions.

💡Standard Form

Standard form refers to the general representation of a quadratic equation as 'ax^2 + bx + c', where a, b, and c are constants, and a is not equal to zero. The video script explains how to graph a quadratic function when it is given in this form and how to convert it into vertex form to find the vertex and axis of symmetry.

💡Axis of Symmetry

The axis of symmetry is a vertical line that passes through the vertex of a parabola, representing the point around which the two halves of the parabola are mirror images of each other. The script explains that the equation for the axis of symmetry is 'x = h', where h is the x-coordinate of the vertex, and it is crucial for determining the shape and orientation of the graph.

💡Vertex

The vertex of a parabola is the point at which the parabola turns; it is the highest or lowest point on the graph depending on the direction it opens. The script uses the vertex to demonstrate how to find the axis of symmetry and to determine whether the parabola has a maximum or minimum value.

💡Minimum and Maximum Values

Minimum and maximum values are the lowest and highest y-values that a parabola reaches. If the parabola opens upwards (positive leading coefficient), it has a minimum value at the vertex. Conversely, if it opens downwards (negative leading coefficient), it has a maximum value at the vertex. The video script explains how to identify these values based on the orientation of the parabola.

💡Domain and Range

In the context of quadratic functions, the domain refers to all possible x-values that the function can take, while the range refers to the set of all possible y-values. The script clarifies that for quadratic functions, the domain is all real numbers (-∞, ∞), but the range depends on whether the parabola opens upwards or downwards, as it will extend from the minimum or maximum value to either positive or negative infinity.

💡X and Y Intercepts

X and Y intercepts are the points where the graph of the quadratic function intersects the x-axis and y-axis, respectively. The script explains how to find these intercepts by setting y to zero for x-intercepts and x to zero for the y-intercept, which helps in graphing the function and understanding its behavior.

💡Word Problem

A word problem in the context of the video is a real-world scenario that is modeled using a quadratic function. The script presents an example involving an object being thrown upward, where the height of the object over time is represented by a quadratic equation. Solving the word problem involves finding the maximum height reached by the object and the time it takes to hit the ground.

💡Completing the Square

Completing the square is a mathematical technique used to rewrite a quadratic equation in vertex form. The script demonstrates this method as an alternative way to find the vertex of a parabola when the equation is given in standard form. It involves creating a perfect square trinomial and adjusting the equation to maintain equality, which helps in graphing and analyzing the quadratic function.

Highlights

Graphing quadratic functions is focused on, including vertex form, standard form, maximum and minimum values, axis of symmetry, vertex, and equation writing.

Positive x squared creates a parabola opening upwards with a minimum value at the vertex.

Negative x squared results in a parabola opening downwards with a maximum value at the vertex.

Vertex form of a quadratic function is introduced with vertex coordinates (h, k).

Standard form of a quadratic equation is ax squared plus bx plus c.

Graphing technique involves moving from the vertex and using the value of 'a' to determine the next points.

Axis of symmetry (AOS) is the x-value of the vertex, written as x = h.

Domain of a quadratic function is all real numbers from negative infinity to infinity.

Range of a quadratic function varies based on the maximum or minimum y-values.

Graphing examples include vertical and horizontal shifts, and finding x and y-intercepts.

Finding the vertex using the formula x = -b / 2a for standard form equations.

Solving for x-intercepts by setting y to zero and solving the resulting equation.

Solving for y-intercepts by setting x to zero and finding the corresponding y value.

Word problem involving a ball thrown upward with a given speed from a cliff, including height function and maximum height calculation.

Writing the equation of a quadratic function from a graph by using vertex form and factoring.

Converting from vertex form to standard form by expanding and combining like terms.

Completing the square method to rewrite the equation in vertex form when given standard form.

Transcripts

Browse More Related Video

Graphing Quadratic Functions Using a Data Table | Algebra

Business Calculus - Section 1.2 - Graphs of Functions

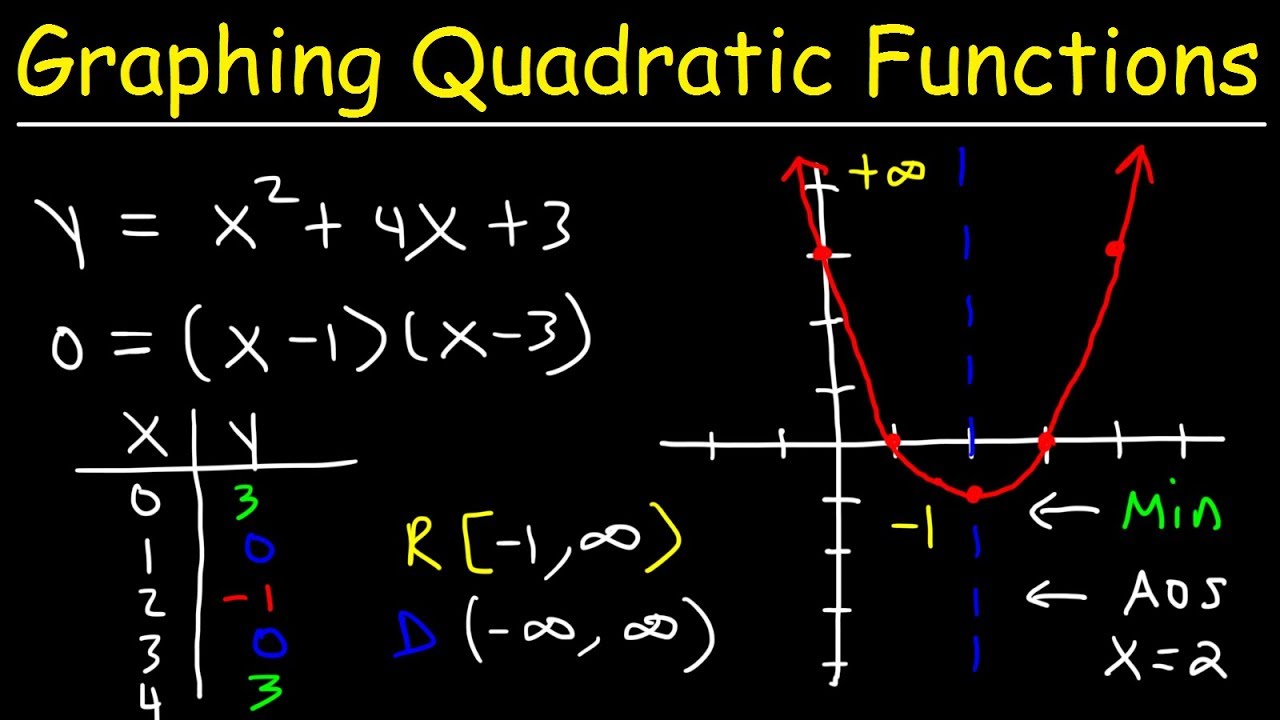
Graphing Quadratic Functions In Standard Form Using X & Y Intercepts | Algebra

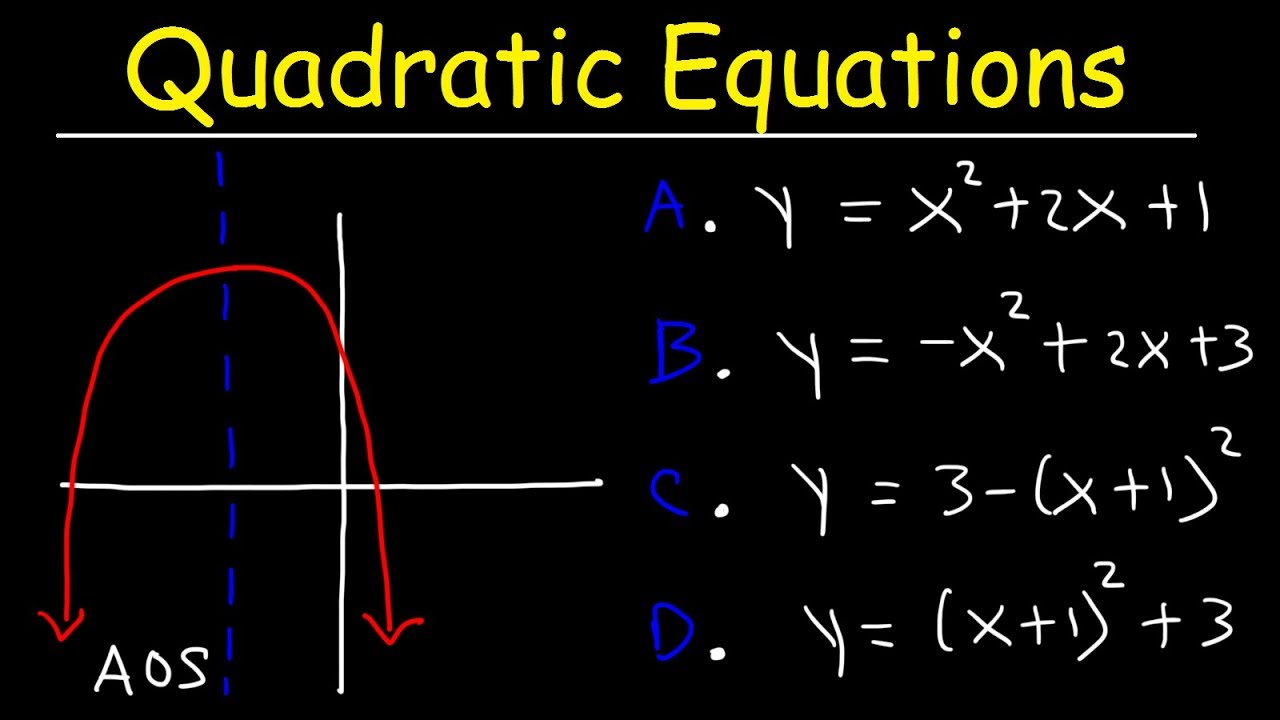
Quadratic Equations Multiple Choice Practice Problems

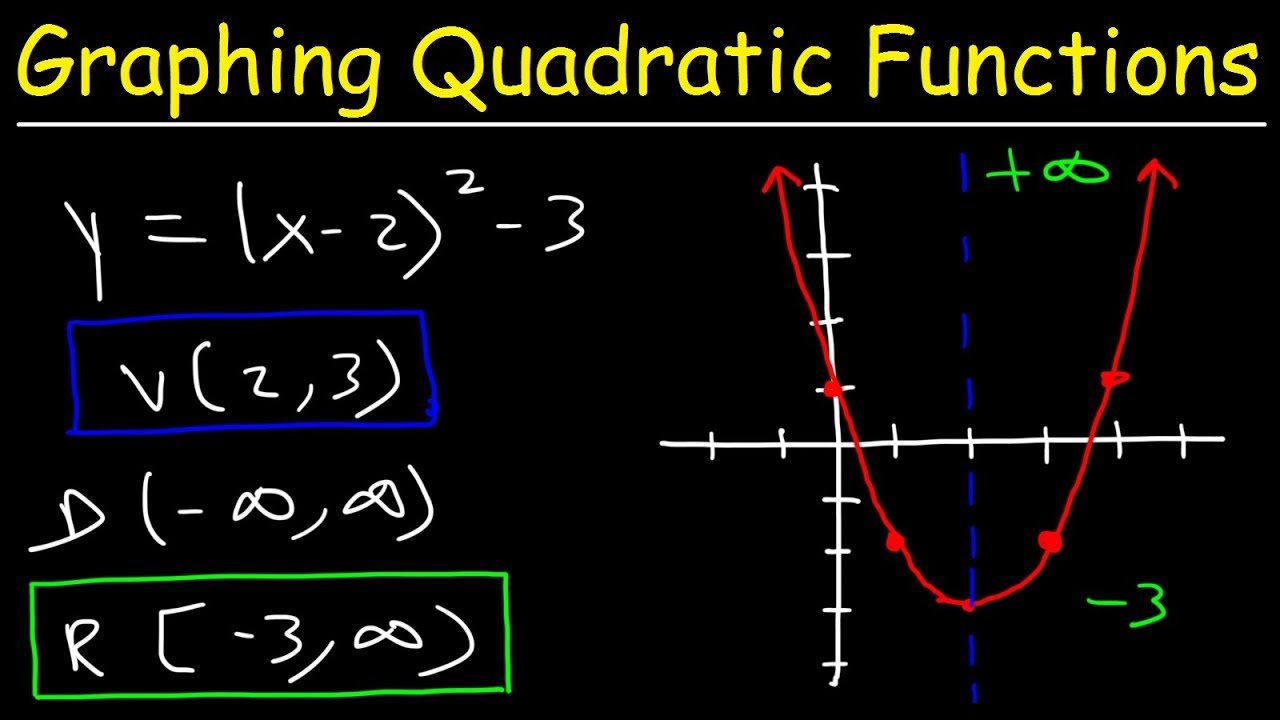
Graphing Quadratic Functions In Vertex Form

How To Graph Equations - Linear, Quadratic, Cubic, Radical, & Rational Functions

Related Tags

Quadratic Functions Graphing Techniques Vertex Form Standard Form Maximum Value Minimum Value Axis of Symmetry Word Problems Mathematics Education Real-world Applications