A pump can fill a pool in 10hrs. Another pump can fill it in 15hrs. Both pumps together will take?
TLDRIn this educational video, John, an experienced math teacher, presents a classic algebra word problem involving two pumps filling a pool in different times. He challenges viewers to solve it and then reveals the correct answer, which is 6 hours when both pumps work together. John explains two algebraic approaches to solve work problems, emphasizing the importance of understanding the problem and using common sense. He also discusses the concept of rates and work in algebra, providing a step-by-step guide to set up and solve equations using the rule of three and the lowest common denominator. The video aims to help students improve their algebra skills, especially in solving word problems, and encourages practice for mastery.
Takeaways
- 📚 The video is a challenge to solve an algebra word problem involving pumps filling a pool, which is a common type of problem that can confuse algebra students.
- 👨🏫 The presenter, John, is a math teacher with decades of experience teaching middle and high school math and offers a math help program at TCM academy.
- 🔢 The problem involves one pump that can fill a pool in 10 hours and another pump that can fill it in 15 hours, and asks how long it will take if both pumps work together.
- 🤔 The incorrect approach of averaging the times (10 and 15 hours) to get the answer is debunked, emphasizing that common sense tells us the combined time must be less than 10 hours.
- 🎯 The correct answer to the problem is revealed to be 6 hours, which is the time it would take for both pumps to fill the pool when working together.
- 📝 John introduces a general formula for solving work problems in algebra, emphasizing the concept of 'one' representing the completion of a job or task.
- 🧩 The formula involves adding the rates of work (as fractions of the job done per unit time) and equating it to the combined rate when multiple entities work together.
- 📉 The process of solving the equation involves finding a common denominator to simplify the algebraic expression and solve for the unknown time variable.
- 🔄 Two methods are presented to structure and solve the problem: one using the rates of work and another using the concept of completing a full job when all parts are combined.
- 👍 The video encourages viewers to practice solving work problems to improve their algebra skills, and it also offers resources for further learning and practice.
- 📈 The video concludes with a reminder of the importance of understanding and practicing algebra, especially for those studying math courses that include algebra.
Q & A
What is the main topic of the video?
-The main topic of the video is solving algebra word problems, specifically a problem involving two pumps filling a pool at different rates.
What is the profession of the person speaking in the video?
-The speaker, John, is a middle and high school math teacher with decades of experience.
What is the specific problem presented in the video?
-The problem is to determine how long it will take for two pumps to fill a pool if one pump can fill it in 10 hours and the other in 15 hours when working together.
What is the correct answer to the pump problem presented in the video?
-The correct answer is that it will take 6 hours for both pumps to fill the pool when working together.
What are the two approaches shown in the video to solve work-related algebra word problems?
-The two approaches shown are using the general formula involving rates and time, and setting up an equation where the sum of the parts of the job done by each pump equals one complete job.
What is the rule of three mentioned in the video?
-The rule of three is to read a problem at least three times before attempting to solve it, ensuring full understanding of the question.
What is the concept of 'one' used in the context of the video?
-In the context of the video, 'one' represents the completion of a job or task, such as filling a pool.
How does the video suggest to simplify the equation 1/10 + 1/15 = 1/x?
-The video suggests multiplying the entire equation by the least common denominator (LCD), which is 30x, to simplify and solve the equation.
What is the alternative equation presented in the video to solve the pump problem?
-The alternative equation is x/10 + x/15 = 1, which also represents the combined work of both pumps filling the pool.
What is the significance of finding the LCD in solving the equation presented in the video?
-Finding the LCD, which is 30 in this case, helps to eliminate the fractions by multiplying the entire equation by the LCD, making it easier to solve.
What type of equations are the ones solved in the video?
-The equations solved in the video are examples of rational equations, which involve rational expressions set equal to each other.
What resources does the speaker offer for those who need help with algebra?
-The speaker offers his Algebra One and Algebra Two courses, as well as a Math Skills Rebuilder course, and suggests checking out his YouTube channel for more practice problems.
Outlines
🔢 Introduction to Algebra Word Problems
This paragraph introduces a challenging algebra word problem involving two pumps filling a pool at different rates. The narrator, John, explains the common misconception of averaging the times and emphasizes the importance of understanding the concept to solve such problems accurately. John also briefly introduces himself and his math teaching program at TCM Academy, encouraging viewers to like and subscribe to his channel for more math content.
📐 Setting Up the Algebra Problem
In this section, John explains the concept of a completed job in algebra word problems, represented by the number one. He describes how to set up an equation using the rates of the two pumps working together to complete the job. John introduces a formula to model this situation algebraically and begins to outline the process of solving the equation step by step.
✏️ Solving the Algebraic Equation
John continues by solving the algebraic equation step-by-step, demonstrating the use of the lowest common denominator (LCD) to clear the fractions. He multiplies the entire equation by the LCD, simplifies, and solves for the unknown variable representing the time both pumps take to fill the pool together. John emphasizes the importance of understanding and practicing these types of problems to improve algebra skills.
🔍 Alternative Approach to Solving the Problem
This paragraph presents an alternative method to solve the same algebra word problem. John explains how to set up a different but equivalent equation and solve it using the concept of a completed job. He demonstrates the process step-by-step, highlighting the similarities and differences between the two approaches. John reassures students that practice and persistence are key to mastering these types of problems.
📚 Additional Resources and Encouragement
John concludes by offering additional resources for students who need more help with algebra and fractions. He mentions various courses available on his website and encourages viewers to check out his YouTube channel for more algebra word problems and other math content. John thanks his audience and wishes them success in their math studies.
Mindmap
Keywords
💡Algebra
💡Word Problems
💡Pump
💡Work Rate
💡Time
💡Rate-Time-Motion Problems
💡Least Common Denominator (LCD)
💡Equation
💡Distributive Property
💡Practice
💡Rational Equations
Highlights
Introduction to a challenge problem involving two pumps with different filling rates working together.
The problem statement: one pump fills a pool in 10 hours, another in 15 hours; find the time if both work together.
Common misconceptions about averaging the times are debunked.
The correct answer is revealed to be 6 hours when both pumps work together.
Introduction to the presenter, John, a math teacher with decades of experience.
Invitation to subscribe and use the math health program for learning assistance.
Explanation of the importance of reading a problem multiple times to understand it fully.
Use of common sense to deduce that the combined time must be less than 10 hours.
Introduction to the general formula for solving work problems in algebra.
Explanation of the concept of 'one' representing the completion of a job in work problems.
Presentation of an algebraic equation to model the work done by the pumps.
The process of solving the equation using the lowest common denominator (LCD) method.
A request for support by subscribing to the YouTube channel and hitting the notification bell.
An alternative approach to solving the problem by setting up a different equation.
Emphasis on the importance of practice in solving algebra word problems.
Recommendations for courses and resources for further learning in algebra.
Final encouragement and well wishes for viewers' mathematical journeys.
Transcripts
Browse More Related Video
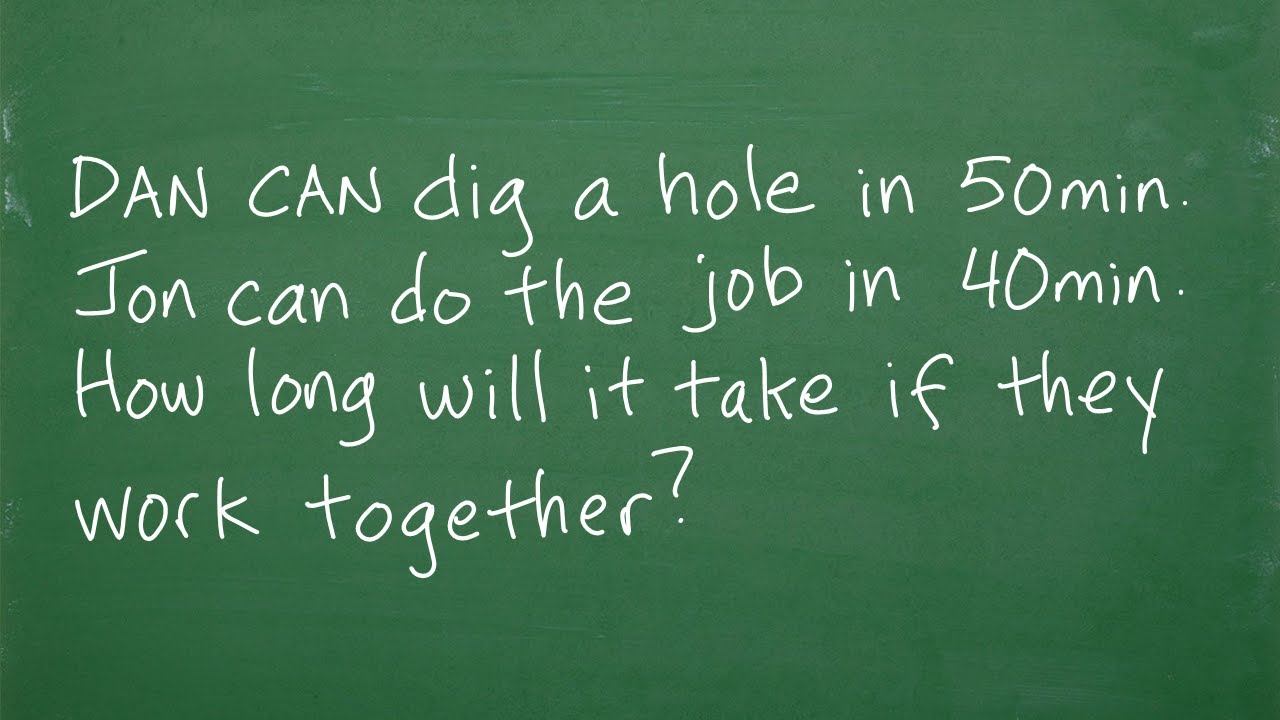
Dan can dig a hole in 50min, Jon can do it in 40min, how long will it take if they work together?

Algebra SYSTEM WORD PROBLEM – Let’s solve it step-by-step...

Quadratic Formula – MOST COMMON MISTAKES!

MANY ARE STRUGGLING ON THIS WORD PROBLEM

How many oz of 5% acid and 20% acid must be mixed to get 10 oz of a solution that is 12.5% acid?
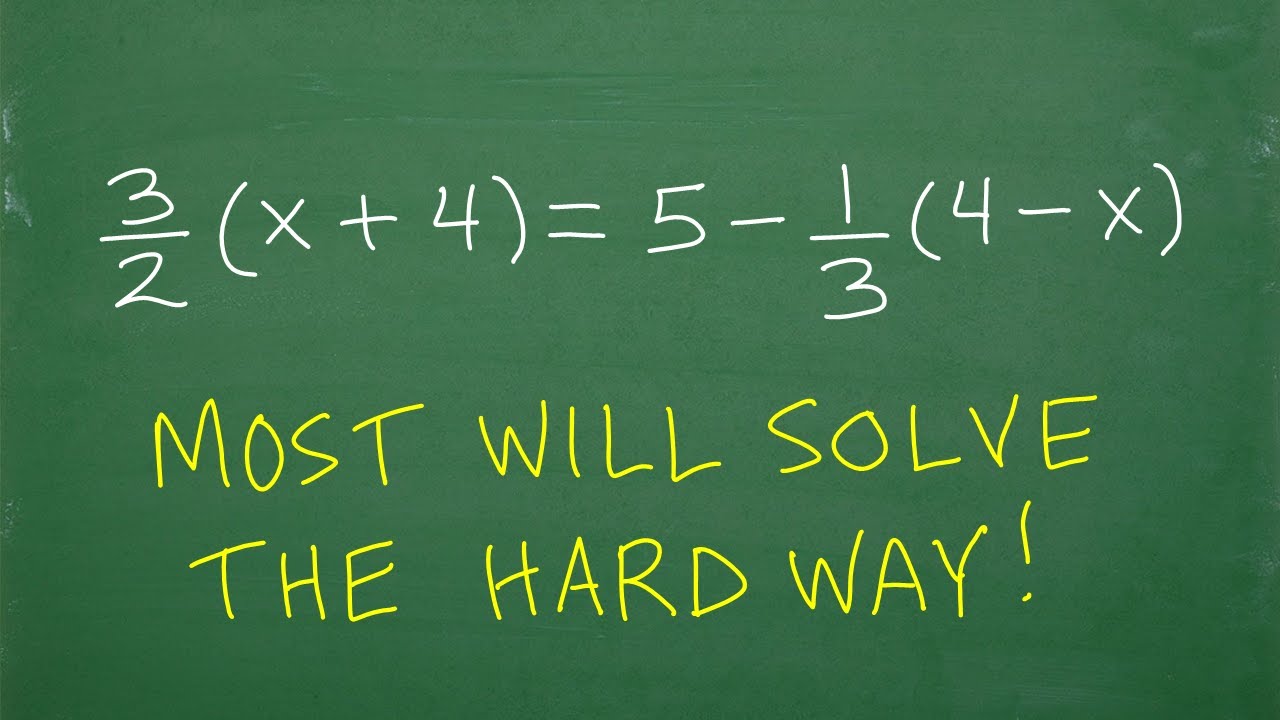
3/2(x + 4) = 5 – 1/3(4 – x), many don’t know the EASY way to solve!
5.0 / 5 (0 votes)
Thanks for rating: