Parabola/Quadratic function 2023 GCE paper 1

Jacob Sichamba Online Math
9 Oct 202308:46
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script guides viewers through solving a quadratic function to find the coordinates of points B and C on its graph. The presenter demonstrates how to set the quadratic equation y = x^2 + 2x - 15 to zero to find the x-intercepts, using factorization to solve for x = 3 and x = -5, resulting in coordinates (3,0) for B and (-5,0) for C. The script then explains how to find the minimum value of y using the formula 4ac - b^2 / 4a, correctly identifying the coefficients and calculating the minimum y value as 16. The video concludes with an offer to help students with various subjects and provides a contact number for further assistance.

Takeaways
  • 📚 The video discusses solving a quadratic function to find the coordinates of points B and C on its graph.
  • 🔍 The given quadratic equation is y = x^2 + 2x - 15, which is set to zero to find the x-intercepts.
  • 📐 Factorization is used to solve the quadratic equation, looking for two numbers that multiply to -15 and add up to 2.
  • 🔢 The factors found are (x + 5) and (x - 3), leading to the solutions x = 3 and x = -5 for the x-intercepts.
  • 📍 Point B's coordinates are determined to be (3, 0), as it is an x-intercept where y = 0.
  • 📉 For point C, the y-intercept is found by substituting x = 0 into the equation, resulting in y = -15, so C's coordinates are (0, -15).
  • 📈 The minimum value of y for the quadratic function is calculated using the formula 4ac - b^2 / 4a.
  • ✅ The values a = 1, b = 2, and c = -15 are identified from the quadratic equation for use in the formula.
  • 🔢 The calculation for the minimum value of y results in 16, indicating the vertex of the parabola is at y = 16.
  • 📝 The video emphasizes the importance of correctly applying the formula and identifying the coefficients a, b, and c.
  • 📞 The video concludes with an invitation to contact for help in various subjects, providing a phone number for further assistance.
Q & A

  • What is the given quadratic function in the script?

    -The given quadratic function is y = x^2 + 2x - 15.

  • What is the purpose of setting the quadratic equation to zero?

    -Setting the quadratic equation to zero helps in finding the x-intercepts, which are the points where the graph of the function intersects the x-axis.

  • What method does the script use to solve the quadratic equation?

    -The script uses the factorization method to solve the quadratic equation.

  • What are the two numbers that the script identifies to factorize the quadratic equation?

    -The two numbers identified are 5 and -3, because 5 * -3 = -15 and 5 + (-3) = 2.

  • How does the script group the terms for factorization?

    -The script groups the terms by combining the x terms (2x and 5x) and the constant terms (-15) separately, then factors out the common x and -3.

  • What are the solutions for x in the quadratic equation according to the script?

    -The solutions for x are x = 3 and x = -5.

  • What are the coordinates of point B as mentioned in the script?

    -The coordinates of point B are (3, 0), as it is an x-intercept where y is zero.

  • What is the process to find the coordinates of point C?

    -To find the coordinates of point C, which is a y-intercept, the script sets x to zero and solves for y, resulting in y = -5.

  • What is the formula used in the script to find the minimum value of y for a quadratic function?

    -The formula used is y = (4ac - b^2) / (4a), where a, b, and c are coefficients from the quadratic equation ax^2 + bx + c.

  • What is the minimum value of y for the given quadratic function according to the script?

    -The minimum value of y for the given quadratic function is 16.

  • What additional subjects does the script mention for learning and exam preparation?

    -The script mentions subjects such as science, mathematics, English, additional mathematics, civic education, and more.

  • How can one get in touch with the service mentioned in the script for further learning?

    -One can get in touch by calling the number 0969 17571 for assistance with learning and exam preparation.

Outlines
00:00
📚 Solving a Quadratic Equation to Find Coordinates B and C

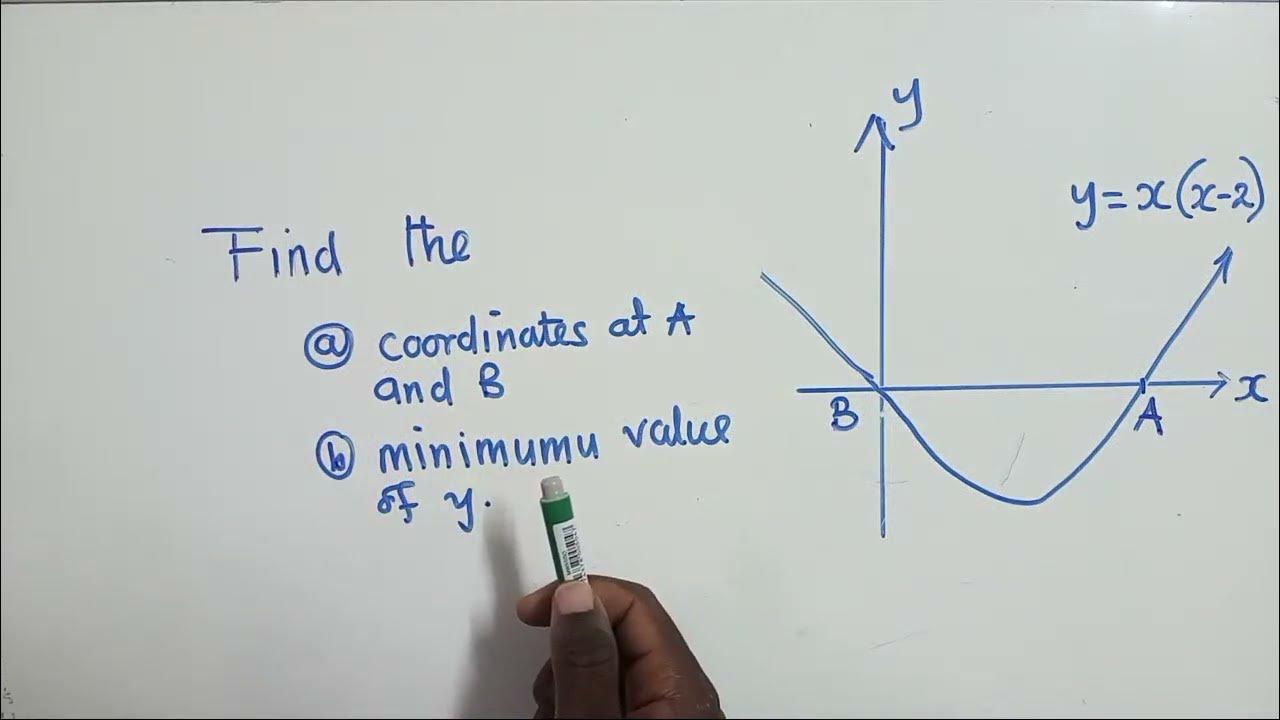
The video script begins with a problem involving a quadratic function, aiming to find the coordinates of points B and C on its graph. The equation given is y = x^2 + 2x - 15. To find the x-intercepts, the equation is set to zero, leading to the factorization method being used to solve for x. The factors that satisfy the conditions (product of -5 and sum of 2) are identified as 5 and -3, resulting in the equation (x + 5)(x - 3) = 0. Solving this gives the x-intercepts x = 3 and x = -5, corresponding to the coordinates (3,0) for point B and (-5,0) for point C, with the understanding that at these points, y is zero since they lie on the x-axis.

05:02
📉 Finding the Minimum Value of a Quadratic Function

The second part of the script addresses the task of finding the minimum value of y for the given quadratic function. The formula used for this purpose is derived from the standard form of a quadratic equation, ax^2 + bx + c. The script identifies the coefficients a, b, and c from the original equation and substitutes them into the formula 4ac - b^2 / 4a to calculate the minimum y value. After correctly identifying a as 1, b as 2, and c as -15, the calculation proceeds with the values plugged into the formula, resulting in the minimum value of y being 16. The script concludes with a reminder to use the correct formula and an invitation for viewers to contact for assistance with various subjects, ending with a contact number and a farewell.

Mindmap
Keywords
💡Quadratic Function
A quadratic function is a polynomial function of degree two. It is generally represented as y = ax^2 + bx + c, where a, b, and c are constants, with 'a' not equal to zero. In the video's context, the quadratic function is y = x^2 + 2x - 15, which is used to find the x-intercepts and the minimum value of y. The script discusses finding the coordinates of points B and C on the graph of this quadratic function.
💡Graph
In mathematics, a graph is a visual representation of data or functions, typically consisting of a set of vertices (points) and edges (lines connecting the points). In the script, the graph represents the quadratic function, and the task is to find specific points on this graph, namely the x-intercepts B and C.
💡Coordinates
Coordinates are pairs of numerical values that determine the position of a point in a plane. In the context of the video, the coordinates for points B and C are sought. For point B, the script describes finding the x-intercept where the y-coordinate is zero, resulting in the coordinates (3, 0). For point C, the y-intercept is found where x is zero, leading to the coordinates (0, y-value).
💡X-intercept
An x-intercept is the point where a graph crosses or touches the x-axis. At this point, the y-value is zero. The script explains how to find the x-intercepts by setting the quadratic equation equal to zero and solving for x, which yields the coordinates (3, 0) and (-5, 0).
💡Y-intercept
A y-intercept is the point where a graph crosses or touches the y-axis. At this point, the x-value is zero. The script mentions finding the y-intercept by substituting x = 0 in the quadratic equation, which helps determine the y-coordinate of point C.
💡Factorization
Factorization is a method used to solve quadratic equations by expressing the quadratic as a product of two binomials. In the script, factorization is used to find the x-intercepts of the quadratic function by finding two numbers that multiply to -15 and add up to 2, resulting in the factors (x + 5) and (x - 3).
💡Minimum Value
The minimum value of a quadratic function is the lowest point on its graph. For a standard quadratic equation in the form of y = ax^2 + bx + c, the minimum value can be found using the formula -b/(2a). The script provides a detailed explanation of how to calculate the minimum value of y for the given quadratic function.
💡Vertex
The vertex of a parabola, which is the graph of a quadratic function, is the point at which the parabola changes direction, either from increasing to decreasing or vice versa. The vertex represents the maximum or minimum point on the graph. The script discusses finding the minimum value of y, which occurs at the vertex of the parabola described by the quadratic function.
💡Coefficient
In a quadratic equation, coefficients are the numerical factors that multiply the variables. The script refers to the coefficients a, b, and c in the quadratic equation y = ax^2 + bx + c, using them to find the minimum value of y with the formula 4ac - b^2 / 4a.
💡Educational Support
The script concludes with an offer of educational support for various subjects, including science, mathematics, English, and civic education. This suggests that the video is part of a series or a program aimed at helping students prepare for exams and improve their understanding of different academic topics.
Highlights

Introduction to solving a quadratic function graph to find coordinates of points B and C.

Equation given: y = x^2 + 2x - 15, which is the basis for finding the coordinates.

Setting the equation to zero to find x-intercepts, which are the coordinates for point B.

Using factorization to solve the quadratic equation, looking for two numbers that multiply to -15 and add up to 2.

Identifying the factors as (5, -3) to rewrite the equation as x^2 + 5x - 3x - 15 = 0.

Grouping terms and factoring out the common x to simplify the equation to (x + 5)(x - 3) = 0.

Solving for x to get the x-intercepts x = 3 and x = -5, corresponding to points on the graph.

Determining the coordinates for point B as (3, 0) based on the x-intercept.

Explaining that point A at (-5, 0) was not requested, focusing only on point B.

Shifting focus to point C, which is a y-intercept where x = 0.

Substituting x = 0 into the equation to find the y-coordinate for point C.

Calculating the y-coordinate for point C as -5, completing the coordinate (0, -5).

Providing the formula for finding the minimum value of y for a quadratic equation.

Identifying the coefficients a, b, and c from the given quadratic equation for the formula.

Plugging the coefficients into the formula to calculate the minimum value of y.

Final calculation showing the minimum value of y as 16.

Emphasizing the importance of using the correct formula for finding the minimum value of y.

Offering additional educational support in various subjects and providing a contact number.

Closing with an encouragement to get in touch for exam preparation assistance.

Transcripts

Browse More Related Video

ECZ Parabola Paper 1

Draw Cubic Graph Grade 12

Equation of a tangent line [IB Maths AI SL/HL]

Business Calculus - Section 1.2 - Graphs of Functions

Calculus Grade 12: Practice

How to find the equation of an ellipse given foci and vertices | @ProfD

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Quadratic FunctionsMathematicsEducationalFactorizationX-InterceptY-InterceptMinimum ValueFormula ApplicationProblem SolvingExam Preparation