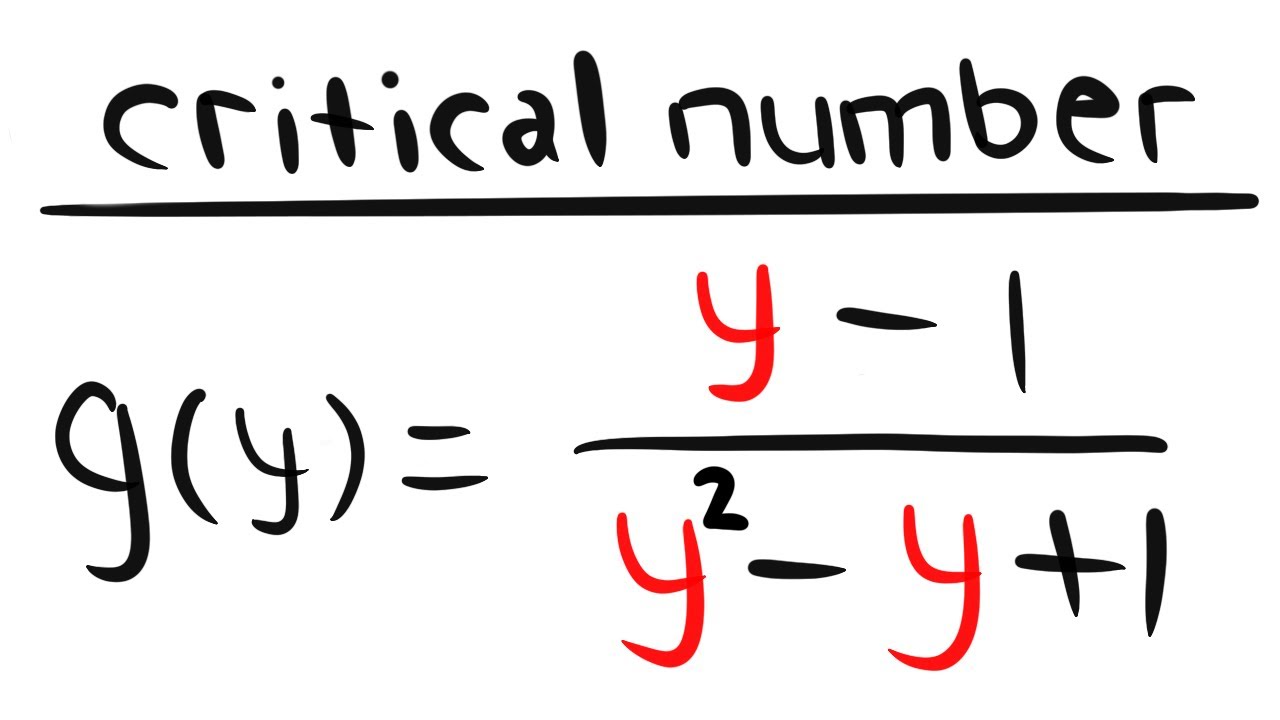Find the critical numbers of 2cos(x)+sin^2(x)
TLDRThis video tutorial guides viewers through the process of finding the critical points of a function, which are the points where the first derivative equals zero or does not exist. The presenter begins by calculating the first derivative of a given function involving sine and cosine terms. They simplify the derivative and set it to zero to find critical numbers. The solution involves factoring and applying trigonometric identities, leading to a set of critical angles based on the unit circle. The video concludes by identifying all possible critical numbers expressed as integer multiples of pi, both positive and negative.
Takeaways
- 📚 The lesson is about finding the equivalence of a function and identifying critical numbers where the first derivative equals zero or doesn't exist.
- 🔍 The first step involves calculating the first derivative of the function, denoted as F'(theta), which includes the derivative of 2cos(theta) and sin^2(theta).
- 📉 The derivative of cos(theta) is -sin(theta), and for sin^2(theta), it's 2sin(theta)cos(theta) using the power and chain rules.
- 🧩 The derivative is assumed to exist everywhere since there are no fractions that could lead to undefined expressions.
- 🔍 To find critical numbers, the derivative is set to zero, and factoring is used to simplify the equation.
- 🔢 The equation simplifies to -2sin(theta)sin(theta) + (1 - cos(theta)) = 0, which is then factored into -2sin(theta) and (1 - cos(theta)).
- 📐 The unit circle is referenced to find angles where sin(theta) = 0 and cos(theta) = 1, which correspond to specific points on the circle.
- 📈 The critical numbers are found by solving for theta, resulting in multiples of π (pi), both positive and negative, including zero.
- 🔑 The final critical numbers are given as theta = nπ, where n is any integer, indicating all possible solutions for the critical points.
- 📝 The process involves a combination of calculus techniques, including differentiation, factoring, and understanding the unit circle.
Q & A
What is the main topic of the video script?
-The main topic of the video script is finding the critical numbers of a function by determining where the first derivative equals zero or does not exist.
What is the significance of critical numbers in calculus?
-Critical numbers are significant in calculus as they are the values of the variable where the derivative is zero or undefined, which often indicate potential local maxima, minima, or points of inflection for the function.
What is the first step in finding the critical numbers of a function?
-The first step in finding the critical numbers of a function is to calculate the first derivative of the function.
What is the derivative of 2cosine(theta) with respect to theta?
-The derivative of 2cosine(theta) with respect to theta is -2sine(theta), since the derivative of cosine(theta) is -sine(theta) and the constant multiplier 2 comes out in front of the derivative.
How is the derivative of sine squared theta found?
-The derivative of sine squared theta is found by using the power rule and the chain rule. It simplifies to 2sine(theta) * cosine(theta), where the power rule brings down the sine(theta) and the chain rule multiplies by the derivative of sine(theta), which is cosine(theta).
Why is it necessary to check for points where the derivative does not exist?
-It is necessary to check for points where the derivative does not exist because these points can also be critical numbers, and they are important for a complete analysis of the function's behavior.
What does it mean for a derivative to exist everywhere?
-For a derivative to exist everywhere means that for every point in the domain of the function, the derivative is defined and there are no discontinuities or undefined points.
How does the video script simplify the equation to find critical numbers?
-The video script simplifies the equation by factoring out common terms, such as -2sine(theta), and setting each factor equal to zero to solve for the critical numbers.
What is the significance of the unit circle in solving for critical numbers?
-The unit circle is significant in solving for critical numbers because it provides a visual and conceptual tool to determine the angles where sine and cosine have specific values, such as zero or one, which are often solutions to the equations derived from setting the derivative equal to zero.
How does the script handle the case of 1 - cosine(theta) equal to zero?
-The script handles the case of 1 - cosine(theta) equal to zero by isolating cosine(theta) and setting it to 1, then referring to the unit circle to find the angles where cosine is equal to 1, which are at 0 and 2pi radians.
What are the general solutions for the critical numbers found in the script?
-The general solutions for the critical numbers found in the script are integer multiples of pi (n*pi), where n is any integer, both positive and negative, indicating the periodic nature of trigonometric functions.
Outlines
📚 Calculus: Finding Critical Numbers of a Function
The first paragraph of the video script introduces the concept of finding critical numbers of a function, which are points where the first derivative is zero or does not exist. The speaker begins by writing down the derivative of a given function, denoted as F'(theta), which involves differentiating terms such as 2cos(theta) and sin^2(theta). The derivative is simplified, and the speaker notes that it will exist everywhere, as there are no fractions that could cause discontinuities. The critical numbers are then found by setting the derivative equal to zero and factoring out common terms. The equation is solved for theta, yielding a series of solutions that are multiples of pi, both positive and negative, indicating the angles at which the function's rate of change is zero.
📘 Critical Numbers: Solving for Specific Angles
In the second paragraph, the speaker continues the discussion on critical numbers by solving the equation further to find specific angles. The solutions are presented as even integer multiples of pi, which include 0, 2pi, 4pi, and so on, both positive and negative. The speaker emphasizes that these are the critical points where the function's derivative equals zero. Additionally, the speaker mentions that for the equation 1 - cos(theta) = 0, the solution is when cos(theta) equals 1, which occurs at theta = 0 and theta = 2pi, corresponding to the points on the unit circle where the x-coordinate is 1. The speaker concludes by summarizing the critical numbers as theta equals n*pi, where n is any integer, indicating the general solution for all critical points of the function.
Mindmap
Keywords
💡Equivalence
💡Critical Number
💡First Derivative
💡Constant Multiple
💡Chain Rule
💡Power Rule
💡Factoring
💡Unit Circle
💡Sine and Cosine Functions
💡Angles
Highlights
Introduction to finding the equivalence of a function and the concept of critical numbers.
Explanation of critical numbers as points where the first derivative equals zero or doesn't exist.
Derivation of the first derivative of the function F'(theta), involving differentiation of cosine and sine functions.
Differentiation of 2cosine(theta) as a constant multiple, resulting in -2sine(theta).
Differentiation of sine^2(theta) using the power rule and chain rule, yielding 2sine(theta)cosine(theta).
Observation that the derivative exists everywhere, as there are no fractions in the expression.
Setting the derivative to zero to find critical numbers, without worrying about points where the derivative doesn't exist.
Factoring out -2sine(theta) from the derivative equation to simplify the equation.
Solving the simplified equation by setting components equal to zero, resulting in multiple potential solutions.
Division by -2 to isolate sine(theta) and cosine(theta) in the equation.
Use of the unit circle to find angles where sine(theta) and cosine(theta) equal zero or one.
Identification of critical numbers as integer multiples of pi, both positive and negative.
Explanation of how to find all solutions for critical numbers by considering the entire range from 0 to 2pi and beyond.
Inclusion of negative angles in the search for critical numbers, extending the range to negative multiples of pi.
Final summary of critical numbers as n*pi, where n is any integer, representing all possible solutions.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: