Finding Critical Values

Sun Surfer Math
2 Mar 202208:52
EducationalLearning
32 Likes 10 Comments

TLDRThis video script introduces the concept of critical values in calculus, which are points where the first derivative of a function is either zero or undefined. The presenter walks through several examples to illustrate how to find these critical points. In the first example, the derivative is 6x - 12, and the critical value is found at x = 2. The second example involves the derivative 6x^2 - 24, with critical values at x = ±2. The third example's derivative is 12x^3 - 12x^2, leading to critical values at x = 0 and x = 1. A more complex example with a derivative involving a cube root and division by x results in critical points at x = 0 and approximately x = ±0.221. The video also covers cases with radicals, where the derivative involves square roots and the critical points are found where the derivative is undefined, such as at x = 0 for the function with a square root. The script effectively demonstrates the process of identifying critical values, which are essential for understanding the behavior of functions and their extrema.

Takeaways
  • 📌 A critical value is a point where the first derivative of a function is either equal to zero or undefined.
  • 🔍 To find critical values, first calculate the first derivative of the function.
  • ✅ Look for points where the derivative equals zero, which indicates a potential critical value.
  • ⚠️ Check for undefined points in the derivative, often caused by division by zero.
  • 🔢 For functions without division, the derivative is defined everywhere, so focus on where it equals zero.
  • 💡 Factor out common terms in the derivative to simplify the equation and find critical values.
  • 🛑 Remember to consider both positive and negative roots when solving for critical values.
  • 📊 In more complex functions, use algebraic manipulation to find critical values, including factoring and taking roots.
  • 🚫 Division by zero is a clear indicator of an undefined derivative and thus a critical value.
  • 🧮 For functions with radicals, change the expression to a form that makes differentiation simpler.
  • 🔑 The process of finding critical values involves setting the derivative equal to zero and solving for x, accounting for undefined cases.
Q & A

  • What is a critical value in the context of calculus?

    -A critical value is a value of the independent variable (usually denoted as 'x') at which the first derivative of a function is either equal to zero or undefined.

  • How does the first derivative being equal to zero indicate a critical value?

    -When the first derivative equals zero, it suggests a potential change in the function's behavior, such as a local maximum or minimum, which is why these points are considered critical.

  • What is the significance of the first derivative being undefined?

    -An undefined first derivative often occurs at points where the function is not differentiable, such as sharp corners or vertical tangents, making these points critical in the analysis of the function.

  • In the first example, how is the first derivative found?

    -The first derivative is found by differentiating the given function. In the first example, the derivative is 6x - 12, obtained by applying the power rule of differentiation.

  • Why is there no division by zero in the first derivative of the first example?

    -There is no division by zero in the first derivative of the first example because the derivative, 6x - 12, does not involve any fractions that could lead to division by zero.

  • How many critical values are found in the first example?

    -In the first example, there is one critical value found at x = 2, which is determined by setting the first derivative equal to zero.

  • What is the process to find critical values when the first derivative is a quadratic expression?

    -To find critical values when the first derivative is a quadratic expression, you set the derivative equal to zero and solve for 'x'. This may involve factoring or using the quadratic formula.

  • How does the presence of a cube root in the function affect the critical values?

    -The presence of a cube root in the function introduces the possibility of division by zero, which can lead to an undefined first derivative. This occurs when the variable in the denominator is zero, which is a critical value.

  • In the problem involving a cube root, how many critical points are there?

    -In the problem involving a cube root, there are three critical points. One is where the derivative is undefined (x = 0), and the other two are found by setting the derivative equal to zero and solving for 'x'.

  • What is the role of the chain rule in finding the derivative of a function with a radical?

    -The chain rule is used to find the derivative of a composite function, such as one involving a radical. It allows you to differentiate the outer function (the radical) and then multiply by the derivative of the inner function (what's inside the radical).

  • What is the critical value found when the derivative involves a square root?

    -When the derivative involves a square root, the critical value is found at x = 0, where the derivative is undefined due to division by the square root of zero.

Outlines
00:00
📌 Introduction to Critical Values

The video begins by introducing the concept of critical values, which are points where the first derivative of a function is either zero or undefined. The presenter explains that these values are significant for analyzing the behavior of a function. The first example involves finding the first derivative, which is 6x - 12, and determining that it is defined everywhere since there's no division by zero. The critical value is found by setting the derivative equal to zero, resulting in x = 2. The second example involves the derivative 6x^2 - 24, which also has no undefined points and yields two critical values, x = ±2. The third example has the derivative 12x^3 - 12x^2, leading to critical values at x = 0 and x = 1 after factoring and solving for zero. The fourth example, which includes a division by zero at x = 0, results in a critical value there and two more found by solving the equation 4x^(-1/3) - 30x = 0, leading to three critical points in total. The fifth example involves a radical function, with the derivative 4/√x, which is undefined at x = 0, making it a critical point. No other critical points are found by setting the derivative to zero, resulting in just one critical value at x = 0.

05:03
🔍 Finding Critical Values with Radical Functions

The second paragraph continues the discussion on finding critical values but focuses on a function involving a radical expression. The derivative of the given function is transformed into 4x^(-1/2), which is undefined at x = 0, thus identifying a critical point there. The derivative is then set to zero, but no solution is found, indicating there are no additional critical points from this step. However, the presenter notes that division by zero would occur at x = 2, which introduces another critical value. The final example involves the derivative 2(x - 2)^(-1/2), which, when set to zero, does not yield a solution. Nonetheless, the potential for division by zero at x = 2 is identified, which provides a critical value at this point. The paragraph concludes by emphasizing the process of finding critical values, which involves calculating derivatives and identifying points of undefined behavior or where the derivative equals zero.

Mindmap
Keywords
💡Critical Value
A critical value is a value of the independent variable (often denoted as 'x') at which the derivative of a function changes its sign, indicating a potential local maximum or minimum. In the video, critical values are identified as points where the first derivative is either zero or undefined. They are crucial for analyzing the behavior of functions, particularly in optimization problems and understanding the function's local extrema.
💡First Derivative
The first derivative of a function, often denoted as f'(x) or df/dx, measures the rate at which the function's value changes with respect to changes in its input. It is a fundamental concept in calculus and is used in the video to find critical points by setting the first derivative equal to zero or checking for undefined values, which can occur at points of discontinuity.
💡Undefined Derivative
A derivative is considered undefined at a point where the function itself is not differentiable, often due to a sharp corner, vertical tangent, or division by zero. In the context of the video, the undefined derivative is one of the two scenarios where a critical value might occur. For instance, the script mentions that if there's division by zero in the derivative, it would be undefined at that point.
💡Division by Zero
Division by zero is an operation that results in an undefined or indeterminate value in mathematics. It is a common issue when dealing with fractions or ratios. In the script, it is mentioned as a specific case where the first derivative of a function can become undefined, which is a key consideration when identifying critical values.
💡Local Maximum/Minimum
A local maximum or minimum refers to the highest or lowest point of a function within a particular neighborhood, not necessarily the entire domain. Critical values are significant because they often correspond to local maxima or minima. The video discusses finding critical values as a means to locate these points by analyzing the first derivative of the function.
💡Setting to Zero
In the process of finding critical values, setting the first derivative to zero is a common technique. This is because the first derivative being zero often indicates a change in the slope of the tangent to the curve, which can correspond to a local maximum, minimum, or inflection point. The video demonstrates this by showing how to set the first derivative equal to zero and solve for 'x'.
💡Square Root
The square root operation, denoted as √ or x^(1/2), is used to find a value that, when multiplied by itself, gives the original number. In the video, taking the square root is part of the process to find critical values after setting the first derivative equal to zero, especially when dealing with expressions that are quadratic in form.
💡Factoring
Factoring is the process of breaking down a polynomial into its constituent factors, which are simpler expressions that when multiplied together give the original polynomial. In the context of the video, factoring is used as a method to simplify the derivative and find its zeros, which correspond to critical values.
💡Chain Rule
The chain rule is a fundamental theorem in calculus for deriving the derivative of a composite function. It is used when the derivative of a function involves an inner function. In the video, the chain rule is implicitly mentioned when differentiating expressions involving powers of other functions, such as (x - 2)^(1/2).
💡Cube Root
The cube root of a number is a value that, when raised to the power of three, gives the original number. It is denoted as x^(1/3). The cube root is used in the video when dealing with expressions involving powers of x that are not whole numbers, specifically when finding critical values by setting the derivative equal to zero.
💡Reciprocal Power
A reciprocal power is when a variable is raised to the power of a fraction, which effectively inverts the variable and adjusts its magnitude. In the video, the reciprocal power is used to simplify expressions involving roots and to find critical values by manipulating the equation into a form that can be solved for 'x'.
Highlights

A critical value occurs where the first derivative is equal to zero or is undefined.

The first derivative of the function is found to be 6x - 12, and it is defined everywhere.

Setting the first derivative equal to zero gives x = 2 as the critical value.

For the next example, the first derivative is 6x^2 - 24, with no division by zero, hence defined everywhere.

Solving 6x^2 = 24 yields two critical values, x = ±2.

In the third example, the first derivative is 12x^3 - 12x^2, with no division by zero.

Factoring out 12x^2 from the derivative leads to x - 1 = 0, giving two critical points at x = 0 and x = 1.

For the fourth example, the first derivative involves division by zero at x = 0, making it a critical value.

Setting the derivative equal to zero leads to a complex solution, indicating two more critical points.

The fifth example involves a radical function, with the derivative being 4/√x.

Division by zero occurs at x = 0, making it a critical point.

The derivative of 4(x - 2)^(1/2) is 2(x - 2)^(-1/2), with a critical value at x = 2 due to division by zero.

Critical values are essential for determining the local maxima, minima, and points of inflection of a function.

The process of finding critical values involves setting the first derivative to zero and checking for undefined points.

Factoring is a common technique used to simplify the equation when finding critical values.

The presence of radicals in a function can introduce division by zero as a potential for critical values.

Critical points can be found by taking the square root of both sides of an equation when dealing with quadratic terms.

The concept of critical values is fundamental in calculus, used to analyze the behavior of functions.

The video provides a step-by-step guide on how to find critical values for various types of functions.

Transcripts

Browse More Related Video

Analyzing a function with its derivative | AP Calculus AB | Khan Academy

Absolute Maximum and Minimum Values - Finding absolute MAX & MIN of Functions - Calculus

Finding the Critical Points of a Function

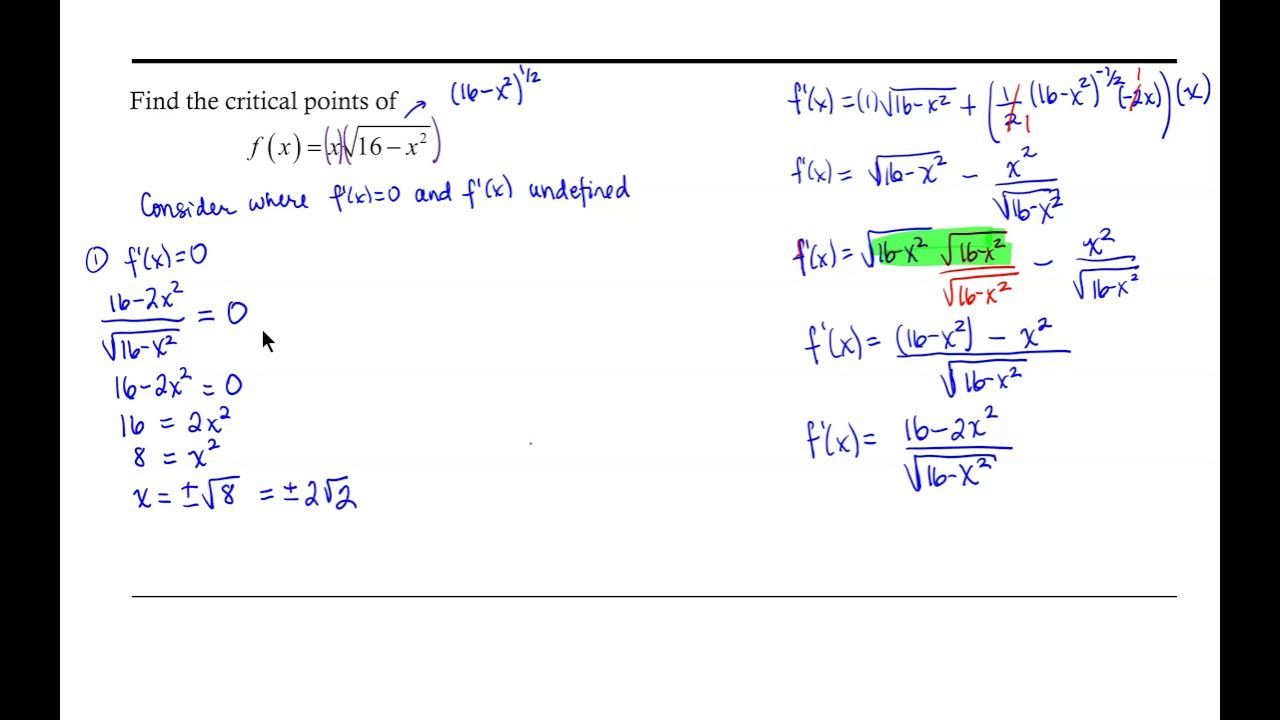
Critical Points: Square Root Function

Maxima , minima and point of inflection

Finding Concavity and Inflection Points

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Critical ValuesCalculusDerivativesMathematicsEducationalFirst DerivativeUndefined PointsZero DivisionRoot FindingAlgebraic FunctionsEducational ContentMath TutorialCritical PointsX Equals ZeroSquared RootsCubed Roots