What is Calculus - Lesson 3 | Differentiation | Don't Memorise
TLDRIn this educational video, Nora's quest to determine the instantaneous speed of a ball at a specific point 'B' introduces the fundamental concept of differentiation in calculus. The script explains how traditional methods for calculating average speed fall short, leading to the exploration of limits and the limit process. By progressively narrowing the time intervals and calculating average speeds closer to point 'B', the video demonstrates how the instantaneous speed can be approximated. This process illustrates the core of differentiation, which is the rate of change of an object's position with respect to time. The video promises to delve deeper into these concepts and their applications in future episodes.
Takeaways
- 🤔 Nora's dilemma is about finding the instantaneous speed of a ball at a specific position 'B', which leads to the concept of differentiation in calculus.
- 📚 Differentiation is based on the idea of limits, which is a fundamental concept in calculus and was previously discussed.
- 🏀 The ball's speed increases as it falls, making the traditional formula for average speed inadequate for finding instantaneous speed.
- 🔢 Nora had calculated the average speed of the ball to be 'fifty centimetres per second' when it reached midway at position 'B'.
- 💡 The average speed of 50 cm/s suggests that the instantaneous speed at any point, including 'B', would be greater than this average as the ball's speed is increasing.
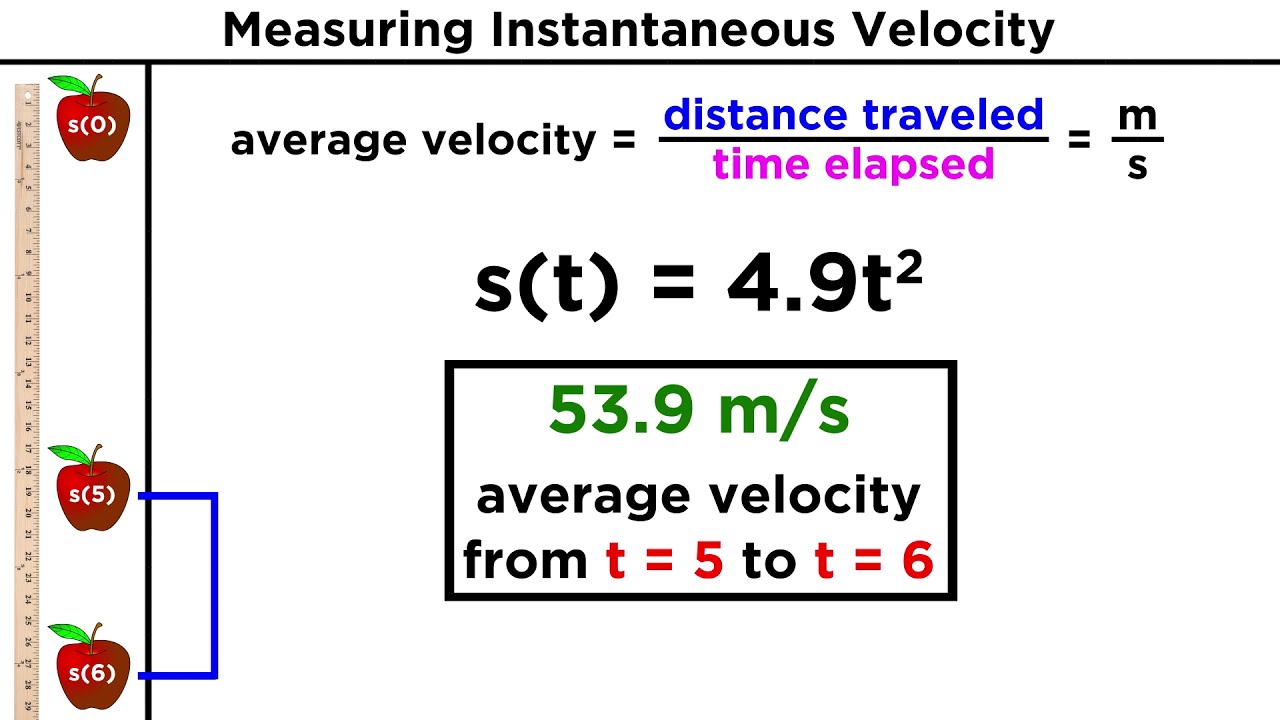
- 📉 The concept of average speed is used to estimate the instantaneous speed by considering the speed between two points and narrowing down the range.
- 📌 By calculating the average speed between points closer to 'B', the estimate for the instantaneous speed at 'B' becomes more precise.
- 📉 Nora uses horizontal bars as an analogy to explain how an average can be deduced from known values and unknown extremes.
- 🔍 The process involves finding average speeds in smaller and smaller intervals around position 'B' to approximate the instantaneous speed.
- 🎯 The limit process is described, where as the time interval approaches zero, the average speed approaches the instantaneous speed.
- 📈 Nora concludes that the instantaneous speed of the ball at 'B' is 100 centimetres per second, illustrating the power of calculus in solving real-world problems.
Q & A
What central idea of calculus is discussed in the video script?
-The central idea of calculus discussed in the video script is differentiation, which is built upon the concept of limits.
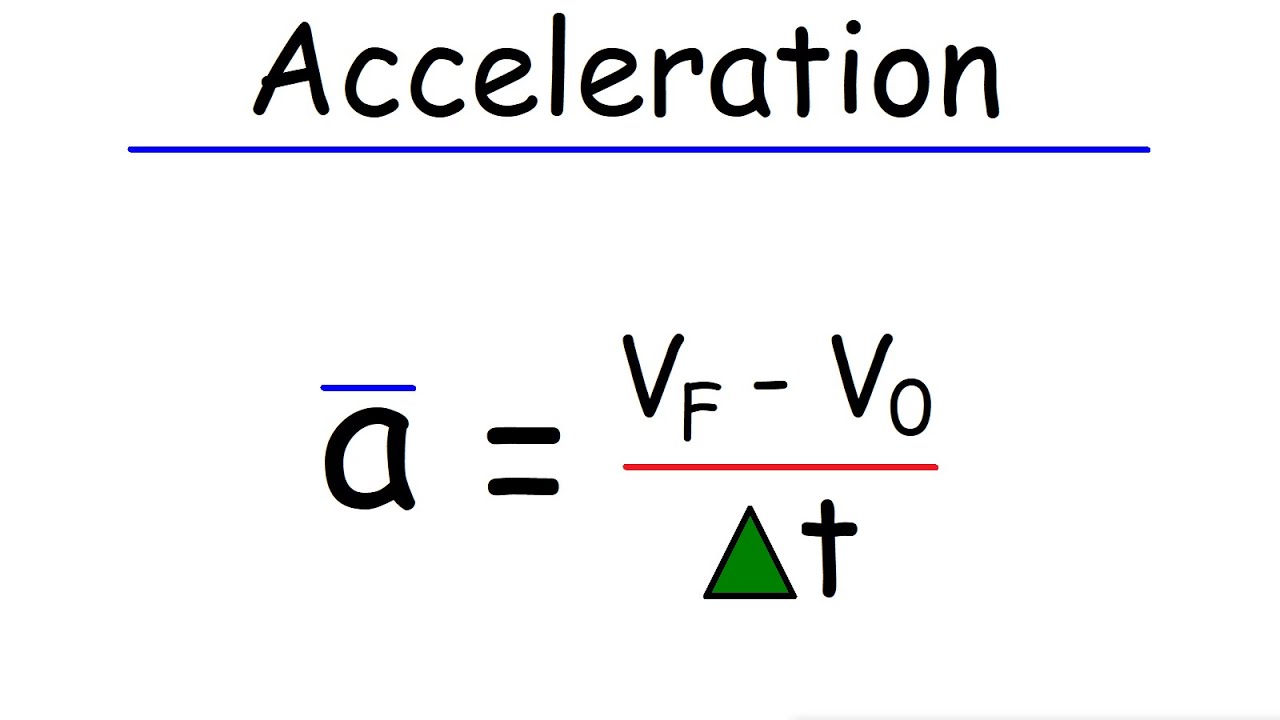
Why can't we use the formula for constant speed to find the instantaneous speed of a ball in free fall?
-We can't use the formula for constant speed because the speed of a ball in free fall is not constant; it increases as the ball falls, making the formula applicable only for average speed, not instantaneous speed.
What does the average speed of fifty centimetres per second tell us about the ball's motion?
-The average speed of fifty centimetres per second tells us that, on average, the ball travels at this speed over the distance it covers. It serves as a ballpark figure for the speed during that duration.
How can we infer the instantaneous speed of the ball at position B if we know the average speed and the initial speed?
-Since the initial speed is zero and the average speed is fifty, we can infer that the instantaneous speed at position B must be greater than fifty because the ball's speed increases from zero as it falls.
What is the significance of the horizontal bars analogy in the script?
-The horizontal bars analogy is used to illustrate the concept that if the average length of bars is 50 and the first bar is 10, the last bar must be greater than 50 to achieve the average. This helps to understand why the instantaneous speed at point B must be greater than the average speed.
What is the average speed of the ball between positions A and C, and what does it indicate?
-The average speed between positions A and C is twenty-five centimetres per second. This indicates that the speed of the ball at any point between A and C is less than or equal to twenty-five centimetres per second.
How does the concept of limits play a role in finding the instantaneous speed?
-The concept of limits is used to approach the problem by considering smaller and smaller time intervals around the instant we are interested in (position B). As these intervals approach zero, the average speed approaches the instantaneous speed.
What does the average speed between positions B and D tell us about the instantaneous speed at B?
-The average speed between positions B and D tells us that the instantaneous speed at B will be less than this average speed because the ball's speed increases continuously as it falls.
How does the process of finding the instantaneous speed at B relate to the concept of differentiation?
-The process of finding the instantaneous speed at B involves looking at the rate of change of the ball's position in very small time intervals. This is the essence of differentiation, which is the mathematical process of finding the rate of change of a quantity with respect to another.
What is the final conclusion about the instantaneous speed of the ball at position B?
-The final conclusion is that the instantaneous speed of the ball at position B is 100 centimetres per second, which is found by taking the limit of the average speeds in smaller and smaller intervals around B.
Outlines
📚 Introduction to Instantaneous Speed and Differentiation
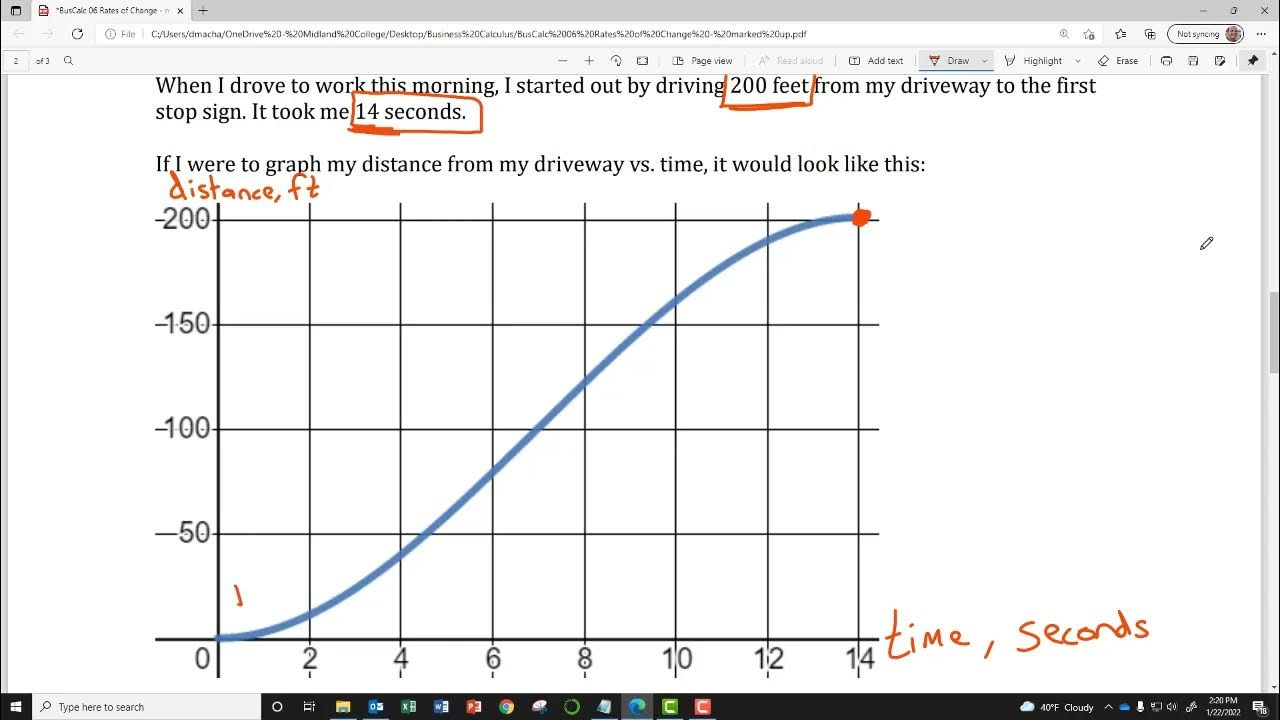
This paragraph introduces the problem of finding the instantaneous speed of a ball at a specific position 'B'. It explains the concept of differentiation, which is central to calculus, and is built upon the concept of limits. The paragraph highlights the difficulty in determining instantaneous speed when dealing with objects that accelerate, as traditional methods only provide average speed. Nora's challenge is presented, along with the idea that the instantaneous speed at 'B' must be greater than the average speed due to the nature of acceleration. The paragraph concludes with an example of calculating average speeds at different points to infer the instantaneous speed at 'B', emphasizing the increasing nature of the ball's speed as it falls.
🔍 Narrowing Down the Range of Instantaneous Speed
The second paragraph delves into the process of narrowing down the range of the ball's instantaneous speed at position 'B'. It starts by establishing that the ball's speed at any point below 'B' will be greater than at 'B', and uses this to infer that the instantaneous speed at 'B' is less than the average speed between 'B' and a point 'D' below it. The paragraph provides specific values for positions 'C', 'D', 'E', and 'F', along with the corresponding times and distances, to calculate average speeds and further refine the range of the instantaneous speed at 'B'. The concept of the limit process is introduced, where the time interval approaches zero, and the average speed approaches the instantaneous speed. The paragraph concludes with the understanding that the instantaneous speed at 'B' is approximately 100 centimeters per second, illustrating the limit process in action.
📈 Understanding Differentiation and Its Applications
The final paragraph explains the concept of differentiation in the context of finding the rate of change of an object's position, which is essentially the instantaneous speed. It emphasizes that differentiation allows for the analysis of how things change and is not limited to speed but can be applied to find the instantaneous rate of change of any quantity. The paragraph hints at the upcoming exploration of differentiation in relation to finding areas of shapes, suggesting a broader application of the concept. It concludes by reinforcing the idea that calculus, and by extension differentiation, is an extension of algebra, stretched to its limits.
Mindmap
Keywords
💡Instantaneous Speed
💡Differentiation
💡Average Speed
💡Limit
💡Acceleration
💡Position 'B'
💡Rate of Change
💡Calculus
💡Zero Over Zero
💡Algebra
Highlights
Nora's problem of finding the instantaneous speed of a ball introduces the central idea of calculus, differentiation.
Differentiation is built upon the concept of limits.
The challenge of finding instantaneous speed when the ball's speed increases over time.
The formula for average speed does not apply to find instantaneous speed at a specific point.
Nora's realization of the ball's average speed being fifty centimetres per second.
Understanding that the ball's instantaneous speed at position B must be greater than the average speed.
The analogy of horizontal bars to explain why the instantaneous speed is greater than the average.
Finding the ball's position and average speed at different points to narrow down the instantaneous speed at B.
The concept that the ball's speed at B is greater than at C, leading to an average speed calculation between B and C.
Determining that the instantaneous speed at B is greater than 75 cm/s based on the average speed between B and C.
The idea of finding the average speed between B and points below it to establish an upper limit for the instantaneous speed at B.
Calculating the average speed between B and D to find that the instantaneous speed at B is less than 112.5 cm/s.
Approaching the instantaneous speed at B by calculating average speeds in smaller intervals.
The discovery that the instantaneous speed at B converges towards 100 cm/s as intervals become smaller.
The limit process in calculus as the time interval approaches zero, revealing the instantaneous speed.
Nora's understanding that calculus is an extension of algebra to its limit.
Differentiation as the process of finding the rate of change of an object's position.
The upcoming exploration of differentiation in relation to finding areas of shapes.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: