CALCULATOR TECHNIQUES: BOARD EXAM REVIEW PART 1

EngineerProf PH
6 Jul 202019:28
EducationalLearning
32 Likes 10 Comments

TLDRThis instructional video delves into calculator techniques, essential for time-saving during exams. It guides viewers through solving equations, including linear and quadratic problems, and systems of equations with multiple unknowns. The tutorial also covers finding terms and sums in arithmetic and geometric progressions, as well as handling complex numbers and limits in calculus. The presenter emphasizes the importance of these 'skull techs' for efficient problem-solving under time constraints, promising further exploration of these techniques in future videos.

Takeaways
  • 🧮 The video focuses on calculator techniques, which are essential for saving time in solving problems, especially in board examinations.
  • 🔢 The first problem demonstrates how to solve an equation for 'x' using a calculator, emphasizing the use of the 'shift' function to input variables and equations.
  • 📈 For the second problem, the video explains how to input and solve exponential equations using a calculator, including setting a limit to find a solution.
  • 🔑 The video introduces the 'quadratic' mode on a calculator to solve quadratic equations by inputting coefficients and constants.
  • 🔍 In solving a system of three equations with three unknowns, the video shows how to use the 'zoom' mode and input coefficients correctly to find the values of 'x', 'y', and 'z'.
  • 📚 The script covers how to use calculator functions for arithmetic progressions, including finding terms and sums of sequences with a common difference.
  • 📉 Similarly, for geometric progressions, the video explains how to use calculator functions to find terms and sums of sequences with a common ratio.
  • 🤔 The video also addresses complex number calculations, including finding products and powers of complex numbers using the calculator's complex mode.
  • 📐 The script touches on converting polar to rectangular form for complex numbers, ensuring the calculator is in the correct mode for such conversions.
  • 📘 Limits are calculated in the script using the calculator's limit function, with examples provided for approaching different values.
  • 👨‍🏫 The importance of mastering calculator techniques is emphasized for efficiency in exams, where time is a critical factor.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is calculator techniques, specifically those useful for saving time when solving problems, particularly in board examinations.

  • Why are calculator techniques important in board examinations?

    -Calculator techniques are important in board examinations because they help save time and allow for the quick solving of problems under time pressure.

  • What is the first problem presented in the video?

    -The first problem presented is to find the value of x in the equation (x + 1) / 3 + (2x / 4) = 47 - x.

  • How does the video suggest solving the first equation for x?

    -The video suggests using the calculator technique by inputting the equation correctly, pressing shift and then equals to find the value of x, which is 25.45.

  • What is the significance of setting a limit when solving equations with a calculator?

    -Setting a limit helps the calculator to solve the equation in a lesser amount of time by narrowing down the range of possible values for the variable.

  • How does the video demonstrate solving a quadratic equation using a calculator?

    -The video demonstrates solving a quadratic equation by using the quadratic mode on the calculator, inputting the coefficients of the variables and constants, and then pressing equals to find the values for x.

  • What is the method for solving a system of three equations with three unknowns as shown in the video?

    -The method involves using the zoom mode on the calculator, setting up the equations with their respective coefficients and constants, and then pressing equals to find the values for the unknowns.

  • How can you find the nth term of an arithmetic progression using a calculator?

    -You can find the nth term of an arithmetic progression by using the linear regression mode on the calculator, inputting the first term, common difference, and the position of the term you want to find.

  • What is the process for finding the sum of an arithmetic progression as shown in the video?

    -The process involves using the summation function on the calculator, inputting the first term, common difference, and the number of terms, and then pressing equals to find the sum.

  • How does the video explain finding the sum of a geometric progression?

    -The video explains finding the sum of a geometric progression by using the summation function in the calculator, inputting the first term, common ratio, and the number of terms, and then pressing equals to find the sum.

  • What calculator mode is recommended for dealing with complex numbers?

    -The complex number mode is recommended for dealing with complex numbers on the calculator.

  • How can you convert from polar to rectangular form using a calculator?

    -You can convert from polar to rectangular form using the calculator by using the appropriate mode and inputting the polar form values, then pressing equals to get the rectangular form.

  • What is the purpose of setting the calculator to Radian mode when dealing with trigonometric functions?

    -Setting the calculator to Radian mode ensures accurate calculations when dealing with trigonometric functions, as it uses radians instead of degrees.

  • How does the video demonstrate finding the limit of a function as x approaches a certain value?

    -The video demonstrates finding the limit by inputting the function into the calculator, using the limit function, and then inputting the value x is approaching to get the result.

Outlines
00:00
🧮 Introduction to Calculator Techniques

This paragraph introduces the topic of the video, which is focused on calculator techniques. The speaker emphasizes the importance of these techniques in saving time when solving problems, particularly during board examinations. The video aims to guide viewers on how to use calculators efficiently to find solutions to various mathematical problems, starting with an equation involving the variable x. The speaker demonstrates the use of the calculator's shift function to solve the equation and find the value of x, which is 25.45. The paragraph sets the stage for further exploration of calculator techniques throughout the video.

05:06
🔢 Solving Equations and Progressions with Calculators

The second paragraph delves into using calculators to solve more complex mathematical problems, such as equations with exponents and arithmetic progressions. The speaker explains how to input expressions correctly into the calculator and use specific calculator functions to find values for variables like x. An example given is solving an equation with an exponent to find the value of x, which is approximately 2.1. The paragraph also covers finding terms and sums in arithmetic progressions, demonstrating the process with specific examples, such as finding the 30th term and the sum of the first 61 odd numbers. The speaker highlights the efficiency of using calculator functions like 'stat' and 'regression' for these types of problems.

10:09
📚 Advanced Calculator Techniques for Sequences and Series

This paragraph continues the theme of using calculators to solve mathematical problems, but it introduces more advanced topics like geometric progressions and summation of series. The speaker provides a step-by-step guide on how to use calculator functions to find the first term of a geometric progression given later terms and how to calculate the sum of the first n terms of a sequence. Examples include finding the sum of a geometric progression with terms 2, 4, 8, 16, and evaluating complex expressions involving square roots of negative numbers. The speaker also touches on the conversion of complex numbers from polar to rectangular form and ends with a brief mention of calculus, specifically finding limits.

15:10
📈 Complex Numbers and Calculus with Calculators

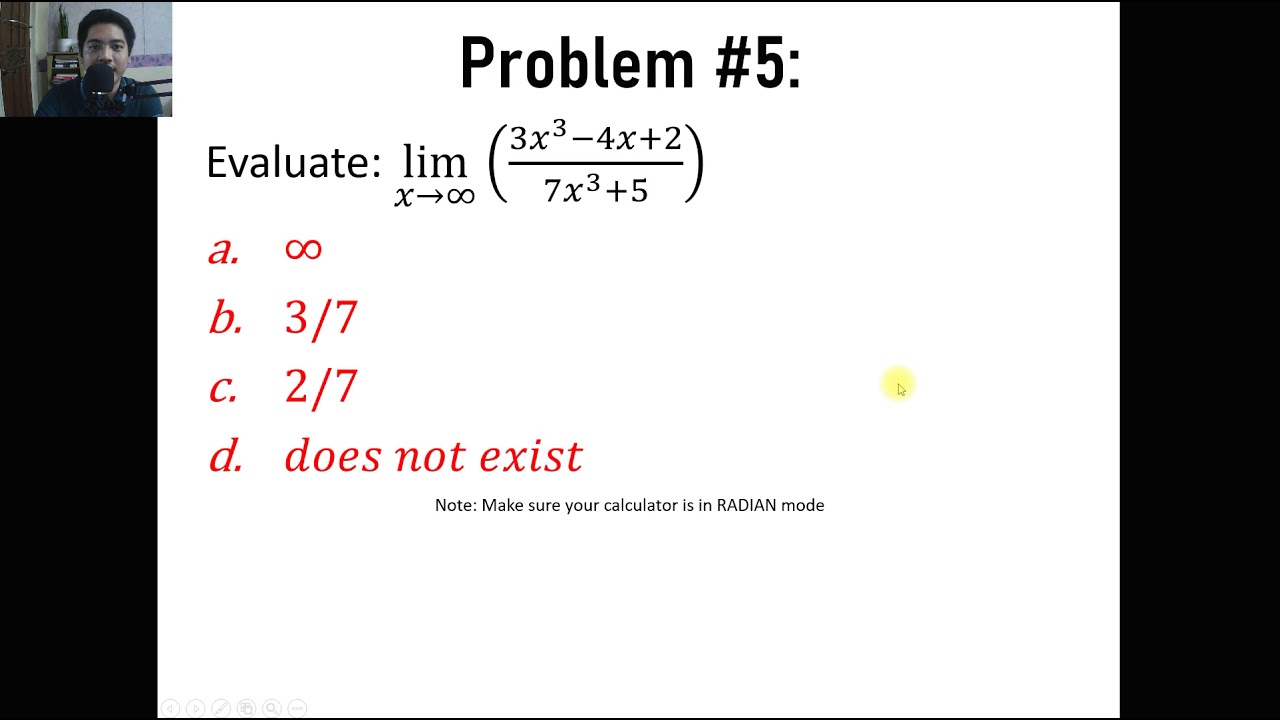
The final paragraph focuses on the use of calculators for complex number operations and calculus problems. The speaker demonstrates how to input and evaluate complex numbers, including raising them to powers and finding products. An example calculation involves finding the value of (1 + i) raised to the 6th power, resulting in -8i. The paragraph also covers the conversion of complex numbers from polar to rectangular form using the calculator. Additionally, the speaker explains how to use the calculator to find limits of functions as x approaches certain values, with examples provided. The video concludes with a reminder of the importance of mastering calculator techniques for time-efficient problem-solving in exams and promises further exploration of these techniques in future videos.

Mindmap
Keywords
💡Calculator Techniques
Calculator Techniques refer to the methods and shortcuts used to efficiently solve mathematical problems using a calculator. In the context of the video, these techniques are crucial for saving time during exams, particularly in board examinations where speed and accuracy are important. The script provides several examples of how to input equations and use specific calculator functions to find values of variables, solve equations, and determine terms and sums in sequences.
💡Equation
An equation is a mathematical statement that asserts the equality of two expressions, typically represented by putting an equal sign '=' between them. In the video, equations are used to demonstrate how to solve for variables like 'x' using calculator techniques. For instance, the script describes how to input and solve an equation to find the value of 'x' in the equation (x + 1)/3 + (2x / 4) = 47 - x.
💡Shift Function
The Shift function on a calculator is used to change the mode or to access more advanced functions. In the script, the Shift function is mentioned as a key part of the calculator technique to solve equations with one unknown directly. It is used in conjunction with other keys like 'ALPHA' and 'CLC' to input and solve equations efficiently.
💡Limit
In mathematics, a limit is the value that a function or sequence approaches as the input or index approaches some value. The concept of limits is central to calculus. The video script discusses finding limits, such as the limit of (x^2 - 16) / (x - 4) as x approaches 4, using calculator techniques to approximate the value that the function approaches.
💡Arithmetic Progression
An Arithmetic Progression (AP) is a sequence of numbers in which each term after the first is obtained by adding a constant difference to the previous term. The video script explains how to find terms and sums within an arithmetic progression using calculator techniques. For example, it shows how to calculate the 30th term and the sum of odd numbers from 1 to 61 using specific calculator inputs.
💡Geometric Progression
A Geometric Progression is a sequence of numbers where each term after the first is obtained by multiplying the previous term by a constant called the common ratio. The script provides an example of finding the first term of a geometric progression given the third and sixth terms, illustrating the use of calculator techniques to solve for unknowns in such sequences.
💡Complex Numbers
Complex numbers are numbers that consist of a real part and an imaginary part, usually written in the form a + bi, where 'i' is the imaginary unit. The video script discusses operations with complex numbers, such as finding the product of complex numbers and converting polar to rectangular form using calculator techniques. This showcases the calculator's ability to handle advanced mathematical concepts.
💡Quadratic Equation
A quadratic equation is a polynomial equation of degree two, typically in the form ax^2 + bx + c = 0. The video script mentions using calculator techniques to solve quadratic equations, such as inputting coefficients and using specific calculator modes to find the roots of the equation.
💡Trigonometric Functions
Trigonometric functions are mathematical functions that relate the angles of a triangle to the lengths of its sides. The script refers to the use of trigonometric functions in limits, such as finding the limit of (1 - cos(x)) / x^2 as x approaches zero. It emphasizes the importance of setting the calculator to Radian mode for accurate trigonometric calculations.
💡Mode
In the context of calculators, 'Mode' refers to the setting or configuration that determines how the calculator will perform certain operations. The video script frequently mentions changing modes, such as switching to 'ALPHA' mode for variable input or 'Radian' mode for trigonometric functions, to perform specific calculator techniques.
Highlights

Introduction to calculator techniques for saving time in problem-solving, especially in board examinations.

Demonstration of solving the first algebraic equation using calculator shift and clear functions.

Explanation of setting a limit to solve equations more efficiently on the calculator.

Guide on solving a second algebraic problem involving exponents and addition.

How to use the quadratic equation mode on the calculator to find the roots of a quadratic equation.

Approach to solving a system of three linear equations with three unknowns using calculator techniques.

Method for finding the value of x and y in a system of two linear equations with two unknowns.

Technique to find the 30th term of an arithmetic progression using calculator functions.

Process for finding the 22nd term in an arithmetic progression given the 4th and 10th terms.

Calculation of the sum of odd numbers from 1 to 61 using arithmetic progression mode on the calculator.

How to find the first term of a geometric progression given the third and sixth terms.

Summation of the first n terms of a geometric progression using calculator functions.

Evaluation of the square root of negative numbers and complex number arithmetic on the calculator.

Conversion of complex numbers from polar to rectangular form using calculator functions.

Finding the limit of a function as x approaches a certain value using calculator techniques.

Importance of calculator techniques in board examinations due to time constraints and problem volume.

Encouragement to familiarize with calculator techniques for efficient problem-solving.

Transcripts

Browse More Related Video

Quadratic and Cubic Equations | Calculator Techniques | Engineering Board Exam | #AbatAndChill

Factorizing Using Casio 570MS Calculator

Solving arithmetic progression with casio calculator @FUNAI_1010 (calculator tricks part 3)

LIMITS CALCULATOR TECHNIQUES - DIFFERENTIAL CALCULUS | ENGINEERING BOARD EXAM REVIEW | YU JEI ABAT

Algebra - How To Solve Equations Quickly!

Arithmetic Progression and Sequence Calculator Techniques | Engr. Yu Jei Abat | AbatAndChill

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculator TechniquesExam PreparationTime ManagementEducational ContentMathematics TipsProblem SolvingEducational VideoStudy SkillsEfficiency ToolsLearning Resources