AP Physics 1: Linear Momentum and Impulse Review
TLDRIn this educational video, Mr. P and his colleagues delve into the concepts of linear momentum, impulse, and collisions, which are crucial for the AP Physics 1 exam. They explain that momentum, the product of mass and velocity, is a vector quantity conserved in collisions. The video distinguishes between elastic and perfectly inelastic collisions, noting that while momentum is conserved in both, kinetic energy is only conserved in elastic collisions. Newton's Second Law is revisited, leading to an exploration of impulse, which is the change in momentum over time, and is central to how safety equipment functions, by increasing the duration of impact to reduce force. The video concludes with a reminder of the importance of understanding these principles for both the exam and real-world applications.
Takeaways
- 📚 Momentum is the product of mass and velocity and is represented by the lower-case letter 'p'.
- 📐 Momentum has the dimensions of kilograms times meters per second and is a vector quantity.
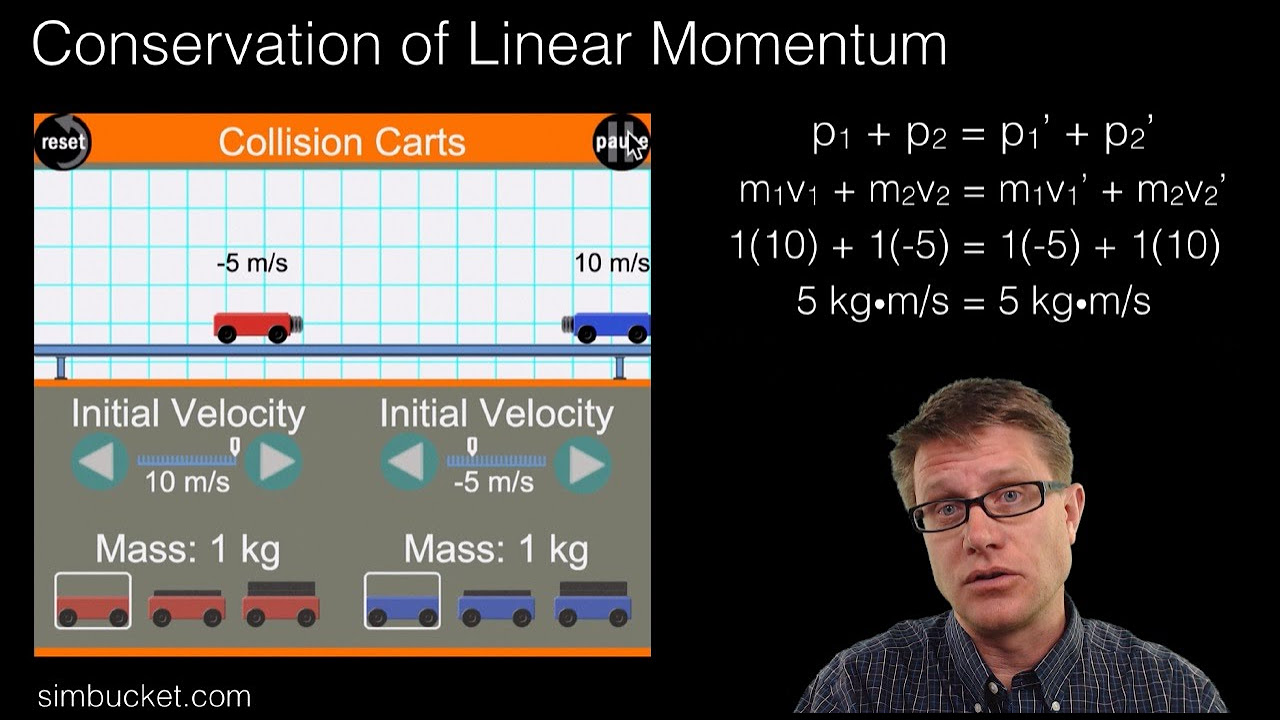
- 🔄 Momentum is conserved during all collisions and explosions, meaning the sum of initial and final momenta are equal.
- 📉 In elastic collisions, both momentum and kinetic energy are conserved, whereas in perfectly inelastic collisions, only momentum is conserved.
- 🚫 Inelastic collisions fall between elastic and perfectly inelastic collisions, conserving momentum but not kinetic energy.
- ⚖️ Newton's Second Law can be restated in terms of momentum, where the sum of forces equals the change in momentum over time.
- 🌟 Impulse is the change in momentum and is equal to the net force times the change in time, represented by the upper-case letter 'J'.
- 🛡 Safety equipment like seat belts, airbags, and helmets work on the principle of impulse to reduce the force of impact during collisions.
- 🔢 Impulse has the same dimensions as momentum, which are Newtons times seconds or kilograms times meters per second.
- 📈 When the impact force is not constant, the average impact force or the area under the force-time graph can be used to calculate impulse.
- 🔗 The video script is a review of linear momentum and impulse for AP Physics 1, with a follow-up video on rotational kinematics.
Q & A
What is the definition of linear momentum?
-Linear momentum is defined as the product of an object's mass and its velocity, and it has the dimensions of kilograms times meters per second.
Is momentum a scalar or a vector quantity?
-Momentum is a vector quantity because it is dependent on the direction of velocity.
What symbol is commonly used to represent momentum?
-The symbol for momentum is a lowercase 'p', which should not be confused with an uppercase 'P' for power or the lowercase Greek letter 'ρ' for volumetric mass density.
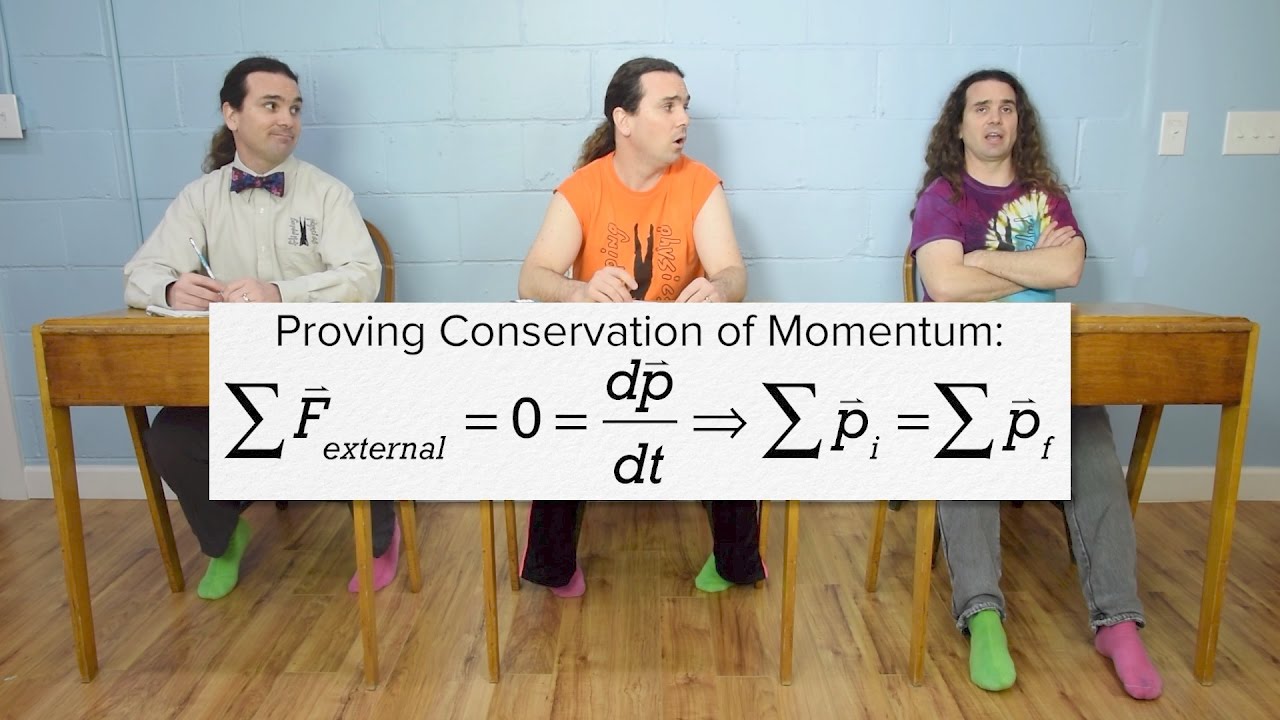
What is the principle of conservation of momentum?
-The principle of conservation of momentum states that the total momentum before a collision or explosion is equal to the total momentum after the event.
How does the conservation of momentum differ from the conservation of energy in terms of identifying initial and final points?
-Unlike conservation of energy, the conservation of momentum does not require identifying specific initial and final points because the initial point is always right before and the final point is always right after the collision or explosion.
What are the two types of collisions mentioned in the script?
-The two types of collisions mentioned are elastic collisions, where both momentum and kinetic energy are conserved, and perfectly inelastic collisions, where momentum is conserved but kinetic energy is not.
What is the difference between elastic and perfectly inelastic collisions?
-In an elastic collision, both momentum and kinetic energy are conserved, and the objects bounce off each other. In a perfectly inelastic collision, the objects stick together after the collision, and while momentum is conserved, kinetic energy is not.
What is the relationship between Newton's Second Law and the concept of impulse?
-Newton's Second Law states that the net force on an object is equal to its mass times its acceleration. By substituting the equation for acceleration with the change in velocity over time, we can derive the concept of impulse, which is the change in momentum over time.
How is impulse related to force and momentum?
-Impulse is equal to the change in momentum, which can also be expressed as the net force times the change in time. It is typically represented by the symbol J.
What is the impulse approximation, and how is it used in collisions?
-The impulse approximation states that during the short time interval of a collision, the force of impact is so much larger than other forces that the net force can be approximated as the force of impact. This allows us to calculate impulse as the change in momentum during the collision.
How do safety devices like airbags and helmets work based on the concept of impulse?
-Safety devices work on the principle of impulse by increasing the time over which a collision occurs, thereby decreasing the force of impact on the body, even though the change in momentum remains the same.
What are the dimensions of impulse, and how do they relate to momentum?
-The dimensions of impulse are Newtons times seconds, which is equivalent to kilograms times meters per second, the same as the dimensions of momentum.
Outlines
🚀 Introduction to Linear Momentum and Collisions
This paragraph introduces the concept of linear momentum, impulse, and collisions, which are key topics for the AP Physics 1 Exam. Momentum is defined as the product of mass and velocity, with units of kilograms times meters per second, and is a vector quantity. The symbol for momentum is a lowercase 'p', which should not be confused with uppercase 'P' for power or the lowercase Greek letter 'ρ' for volumetric mass density. The principle of conservation of momentum is highlighted, stating that the total momentum before and after a collision or explosion remains constant, without the need to identify specific points in time. The paragraph also distinguishes between elastic and perfectly inelastic collisions, with the former conserving both momentum and kinetic energy, and the latter conserving momentum but not kinetic energy. An elastic collision is characterized by objects bouncing off each other, while in a perfectly inelastic collision, objects stick together post-collision. The concept is further explored through Newton's Second Law, leading to the equation for impulse, which is the change in momentum over time, equated to the net force times the change in time, represented by the symbol 'J'. The paragraph concludes with an explanation of how safety equipment like airbags and helmets work based on the principle of impulse, by increasing the time of collision to reduce the impact force on the body.
📏 Dimensions of Impulse and Conclusion
In this paragraph, the dimensions of impulse are discussed, with Billy explaining that impulse is the product of impact force and change in time, measured in Newtons times seconds, which is equivalent to kilograms times meters per second, matching the dimensions of momentum. The conversation confirms that impulse shares the same units as momentum, reinforcing the relationship between the two concepts. The paragraph wraps up with Mr. P thanking the viewers for joining the review of linear momentum and impulse for AP Physics 1 and inviting them to continue learning with the next video on rotational kinematics. Additionally, viewers are directed to the website flippingphysics.com for organized AP Physics 1 review videos and lecture notes, emphasizing the value of the learning experience provided.
Mindmap
Keywords
💡Momentum
💡Impulse
💡Collision
💡Elastic Collision
💡Inelastic Collision
💡Perfectly Inelastic Collision
💡Newton's Second Law
💡Impulse Approximation
💡Force
💡Safety Equipment
Highlights
Good morning and introduction to the topics of linear momentum, impulse, and collisions covered on the AP Physics 1 Exam.
Momentum is defined as the product of mass and velocity, with dimensions of kilograms times meters per second.
Momentum is a vector quantity, indicated by a lower-case 'p' to avoid confusion with other symbols.
Momentum is conserved during all collisions and explosions, with the sum of initial momentums equal to the sum of final momentums.
In two-dimensional motion, two separate conservation of momentum equations are needed for the x and y directions.
Types of collisions are explained: elastic, perfectly inelastic, and inelastic collisions, each with different conservation properties.
Newton's Second Law is connected to momentum through the equation involving mass, change in velocity, and time.
Impulse is defined as the change in momentum, which equals the net force times the change in time.
Impulse approximation is introduced, simplifying the calculation of impulse during collisions.
Safety equipment like airbags and helmets work on the principle of impulse to reduce impact force.
An example of riding a bike off a dock to demonstrate the concept of impulse in a flipping physics video.
Impulse can be calculated using average impact force or graphically by the area under the force-time curve.
Dimensions of impulse are discussed, equating to Newtons times seconds or kilograms times meters per second.
Upcoming review video on rotational kinematics and the availability of organized AP Physics 1 review videos on the website.
Closing remarks, expressing gratitude for learning together and inviting viewers to continue their education with the next video.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: