FINDING THE EQUATION OF THE PARABOLA | GRAPHING | VERTEX AT THE ORIGIN | PROF D

Prof D
30 Mar 202115:40
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video delves into the mathematical concept of parabolas, focusing on their equations and geometric properties. The host introduces a parabola with its vertex at the origin and a focus at (5,0), deriving its equation as y^2 = 20x. The video explains the parabola's orientation, axis of symmetry, and latus rectum. It also covers examples with different orientations and directrix equations, calculating the corresponding equations and properties for each scenario. The host skillfully illustrates how to find the focus, directrix, and latus rectum for parabolas opening to the right, left, and upwards, providing a comprehensive understanding of these shapes.

Takeaways
  • 📚 The video discusses the equation of a parabola with its vertex at the origin (0,0) and how to satisfy given conditions.
  • 📏 The focus of the parabola, with coordinates (5,0), is used to derive the equation y^2 = 4px, where p is the distance from the vertex to the focus.
  • 🔍 The opening direction of the parabola is determined by the sign of 'p'; if 'p' is positive, the parabola opens to the right.
  • 📐 The latus rectum, a line that passes through the focus and is perpendicular to the directrix, is calculated as p, ± 2p for the given examples.
  • 🟣 The directrix is a line that is perpendicular to the axis of symmetry of the parabola, and its equation is given as x = -p for right-opening parabolas.
  • 📈 For a parabola opening to the left, the equation is derived as y^2 = -4px, indicating a negative value of 'p'.
  • 🤔 The length of the latus rectum is given as 8 units for one of the examples, which helps in finding the value of 'p'.
  • 🆙 A parabola that opens upward has its equation in the form x^2 = 4py, where 'p' is positive.
  • ⏫ The focus of an upward-opening parabola is found at (0, p), and the directrix is a horizontal line at y = -p.
  • 🖋️ The video concludes with a prompt for viewers to ask questions or seek clarifications in the comments section.
  • 👋 The presenter, Prof D, signs off with a friendly farewell, indicating the end of the video.
Q & A

  • What is the vertex of the parabola discussed in the video?

    -The vertex of the parabola discussed in the video is at the origin, which is coordinates (0, 0).

  • What is the equation of a parabola with a focus at (5, 0) and opening to the right?

    -The equation of the parabola with a focus at (5, 0) and opening to the right is y^2 = 20x.

  • What is the directrix of the parabola with a focus at (5, 0) and opening to the right?

    -The directrix of the parabola with a focus at (5, 0) and opening to the right is x = -5.

  • What are the coordinates of the vertex and the focus of the parabola that opens to the left?

    -The vertex of the parabola that opens to the left is at the origin (0, 0), and the focus is at (-3, 0).

  • What is the equation of the parabola that opens to the left with a directrix of x = 3?

    -The equation of the parabola that opens to the left with a directrix of x = 3 is y^2 = -12x.

  • What is the length of the latus rectum for a parabola that opens upward with a length of 8?

    -The length of the latus rectum for a parabola that opens upward is given by 4p, where p is the distance from the vertex to the focus. If the length is 8, then 4p = 8, so p = 2.

  • What is the equation of the parabola that opens upward with a latus rectum length of 8?

    -The equation of the parabola that opens upward with a latus rectum length of 8 is x^2 = 8y.

  • What are the coordinates of the focus and directrix for the parabola that opens upward with a latus rectum length of 8?

    -The focus for the parabola that opens upward with a latus rectum length of 8 is at (0, 2), and the directrix is y = -2.

  • What is the standard form of the equation of a parabola with vertex at the origin?

    -The standard form of the equation of a parabola with vertex at the origin and opening to the right is y^2 = 4px, where p is the distance from the vertex to the focus.

  • How does the video script describe the process of finding the equation of a parabola given its focus and directrix?

    -The video script describes the process by first identifying the vertex, then using the given focus and directrix to determine the value of p, and finally substituting this value into the standard form equation of a parabola.

  • What is the latus rectum of a parabola, and how is it related to the parabola's focus and directrix?

    -The latus rectum of a parabola is a line segment that is perpendicular to the directrix and passes through the focus. Its length is given by 4p, where p is the distance from the vertex to the focus.

Outlines
00:00
📚 Introduction to Parabola Equations and Properties

This paragraph introduces the topic of the video, focusing on deriving the equation of a parabola with its vertex at the origin. It discusses the conditions for drawing a parabola, including its focus and directrix. The example provided involves a parabola with a focus at (5,0), and the process of finding its equation, opening direction, and lattice points is explained. The video also covers the concept of the directrix for a parabola opening to the right.

05:01
🔍 Analyzing Parabola with Given Directrix

In this paragraph, the focus shifts to another example, where the directrix of the parabola is given as x - 3 = 0. The video explains how to find the equation of the parabola using the directrix and the standard formula y^2 = 4px. The process of identifying the value of p, the opening direction of the parabola, and the equation of the parabola is detailed. The lattice points and the directrix are also calculated and explained.

10:01
📐 Parabola with Lattice Rectum Length and Direction

The third paragraph deals with a parabola that opens upward and has a lattice rectum length of eight. The video outlines the standard form of the parabola's equation and how to find the value of p using the length of the lattice rectum. It then explains how to determine the focus of the parabola, the lattice points, and the directrix. The process involves solving for p, substituting it into the equation, and sketching the parabola with the identified parts.

15:02
🎓 Conclusion and Viewer Engagement

The final paragraph concludes the video by summarizing the key points covered and inviting viewers to ask questions or seek clarifications in the comment section. The host, Prof D, thanks the viewers for watching and signs off, indicating the end of the educational content.

Mindmap
Keywords
💡Parabola
A parabola is a conic section, the set of all points in a plane that are equidistant from a fixed point (the focus) and a fixed line (the directrix). In the video, the theme revolves around writing the equations of parabolas with vertices at the origin and satisfying certain conditions, such as having a focus at (5,0) or a directrix at x=3.
💡Vertex
The vertex of a parabola is the point where the parabola changes direction. In the context of the video, the vertex is located at the origin (0,0), which is a common reference point for the parabola's position and orientation.
💡Focus
The focus of a parabola is a point used to define its shape. It is one of the two points equidistant from the directrix and any point on the parabola. In the script, examples are given where the focus has coordinates (5,0), which helps in determining the equation of the parabola.
💡Directrix
The directrix is a line that is perpendicular to the axis of symmetry of the parabola and is used to define its shape. In the video, the directrix is mentioned as a horizontal line at x=-5 or x=3, depending on the example, which influences the parabola's orientation.
💡Equation
The equation of a parabola is a mathematical formula that describes its shape and position in the coordinate plane. The video script discusses deriving the equations of parabolas based on their vertices, foci, and directrices, such as 'y^2 = 4px'.
💡Latus Rectum
The latus rectum of a parabola is a line segment that passes through the focus and is perpendicular to the directrix. It is used to find points on the parabola and its length is given by 4p. In the video, the latus rectum is calculated for different parabolas, such as when the length is given as 8.
💡Opening Direction
The opening direction of a parabola refers to the way it opens, either to the left, right, up, or down. In the script, the parabolas are described as opening to the right or upward, which affects the form of their equations.
💡Standard Equation
The standard equation of a parabola is a specific form that describes its position and orientation. The video mentions the standard equation 'y^2 = 4px' for parabolas opening to the right and 'x^2 = 4py' for those opening upward.
💡P-value
In the context of parabolas, the p-value is a parameter that determines the distance between the vertex and the focus or directrix. The script uses the p-value to derive the equations of parabolas and calculate the latus rectum.
💡Conic Sections
Conic sections are curves obtained by intersecting a cone with a plane. Parabolas are one type of conic section, and the video script discusses their properties and equations in detail.
💡Sketching
Sketching in the context of the video refers to drawing the parabola, its focus, directrix, and latus rectum based on the derived equations. It helps visualize the parabola's shape and orientation in the coordinate plane.
Highlights

Introduction to the topic of finding the equation of a parabola with vertex at the origin.

Explanation of how to draw the parabola, its focus, and directrix.

Example A: Focus at (5,0) and the derivation of the parabola's equation.

Use of the standard equation y^2 = 4px to find the parabola's equation.

Determination of the parabola's opening direction based on the value of p.

Calculation of the lattice rectangle for a parabola opening to the right.

Identification of the directrix for a parabola with a given focus.

Example B: Given directrix and deriving the parabola's equation.

Use of the directrix to find the value of p and the parabola's orientation.

Derivation of the parabola's equation when it opens to the left.

Example C: Parabola opening upward with a given lattice rectangle length.

Calculation of p using the length of the lattice rectangle.

Formation of the parabola's equation with a positive p value.

Determination of the focus, lattice rectangle, and directrix for the upward-opening parabola.

Sketching the parabola with the identified elements.

Conclusion of the video with an invitation for questions and feedback.

Transcripts

Browse More Related Video

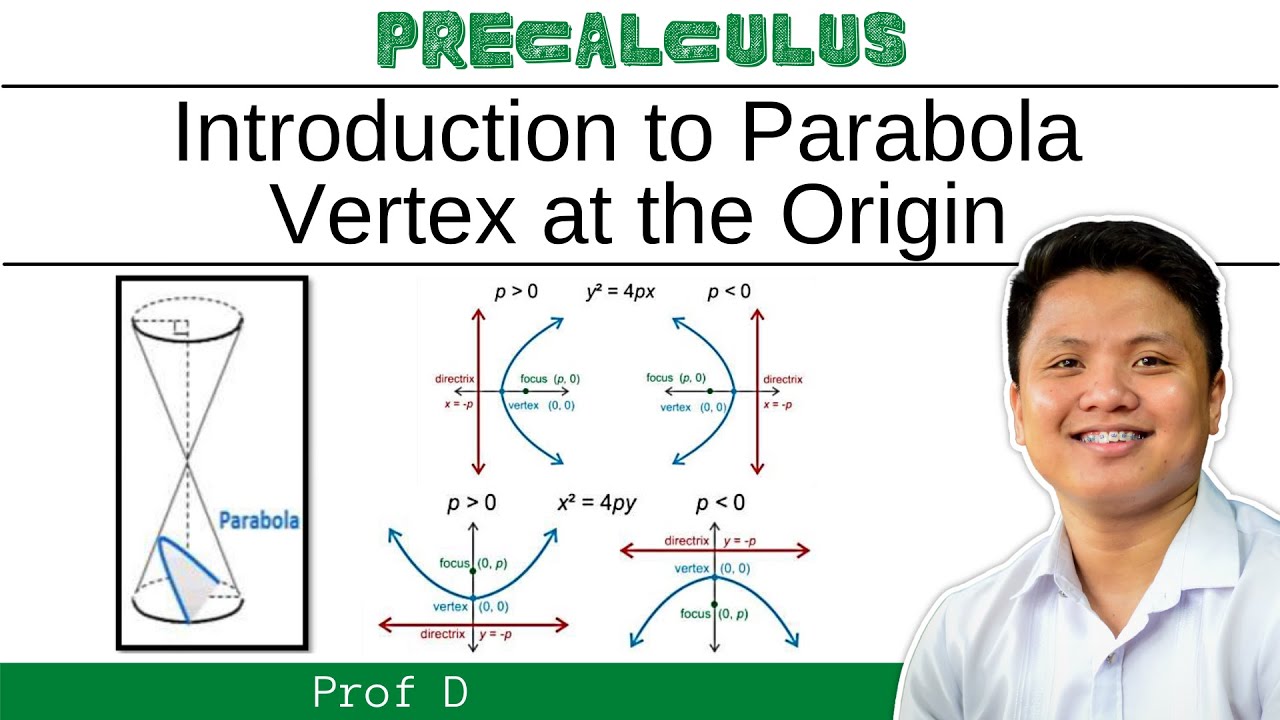
INTRODUCTION TO PARABOLA | GRAPHING | VERTEX AT THE ORIGIN | PROF D

STANDARD FORM OF THE EQUATION OF A PARABOLA WITH VERTEX AT THE ORIGIN || PRE-CALCULUS

Ch. 11.1 Parabolas

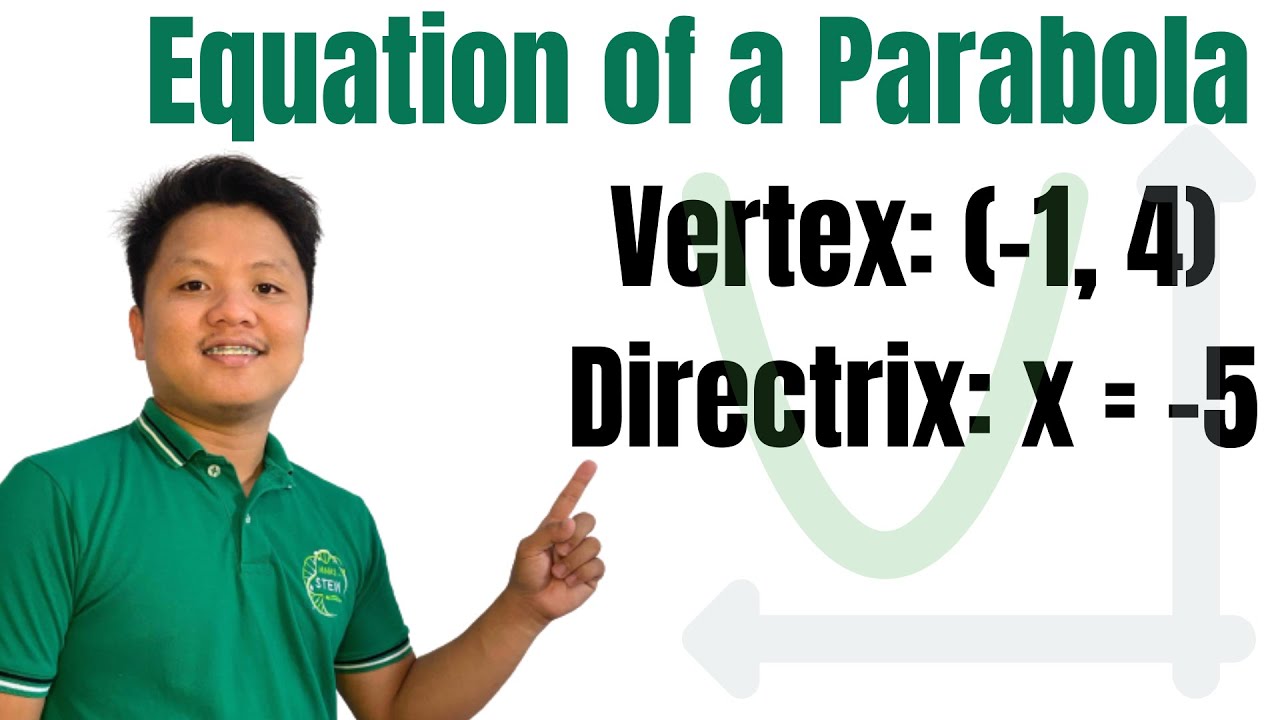
How to find the equation of a parabola given vertex and directrix | @ProfD

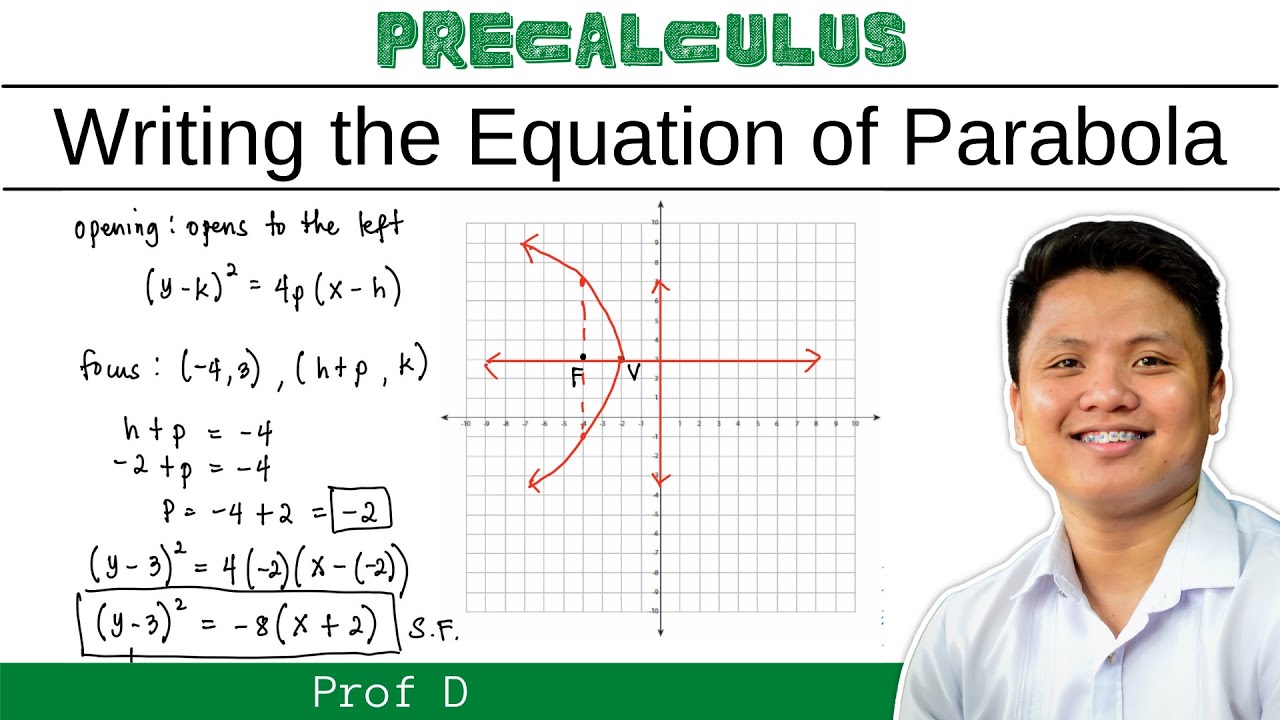
Writing Equation of A Parabola in Standard and General Form | @ProfD

Quadratic Equations using a TI 83 Plus Graphing Calculator

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Parabola EquationsVertex OriginFocus PointDirectrix LineLattice PointsMathematics TutorialEducational VideoGeometry ConceptsGraph SketchingProblem SolvingAlgebraic Geometry