Intermediate Value Theorem Explained - To Find Zeros, Roots or C value - Calculus

The Organic Chemistry Tutor
14 Sept 201613:11
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers a comprehensive tutorial on applying the Intermediate Value Theorem to determine the existence of roots within a specified interval for a given function. It demonstrates the process using the function f(x) = x^2 - x - 12 with the interval [3, 5], showing that a root exists by evaluating f(3) and f(5) and confirming their signs differ. The script also explains how to factor polynomials to find roots and extends the concept to finding a specific value of a function within an interval, as exemplified with f(x) = 2x^2 + 3x + 5 and an interval of [1, 4]. The explanation includes step-by-step instructions on evaluating function values at endpoints, solving for x when a function value is given, and using factoring techniques to find the value of x that satisfies the conditions. The tutorial is designed to help viewers understand and apply the theorem effectively.

Takeaways
  • 📚 The Intermediate Value Theorem (IVT) is used to show the existence of a root for a continuous function within a specified interval.
  • 🔍 To apply IVT, the function must be continuous and the values at the endpoints of the interval must have opposite signs, indicating that the function crosses zero.
  • 📈 The function f(x) = x^2 - x - 12 is used as an example to demonstrate the IVT, with the interval [3, 5].
  • 🧩 Calculating f(3) and f(5) shows that one value is negative and the other is positive, satisfying the conditions for IVT.
  • 🔑 The root of the equation is found by setting the function equal to zero and solving for x, leading to potential solutions x = 4 and x = -3.
  • 🎯 The correct root within the interval [3, 5] is x = 4, as it is the only value that lies within the specified interval.
  • 📉 In a second example, the function f(x) = 2x^2 + 3x + 5 is used to find a value of C within the interval [1, 4] where f(C) = 19.
  • 📌 The values of f(1) and f(4) are calculated to be 10 and 49, respectively, ensuring that 19 lies between them and can be found using IVT.
  • 📝 To find C, the equation 19 = 2x^2 + 3x + 5 is solved, leading to potential solutions x = 2 and x = -7/2.
  • 🏁 The value x = 2 is within the interval [1, 4] and is the correct C value where f(C) = 19.
  • 📚 The video script provides a step-by-step guide on how to apply the Intermediate Value Theorem to find roots or specific function values within an interval.
Q & A

  • What is the Intermediate Value Theorem?

    -The Intermediate Value Theorem states that if a function is continuous on a closed interval [a, b] and takes on different signs at a and b, then there is at least one value c within the interval (a, b) where the function equals zero.

  • What is the given function in the script?

    -The given function in the script is f(x) = x^2 - x - 12.

  • What interval is specified for the function f(x) = x^2 - x - 12?

    -The specified interval for the function is from 3 to 5.

  • How can you determine if the Intermediate Value Theorem can be applied to the function f(x) = x^2 - x - 12 on the interval [3, 5]?

    -You can determine if the theorem can be applied by checking if f(3) and f(5) have opposite signs, indicating that the function crosses the x-axis between these two points.

  • What are the values of f(3) and f(5) for the function f(x) = x^2 - x - 12?

    -The value of f(3) is -6 and the value of f(5) is 8. Since -6 is negative and 8 is positive, the function crosses the x-axis between 3 and 5.

  • What is the root of the equation x^2 - x - 12 = 0?

    -The roots of the equation are x = 4 and x = -3. However, since we are looking for a root within the interval [3, 5], the correct root is x = 4.

  • What is the process to find a root of a polynomial using the Intermediate Value Theorem?

    -First, ensure the function is continuous and that the function values at the endpoints of the interval have opposite signs. Then, set the function equal to zero and solve for x to find the root within the interval.

  • Can the Intermediate Value Theorem be used to find a specific value of a function within an interval, not necessarily a root?

    -Yes, the theorem can be used to find a specific value of a function within an interval if the function is continuous and the specified value lies between the function values at the endpoints of the interval.

  • How do you factor a trinomial with a leading coefficient of 1?

    -To factor a trinomial with a leading coefficient of 1, find two numbers that multiply to the constant term and add up to the linear coefficient.

  • What is the process to find the value of x if you are given a specific function value within an interval?

    -First, find the function values at the endpoints of the interval. If the given function value lies between these two values, set the function equal to the given value and solve for x. Ensure that the solution for x is within the interval.

  • How do you factor a trinomial with a leading coefficient other than 1?

    -First, multiply the leading coefficient by the constant term to get a new constant. Then, find two numbers that multiply to this new constant and add up to the linear coefficient. Replace the linear term with the sum of these two terms and factor by grouping.

Outlines
00:00
📚 Applying the Intermediate Value Theorem

This paragraph explains how to use the Intermediate Value Theorem to find a root of a given equation within a specified interval. The function f(x) = x^2 - x - 12 is provided, with the interval [3, 5]. To apply the theorem, it's necessary to show that f(3) and f(5) have opposite signs, which ensures a root exists between them. The calculations show f(3) = -6 and f(5) = 8, confirming the existence of a root. Factoring the function reveals two possible roots, x = 4 and x = -3, but only x = 4 lies within the given interval. Thus, the root of the equation in the interval is C = 4.

05:01
🔍 Finding a Value of C Using the Intermediate Value Theorem

The second paragraph illustrates another application of the Intermediate Value Theorem, this time to find a value of C within the interval [1, 4] for the function f(x) = 2x^2 + 3x + 5, given that f(C) = 19. The process involves evaluating the function at the endpoints of the interval, finding f(1) = 10 and f(4) = 49. Since 19 is between 10 and 49, a value C exists in the interval where f(C) = 19. By setting the function equal to 19 and solving for x, we can find the value of C. The equation is transformed to 2x^2 + 3x + 5 - 19 = 0, and the function is factored to find the values of x that are within the interval [1, 4]. The correct value of C is identified as C = 2, as it is the only value within the interval that satisfies the equation.

10:02
🧮 Factoring and Solving for C in an Interval

The third paragraph delves into the algebraic process of factoring the equation derived from setting f(x) equal to 19. The goal is to find the value of C within the interval [1, 4]. The equation is rearranged to 2x^2 + 3x - 14 = 0. To factor this trinomial, the leading coefficient is multiplied by the constant term to find two numbers that multiply to -28 and add up to the linear coefficient, 3. These numbers are identified as 7 and -4. The expression is then rewritten to isolate terms that sum to 3x, allowing for factoring by grouping. The factored form is x - 2 and 2x + 7, leading to the solutions x = 2 and x = -7/2. Only x = 2 is within the interval [1, 4], thus confirming that C = 2 is the correct value for which f(C) = 19.

Mindmap
Keywords
💡Intermediate Value Theorem
The Intermediate Value Theorem (IVT) is a fundamental concept in calculus that states if a continuous function takes on different values at two points, then it must take on every value in between at some point within the interval. In the video, the IVT is used to demonstrate the existence of a root for the given function within a specified interval. For example, the function f(x) = x^2 - x - 12 is shown to have a root between x = 3 and x = 5 because f(3) is negative and f(5) is positive.
💡Root
A root of an equation is a value of the variable that makes the equation equal to zero. In the context of the video, finding a root means identifying a value of x for which the function f(x) equals zero. The script uses the IVT to show that there is a root for the function f(x) = x^2 - x - 12 between the interval of 3 and 5, which is later found to be x = 4.
💡Continuous Function
A continuous function is one where there are no breaks or jumps in the graph of the function. It is a necessary condition for the IVT to apply. The video script explains that polynomial functions, like the one given (f(x) = x^2 - x - 12), are always continuous, which allows the use of the IVT to find roots or specific values within an interval.
💡Interval
In mathematics, an interval refers to a continuous range of values on the number line. The video discusses finding a root or a specific value of a function within a given interval, such as between x = 3 and x = 5. The IVT is applied to show that since the function values at the endpoints of the interval are of opposite signs, there must be a root within that interval.
💡Factoring
Factoring is the process of breaking down a polynomial into a product of its factors. In the script, factoring is used to find the roots of the function f(x) = x^2 - x - 12 by finding two numbers that multiply to -12 and add up to 1, which leads to the factors (x - 4) and (x + 3), and thus the roots x = 4 and x = -3.
💡Polynomial Function
A polynomial function is an expression consisting of variables and coefficients, involving only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. The video script uses a polynomial function, f(x) = x^2 - x - 12, to illustrate the application of the IVT and the process of finding roots.
💡Leading Coefficient
The leading coefficient of a polynomial is the coefficient of its highest degree term. In the video, when factoring the polynomial f(x) = x^2 - x - 12, the leading coefficient is 1, which simplifies the factoring process. The script also mentions that when the leading coefficient is not one, as in the second example, additional steps are needed to factor the polynomial.
💡Graphical Interpretation
The video script includes a discussion of the graphical interpretation of the IVT, where it is mentioned that if a continuous curve goes from one value to another, it must cross a certain value in between. This is illustrated with an example where the function's value at the endpoints of the interval is known, and it is explained that the function must cross a given value within that interval.
💡Y-values and X-values
In the context of the video, y-values refer to the output values of the function, and x-values refer to the input values or the points on the x-axis. The script explains that for the IVT to apply, the y-value (like 0 for a root or 19 in the second example) must be between the y-values of the function at the endpoints of the interval, and the corresponding x-value must be between the x-values of those endpoints.
💡Trinomial
A trinomial is a polynomial with three terms. In the video, the function f(x) = x^2 - x - 12 is a trinomial, and the script discusses how to factor it to find its roots. The second example in the script also involves a trinomial, 2x^2 + 3x + 5, where the process of factoring is used to find a specific value of x that gives a y-value of 19.
Highlights

Introduction to the Intermediate Value Theorem and its application to finding roots of equations.

Explanation of a continuous function and the necessity for it to pass through zero when values at endpoints are of opposite signs.

Demonstration using the function f(x) = x^2 - x - 12 to show the existence of a root between the interval 3 to 5.

Calculation of f(3) resulting in a negative value, indicating the need for a positive f(5) to apply the theorem.

Finding f(5) and confirming it is positive, thus satisfying the conditions of the Intermediate Value Theorem.

Verification that the function crosses zero between the interval [3, 5], implying the existence of a root.

Factoring the quadratic equation to find potential roots x = 4 and x = -3.

Determining the correct root within the specified interval, concluding that x = 4 is the root.

Transition to a second example involving the function f(x) = 2x^2 + 3x + 5 and finding a value of C within the interval [1, 4].

Given a specific y-value for f(C), the task is to find the corresponding x-value within the interval.

Calculation of f(1) and f(4) to establish the y-values at the endpoints of the interval.

Using the Intermediate Value Theorem to assert that a value of f(C) = 19 must exist within the interval.

Solving the equation 19 = 2x^2 + 3x + 5 to find the x-values that correspond to f(C).

Factoring the resulting quadratic equation to simplify the process of finding x.

Identifying the x-values that satisfy the equation and determining which is within the interval [1, 4].

Conclusion that x = 2 is the value of C that corresponds to f(C) = 19 within the specified interval.

Summary of the process for using the Intermediate Value Theorem to find roots or specific values within an interval.

Transcripts

Browse More Related Video

Intermediate Value Theorem, calculus 1 tutorial, showing a root of a function on an interval

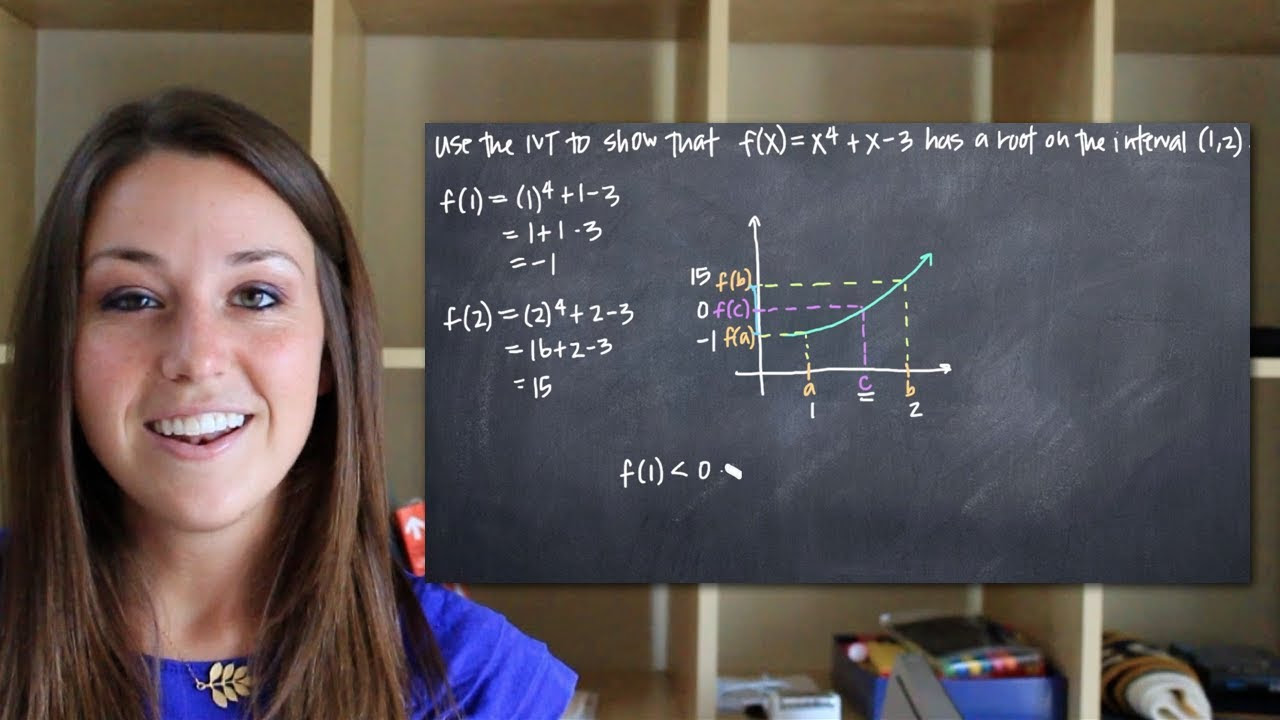
Intermediate value theorem to prove a root in an interval (KristaKingMath)

Average Value of a Function

How to show that a solution exists to a functions using IVT

AP Calculus AB Crash Course Day 8 - Integration and Riemann Sums

2011 Calculus AB free response #4b | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Intermediate Value TheoremRoot FindingMathematicsEquation SolvingContinuous FunctionsFactorizationPolynomial FunctionsEducational ContentTheoretical AnalysisProblem Solving