Logarithmic Differentiation | Example: x^sinx

Dr. Trefor Bazett
12 Sept 201703:36
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the process of logarithmic differentiation, a technique used to find derivatives of complex functions. The presenter explains that while they have mastered the derivatives of polynomials, trigonometric terms, products, compositions, quotients, and sums, exponential functions like X to the power of sine(X) have been challenging. To tackle this, the presenter uses a logarithmic property, transforming the exponent into a product, which simplifies the differentiation process. By applying the natural logarithm to both sides of the equation, the presenter then applies the chain rule and product rule to derive the derivative. The final step involves isolating the derivative to express it as a function of X. The script concludes by emphasizing the utility of logarithmic differentiation in solving previously intractable derivative problems, resulting in complex but solvable expressions.

Takeaways
  • 🎯 The primary goal is to compute derivatives of as many functions as possible, including polynomials, trigonometric terms, products, compositions, quotients, and sums.
  • 🚫 A challenge remains with derivatives of exponential functions, specifically those involving non-constant exponents like X^(sin(X)).
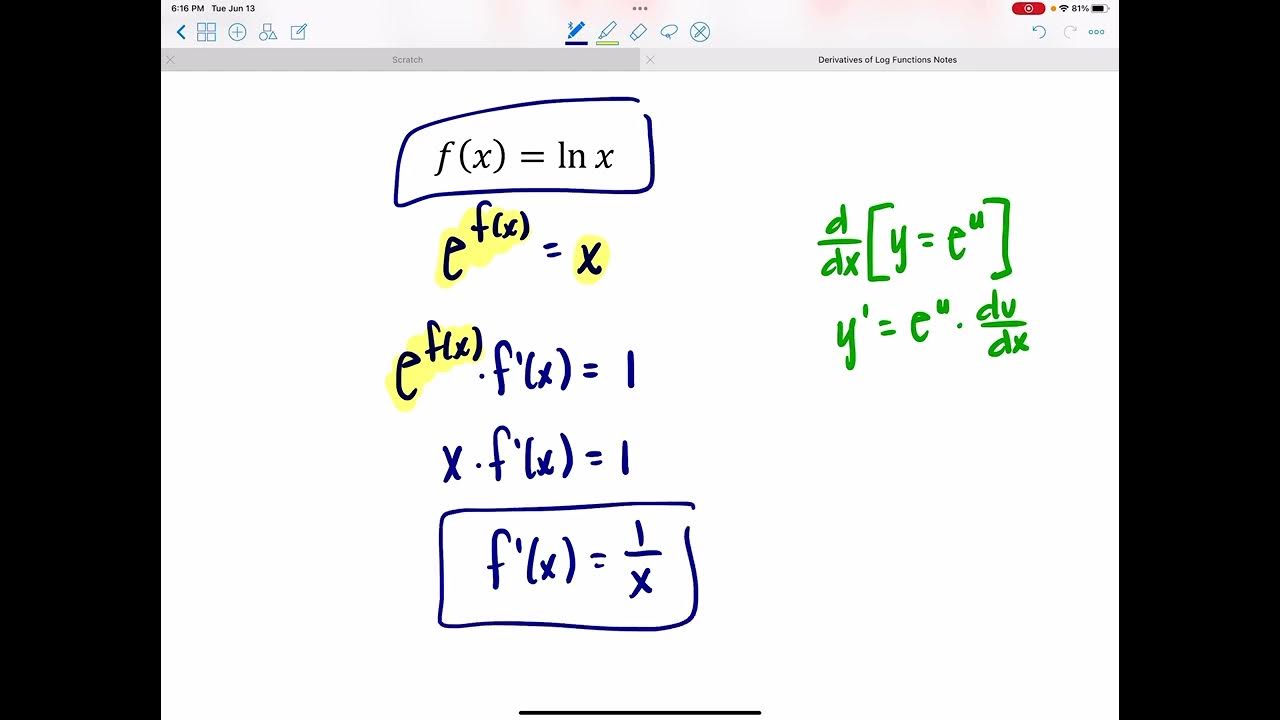
  • 🔍 Logarithms can be used to transform challenging exponential expressions into products, which are easier to differentiate.
  • ✅ The natural logarithm rule allows us to bring down the exponent, turning e^(B) into B * ln(a).
  • 📚 Logarithmic differentiation is a technique that leverages the properties of logarithms to simplify the differentiation of complex functions.
  • 🔧 By taking the natural log of both sides of an equation, we can use the chain rule and product rule to find derivatives.
  • 🌀 Applying the chain rule to ln(y) gives us (1/y) * (dy/dx), which is crucial for differentiating the inside function.
  • 📈 The derivative of sin(X) is used in conjunction with the natural logarithm of X to apply the product rule.
  • 🧹 After applying differentiation rules, it's important to simplify and isolate (dy/dx) to express it as a function of X alone.
  • 📝 The final answer for the derivative of X^(sin(X)) with respect to X is a complex expression, demonstrating the power of logarithmic differentiation.
  • 🔬 This method enables us to find derivatives of functions that were previously difficult to differentiate directly.
Q & A

  • What was the primary goal mentioned in the transcript?

    -The primary goal mentioned was to compute the derivative of as many functions as possible.

  • Which functions have been figured out for derivatives in the transcript?

    -The functions that have been figured out include polynomials, trigonometric terms, products, compositions, quotients, and sums.

  • What class of functions was still a challenge in the transcript?

    -The class of exponential functions, specifically the derivative of X to the power of sine of X, was still a challenge.

  • What mathematical tool is used to simplify the process of differentiating exponential functions?

    -Logarithms are used to simplify the process, particularly by transforming exponents into products.

  • How does the natural logarithm help in differentiating the function y = X to the power of sine of X?

    -The natural logarithm transforms the exponential function into a form that can be differentiated using the product rule, making it easier to handle.

  • What rule is applied when taking the derivative of the natural log of both sides of an equation?

    -The chain rule is applied, which allows differentiation of the composite function.

  • What is the derivative of the sine of X with respect to X?

    -The derivative of the sine of X with respect to X is cosine of X.

  • How is the product rule used in the process of differentiating the given function?

    -The product rule is used to differentiate the product of the derivative of sine times the natural log of X.

  • What is the final expression obtained for the derivative of X to the power of sine of X?

    -The final expression is a long and messy expression involving the derivative of sine, the natural log of X, and the original function.

  • What is the significance of logarithmic differentiation in the context of the transcript?

    -Logarithmic differentiation allows for the computation of derivatives of functions that are otherwise difficult to differentiate directly.

  • How does the process of logarithmic differentiation transform challenging derivatives into simpler forms?

    -Logarithmic differentiation transforms challenging derivatives into simpler forms by converting exponential expressions into products, which can then be handled using the product rule.

  • What is the role of the product rule in the process described in the transcript?

    -The product rule is essential for differentiating the transformed function after applying the logarithm, as it allows for the differentiation of the product of two functions.

Outlines
00:00
📚 Introduction to Derivatives and Logarithmic Differentiation

The paragraph introduces the goal of computing derivatives of various functions. It mentions the success in deriving polynomials, trigonometric terms, products, compositions, quotients, and sums. However, exponential functions, specifically \( X^{\sin(X)} \), present a challenge. The speaker plans to use logarithmic differentiation to tackle this problem by transforming exponential functions into products, which can then be handled using the product rule. The process involves taking the natural logarithm of both sides of the equation and applying the chain rule and product rule to find the derivative.

Mindmap
Keywords
💡Derivative
The derivative is a fundamental concept in calculus that represents the rate at which a function changes at a given point. In the video, the process of computing derivatives is the central theme, with the goal of finding derivatives for various types of functions, including polynomials, trigonometric terms, and exponentials.
💡Logarithms
Logarithms are mathematical operations that allow us to express the power to which a number must be raised to obtain a certain value. In the script, logarithms are used as a tool to transform exponential functions into a form that can be more easily differentiated, which is crucial for solving the main problem of the video.
💡Natural Log
The natural log, often denoted as ln(x), is the logarithm to the base e (approximately 2.71828). It is used in the video to simplify the differentiation of exponential functions by converting them into a form that can be handled with the product rule.
💡Exponential Functions
Exponential functions are mathematical functions of the form f(x) = a^x, where 'a' is a constant. They are challenging to differentiate directly, but the video demonstrates how logarithmic differentiation can be used to find their derivatives.
💡Product Rule
The product rule is a fundamental rule in calculus for differentiating products of two functions. It states that the derivative of the product of two functions is the derivative of the first times the second, plus the first times the derivative of the second. In the video, the product rule is used after applying logarithmic differentiation to handle the differentiation of the function y = x^(sin(x)).
💡Chain Rule
The chain rule is a method in calculus for finding the derivative of a composite function. It is used in the video when differentiating the natural log of y with respect to x, where y is a function of x.
💡Log Rule
The log rule, or logarithmic differentiation, is a technique used to differentiate functions that are products, quotients, or compositions of other functions. In the context of the video, it is applied to transform the exponential function y = x^(sin(x)) into a form that can be differentiated using the product rule.
💡Polynomials
Polynomials are algebraic expressions consisting of variables and coefficients, involving only the operations of addition, subtraction, and multiplication, and non-negative integer exponents of variables. They are mentioned in the video as one of the types of functions for which the derivative has already been figured out.
💡Trigonometric Terms
Trigonometric terms refer to functions based on the ratios of the sides of a right triangle, such as sine, cosine, and tangent. The video mentions that derivatives of these functions have been established, and they serve as a contrast to the more complex exponential functions being discussed.
💡Composition
In calculus, a composition of functions refers to a function that is formed by applying one function to the result of another. The video mentions that the derivative of a composition has been figured out, which is a prerequisite for understanding more complex differentiation techniques.
💡Quotients
Quotients in calculus refer to the process of differentiating a function that is the ratio of two other functions. The video briefly mentions that the derivative of quotients is known, which is part of the foundation for tackling more complex differentiation problems.
Highlights

The goal is to compute the derivative of as many functions as possible.

Derivatives of polynomials, trigonometric terms, products, compositions, quotients, and sums have been figured out.

The challenge is to find the derivative of exponential functions like X to the power of sine of X.

Logarithms can be used to transform exponents into products, making derivatives easier to compute.

Taking the natural log of e to the power of B brings down the exponent B times the natural log of A.

Applying the natural log to both sides of an equation can simplify the process of finding a derivative.

The product rule can be used to find the derivative of a product of two functions.

The chain rule is used to find the derivative of the natural logarithm of a function.

The derivative of y with respect to x can be isolated by moving terms around in the equation.

The final answer for the derivative of X to the sine of X is a long and messy expression.

Logarithmic differentiation allows us to compute derivatives of challenging exponential functions.

The natural log transforms challenging exponential functions into products, making them easier to differentiate.

The product rule and chain rule are key to logarithmic differentiation.

Logarithmic differentiation makes it possible to find derivatives of functions that were previously difficult.

The process involves taking the natural log of both sides, applying the product and chain rules, and isolating the derivative.

The final answer is a function of X, obtained by manipulating the equation and removing unnecessary terms.

The method is demonstrated through an example of finding the derivative of X to the sine of X.

The process is explained step-by-step, making it easier to understand the technique of logarithmic differentiation.

Transcripts

Browse More Related Video

Logarithmic Differentiation

Introduction to Logarithmic Differentiation

Derivatives of Log Functions

Math1325 Lecture 11 1 Derivatives of Logarithm

Derivative of x^(x^x) | Taking derivatives | Differential Calculus | Khan Academy

Derivatives of Logarithmic Functions - More Examples

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Logarithmic DifferentiationDerivative CalculationMathematical TechniquesComplex FunctionsProduct RuleChain RuleTrigonometric FunctionsExponential ExpressionsEducational ContentAdvanced MathCalculus Tutorial