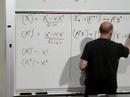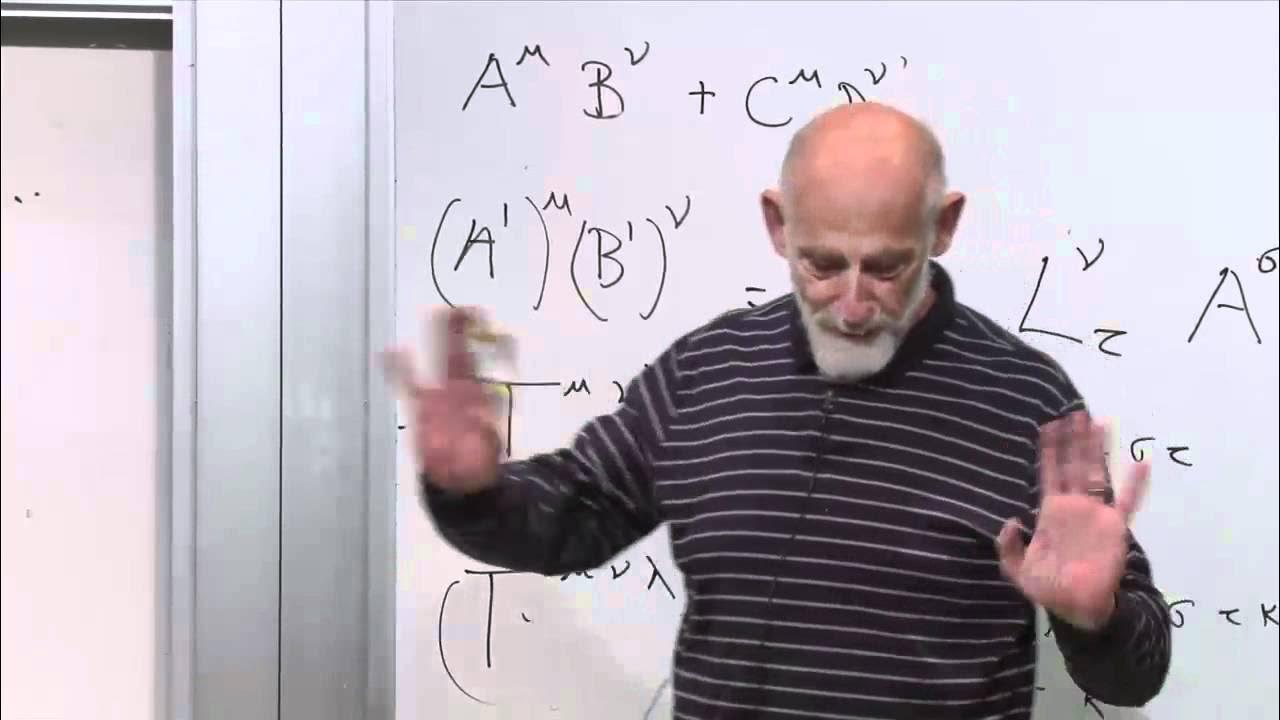Lecture 6 | Quantum Entanglements, Part 3 (Stanford)
TLDRThe video script is an in-depth exploration of the laws of nature and physics, particularly focusing on the principles of relativity. It delves into the concepts of homogeneity and isotropy in space, explaining why certain proposed laws of nature, such as particles colliding when their x-coordinates are the same, violate these principles. The lecturer emphasizes the importance of physical laws being invariant under rotations and transformations, leading to the discussion of four-vectors and their role in expressing these laws in a way that remains consistent across different coordinate systems. The script also touches on the Lorentz transformation and how it relates to the constancy of the speed of light. It further explains the concept of tensors, especially anti-symmetric tensors, which are crucial for understanding the electromagnetic field in the context of special relativity. The summary also highlights the historical development of these ideas, including the contributions of Einstein and Minkowski in formulating the laws of physics in a way that is the same in all reference frames.
Takeaways
- 📐 **Homogeneity and Isotropy**: The laws of physics should remain the same regardless of the location (homogeneity) or orientation (isotropy) of the coordinate system.
- 🌌 **Relativistic Physics**: The laws of physics, particularly those concerning particle interactions, must be invariant under Lorentz transformations to be considered valid in the context of special relativity.
- 🚀 **Four-Vectors**: In physics, four-vectors are used to express quantities that are invariant under Lorentz transformations, such as position in space-time or momentum.
- 🔄 **Tensors**: Tensors are multi-dimensional arrays that extend the concept of vectors and are crucial for describing quantities like the electromagnetic field in a way that is consistent across different frames of reference.
- 🧲 **Electromagnetic Fields**: Electric and magnetic fields are components of a single anti-symmetric tensor, which transforms covariantly under Lorentz transformations.
- 🤝 **Lorentz Force Law**: The force experienced by a charged particle in an electromagnetic field is given by the product of the particle's charge and the electric field, as well as the cross product of its velocity and the magnetic field.
- ⏱️ **Proper Time and Velocity**: The four-velocity of a particle is defined with respect to proper time, which is the time measured by a clock moving with the particle.
- 🌟 **Relativity Principle**: Einstein's principle of relativity states that the laws of physics are the same in all inertial frames, leading to the development of the special theory of relativity.
- 📉 **Minkowski Spacetime**: Minkowski provided a geometric interpretation of special relativity, where space and time are combined into a four-dimensional spacetime, facilitating a more intuitive understanding of relativistic phenomena.
- 🔗 **Covariance**: The equations of physics should be covariant, meaning they take the same form in all inertial frames, which is achieved by using four-vectors and tensors.
- ⚙️ **Transformation Matrices**: Lorentz transformation matrices are used to convert the components of four-vectors and tensors from one inertial frame to another while preserving their invariant properties.
Q & A
What is the importance of expressing laws of physics in terms of vectors?
-Expressing laws of physics in terms of vectors is important because vectors transform in a specific way that maintains the invariance of physical laws under different coordinate systems. This ensures that the laws of physics are the same in every frame of reference, which is a fundamental requirement for a theory to be considered physically valid.
What is the concept of homogeneity in the context of the laws of physics?
-Homogeneity in physics refers to the idea that the laws of nature are independent of where you place the center of your coordinate system. This means that if you translate your coordinate system, the laws of physics remain the same, reflecting the uniformity of space.
What does isotropy of space imply in the context of physical laws?
-Isotropy of space implies that the laws of physics remain unchanged under rotations of the coordinate system. This means that no matter how you orient your axes, the physical laws should hold true, reflecting the symmetry of space.
What is a four-vector and why is it used in physics?
-A four-vector is an object with four components that transform in a specific way under Lorentz transformations, which are the mathematical operations relating different inertial frames in special relativity. Four-vectors are used in physics because they allow for a covariant formulation of physical laws, ensuring that these laws are expressed in a form that is invariant under changes of the observer's frame of reference.
What is the significance of the Lorentz transformation in special relativity?
-The Lorentz transformation is significant because it describes how quantities such as space and time coordinates, and the velocity of a particle, transform from one inertial frame to another. It is a fundamental concept in special relativity that ensures the laws of physics, including the speed of light being constant, are the same in all inertial frames.
How does the concept of proper time relate to the four-velocity?
-Proper time is the time measured by a clock moving with an object, and it is related to the four-velocity of the object. The four-velocity is defined as the rate of change of the space-time position of the object with respect to proper time. It is a four-vector that includes components for spatial velocity and the rate of change of time, normalized so that its contraction with itself is one.
What is the role of tensors in expressing the electromagnetic field in relativistic physics?
-Tensors, specifically anti-symmetric tensors, are used to combine the electric and magnetic fields into a single object that transforms covariantly under Lorentz transformations. This allows for a relativistically invariant formulation of the electromagnetic field, which is crucial for describing the behavior of charged particles in electromagnetic fields across different inertial frames.
What is the relationship between the space components of a four-vector and its time component?
-The space components of a four-vector are related to the time component through the metric tensor of space-time. In the context of special relativity, the time component of a four-vector (such as four-momentum or four-velocity) is related to its space components through the Lorentz transformation, which mixes space and time components in a way that depends on the relative velocity between reference frames.
How does the concept of a scalar differ from that of a vector in physics?
-A scalar is a quantity that has magnitude but no direction, and its value does not change under coordinate transformations. A vector, on the other hand, has both magnitude and direction, and its components change according to specific rules under coordinate transformations. Scalars remain invariant under rotations and Lorentz transformations, while vectors transform according to the rules that preserve their directional properties.
What is the significance of the contravariant and covariant components of a four-vector?
-The contravariant and covariant components of a four-vector are related through the metric tensor, which encodes the geometry of space-time. The contravariant components transform like the coordinates of space-time under Lorentz transformations, while the covariant components are obtained by lowering the index with the metric tensor. This distinction is important for forming scalar products that are invariant under Lorentz transformations.
How does the four-momentum of a particle relate to its energy and momentum?
-The four-momentum of a particle is a four-vector that combines its energy and momentum into a single object. The time component of the four-momentum is the particle's energy, while the space components are its momentum. This combination allows for a covariant description of energy and momentum in different inertial frames, which is essential in relativistic physics.
Outlines
📚 Introduction to the Laws of Nature
The paragraph introduces the topic of natural laws, focusing on the idea that these laws should be consistent across different frames of reference. It uses the example of particle collision to illustrate the violation of isotropy, which is the principle that physical laws remain the same under rotation of the coordinate system. The explanation emphasizes the importance of expressing physical laws in a way that is invariant under transformations, such as translations and rotations.
🌀 Rotational Invariance and Vectors
This section delves into the concept of rotational invariance, which is the property that physical laws remain unchanged under rotations. It contrasts a law that violates this principle with one that does not, highlighting the importance of using vector notation to express laws of physics. The paragraph explains that vectors transform in a consistent way under rotations, which is why they are used to formulate physics laws that are invariant under all orientations of the coordinate system.
⏱️ Time and Relativity
The paragraph discusses the incorporation of time into the framework of physics laws, touching upon the concept of Lorentz invariance. It explains that particle collisions in physics can only occur when particles meet at the same space-time point, a concept that is invariant under Lorentz transformations. The discussion leads to the introduction of four-vectors and the idea that physical laws should be expressed in a way that is invariant under all reference frames, not just spatial but also temporal transformations.
🚀 Four-Vectors and Lorentz Transformations
This section introduces four-vectors, which are objects with four components that transform under Lorentz transformations in a way that preserves their inner product. The paragraph explains how to represent the space-time position of a particle as a four-vector and how the components of this vector transform between different reference frames. It also discusses the concept of scalars, which are quantities that remain unchanged under transformations, and how they can be constructed from the components of four-vectors.
🔄 Transformation Matrices and Scalar Quantities
The paragraph explores the mathematical representation of transformations using matrices. It discusses how the components of vectors and tensors transform under rotations and Lorentz boosts, and how these transformations can be represented in matrix form. The summary also touches on the concept of scalars, which are invariant under all transformations, and how they are used to make statements that hold true in every reference frame.
🧲 Electromagnetic Fields and Relativistic Laws
This section discusses the challenge of expressing the laws governing the motion of charged particles in electric and magnetic fields in a way that is consistent with relativity. It points out that the decomposition of fields into electric and magnetic components is not invariant and that a relativistic law of motion must account for the transformation between these fields under different reference frames. The paragraph sets the stage for a deeper exploration of how to construct such laws using tensors, which are capable of capturing the complexity of the electromagnetic field's behavior under relativistic transformations.
🤔 Tensors and the Electromagnetic Field
The paragraph introduces tensors as the mathematical objects that generalize vectors to more complex transformations, allowing for the combination of multiple vectors into a single object that transforms consistently. It explains that tensors can be of various ranks and that they are characterized by their transformation properties under Lorentz transformations. The discussion also leads to the identification of the electromagnetic field as a second-rank antisymmetric tensor, which is a key step towards formulating a relativistic law of motion for charged particles.
🏛️ Minkowski and the Four-Vector Formulation
This section reflects on the historical development of the four-vector notation and the role of Minkowski in formalizing the concept of space-time and the four-dimensional formulation of physics laws. It discusses Einstein's initial reluctance to adopt Minkowski's ideas but acknowledges his eventual acceptance and the significance of these ideas in the development of the theory of relativity. The paragraph also highlights the importance of systematic transformations and the role of intuition in the advancement of scientific understanding.
Mindmap
Keywords
💡Lorentz Transformation
💡Isotropy of Space
💡Homogeneity of Space
💡Four-Vector
💡Tensor
💡Proper Time
💡Four-Velocity
💡Relativistic Invariance
💡Electromagnetic Field Tensor
💡Cross-Product
💡Lorentz Force Law
Highlights
Discussion on the laws of nature and the violation of homogeneity and isotropy in physics.
Introduction of a hypothetical law of nature where particles collide when their x-coordinates are the same.
Explanation of how the concept of rotational invariance is key to formulating laws of physics.
Use of vectors to express the laws of physics in a way that is invariant under rotations.
The importance of perpendicular coordinates in expressing the laws of physics.
Example of a law of physics that is invariant under rotations, involving particle interaction at the same point.
Introduction of four-vectors and their role in special relativity.
Explanation of how particle collisions in physics are treated in the context of special relativity.
Discussion on the Lorentz invariance and its implications for simple particle collisions.
Transformation properties of four-vectors under Lorentz transformations.
Differentiation between contravariant and covariant forms of a four-vector.
Use of the metric tensor to switch between contravariant and covariant components.
Introduction to the concept of scalars and their invariance under all transformations.
Explanation of the four-velocity and its relationship with proper time and ordinary velocity.
Discussion on the four-momentum of a particle and its components in terms of energy and momentum.
Introduction to tensors as objects that transform with two Lorentz transformation matrices.
Explanation of how electric and magnetic fields combine to form an anti-symmetric tensor.
Historical context on Einstein's development of special relativity and Minkowski's contribution to four-vector notation.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: