Integration
TLDRIn this engaging video, Sara from Starfish Math dives into the concept of integration, which is the inverse process of differentiation. She emphasizes the importance of mastering differentiation before tackling integration and offers a comprehensive guide to both definite and indefinite integration. The video begins with basic integration techniques, illustrating how to integrate simple functions like x squared and 2x, and progresses to more complex terms. Sara explains the integration sign, the process of adding and dividing, and the necessity of including a constant 'C' in indefinite integration. She then explores the practical application of integration in calculating the area under a curve, demonstrating the process through graph sketching and integration within specific limits. The video concludes with an example of an exam question, showcasing how to find the coordinates of points on a curve and calculate the area of a shaded region using integration, which requires both mathematical knowledge and creative thinking. Sara encourages viewers to practice and provides valuable tips to avoid common mistakes, ensuring a solid understanding of integration.
Takeaways
- 📚 Start with the basics: Integration is the reverse process of differentiation, and it involves finding the original function given its derivative.
- 🔍 Practice differentiation: If unsure about differentiation, practice it first before moving on to integration.
- 📈 Definite and indefinite integration: Definite integration deals with finding the area under a curve between two points, while indefinite integration finds the general form of the original function.
- 📝 Integration notation: Use the integral sign ∫ with 'dx' to indicate integration with respect to x.
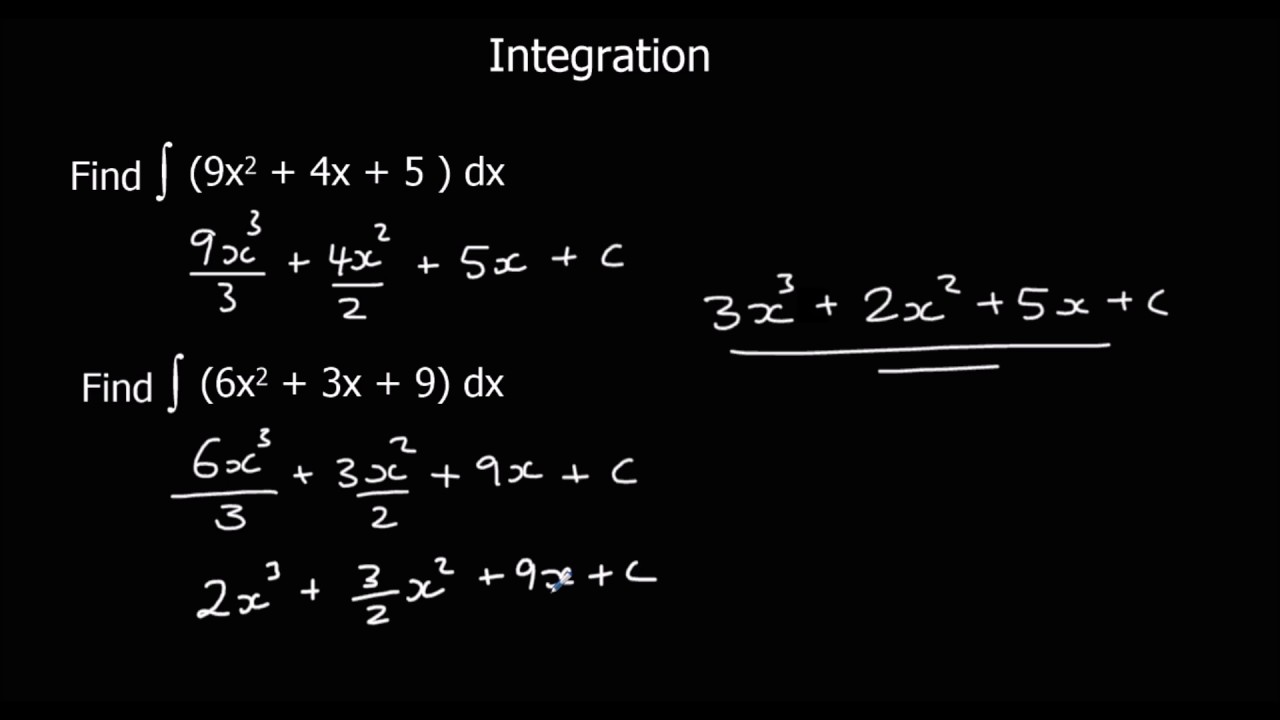
- 🔢 Power rule for integration: When integrating, add 1 to the power and divide the coefficient by the new power.
- 🤔 Constant of integration: Always include a constant 'C' in indefinite integration because differentiation loses constant terms.
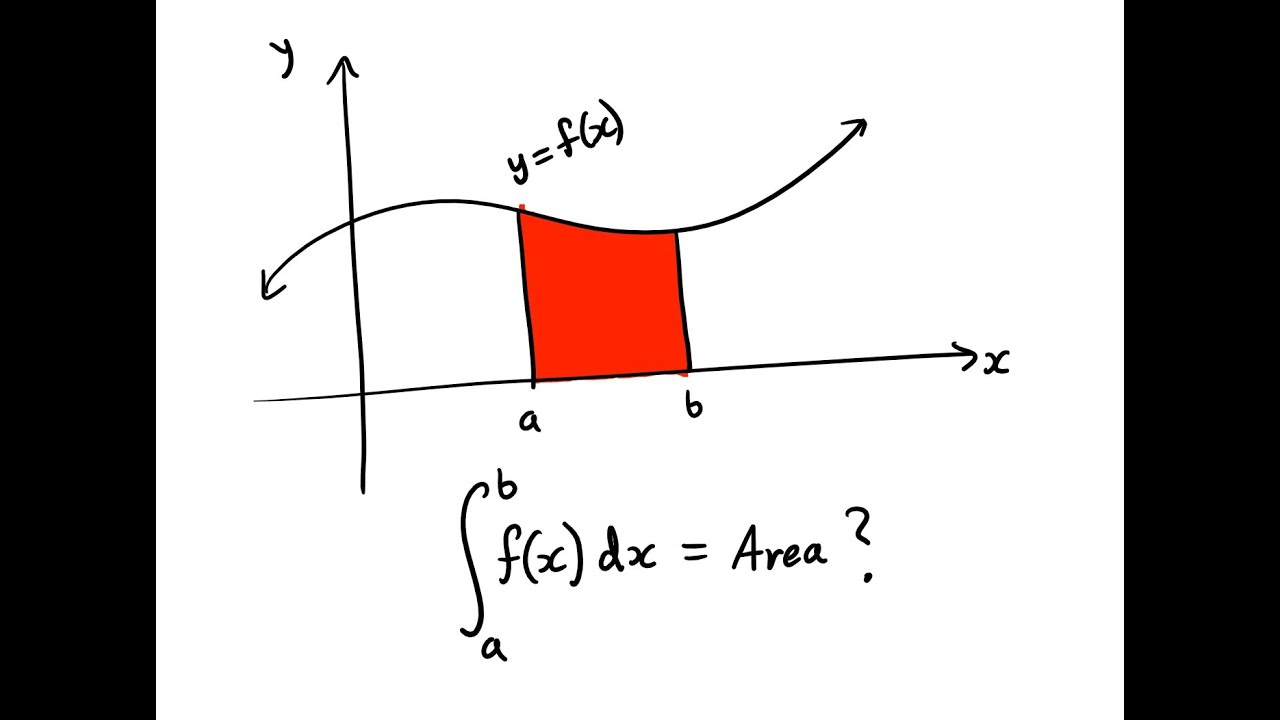
- 📊 Application of integration: A primary application of integration is to find the area under a curve, which is useful for various real-world problems.
- 📉 Sketch the graph: Before integrating to find area, sketch the graph to understand the curve's behavior and where it crosses the axes.
- ✅ Check your work: When performing definite integration, double-check your calculations, especially with signs and limits.
- 🔁 Positive and negative areas: When integrating over areas that cross the x-axis, consider the signs of the areas to get the correct total area.
- 📐 Additional shapes: In exams, be prepared to calculate areas of different shapes like trapeziums or triangles that result from the integration process.
- 🚀 Practice and creativity: For exam questions, practice integrating and be creative in finding the area of different shapes that result from the integration.
Q & A
What is the basic concept of integration?
-Integration is a mathematical operation that is the opposite of differentiation. It is used to find areas under curves, volumes, central points, and many other applications.
Why is it important to add a constant (C) when performing indefinite integration?
-Adding a constant (C) is important because when differentiating a function, any constant term would disappear. Therefore, when integrating, we must account for the possibility that a constant was lost during differentiation.
How do you integrate a function like 2x?
-To integrate 2x, you add 1 to the power to get 3, then divide the coefficient (2) by the new power (3), resulting in \( \frac{2}{3}x^3 \) plus the constant of integration (C).
What is the language of integration used when integrating a function?
-The language of integration uses an integral sign (∫) with 'dx' underneath the function that you want to integrate, indicating the integration is with respect to x.
How does the process of integrating a polynomial function differ from differentiating it?
-When integrating a polynomial function, you add 1 to the power of each term and divide the coefficient by the new power. In contrast, differentiation involves multiplying the coefficient by the power and subtracting 1 from the power.
What is the practical application of integration discussed in the script?
-The practical application of integration discussed in the script is finding the area under a curve between two limits, which is known as definite integration.
How does the sign of the area under a curve affect the calculation in definite integration?
-In definite integration, the sign of the area under a curve indicates whether the area is above or below the x-axis. Positive areas are above the x-axis, while negative areas are below it. When calculating the total area, these signs must be taken into account.
What is the method to find the area of a trapezium?
-The area of a trapezium is found by taking the average of the lengths of the parallel sides (a and b) and multiplying it by the height (h), which gives \( \frac{1}{2}(a + b)h \).
What is the process to find the coordinates of points A and B where two curves intersect?
-To find the coordinates of points A and B, set the equations of the two curves equal to each other and solve the resulting system of equations to find the x-coordinates. Then substitute these x-coordinates back into one of the original equations to find the corresponding y-coordinates.
How does the process of sketching a graph help in integration?
-Sketching a graph helps in understanding the shape of the curve and where it intersects the axes, which is crucial for setting the limits of integration correctly. It also aids in visualizing the areas that are being calculated.
What is the significance of the plus-minus sign in the context of definite integration?
-The plus-minus sign in definite integration indicates the need to consider both the positive and negative areas under the curve. It is used to adjust the calculation to ensure that the total area is correctly represented as a positive value.
Outlines
📚 Introduction to Integration Basics
This paragraph introduces the concept of integration as the reverse process of differentiation. The speaker, Sara, recommends additional practice for those less confident in differentiation. The video covers both definite and indefinite integration, with a focus on increasing difficulty levels. Sara encourages viewers to practice by pausing the video and attempting questions independently. She begins with the fundamental process of integrating basic functions, such as integrating x squared to get 2x, and explains the integration process with the addition of a constant 'C' to account for any vanished constants during differentiation. The language of integration is also introduced, with the integration sign ∫ and dx used to denote the function to be integrated with respect to x. Examples of integrating polynomials and more complex terms are provided, emphasizing the need for careful practice and understanding of the integration process.
🔍 Definite and Indefinite Integration Applications
The second paragraph delves into the application of integration, specifically for finding the area under a curve. Differentiation is contrasted with integration, with the former being used for rates of change and the latter for calculating areas. The process of sketching a graph to find where the curve intersects the axes is demonstrated. Definite integration is introduced, which requires setting limits and is used to find the area between the curve and the x-axis within those limits. The importance of careful calculation, especially with minus signs, is emphasized. The paragraph includes an example of integrating a cubic function and adjusting for areas above and below the x-axis, resulting in a total positive area. The process of sketching the graph, integrating, and combining areas with appropriate signs is detailed, highlighting the need for accuracy and understanding of the integration process.
📏 Calculating Areas with Definite Integration
This paragraph focuses on using definite integration to calculate areas. An example is given where the area under a curve is calculated by integrating from a point 3 to -2, requiring two separate integrations due to the curve crossing the x-axis. The process involves changing the sign of one area to ensure all areas are considered as positive when calculating the total. The paragraph also includes an exam question from an A-Level Mathematics paper, where the coordinates of points A and B on a curve are determined by setting two equations equal to each other. After finding the coordinates, the area under the curve between two x-coordinates is calculated by integrating the curve's equation. The area of a trapezium, which is part of the region to be excluded from the total area, is also calculated using the trapezium's lengths and height. The final step combines the integrated area with the adjusted trapezium area to find the total shaded region's area.
🎓 Exam Preparation and Practice Advice
The final paragraph offers advice for exam preparation, emphasizing the importance of practicing integration problems, especially those that require creative thinking to calculate areas of trapeziums or triangles. The speaker highlights the coincidence of obtaining the same answer, 343/6, from a different question, suggesting that such occurrences are a matter of luck. The paragraph concludes with encouragement to continue practicing and to enjoy the learning process.
Mindmap
Keywords
💡Integration
💡Differentiation
💡Definite Integration
💡Indefinite Integration
💡Power Rule
💡Constant of Integration
💡Area Under a Curve
💡Integration by Substitution
💡Integration Sign
💡Examination Question
💡Trapezium Rule
Highlights
Integration is the opposite of differentiation.
Confidence in differentiation is recommended before studying integration.
Introduction to definite and indefinite integration.
Difficulty level increases quickly as content progresses.
Integration involves adding 1 to the power and dividing the coefficient by the new power.
The necessity to include a constant 'C' in indefinite integration to account for potential constants lost during differentiation.
Integration sign and 'dx' notation is used to denote the function to be integrated.
Practical application of integration is to find the area under a curve.
Differentiation is used for rates of change and finding the gradient of a curve, while integration is for area.
Definite integration requires limits and is used to calculate the area between the curve and the x-axis within those limits.
Integration can result in positive or negative areas depending on the curve's position relative to the x-axis.
The process of sketching the graph is essential for understanding the area under the curve.
Exam questions often require a combination of integration and geometric knowledge, such as calculating the area of a trapezium.
The importance of careful calculation and checking work when performing definite integration to avoid mistakes.
Integration can be used to solve complex exam problems involving finding coordinates of points on a curve and calculating shaded regions.
The use of creative thinking in conjunction with integration to solve exam questions.
The importance of practicing integration problems to solidify understanding and improve problem-solving skills.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: