ANOVA (Analysis of variance) simply explained
TLDRThis video introduces the concept of Analysis of Variance (ANOVA), specifically the one-way or single-factor ANOVA without repeated measurements. It explains why ANOVA is used instead of a t-test when comparing more than two independent groups and discusses how it assesses statistically significant differences between group means. The video uses an example of comparing the ages of users of different statistical software to illustrate how ANOVA works. It also covers the null and alternative hypotheses involved in ANOVA, explains variance within and between groups, and demonstrates how to calculate ANOVA using statistical software like DataTab.
Takeaways
- 🔍 **Analysis of Variance (ANOVA) Overview**: ANOVA is used to determine if there are statistically significant differences between three or more groups.
- 📈 **ANOVA vs. T-test**: ANOVA extends the concept of the t-test for independent samples to more than two groups, whereas t-test is used for comparing two groups.
- 👥 **Independence of Samples**: In ANOVA, as with t-tests, the groups must be independent, meaning members of one group are unrelated to those in another.
- 💡 **Research Question Clarity**: ANOVA helps to answer whether there is a difference in a population between different groups in relation to a dependent variable.
- 🧐 **Null Hypothesis**: The null hypothesis in ANOVA states that there are no differences between the means of the groups in the population.
- 🚫 **Alternative Hypothesis**: The alternative hypothesis suggests that there is a difference between at least two group means.
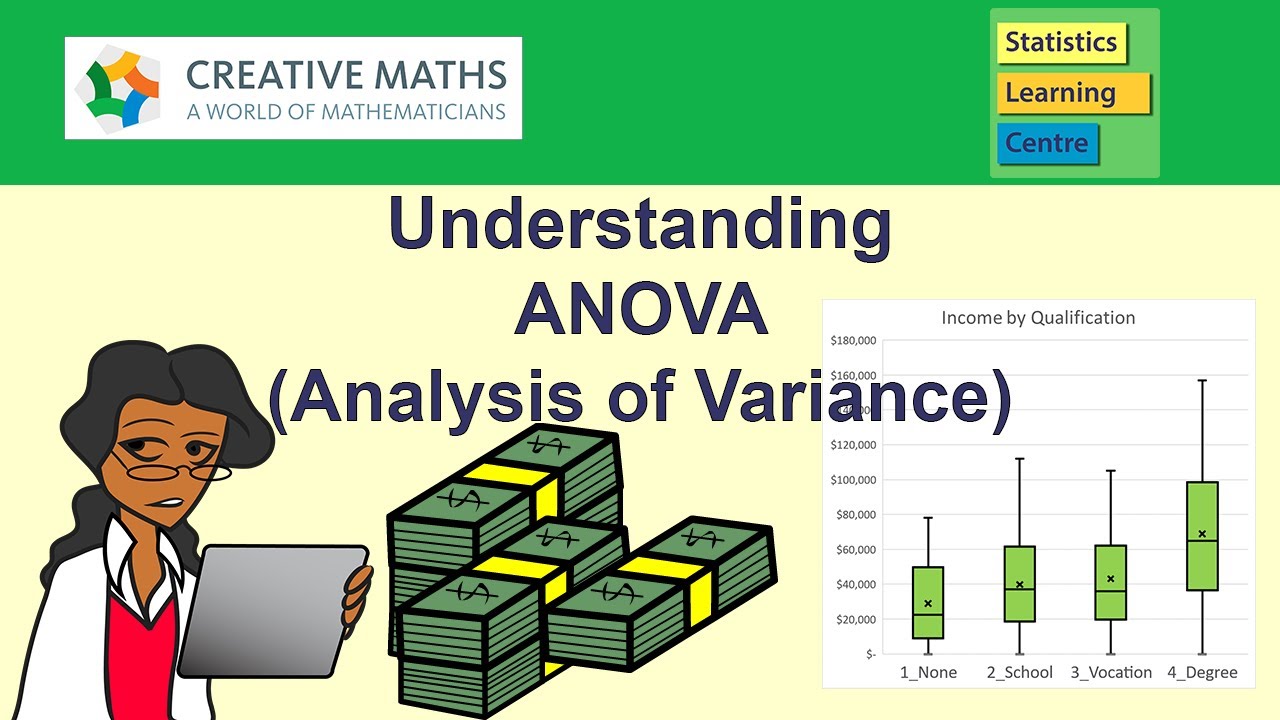
- 📊 **Graphical Representation**: ANOVA can be visualized by comparing the variance within and between groups to understand the influence on a dependent variable.
- 📉 **Variance Explanation**: The goal is to explain some of the variance in a dependent variable by categorizing into different groups.
- 📝 **Calculation Methods**: ANOVA can be calculated manually or using statistical software, with the latter being more practical and common.
- 🌐 **Online Tools**: Tools like DataTab can be used online to easily perform ANOVA by inputting data and selecting the appropriate variables.
- ✅ **Assumptions Check**: Before conducting ANOVA, it's important to check its assumptions, which can often be done within statistical software.
- 📚 **Understanding the P-value**: The p-value resulting from ANOVA indicates the probability of observing the data if the null hypothesis were true; a low p-value suggests a significant difference.
Q & A
What is the primary purpose of an Analysis of Variance (ANOVA)?
-The primary purpose of an ANOVA is to check whether there are statistically significant differences between more than two groups. It is an extension of the t-test for independent samples, allowing for the comparison of means across multiple groups.
What is the difference between a one-way ANOVA and a two-way ANOVA?
-A one-way ANOVA, also known as single-factor ANOVA, is used when there is one independent variable with at least three groups or categories. A two-way ANOVA, on the other hand, involves two independent variables, each with at least two categories, to determine the interaction effect between the variables on the dependent variable.
What is the null hypothesis in an ANOVA?
-The null hypothesis in an ANOVA states that there are no differences between the means of the individual groups in the population. It assumes that any variation observed is due to random chance.
What is the alternative hypothesis in an ANOVA?
-The alternative hypothesis (H1) in an ANOVA suggests that there is a difference between at least two group means in the population, indicating that the groups are not all equal.
How does ANOVA help in understanding the variance within and between groups?
-ANOVA helps in understanding the variance by partitioning the total variability in the data into two components: variance within groups (which is due to random chance) and variance between groups (which is due to the differences between the group means). This allows us to determine if the variation can be explained by the grouping variable.
What is the role of the dependent variable in an ANOVA?
-The dependent variable in an ANOVA is the outcome or the variable that is being measured and is expected to change as a result of the differences between the groups defined by the independent variable. It is the variable that we want to know if it is influenced by the independent variable.
What is a p-value in the context of ANOVA, and how is it interpreted?
-The p-value in ANOVA is a statistical measure that indicates the strength of the evidence against the null hypothesis. A low p-value (typically ≤ 0.05) suggests that the observed differences between group means are statistically significant and not likely due to random chance, leading to the rejection of the null hypothesis.
How can one calculate ANOVA without using statistical software?
-While it's rare to calculate ANOVA by hand due to its complexity, one could theoretically perform the calculations manually using formulas that involve computing the sum of squares between groups, within groups, and total sum of squares, followed by determining the mean squares and using these to calculate the F-statistic and the associated p-value.
What are the assumptions of ANOVA that should be checked before conducting the test?
-The assumptions of ANOVA include normality of the population distribution, homogeneity of variances (equal variance across groups), independence of observations, and the use of interval or ratio level data. Checking these assumptions is crucial for the validity of the ANOVA results.
How does using a statistical software like DataTab simplify the process of conducting an ANOVA?
-Statistical software like DataTab simplifies the ANOVA process by automating the complex calculations involved. Users can input their data into the software, select the appropriate variables, and the software will calculate the ANOVA test, providing results such as the F-statistic, p-value, and checking of assumptions.
What is the significance of the independent variable in an ANOVA?
-The independent variable in an ANOVA is the factor or characteristic that is manipulated or categorized in the study, with the aim of observing its effect on the dependent variable. It is the variable that defines the groups being compared in the analysis.
Can ANOVA be used to determine the direction of a causal relationship?
-No, ANOVA is a tool for determining if there are statistically significant differences between group means but it does not provide information about the direction of a causal relationship. It can indicate that a difference exists but not the cause of that difference.
Outlines
📊 Introduction to ANOVA
The first paragraph introduces the concept of Analysis of Variance (ANOVA), explaining its purpose and how it extends the t-test for independent samples to compare more than two groups. It discusses the need for ANOVA when there are statistically significant differences to be checked between multiple groups. An example is provided where the founder of a statistical software company is interested in the age differences among users of different software. The paragraph also outlines the research question that ANOVA can answer, which is whether there is a difference in the population between different groups in relation to a dependent variable. It concludes by introducing the null and alternative hypotheses associated with ANOVA.
📈 Understanding ANOVA Graphically and Calculation Methods
The second paragraph delves into the graphical representation of ANOVA and how it can be used to explain variation within a dataset, such as salary across different groups. It contrasts two scenarios: one where group division significantly explains variance and another where it does not. The paragraph then transitions into the calculation of ANOVA, mentioning that while manual calculation is not common, understanding the process is beneficial. It highlights the use of statistical software like DataTab for conducting ANOVA and provides a step-by-step guide on how to perform the calculation using the software. The summary also mentions checking the assumptions of ANOVA and concludes with a note of thanks to the viewers.
Mindmap
Keywords
💡Analysis of Variance (ANOVA)
💡One-way ANOVA
💡Statistical significance
💡Independent samples
💡Dependent variable
💡Independent variable
💡Null hypothesis
💡Alternative hypothesis
💡p-value
💡DataTab
💡Assumptions of ANOVA
Highlights
Analysis of variance (ANOVA) is used to check for statistically significant differences between more than two groups.
ANOVA is an extension of the t-test for independent samples, applicable when comparing more than two groups.
The independent t-test is used to compare the means of two independent groups, such as differences in salary between men and women.
For more than two independent groups, ANOVA is utilized instead of the t-test.
ANOVA without repeated measures is used when there are at least three independent samples.
The research question with ANOVA is to determine if there is a difference in a population between different groups of the independent variable in relation to the dependent variable.
The independent variable in the example is the statistical software used, with three groups: Datadeb, SPSS, and R.
The dependent variable in the example is the age of the software users.
ANOVA does not provide information about the direction of the causal relationship.
The null hypothesis in ANOVA assumes no difference in the means of the individual groups in the population.
The alternative hypothesis suggests that there is a difference between at least two group means.
Graphically, ANOVA examines the dispersion of a variable like salary and how much variation can be explained by grouping.
The variance within groups and between groups is compared to determine the influence of the independent variable on the dependent variable.
Statistical software like Datadeb can be used to calculate ANOVA, eliminating the need for manual calculations.
Datadeb.net provides an online platform for easily calculating ANOVA by entering data into a table and selecting the appropriate variables.
The p-value resulting from ANOVA can be interpreted with the help of summary statistics provided by the software.
Assumptions of ANOVA can be checked within the software to ensure the validity of the results.
The video provides a comprehensive guide on understanding and calculating ANOVA using Datadeb.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: