The Quotient Rule

Sun Surfer Math

16 Feb 202222:03

EducationalLearning

32 Likes 10 Comments

TLDRThe video script provides a comprehensive overview of the quotient rule in calculus, which is essential for finding the derivative of a quotient of two functions. The presenter explains that the quotient rule is closely related to the product rule, with the key difference being the presence of a minus sign in the formula. The script walks through several examples to illustrate the application of the quotient rule, including functions involving polynomials, radicals, exponential functions, and logarithms. Each example demonstrates the process of identifying the numerator and denominator, applying the rule, and simplifying the result. The video emphasizes the importance of understanding the quotient rule for solving more complex calculus problems and encourages viewers to practice to become proficient in its application.

Takeaways

📚 The Quotient Rule is a mathematical formula used to find the derivative of a quotient of two functions, f(x)/g(x).
➗ The formula for the Quotient Rule is (f'(x)g(x) - f(x)g'(x)) / (g(x))^2, which is similar to the Product Rule but with a minus sign.
🔍 The numerator of the Quotient Rule involves the derivative of the numerator function times the denominator function minus the numerator function times the derivative of the denominator function.
📉 The denominator is the square of the denominator function, g(x).
📈 Simplification is key when applying the Quotient Rule, often involving distributing and combining like terms.
🌟 Memorizing the Product Rule can help in remembering the Quotient Rule, as they are closely related except for the sign difference.
📌 Example problems in the script demonstrate the application of the Quotient Rule with various functions, including polynomials, radicals, exponentials, and logarithms.
🛠️ Exponents and radicals are common in Quotient Rule problems, requiring the use of exponent rules for simplification.
📘 Factoring is a useful technique in simplifying the expressions resulting from applying the Quotient Rule.
📝 When dealing with complex fractions, multiplying the numerator and denominator by a common factor can simplify the expression.
🧮 The Quotient Rule can handle functions involving e^x, logarithms, and other transcendental functions, showcasing its versatility in calculus.

Q & A

What is the quotient rule used for?
-The quotient rule is used to find the derivative of the quotient of two functions.
How is the quotient rule similar to the product rule?
-The quotient rule is similar to the product rule in that the numerator of the quotient rule looks a lot like the product rule, with the only difference being a minus sign instead of a plus sign.
What is the general formula for the quotient rule?
-The general formula for the quotient rule is (f(x) / g(x))' = (f'(x) * g(x) - f(x) * g'(x)) / (g(x))^2.
What is the derivative of 3x + 1 with respect to x?
-The derivative of 3x + 1 with respect to x is 3.
How is the derivative of a function involving square roots simplified in the quotient rule?
-When the derivative involves square roots, you can simplify by canceling out the square roots and then combining like terms over the common denominator.
What is the derivative of e^x with respect to x?
-The derivative of e^x with respect to x is e^x.
How do you handle the derivative of a logarithmic function in the quotient rule?
-The derivative of the logarithm of x, log(x), with respect to x is 1/x. This is used in the numerator or denominator of the quotient rule as needed.
What is the process for simplifying a complex fraction derived from the quotient rule?
-To simplify a complex fraction, you can multiply both the numerator and the denominator by the reciprocal of the denominator to eliminate the complex fraction form.
What is the final simplified form of the derivative of (sqrt(x) + 1) / (sqrt(x) - 1)?
-The final simplified form of the derivative is -1/(sqrt(x) * (sqrt(x) - 1)^2).
How do you treat the exponents when applying the quotient rule to functions with exponents?
-You apply the exponent rules, such as combining like terms and factoring out common terms, to simplify the expression before or after applying the quotient rule.
What is the role of the denominator g(x) in the quotient rule?
-The denominator g(x) is used as a common factor in the denominator of the final simplified form of the derivative after applying the quotient rule.

Outlines

00:00

📚 Introduction to the Quotient Rule

The first paragraph introduces the quotient rule, which is a mathematical technique used to find the derivative of a quotient of two functions. The rule is presented in a formulaic way, and its similarity to the product rule is highlighted, with the key difference being the presence of a minus sign. An example is given to illustrate how to apply the rule by finding the derivative of a function that is the quotient of two other functions, (3x + 1) / (5x - 4). The process involves simplifying the expression by distributing and combining like terms.

05:02

🔍 Applying the Quotient Rule with Polynomials

The second paragraph delves into applying the quotient rule with polynomial functions. It demonstrates the process of finding the derivative of (3x^2 - 5x + 2) / (2x^2 - 3). The explanation includes multiplying binomials, simplifying the resulting expression, and combining like terms. The final simplified derivative is obtained after distributing negative signs and canceling out terms.

10:03

🌟 Derivatives of Radical and Square Root Functions

The third paragraph focuses on the application of the quotient rule to functions involving radicals and square roots. It shows how to find the derivative of (sqrt(x + 1)) / (sqrt(x - 1)) and (x + x^2) / (2sqrt(x)). The process involves multiplying by the conjugate to simplify the expression and combining like terms. The final results are simplified by canceling out common factors and reducing the exponents where possible.

15:04

📈 Derivatives of Exponential and Logarithmic Functions

The fourth paragraph explores the quotient rule with exponential and logarithmic functions. It presents the derivatives of e^x / x and e^x / log(x). The approach involves factoring out common terms, dealing with complex fractions by multiplying by the reciprocal of the denominator, and simplifying the expressions. The final results are simplified derivatives that are expressed in terms of exponential and logarithmic functions.

20:06

🎓 Final Examples and Simplification Techniques

The fifth and final paragraph provides additional examples of applying the quotient rule, including the derivative of (log(x)) / e^x. It emphasizes the importance of multiplying by the conjugate to eliminate complex fractions and factoring out common terms. The summary concludes with a reminder of the quotient rule's utility in calculus and the importance of practice in mastering its application.

Mindmap

Keywords

💡Quotient Rule

The Quotient Rule is a mathematical theorem used to find the derivative of a quotient of two functions. It is central to the video's theme as it is the main rule being explained and applied. The rule states that the derivative of f(x)/g(x) is [(f'(x)g(x)) - (f(x)g'(x))] / (g(x))^2. It is used throughout the video to derive various functions.

💡Derivative

A derivative in calculus represents the rate of change of a function with respect to its variable. It is a fundamental concept in the video, as the Quotient Rule is a method for finding derivatives. The script provides several examples of derivatives, such as the derivative of 3x + 1 or the square root of x + 1.

💡Product Rule

The Product Rule is another mathematical rule used to find the derivative of a product of two functions. It is mentioned in the script as being similar to the Quotient Rule, with the key difference being the presence of a minus sign in the Quotient Rule instead of a plus sign in the Product Rule. It helps in understanding the structure of the Quotient Rule.

💡Numerator

In a fraction, the numerator is the top number. In the context of the Quotient Rule, the numerator of the derivative is f'(x)g(x) - f(x)g'(x), which is derived from the product rule and is a key part of the formula explained in the video.

💡Denominator

The denominator is the bottom part of a fraction. In the Quotient Rule, the denominator is (g(x))^2, which is the square of the function in the denominator of the original quotient. It is used in every example in the video to complete the derivative calculation.

💡Function

A function in mathematics is a relation between a set of inputs and a set of permissible outputs with the property that each input is related to exactly one output. The video discusses various functions, such as f(x) and g(x), and their derivatives, which are the core of applying the Quotient Rule.

💡Exponent

An exponent is a mathematical notation that indicates the number of times a base is multiplied by itself. Exponents are used in the video when dealing with powers of x, such as in the function 2x^2 - 3 or x^(1/2). Understanding exponents is crucial for simplifying expressions derived using the Quotient Rule.

💡Simplify

To simplify in mathematics means to reduce a complex expression to a more straightforward or standard form. The script demonstrates the process of simplifying derivatives obtained through the Quotient Rule, such as combining like terms or factoring out common factors.

💡Square Root

A square root is a value that, when multiplied by itself, gives the original number. The square root function, such as √x, appears in several examples in the script. It is important because its derivative is a key component in applying the Quotient Rule to radical expressions.

💡Logarithm

A logarithm is the inverse operation to exponentiation, the power to which a number (base) must be raised to produce a given number. The script touches on the derivative of a logarithmic function, which is 1/x, and how it fits into the Quotient Rule when the denominator is a logarithm.

💡Exponential Function

An exponential function is a function of the form f(x) = a^x, where a is a constant. In the video, e^x is used as an example of an exponential function, and its derivative, e^x, is discussed in the context of applying the Quotient Rule.

Highlights

The quotient rule is used to find the derivative of the quotient of two functions.

The derivative of f(x)/g(x) is similar to the product rule but with a minus sign.

The rule includes a denominator squared term, g(x)^2.

The numerator for the quotient rule is always f(x) * f'(x) - g(x) * g'(x).

An example is provided to demonstrate the application of the quotient rule.

The process of simplifying the numerator and denominator is shown step by step.

Terms can be canceled out in the numerator to simplify the derivative.

Differentiation of functions involving x^2, x, and constants is demonstrated.

The derivative of square root functions and their simplification is explained.

The concept of combining like terms and using common denominators is covered.

The derivative of exponential functions e^x with respect to x is e^x.

The derivative of logarithmic functions with respect to x is 1/x.

Complex fractions are simplified by multiplying numerator and denominator by a common factor.

Exponential and logarithmic functions are combined in the quotient rule example.

The final simplified form of the derivative is obtained after factoring and canceling.

The importance of exponent rules and converting between rational and exponents/radicals is emphasized.

The video concludes with a comprehensive example involving e^x, x, and 1/x.

Transcripts

Browse More Related Video

The Quotient Rule for Derivatives — Topic 58 of Machine Learning Foundations

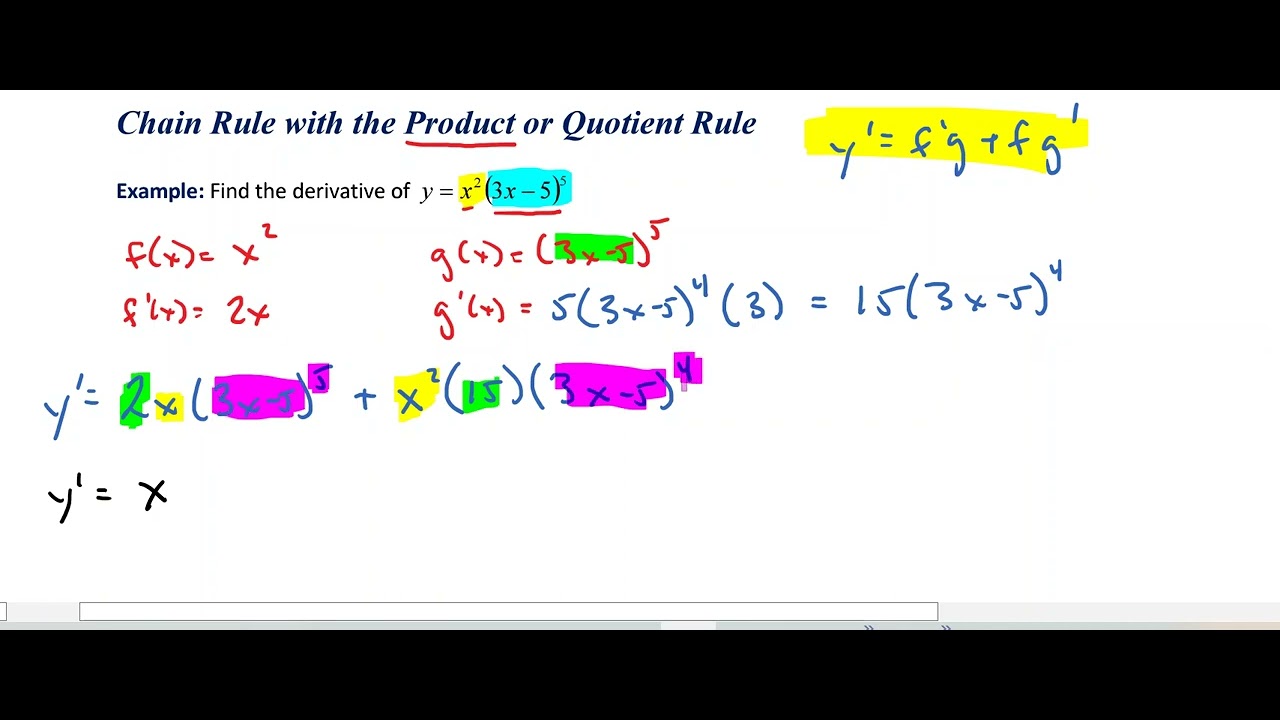
The Chain Rule - Part 2

Math 1325 Lecture 9 5 - Product & Quotient Rule

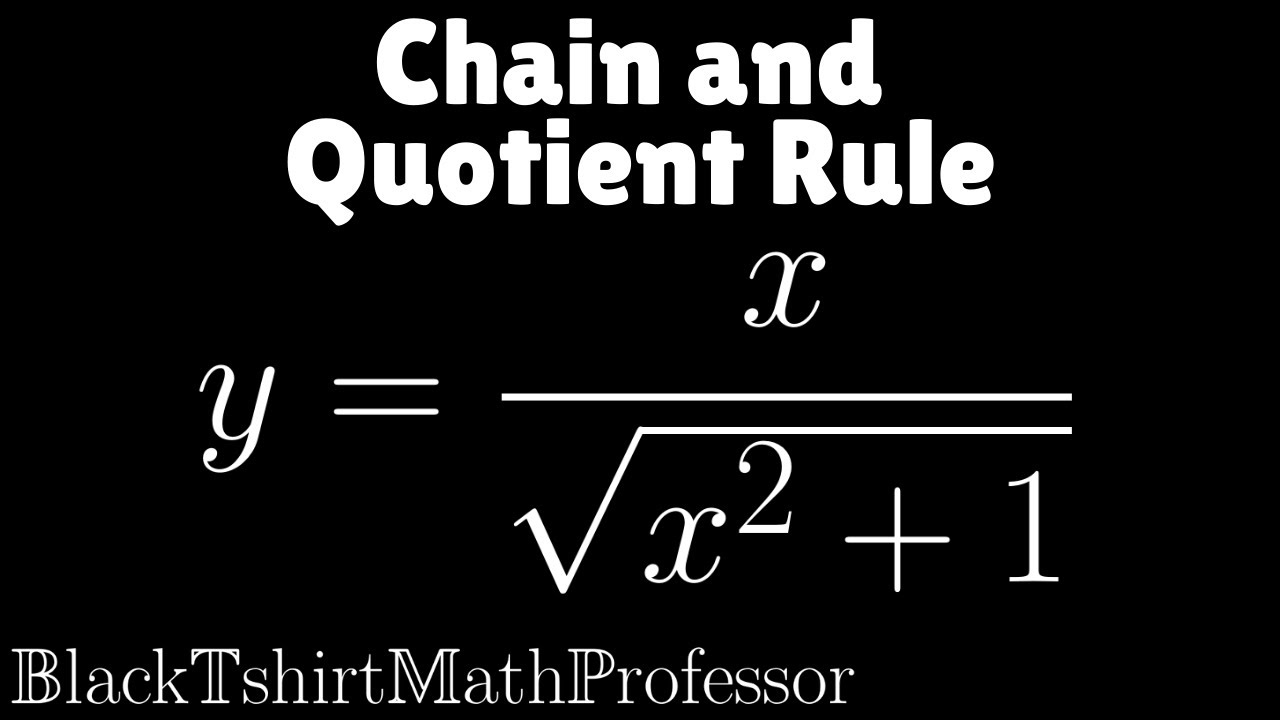
Chain Rule with the Quotient Rule Problem 3 (Calculus 1)

Quotient rule from product & chain rules | Derivative rules | AP Calculus AB | Khan Academy

Derivatives of Composite Functions - Chain Rule, Product & Quotient Rule

Related Tags

Quotient Rule Derivatives Calculus Mathematics Educational Product Rule Simplification Complex Fractions Logarithms Exponential Functions