How to Graph Tangent and Cotangent (Precalculus - Trigonometry 13)

Professor Leonard

22 Jun 202126:13

EducationalLearning

32 Likes 10 Comments

TLDRThe video script discusses the graphs of tangent and cotangent functions, drawing parallels to the previously covered sine and cosine graphs. It explains the origin of these functions graphically by examining the unit circle. The video aims to clarify why tangent and cotangent have vertical asymptotes and x-intercepts at certain intervals, and how they relate to each other. It delves into the periodic nature of these functions, highlighting that the period of tangent and cotangent is π, which is half that of the sine and cosine functions. The script also touches on the concepts of even and odd functions and the importance of understanding the key features of the graphs, such as the behavior at the ends, center, and corners of the period. This foundational understanding sets the stage for subsequent videos that will cover transformations of the tangent and cotangent graphs.

Takeaways

📐 The tangent function is the ratio of the y-coordinate to the x-coordinate on a unit circle, which leads to its characteristic graph with vertical asymptotes and x-intercepts.
🔄 Tangent has a period of π, which is half that of the sine and cosine functions, meaning its pattern repeats every π interval.
🚫 Tangent is undefined wherever the x-coordinate on the unit circle is zero, which occurs at odd multiples of π/2, leading to vertical asymptotes at these points.
➗ The x-intercepts of the tangent function occur at integer multiples of π, as these are the points where the y-coordinate is zero while the x-coordinate is not.
🔢 The range of the tangent function is all real numbers, as it can take on values from positive to negative infinity due to the nature of the ratio y/x.
🔁 Tangent is an odd function, which means it is symmetric about the origin, and its graph will reflect across the origin if rotated 180 degrees.
🤔 Cotangent is the reciprocal of the tangent function, which swaps the positions of the zeros and vertical asymptotes of the tangent function.
🛑 The cotangent function has vertical asymptotes at integer multiples of π, where the tangent function has x-intercepts.
🔄 Like tangent, cotangent also has a period of π, but its graph starts at a different point on the x-axis, typically from 0 to π.
🔢 The range of the cotangent function is also all real numbers, similar to the tangent function, due to the reciprocal nature of the function.
🔁 Cotangent is also an odd function, maintaining symmetry about the origin, and its key points are phase shifted compared to the tangent function.

Q & A

What are the key features of the tangent function's graph that we should pay attention to?
-The key features of the tangent function's graph include its vertical asymptotes, x-intercepts, and its relationship to the unit circle. The vertical asymptotes occur when the denominator (x-coordinate) is zero, which happens at odd multiples of pi/2. The x-intercepts occur when the numerator (y-coordinate) is zero, which happens at integer multiples of pi. Understanding these features is crucial for transforming the graph of the tangent function.
Why does the tangent function have vertical asymptotes?
-The tangent function has vertical asymptotes because it is a ratio of the y-coordinate to the x-coordinate on the unit circle. When the x-coordinate is zero, the function becomes undefined as division by zero is not possible, hence a vertical asymptote is present at these points.
What is the period of the tangent function?
-The period of the tangent function is π (pi). This is because the tangent function repeats its values every π radians, which is half the period of the sine and cosine functions.
How are the x-intercepts of the tangent function determined?
-The x-intercepts of the tangent function occur where the y-coordinate on the unit circle is zero, while the x-coordinate is not zero. This happens at integer multiples of π, specifically at 0, ±π, ±2π, and so on.
What is the domain of the tangent function?
-The domain of the tangent function is all real numbers except where the function is undefined, which is at odd multiples of π/2. Therefore, the domain is all real numbers except where x equals π/2 + kπ, where k is any integer.
What is the range of the tangent function?
-The range of the tangent function is all real numbers. This is because the tangent function can take on any real number value as it compares the y-coordinate to the x-coordinate on the unit circle, which can vary from negative infinity to positive infinity.
What is the relationship between the tangent and cotangent functions?
-The cotangent function is the reciprocal of the tangent function. Where the tangent function is y/x, the cotangent function is x/y. This means that the zeros of the tangent function correspond to the vertical asymptotes of the cotangent function, and vice versa.
Why are the tangent and cotangent functions considered odd functions?
-The tangent and cotangent functions are considered odd functions because they are symmetric about the origin. This means that for every point (x, y) on the graph of an odd function, there is a corresponding point (-x, -y) that also lies on the graph.
What are the key points to identify when graphing the tangent function?
-When graphing the tangent function, one should identify the period, mark the vertical asymptotes at the ends of the period, place an x-intercept at the center of the period, and note the values at the quarters of the period (which are ±1).
How does the graph of the cotangent function differ from that of the tangent function?
-The graph of the cotangent function differs from the tangent function in that the x-intercepts of the tangent function become the vertical asymptotes of the cotangent function, and the vertical asymptotes of the tangent function become the x-intercepts of the cotangent function. However, both functions have the same period of π.
What is the significance of understanding the transformations of the tangent and cotangent graphs?
-Understanding the transformations of the tangent and cotangent graphs is important for manipulating and analyzing the functions in various mathematical and real-world applications. It allows for the prediction of function behavior, the creation of new functions through transformations, and the solution of complex trigonometric problems.
What are the key points to remember when transforming the tangent function in future videos?
-When transforming the tangent function, it's important to remember that the period of the function is π and that vertical asymptotes occur at the ends of each period. Additionally, there is an x-intercept at the center of the period, and the values at the quarters of the period are ±1, which will be key points for manipulation.

Outlines

00:00

📈 Introduction to Tangent and Cotangent Graphs

The video begins with an introduction to the graphs of tangent and cotangent functions, drawing parallels to the previously discussed sine and cosine graphs. The focus is on understanding the graphical representation of these functions, their origins, and the key features that define their appearance, such as vertical asymptotes and x-intercepts. The video promises to delve into the periodicity and the relationship between tangent and cotangent, as well as their even or odd nature.

05:01

🔢 Understanding Tangent's Undefined Values and Vertical Asymptotes

This paragraph explores why the tangent function has undefined values and vertical asymptotes. Unlike sine and cosine, tangent is a ratio of y to x, which becomes undefined when the denominator (x) is zero. The angles at which x equals zero on the unit circle are identified as π/2, 3π/2, and their negative counterparts, leading to vertical asymptotes at these points. The domain of tangent is all real numbers except for these specific angles, and the period of the tangent function is established as π.

10:02

📉 The Range and Behavior of the Tangent Function

The range of the tangent function is discussed, contrasting it with the range of sine and cosine. Since tangent can take on values greater than one or less than negative one, it can extend to positive and negative infinity. The behavior of the tangent graph is described as an 'S' curve, and key angles such as π/4, 3π/4, and 5π/4 are used to illustrate the pattern of the function's increase and decrease. The importance of understanding the function's period and the repetition of its pattern every π is emphasized.

15:02

🤔 Characteristics of the Cotangent Function

The cotangent function is introduced as the reciprocal of the tangent function, which swaps the positions of zeros and vertical asymptotes. The cotangent's x-intercepts occur at the angles where the tangent function was undefined, and vice versa. The video explains that cotangent's domain excludes values where the tangent had x-intercepts, and its range is all real numbers, similar to tangent. The period of cotangent is also π, and it is identified as an odd function due to its symmetry about the origin.

20:03

🔁 Reciprocal Relationship and Graphs of Tangent and Cotangent

The reciprocal relationship between tangent and cotangent is further explored, noting that they share the same period but have different starting points for their graphs. The video discusses how the graphs of cotangent appear as phase shifts and reflections of the tangent graph. The domain and range of cotangent are outlined, and it is confirmed that cotangent, like tangent, is an odd function with a period of π. The video concludes by highlighting the importance of understanding the starting points and key points of the tangent and cotangent functions for future graph transformations.

25:03

📚 Memorizing Key Points for Graph Manipulation

The video emphasizes the need to memorize the starting points and key points of the tangent and cotangent functions, which are crucial for graph manipulation. The original periods for tangent and cotangent are specified as negative π/2 to π/2 and 0 to π, respectively. The video encourages viewers to understand these starting points and key points, as they will be essential for graph transformations in upcoming videos.

Mindmap

Keywords

💡Tangent

The tangent function, often abbreviated as 'tan', is a trigonometric function that measures the ratio of the y-coordinate to the x-coordinate for a point on the unit circle. In the video, tangent is discussed in the context of its graph, which has a period of π and exhibits vertical asymptotes at π/2 + kπ, where k is any integer. The tangent function is also described as an odd function, which means it is symmetric about the origin.

💡Cotangent

Cotangent, represented as 'cot' or 'cot(x)', is the reciprocal of the tangent function. It is also a trigonometric function that relates the x-coordinate to the y-coordinate on the unit circle. The video explains that cotangent has x-intercepts where tangent has vertical asymptotes and vice versa, and it shares the same period of π with tangent, but starts at a different point on the x-axis.

💡Unit Circle

A unit circle is a circle with a radius of one and is centered at the origin of the coordinate plane. It is fundamental in defining trigonometric functions like tangent and cotangent. In the video, the unit circle is used to illustrate the points from which the values of tangent and cotangent are derived, and how these points lead to the functions' respective graphs.

💡Vertical Asymptote

A vertical asymptote is a vertical line that a graph approaches but never crosses. In the context of the video, the tangent function has vertical asymptotes at π/2 + kπ, which are points where the function becomes undefined because the denominator (x-coordinate) of the ratio becomes zero. The video also discusses how cotangent has x-intercepts at these points, making them vertical asymptotes for cotangent.

💡X-Intercept

An x-intercept is a point where a graph crosses or touches the x-axis, meaning the y-coordinate is zero at that point. The video explains that the tangent function has x-intercepts at integer multiples of π, which are the points where the y-coordinate on the unit circle is zero. Conversely, cotangent has x-intercepts at the points where tangent has vertical asymptotes.

💡Period

The period of a function is the length of the interval over which the function's graph repeats. For both tangent and cotangent functions, as discussed in the video, the period is π. This means that the graph of these functions repeats its pattern every π units along the x-axis.

💡Odd Function

An odd function is a function that is symmetric about the origin, meaning that for every point (x, y) on the graph, there is a corresponding point (-x, -y). The video identifies the tangent function as an odd function, which is evident from its graph that is symmetric with respect to the origin.

💡Undefined

In mathematics, a function is said to be undefined at a certain point when it does not have a value at that point. In the context of the video, the tangent function is undefined at vertical asymptotes where the x-coordinate is zero, as the function attempts to divide by zero, which is not allowed in mathematics.

💡Domain

The domain of a function is the set of all possible input values (x-values) for which the function is defined. The video explains that the domain of the tangent function is all real numbers except for π/2 + kπ, where it is undefined due to division by zero. For cotangent, the domain is all real numbers except for the points where the function has vertical asymptotes, which are the integer multiples of π.

💡Range

The range of a function is the set of all possible output values (y-values) that result from applying the function to its domain. The video states that the range for both tangent and cotangent functions is all real numbers, reflecting the fact that the output of these functions can extend from negative infinity to positive infinity.

💡Graph Transformation

Graph transformation refers to the process of altering the graph of a function, typically through shifting, stretching, or reflecting. The video hints at the next topic, which will involve transforming the graphs of the tangent and cotangent functions. Understanding the original graphs and their key features, such as x-intercepts and asymptotes, is crucial for performing these transformations.

Highlights

This video discusses the graphs of tangent and cotangent, exploring their origins and graphical representations.

The video explains the relationship between tangent and the unit circle, and how it is derived from the ratio of y-coordinate to x-coordinate.

Tangent and cotangent graphs are analyzed for their periodicity, with a focus on identifying key features like vertical asymptotes and x-intercepts.

The concept of vertical asymptotes in the tangent function is introduced, explaining why they occur at every multiple of pi/2.

The video clarifies that tangent is undefined when the x-coordinate of the unit circle is zero, leading to vertical asymptotes.

Cotangent is introduced as the reciprocal of tangent, swapping zeros and vertical asymptotes.

The period of the tangent function is shown to be π, which is half that of the sine and cosine functions.

The domain of the tangent function is all real numbers except where it is undefined, specifically not including values of pi/2 + kπ.

The range of the tangent function is all real numbers, due to the nature of the ratio y/x as x approaches zero.

The video demonstrates that tangent is an odd function due to its symmetry about the origin.

Key points of the tangent graph are identified, including the behavior at the ends, center, and quarters of its period.

The video outlines how to transform the tangent and cotangent graphs, emphasizing the importance of understanding their key features.

Cotangent's graph is shown to be similar to tangent's but with a phase shift and reflection, maintaining the same period of π.

The domain and range of cotangent are discussed, mirroring those of tangent but with switched x-intercepts and vertical asymptotes.

The video emphasizes the importance of memorizing the starting points of the periods for both tangent and cotangent for graph manipulation.

The video concludes by reinforcing the understanding of the key points and the behavior of tangent and cotangent at the quarters of their periods.

Transcripts

Browse More Related Video

Graphing Transformations with Tangent and Cotangent (Precalculus - Trigonometry 14)

Graphing Trigonometric Functions

Trig - 0.5 Periodic and Even and Odd Function Properties

Trig - 0.3 Domains of Trigonometric Functions

Basic Properties of Trigonometric Functions (Precalculus - Trigonometry 8)

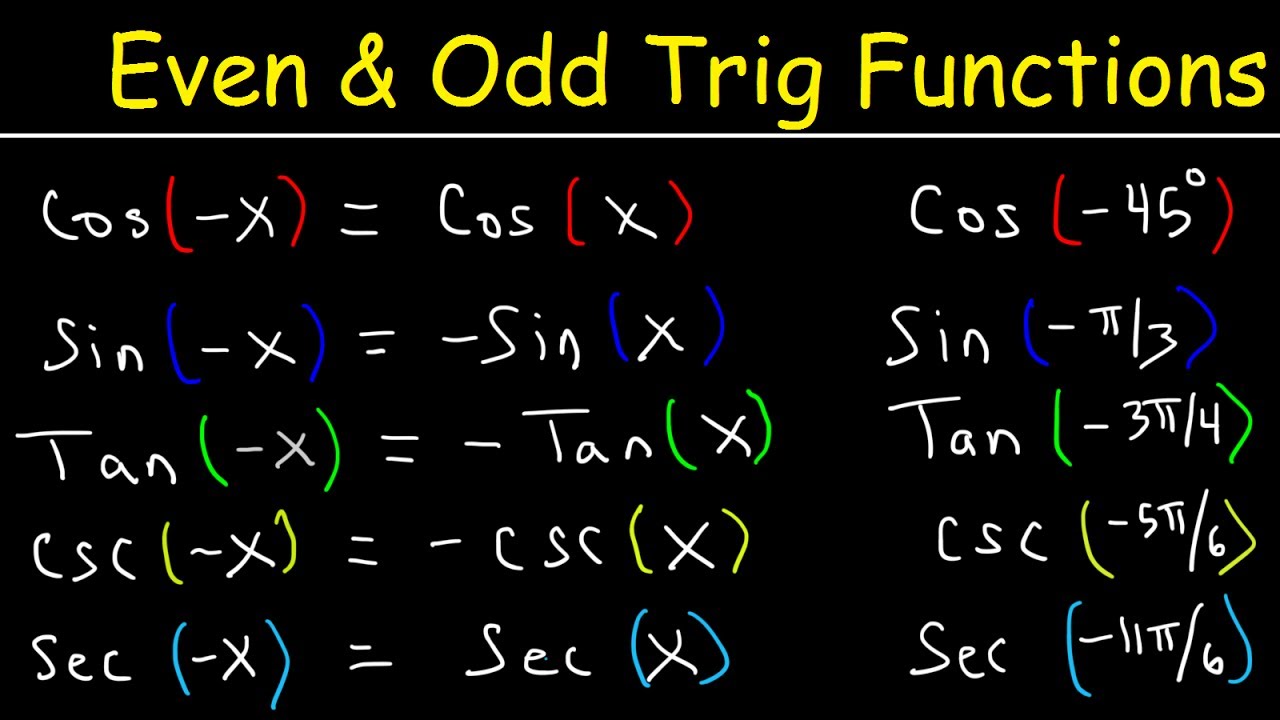
Even and Odd Trigonometric Functions & Identities - Evaluating Sine, Cosine, & Tangent

Related Tags

Trigonometry Graph Analysis Tangent Function Cotangent Function Vertical Asymptote Periodic Functions Math Transformations Unit Circle Trig Functions Sine Cosine Odd Functions