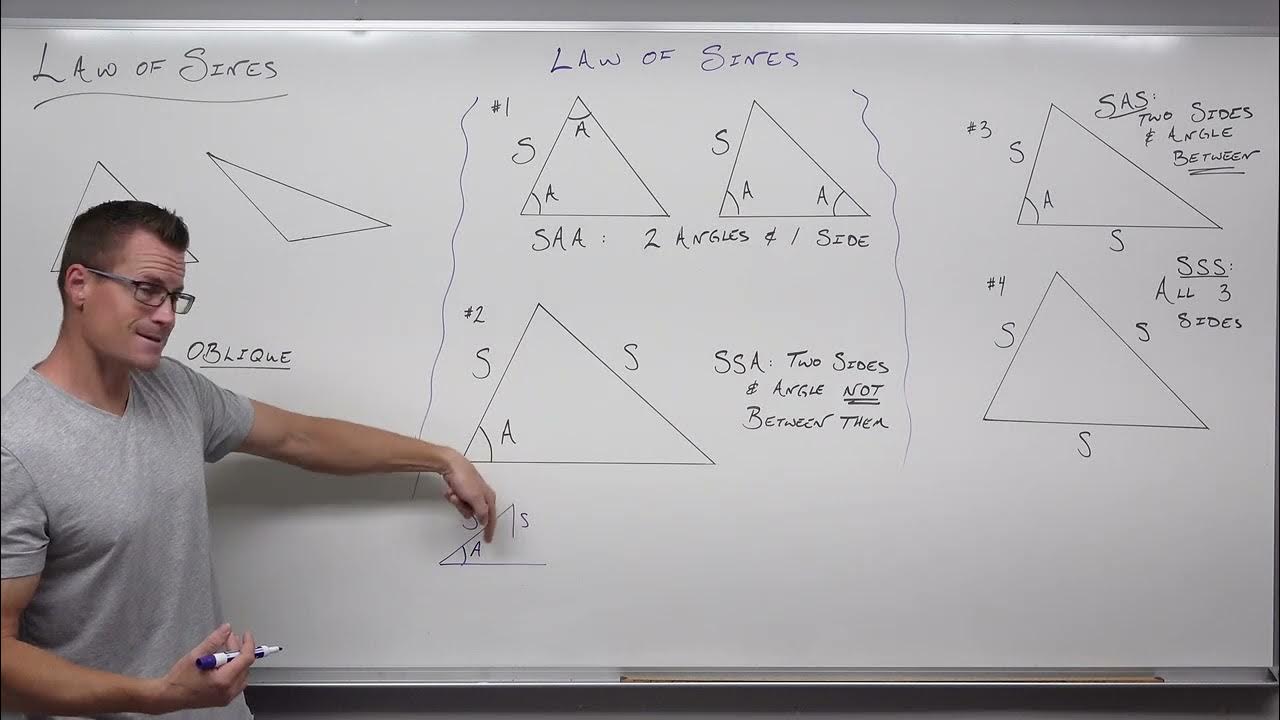
Why is Trigonometry so important?
TLDRThe video script delves into the fascinating world of trigonometry, a subject that is often misunderstood but is fundamental to understanding various aspects of geometry and physics. It begins by breaking down the term 'trigonometry' itself, explaining that it is derived from 'trigon', meaning three angles, and 'metry', referring to measurement. The speaker emphasizes that trigonometry is not just about measuring triangles but also plays a crucial role in understanding periodic phenomena and cyclical patterns, such as those found in simple harmonic motion and projectile motion. The script illustrates how trigonometric functions are used to describe the motion of objects in two dimensions, such as in circular motion, where concepts like linear and angular velocity are derived from the underlying geometry of triangles. The essence of the video is to highlight the pervasive nature of trigonometry, showing that it is not limited to the study of triangles alone but is a fundamental tool for analyzing a wide range of natural and physical phenomena.
Takeaways
- 📐 The term 'trigonometry' comes from 'trigonon' meaning 'triangle' and 'metron' meaning 'measure', indicating it's about measuring triangles.
- 🔍 Trigonometry is fundamental to understanding periodic phenomena because circles, which represent cycles, are composed of an infinite number of triangles.
- 🌀 Trigonometric functions are used to describe simple harmonic motion, which includes any motion that oscillates or moves in a repetitive pattern.
- 🚀 Projectile motion, such as the trajectory of an object launched at an angle, is analyzed using trigonometry to determine its height and distance.
- ⭕ In circular motion, the concepts of linear and angular velocity are related to the angles and triangles formed as an object moves in a circular path.
- 📈 The 'metry' suffix in words like 'geography', 'topology', and 'optometry' is related to 'metric', which pertains to measurement, emphasizing the measurement aspect of trigonometry.
- 🔢 Trigonometry is not limited to just triangles but extends to any situation involving angles, making it a versatile tool in various scientific and mathematical contexts.
- 🔄 The presence of triangles in the structure of circles allows trigonometry to be applied to cyclic and periodic processes.
- 🧭 Trigonometry is essential for understanding and measuring phenomena that involve rotation or circular paths, such as the motion of objects on a string around a circle.
- 📉 Trigonometry underlies the study of waves and oscillations, which are common in physics and engineering.
- 🌟 The study of trigonometry begins with simple shapes but extends to complex and seemingly unrelated areas due to the ubiquity of triangles in geometric forms.
Q & A
What is the meaning of the term 'trigonometry'?
-Trigonometry is the study of the relationships involving lengths and angles of triangles, and it is derived from the Greek words 'trigonon' meaning 'three angles' and 'metron' meaning 'measure'. It is used to measure triangles and has broader applications in various fields involving periodic or cyclic phenomena.
What does the prefix 'trigono-' in the word 'trigonometry' signify?
-The prefix 'trigono-' signifies 'three angles', indicating that the field of trigonometry is concerned with the study of triangles.
How is the suffix '-metry' related to the field of trigonometry?
-The suffix '-metry' is related to the concept of measurement. In the context of trigonometry, it implies that the field is about measuring angles and sides of triangles.
Why is trigonometry relevant to cyclic or periodic phenomena?
-Trigonometry is relevant to cyclic or periodic phenomena because circles, which are fundamental to such phenomena, can be divided into a series of triangles. This allows for the application of trigonometric principles to analyze and understand these cyclic patterns.
What is simple harmonic motion and how does it relate to trigonometry?
-Simple harmonic motion is a type of periodic motion where an object moves back and forth or around in a repetitive pattern, such as a pendulum swinging or a spring vibrating. It relates to trigonometry because the motion can be described using trigonometric functions, which model the oscillation in terms of sine and cosine waves.
How does trigonometry apply to projectile motion?
-Trigonometry applies to projectile motion by allowing for the calculation of the object's trajectory, including how high it will go and how far it will travel. This is done by analyzing the initial velocity and angle at which the object is launched, using trigonometric functions to break down the motion into horizontal and vertical components.
What is the relationship between linear velocity and angular velocity?
-Linear velocity is the rate of change of an object's position along a straight line, while angular velocity is the rate of change of an angle over time. In the context of circular motion, these two types of velocities are related through the radius of the circle; the linear velocity is the product of the angular velocity and the radius.
What is the significance of the tangent line in the context of circular motion?
-In circular motion, the tangent line at any point on the circle represents the direction of the object's instantaneous velocity at that point. Trigonometry is used to determine the relationship between the angle of motion and the velocity vector at any given point along the circle.
How does the concept of 'metrics' relate to the metric system?
-The term 'metrics' refers to the science of measurement, which is the basis of the metric system. The metric system is a decimal system of measurement that uses standardized units, such as meters for length, liters for volume, and grams for mass.
Why are triangles considered fundamental in trigonometry?
-Triangles are fundamental in trigonometry because the relationships between their angles and sides form the basis for the field. These relationships are encapsulated in trigonometric ratios (sine, cosine, and tangent) that are used to solve for unknown angles or sides in various geometric and real-world problems.
How does trigonometry extend beyond the study of triangles into other areas of mathematics and physics?
-Trigonometry extends beyond the study of triangles by providing tools to analyze and model periodic phenomena, such as sound and light waves, alternating electrical currents, and the motion of objects in physics. Its principles are also used in calculus, engineering, and even in the study of three-dimensional space.
What is the significance of the word 'geography' in relation to the concept of 'metry'?
-The word 'geography' is derived from 'geo-' meaning 'earth' and '-graphy', which is related to 'metry' and signifies 'writing' or 'description'. Thus, geography is the study of the earth's features and phenomena, which involves measurement and description, similar to how trigonometry involves the measurement of angles and sides in triangles.
Outlines
📐 Understanding the Roots of Trigonometry
The first paragraph delves into the etymology of the term 'trigonometry,' which is derived from 'trigon' meaning 'three angles' and 'metry' relating to measurement. It explains that trigonometry is fundamentally about measuring triangles. The speaker then expands on the concept, illustrating how trigonometry extends beyond its initial scope to encompass periodic phenomena and cyclic patterns, such as those found in simple harmonic motion and projectile motion. The paragraph concludes by highlighting the ubiquity of triangles in various forms of motion and the pervasive role of trigonometry in describing these motions.
🔍 Trigonometry's Hidden Presence in Nature and Science
The second paragraph emphasizes the omnipresence of triangles in trigonometry, suggesting that once one becomes aware of their presence, they can be seen everywhere. It builds on the idea that trigonometry starts with simple concepts but extends to a wide array of applications due to the hidden triangles found in various natural and scientific phenomena. The speaker reinforces the notion that trigonometry is not just limited to the study of geometric shapes but is a fundamental tool for understanding a vast range of cyclic and periodic processes.
Mindmap
Keywords
💡Trigonometry
💡Angles
💡Periodicity
💡Cyclic
💡Simple Harmonic Motion
💡Projectile Motion
💡Circular Motion
💡Linear Velocity
💡Angular Velocity
💡Tangent
💡Metric System
Highlights
Trigonometry is derived from the Greek words 'trigonon' meaning 'three angles' and 'metron' meaning 'measure', thus it's about measuring triangles.
The concept of 'trigon' refers to a three-angled shape, not necessarily a three-sided shape.
The suffix '-metry' in trigonometry relates to measurement, similar to how 'metric' is used in the context of the metric system.
Trigonometry extends beyond the basics of triangle measurements, finding its way into various fields due to the prevalence of triangles in many natural and mathematical phenomena.
Cyclic or periodic phenomena, such as those found in circles, are inherently described using trigonometric functions, despite not appearing to be directly related to triangles.
Every circle can be thought of as being composed of a series of triangles, which is why trigonometry is applicable in measuring circular motions.
Simple harmonic motion, which involves up and down or left and right movements, is described using trigonometric functions.
Projectile motion, where an object is launched at a certain angle and velocity, is analyzed using trigonometry to predict its trajectory.
In extension courses, circular motion is studied, which involves two-dimensional movement and is also described by trigonometric principles.
Linear velocity and angular velocity are compared using trigonometric concepts, which are fundamental to understanding circular motion.
Trigonometry is not limited to static triangles but is also applied to dynamic scenarios where triangles are formed in the process of motion.
The concept of tangents is used to describe the direction of motion in circular motion, which is inherently linked to triangles.
Trigonometry's application is widespread because triangles are a fundamental shape found in many mathematical and physical phenomena.
The speaker emphasizes that once you understand the principles of trigonometry, you can see how it applies to a wide range of subjects and problems.
Trigonometry begins with simple concepts but extends to complex applications due to the ubiquity of triangles in various forms of motion and geometry.
The study of trigonometry reveals how mathematical principles can be applied to real-world problems, such as those involving angles and periodic phenomena.
Understanding the etymology and fundamental principles of trigonometry provides insight into its broad applicability across different fields of study.
The speaker uses the analogy of 'seeing triangles everywhere' to illustrate how trigonometry can be applied to a wide array of problems, once the foundational concepts are grasped.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: