PreCalculus - Matrices & Matrix Applications (21 of 33) Using the Determinant to Find the Inverse

Michel van Biezen

30 Jun 201504:29

EducationalLearning

32 Likes 10 Comments

TLDRThis video tutorial introduces a quick method for finding the inverse of a 2x2 matrix, focusing on the use of determinants and matrix element manipulation. It explains that the inverse is calculated by swapping the positions of elements A and D and changing the signs of elements B and C, all divided by the determinant (AD - BC). A clear example is provided, demonstrating how to calculate the determinant, form the inverse matrix, and verify it by multiplying it with the original matrix to obtain the identity matrix. The video also teases a more general method for matrices of higher dimensions in a forthcoming tutorial.

Takeaways

📌 The video introduces two methods for finding the inverse of a matrix, with a focus on the specific technique for 2x2 matrices.
🔍 The method discussed is applicable only to 2x2 matrices and is a quick way to find the inverse.
👉 The inverse of a matrix A with elements a, b, c, and d is found by swapping a and d and changing the signs of b and c.
🧮 The determinant (denoted as D) of a 2x2 matrix is calculated as (ad - bc) and is crucial for finding the inverse.
⚙️ The formula for the inverse of a 2x2 matrix is given by 1/D * [a -b; -c d], where D is the determinant.
🌟 The video provides a step-by-step example of finding the inverse of a matrix with elements 2, 1, 5, and 3.
🔢 The determinant in the example is calculated as (2*3 - 1*5) which equals 1.
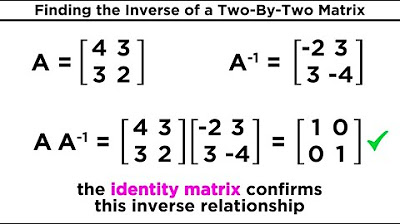
🔄 To verify the inverse, the video demonstrates multiplying the original matrix by its inverse, which should yield the identity matrix.
📈 The result of the multiplication confirms the correctness of the inverse, showing the identity matrix as proof.
🎥 The video concludes by mentioning that a more general method for finding the inverse of matrices of any dimension will be covered in the next video.
📚 The video serves as a tutorial for those looking to understand and apply the process of matrix inversion, particularly for 2x2 matrices.

Q & A

What is the main topic of the video?
-The main topic of the video is how to find the inverse of a 2x2 matrix.
How many methods are mentioned for finding the inverse of a matrix?
-Two basic methods are mentioned in the video, one specific for 2x2 matrices and another more general method for matrices of any dimension.
What is the determinant in the context of a 2x2 matrix?
-The determinant is the product of the diagonal elements of the matrix minus the product of the off-diagonal elements.
How is the inverse of a 2x2 matrix calculated?
-The inverse of a 2x2 matrix is calculated by taking the reciprocal of the determinant and then swapping the positions of elements A and D, and changing the signs of elements B and C.
What happens to elements B and C when finding the inverse of a matrix?
-When finding the inverse of a matrix, elements B and C change to the opposite sign; if B is positive, it becomes negative, and the same applies to C.
How do you verify the correctness of the calculated inverse?
-To verify the correctness of the calculated inverse, you multiply the original matrix by its inverse, which should result in the identity matrix.
What is the identity matrix?
-The identity matrix is a square matrix with ones on the diagonal and zeros elsewhere, such that when multiplied by another matrix, the original matrix is returned.
What are the elements of the example matrix provided in the video?
-The elements of the example matrix provided in the video are 2, 1, 5, and 3.
What is the determinant of the example matrix?
-The determinant of the example matrix is 1, calculated as (2 * 3) - (1 * 5).
What is the inverse of the example matrix?
-The inverse of the example matrix is the matrix [3, -1, -5, 2].
What will be covered in the next video?
-In the next video, a more general method for finding the inverse of a matrix will be shown, starting with a 2x2 matrix and then extending the technique to a 3x3 matrix.

Outlines

00:00

📚 Introduction to Finding the Inverse of a 2x2 Matrix

This paragraph introduces the topic of finding the inverse of a 2x2 matrix. It explains that there are two basic methods to do this, with the one presented being specific to 2x2 matrices. The other method, to be discussed in a subsequent video, is more general and applicable to matrices of any dimension, albeit more complex for larger matrices. The paragraph emphasizes the practicality and speed of the method being introduced, which relies on the concept of the matrix determinant and the transposition of matrix elements.

Mindmap

Keywords

💡2x2 matrix

A 2x2 matrix is a square array of numbers with two rows and two columns, used in mathematics to represent linear transformations or systems of equations. In the video, the 2x2 matrix is used to demonstrate a method for finding its inverse. The matrix example given, with elements 2, 1, 5, and 3, shows how matrices can be manipulated to find their inverses, an essential concept in linear algebra.

💡Inverse of a matrix

The inverse of a matrix is a key concept in linear algebra, representing a matrix that, when multiplied by the original matrix, yields the identity matrix. The video explains how to find the inverse of a 2x2 matrix specifically, showing the practical application of this concept by interchanging elements and changing the signs of the off-diagonal elements. The inverse is used to solve systems of linear equations and in various applications in physics and engineering.

💡Determinant

The determinant is a scalar value that can be computed from the elements of a square matrix and encapsulates certain properties of the matrix. In the video, the determinant is calculated as ad - bc for a 2x2 matrix (elements a, b, c, d). The determinant is crucial because the inverse of the matrix exists only when the determinant is non-zero. The video uses the determinant to demonstrate its role in calculating the inverse of the matrix.

💡Identity matrix

The identity matrix, usually denoted as I, is a square matrix in which all the elements of the principal diagonal are ones, and all other elements are zeros. The video concludes with the multiplication of a matrix and its inverse to yield the identity matrix, demonstrating the correctness of the inverse calculation. This concept is fundamental in matrix theory, emphasizing the matrix's role as the multiplicative identity in linear transformations.

💡Diagonal elements

Diagonal elements refer to the elements of a matrix that are located from the top left to the bottom right corner. In the video, these elements (a and d in a 2x2 matrix) are crucial in both the calculation of the determinant and the formation of the inverse matrix, where they are swapped. The manipulation of these elements illustrates their importance in matrix operations.

💡Off-diagonal elements

Off-diagonal elements in a matrix are those elements that are not on the main diagonal. In the video, the off-diagonal elements (b and c in a 2x2 matrix) are essential in calculating the determinant and are changed in sign when forming the inverse. This highlights how the properties of these elements affect the characteristics and inverse of the matrix.

💡Matrix multiplication

Matrix multiplication is a binary operation that produces a matrix from two matrices. The video demonstrates matrix multiplication to verify the inverse of a matrix, showing how the product of a matrix and its inverse results in the identity matrix. This process is fundamental in confirming that the inverse has been correctly calculated.

💡Linear algebra

Linear algebra is a branch of mathematics focusing on vector spaces and linear mappings between these spaces. It includes the study of lines, planes, and subspaces, but is also concerned with properties common to all vector spaces. The video specifically addresses linear algebra concepts such as matrices, determinants, and inverses, crucial for solving systems of linear equations and other applications.

💡General method

The general method mentioned in the video refers to a more universal approach for finding matrix inverses that can be applied to matrices of any dimension, not just 2x2. This method is contrasted with the specific method for 2x2 matrices, highlighting the necessity of different approaches depending on the matrix size and complexity. The video teases this method as a topic for future discussion, underscoring its relevance in more complex algebraic contexts.

💡Elementary operations

Elementary operations in the context of matrices involve operations such as row swapping, scalar multiplication of rows, and adding multiples of one row to another. These operations are implicit in the process of finding the inverse of a matrix as shown in the video, where elements are interchanged and signs are reversed. Understanding these operations is key in linear algebra, particularly in matrix manipulation and solving linear systems.

Highlights

Introduction to finding the inverse of a 2x2 matrix

Two basic methods for finding the inverse of a matrix, with one specific to 2x2 matrices

The determinant is crucial for calculating the inverse of a 2x2 matrix

Interchanging elements A and D, and inverting the signs of elements B and C to find the inverse

The determinant is calculated as (A*D) - (B*C)

The inverse of a matrix is found by multiplying 1 over the determinant with the matrix elements

Example given: finding the inverse of a 2x2 matrix with elements 2, 1, 5, and 3

Calculating the determinant for the example matrix results in 1

The inverse of the example matrix is found by rearranging and inverting the signs of its elements

Verification of the inverse matrix by multiplying it with the original matrix to obtain the identity matrix

Demonstration of the matrix multiplication to confirm the inverse

Result of the multiplication showing the identity matrix, confirming the inverse is correct

预告下一个视频将展示更通用的矩阵求逆方法

即将展示如何将2x2矩阵求逆的通用方法扩展到3x3矩阵

This method is efficient for 2x2 matrices and can be done quickly

Transcripts

Browse More Related Video

Inverse of a 2x2 Matrix

Inverse Matrices and Their Properties

Inverse of a 3x3 Matrix | Co-factor Method

How to find the Inverse of a Matrix

Inverse of 3x3 matrix

How to Invert Matrices - Exercises (Step by Step)

PreCalculus - Matrices & Matrix Applications (21 of 33) Using the Determinant to Find the Inverse

Takeaways

Q & A

What is the main topic of the video?

How many methods are mentioned for finding the inverse of a matrix?

What is the determinant in the context of a 2x2 matrix?

How is the inverse of a 2x2 matrix calculated?

What happens to elements B and C when finding the inverse of a matrix?

How do you verify the correctness of the calculated inverse?

What is the identity matrix?

What are the elements of the example matrix provided in the video?

What is the determinant of the example matrix?

What is the inverse of the example matrix?

What will be covered in the next video?