Identity matrix | Matrices | Precalculus | Khan Academy
TLDRThe video script explores the concept of the identity matrix in the context of matrix multiplication. It explains that just as 1 times a number equals the number itself, the identity matrix has a similar property for matrix multiplication. The script guides the viewer through constructing a 3x3 identity matrix with ones on the diagonal and zeros elsewhere, ensuring that when multiplied by any matrix, the original matrix is returned. It also hints at the importance of the order of multiplication, prompting viewers to consider what happens when a matrix is multiplied by the identity matrix rather than the other way around.
Takeaways
- 📚 The fundamental concept of multiplication introduced in the script is that 1 times any number equals the number itself, which is an intuitive identity property.
- 🔍 The script explores the concept of an identity matrix in the context of matrix multiplication, questioning if there exists a matrix that maintains the identity property for matrices.
- 🌟 It is established that for a matrix I to have the identity property in matrix multiplication, it must be square and have the same dimensions as the matrix it is being multiplied with.
- 📏 The dimensions of the product matrix are defined by the rows of the first matrix and the columns of the second matrix.
- 🤔 The script encourages viewers to pause and think about constructing an identity matrix, considering the dimensions and properties it must satisfy.
- 🛠️ A 3 by 3 identity matrix is constructed with ones on the diagonal and zeros elsewhere: [1, 0, 0; 0, 1, 0; 0, 0, 1].
- 🧩 The identity matrix has a pattern where ones are placed on the diagonal from the top left to the bottom right, and zeros fill the rest of the matrix.
- 🔄 The property of the identity matrix is such that when it is multiplied by any matrix, the result is the original matrix.
- ⏪ The script also prompts viewers to consider the order of matrix multiplication and question whether A times I would yield the same result as I times A.
- 📖 The concept of identity matrices is a foundational aspect of linear algebra and has broad applications in various fields of mathematics and science.
Q & A
What is the identity property of multiplication mentioned in the script?
-The identity property of multiplication mentioned in the script is that 1 times any number is equal to that number again. This means that when you multiply a number by 1, the result is the original number itself.
What is the primary characteristic of an identity matrix?
-The primary characteristic of an identity matrix is that it has 1s along its main diagonal and 0s elsewhere. When multiplied by any matrix of the same dimensions, the resulting product is the original matrix.
How does the identity matrix affect matrix multiplication?
-The identity matrix has the unique property that when it is multiplied by any matrix, the resulting product is the original matrix. This is similar to the identity property of the number 1 in regular multiplication.
What are the dimensions of the identity matrix?
-The dimensions of the identity matrix must have the same number of rows as the matrix it is being multiplied with and the same number of columns as the matrix it is being multiplied by. For example, if multiplying with a 3x3 matrix, the identity matrix must also be 3x3.
How does one construct an identity matrix for a given size?
-To construct an identity matrix, you place 1s along the main diagonal from the top left to the bottom right and fill the off-diagonal elements with 0s. The pattern is consistent regardless of the size of the matrix.
What happens when the order of matrix multiplication is changed, as in A times I versus I times A?
-The order of matrix multiplication matters. While I times A results in A (the original matrix), A times I may not necessarily result in A unless A is a square matrix with the same dimensions as the identity matrix.
What is the 3 by 3 identity matrix as described in the script?
-The 3 by 3 identity matrix described in the script is: 1, 0, 0; 0, 1, 0; 0, 0, 1. This matrix has 1s along its main diagonal and 0s in all other positions.
Why is the identity matrix important in linear algebra?
-The identity matrix is important in linear algebra because it serves as the multiplicative identity for matrices. It is a fundamental component in matrix operations, ensuring that when it is multiplied by any matrix, the original matrix is preserved.
How does the script illustrate the construction of an identity matrix?
-The script illustrates the construction of an identity matrix by walking through the process of creating a 3 by 3 matrix. It explains that the identity matrix must have 1s along the diagonal and 0s elsewhere to ensure that when it is multiplied by any matrix, the resulting product is the original matrix.
What is the 2 by 2 identity matrix according to the script?
-The 2 by 2 identity matrix, as mentioned in the script, is: 1, 0; 0, 1. It has 1s along the main diagonal and 0s in the off-diagonal positions.
How does the script encourage further exploration of the identity matrix?
-The script encourages further exploration by posing a question about the result of A times I, after having shown that I times A results in A. This prompts the viewer to consider the effects of changing the order of matrix multiplication and to explore the properties of identity matrices on their own.
What is the significance of the identity matrix in solving systems of linear equations?
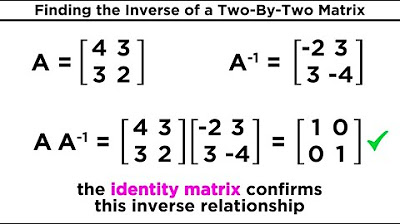
-The identity matrix is significant in solving systems of linear equations because it can be used to find the inverse of a matrix (when it exists), which is essential for solving certain types of linear equations. The identity matrix, when multiplied by the inverse of another matrix, results in the original matrix, which is a key step in the process of solving these equations.
Outlines
🧠 Introduction to the Concept of Identity Matrix
This paragraph introduces the concept of the identity matrix in the context of matrix multiplication. It begins by drawing an analogy with basic multiplication, where one times a number equals the number itself, and explores whether a similar property exists for matrices. The voiceover poses a question about the existence of a matrix, denoted as I, which when multiplied by any other matrix A, results in A. The discussion then focuses on the dimensions required for such an identity matrix, using a 3x3 matrix A as an example. The key points include the necessity for the identity matrix to have the same number of columns as the rows of matrix A and the resulting product to be a 3x3 matrix, aligning with the standard conventions of matrix multiplication.
🔍 Constructing the 3x3 Identity Matrix
This paragraph delves into the process of constructing a 3x3 identity matrix. It explains how the elements of the identity matrix are determined by ensuring that when it is multiplied by a given matrix A, the original matrix A is obtained. The paragraph outlines the step-by-step construction of the identity matrix, starting with the first row and moving on to the subsequent rows, and how the dot product of the corresponding elements must add up to the original matrix A's entries. The summary highlights the pattern of the identity matrix, with 1s along the diagonal and 0s elsewhere, and emphasizes the property that when any matrix is multiplied by the identity matrix, the original matrix is returned. It also encourages the viewer to explore whether the order of matrix multiplication affects the outcome, questioning if A times I is equivalent to I times A.
Mindmap
Keywords
💡multiplication
💡scalar multiplication
💡identity property
💡matrices
💡matrix multiplication
💡identity matrix
💡dimensions
💡dot product
💡diagonal
💡rows and columns
💡order of multiplication
Highlights
The concept of 1 times a number being equal to that number is explored in the context of multiplication and extended to matrix multiplication.
The identity property of multiplication is introduced, where 1 times a number equals the number itself.
The question of whether a matrix exists that has the same identity property for matrix multiplication is posed.
The hypothetical matrix I is introduced, with the property that I times any matrix A results in matrix A.
A 3 by 3 matrix A is used as an example to illustrate the concept of constructing an identity matrix.
The importance of the dimensions of the matrices for multiplication to be defined is discussed.
The rule that the number of columns in the first matrix must equal the number of rows in the second matrix for matrix multiplication is highlighted.
The construction of a 3 by 3 identity matrix is detailed, with the pattern of 1s on the diagonal and 0s elsewhere.
The identity matrix is shown to have the property that when multiplied by any matrix, the original matrix is returned.
The pattern of identity matrices is generalized for different sizes, such as 2 by 2 and 4 by 4 matrices.
The practical application of identity matrices in matrix multiplication is emphasized, showing their utility in the field of mathematics.
The video encourages the audience to pause and think about constructing the identity matrix themselves, promoting active learning.
The process of matrix multiplication is explained through the example of the identity matrix, providing a clear and understandable illustration.
The concept of dot product is implicitly introduced when discussing how to achieve the original matrix elements after multiplication with the identity matrix.
The video poses a follow-up question about the order of matrix multiplication, suggesting A times I versus I times A, prompting further exploration.
The transcript provides a step-by-step guide on how to construct an identity matrix, which is beneficial for educational purposes.
The transcript explains the concept of identity matrices in a way that is accessible to those who may be new to the topic, making complex mathematical ideas more understandable.
Transcripts
Browse More Related Video

PreCalculus - Matrices & Matrix Applications (19 of 33) What is an Identity Matrix?

Dimensions of identity matrix | Matrices | Precalculus | Khan Academy

Zero matrix | Matrices | Precalculus | Khan Academy

Idea behind inverting a 2x2 matrix | Matrices | Precalculus | Khan Academy

Defined and undefined matrix operations | Matrices | Precalculus | Khan Academy
Inverse Matrices and Their Properties
5.0 / 5 (0 votes)
Thanks for rating: