Calculus 1 Lecture 2.6: Discussion of the Chain Rule for Derivatives of Functions
TLDRThe video script discusses the application of the chain rule in calculus, emphasizing its importance in handling derivatives of composite functions. The transcript is a detailed walk-through of how to apply the chain rule, including the general power rule and its use in simplifying complex derivatives. The speaker provides examples and explanations, highlighting common mistakes to avoid and offering tips for successful problem-solving. The session aims to deepen the understanding of calculus concepts and build confidence in applying these rules to a variety of mathematical problems.
Takeaways
- 📚 The chain rule is essential in calculus for finding derivatives of composite functions, allowing for the simplification of complex derivative calculations.
- 🔗 Understanding the concept of compositions in functions is key to applying the chain rule effectively, by identifying the 'outside' and 'inside' functions.
- 📈 The general power rule is an extension of the chain rule, applicable when a function is raised to a power, simplifying the process of differentiating such expressions.
- 🌟 The chain rule states that the derivative of a composite function is the derivative of the outer function multiplied by the derivative of the inner function.
- 🔄 When applying the chain rule, it's important to first take the derivative of the outermost function, then multiply by the derivative of the inner function(s).
- 📊 The process of differentiating complex functions often involves multiple steps, including product rule, quotient rule, and chain rule, which must be applied in the correct order.
- 🤔 Identifying whether a problem requires the chain rule, general power rule, or another method is crucial for efficient problem-solving.
- 🧩 Breaking down complex derivatives into smaller, manageable parts allows for easier application of the appropriate calculus rules.
- 👉 The chain rule is not only about multiplication; it also applies to other operations, including addition and subtraction within composite functions.
- 📝 When expressing the final result of a derivative calculation, it's important to organize and simplify the expression for clarity and correctness.
- 🎓 Mastering the chain rule and its applications can significantly enhance one's ability to tackle a wide range of calculus problems.
Q & A
What is the main topic discussed in the transcript?
-The main topic discussed in the transcript is the chain rule in calculus, its applications, and how it relates to other derivative rules such as the product and general power rules.
What is the chain rule in calculus?
-The chain rule in calculus is a method for finding the derivative of a composite function. It states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function.
How does the chain rule help in simplifying calculus problems?
-The chain rule helps in simplifying calculus problems by providing a systematic way to handle complex derivatives involving composite functions. It allows breaking down the problem into smaller, more manageable parts and applying the appropriate rules to each part.
What is the general power rule mentioned in the transcript?
-The general power rule mentioned in the transcript is a specific type of chain rule that applies when a function is raised to a power. It involves bringing down the exponent, subtracting one from it, and multiplying by the derivative of the base function.
Can you provide an example of the chain rule in action from the transcript?
-An example of the chain rule in action from the transcript is when the instructor takes the derivative of (3x^2 - 4)^100. The derivative is found by applying the chain rule, which results in 100 * (3x^2 - 4)^99 * 6x.
How does the transcript emphasize the importance of order when applying calculus rules?
-The transcript emphasizes the importance of order when applying calculus rules by stating that the rule which encompasses the whole problem should be applied first, and then the rules within that. This order helps in systematically breaking down and solving complex calculus problems.
What is the significance of the 'Dy du' and 'Du DX' terms in the chain rule explanation?
-In the chain rule explanation, 'Dy du' represents the derivative of the outer function with respect to an intermediate variable 'u', and 'Du DX' represents the derivative of the inner function 'u' with respect to 'x'. These terms are crucial for applying the chain rule to find the overall derivative 'dy/dx'.
How does the transcript address common mistakes made when applying the chain rule?
-The transcript addresses common mistakes by highlighting the importance of not forgetting to multiply by the derivative of the inner function and by emphasizing the need to correctly identify and apply the chain rule in various scenarios, such as when dealing with composite functions and general power rules.
What is the role of the product rule in the transcript's explanation?
-The product rule plays a supporting role in the transcript's explanation, as it is used in conjunction with the chain rule to solve more complex derivative problems. The product rule is applied when a function is a product of two or more other functions.
What is the significance of the 'general power rule' in relation to the chain rule?
-The 'general power rule' is a specific application of the chain rule that deals with functions raised to a power. It is significant because it extends the basic chain rule concept to handle exponents, thereby broadening the scope of problems that can be solved using the chain rule.
Outlines
📚 Introduction to the Chain Rule
The paragraph introduces the concept of the chain rule in calculus, emphasizing its importance in dealing with derivatives of composite functions. It explains how the chain rule simplifies the process of finding derivatives by using compositions and highlights its application in solving related rates problems. The speaker also previews the upcoming topics, including implicit differentiation and the general power rule.
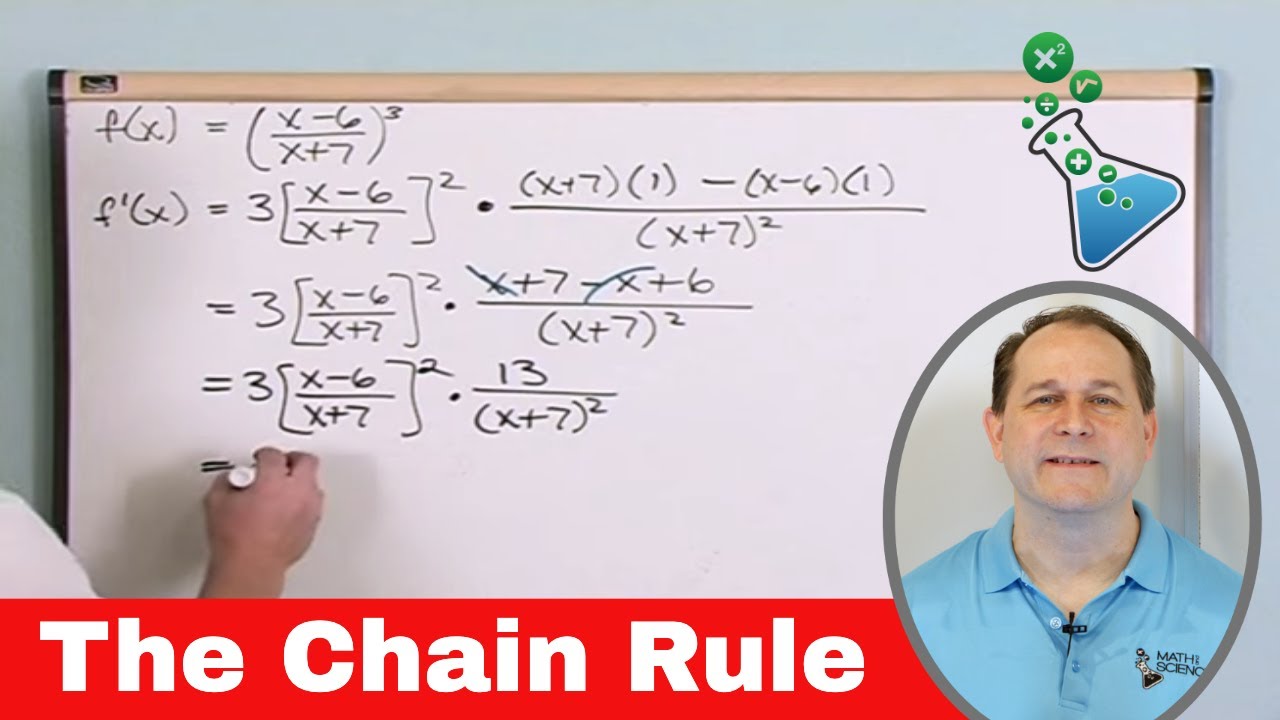
🔍 Applying the Chain Rule: Example Analysis
This paragraph delves into a specific example to illustrate the application of the chain rule. It walks through the process of identifying the inner and outer functions of a composite function and demonstrates how to calculate the derivative using the chain rule. The explanation includes a step-by-step breakdown of the calculations and emphasizes the importance of understanding the underlying process.
📈 General Power Rule: A Chain Rule Extension
The paragraph introduces the general power rule as an extension of the chain rule, applicable when dealing with functions raised to a power. It explains how the general power rule simplifies the process of finding derivatives of power functions and provides a formula for applying the rule. The speaker also emphasizes the importance of understanding the relationship between the chain rule and the general power rule.
🤔 Common Mistakes and Troubleshooting
This paragraph discusses common mistakes made when applying the general power rule and provides guidance on how to avoid them. It emphasizes the importance of correctly identifying the inner function and remembering to multiply by its derivative. The speaker also provides a proof to solidify understanding and offers reassurance to learners that the rules are not as difficult as they may seem at first glance.
🌟 Further Applications of the Chain Rule
The paragraph discusses further applications of the chain rule, particularly in the context of trigonometric functions. It explains how the chain rule can be used to find derivatives of trigonometric expressions and emphasizes the importance of correctly identifying the inner function. The speaker also hints at upcoming topics, such as implicit differentiation and related rates, which will be explored in more depth later.
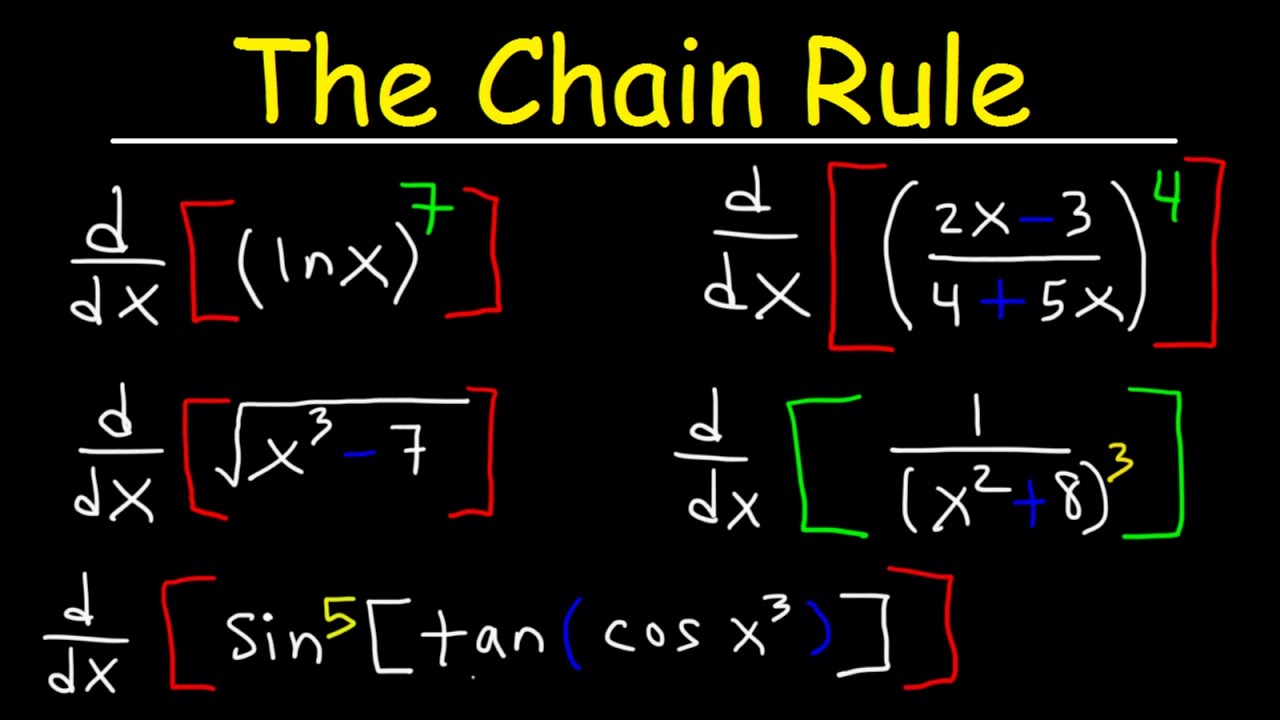
📝 Practice Problems and Troubleshooting
This paragraph presents practice problems to help learners apply the chain rule and general power rule. It emphasizes the importance of showing work and following the correct order of operations when solving these problems. The speaker also provides guidance on how to approach complex problems by breaking them down into smaller, manageable parts.
Mindmap
Keywords
💡Chain Rule
💡General Power Rule
💡Derivative
💡Trigonometric Functions
💡Composition of Functions
💡Rate of Change
💡Simplification
💡Algebraic Manipulation
💡Instantaneous Slope
💡Exponents
Highlights
The introduction of the chain rule in calculus, which allows for the differentiation of composite functions.
The explanation of how the chain rule simplifies the process of differentiation by eliminating the need for distributing terms in complex functions.
The concept of expressing a complex function as a composition to apply the chain rule, by identifying the inner and outer functions.
The method of covering up the inner function to find the derivative of the outer function with respect to the intermediate variable (u).
The step-by-step process of finding the derivative of the inner function (Du/Dx) and multiplying it by the derivative of the outer function with respect to u (Dy/Du).
The general power rule as an extension of the chain rule, which simplifies the differentiation of functions raised to a power.
The application of the chain rule to real-world problems, such as finding the derivative of (3x^2 - 4)^(100), and the explanation of how to substitute back to the original variable.
The discussion on the importance of not distributing terms when a function is raised to a power, and the correct application of the chain rule in such cases.
The emphasis on the practicality of the chain rule and its usefulness in solving more complex calculus problems.
The explanation of how to handle the derivative of trigonometric functions, like cosine, within the chain rule framework.
The clear distinction between the chain rule and the general power rule, and when to apply each in problem-solving.
The demonstration of how to deal with negative exponents and roots in the context of the general power rule.
The guidance on how to organize and simplify the results of applying the chain rule and general power rule for complex expressions.
The reminder to always consider the order of operations and the correct application of calculus rules, especially when dealing with products and quotients.
The final emphasis on the importance of showing all steps when applying the chain rule and related calculus techniques for accurate problem-solving and grading.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: