The Comparison Theorem for Improper Integrals | Step by Step Explanation | Math with Professor V
TLDRIn this informative video, Professor V explains the comparison theorem for improper integrals, focusing on its statement and application through various examples. The theorem states that if a larger continuous function's integral from a point to infinity converges, then the integral of a smaller function also converges. Conversely, if the integral of the smaller function diverges, so does the larger function's integral. The video emphasizes understanding the theorem's essence and using it to draw conclusions about the convergence of improper integrals, highlighting the importance of recognizing the direction of inequalities and the utility of P-series in applying the theorem.
Takeaways
- 📚 The comparison theorem for improper integrals states that if a continuous function f(x) ≥ g(x) ≥ 0 for all x ≥ a, and the improper integral of f(x) converges, then the improper integral of g(x) also converges.
- 🔄 Understanding the essence of the comparison theorem is more important than memorizing the specific functions f and g, as the theorem's applicability depends on the relationship between the functions and their inequalities.
- 📈 The comparison theorem can be applied to improper integrals with one limit being infinity or to integrals where the function has a discontinuity, as long as the functions involved meet the theorem's criteria.
- 🤔 When applying the theorem, one must have an initial suspicion of whether the integral converges or diverges, as this guides the selection of a comparable function and the establishment of the necessary inequality.
- 📊 The p-series integral converges if the exponent p > 1 and diverges if p ≤ 1. This is a key basis for applying the comparison theorem to determine the convergence of other integrals.
- 📌 The comparison theorem is not limited to integrals with limits from 1 to infinity; it can also be used for integrals with other starting points, provided the function and its limits meet the theorem's requirements.
- 🧠 Mastering the comparison theorem requires practice, patience, and the development of mathematical intuition. It is a nuanced concept that may involve trial and error.
- 📈 The video provides several examples to illustrate the application of the comparison theorem, emphasizing the importance of establishing the correct inequality to draw valid conclusions.
- 🔍 When an improper integral is suspected to converge, one should find a function with a known convergent integral that the given function is less than or equal to. Conversely, for suspected divergence, the given function should be greater than or equal to a function with a known divergent integral.
- 🚫 If the comparison theorem cannot be applied directly, other methods such as the limit comparison test for improper integrals may be considered, although it is less commonly taught and may not be covered in standard textbooks.
Q & A
What is the comparison theorem for improper integrals?
-The comparison theorem for improper integrals states that if two continuous functions f and g satisfy f(x) >= g(x) for all x greater than or equal to a, and if the integral from a to infinity of f(x) dx is convergent, then the integral from a to infinity of g(x) dx is also convergent. Conversely, if the integral of g(x) is divergent, then the integral of f(x) is also divergent.
Why is it important to understand the comparison theorem?
-Understanding the comparison theorem is crucial because it provides a method to determine the convergence or divergence of an improper integral without having to evaluate the integral itself. This is particularly useful when dealing with integrals that are difficult or impossible to evaluate directly.
How does the comparison theorem relate to the concept of area under a curve?
-The comparison theorem relates to the concept of area under a curve by considering the improper integral as the accumulation of area under the curve over an infinite interval. If the area under the larger function is convergent (finite), then the area under the smaller function must also be finite and convergent. Conversely, if the area under the smaller function diverges (goes to infinity), then the area under the larger function must also diverge.
What is the significance of the direction of inequality in the comparison theorem?
-The direction of inequality is significant because it determines whether the comparison theorem can be applied to conclude convergence or divergence. The theorem only allows conclusions when one function is strictly greater than or less than the other, as it is necessary to establish which integral converges or diverges based on the behavior of the functions.
How can the comparison theorem be applied to improper integrals with a lower limit of zero?
-The comparison theorem can be applied to improper integrals with a lower limit of zero by comparing the function with another function whose integral is known to converge or diverge. The comparison is made based on the behavior of the functions near the lower limit, and the theorem is used to draw conclusions about the convergence of the original improper integral.
What is the role of p-series in applying the comparison theorem?
-p-series play a crucial role in applying the comparison theorem because they provide a known convergence or divergence behavior for integrals of the form 1/x^p from 1 to infinity. By comparing the given function with a p-series, one can determine the convergence or divergence of the improper integral based on the value of p.
How does the comparison theorem help in evaluating integrals that are difficult to integrate directly?
-The comparison theorem helps in evaluating difficult integrals by allowing us to compare the given function with another function whose integral is easier to evaluate or is already known. This comparison bypasses the need for direct integration and leverages the properties of convergence and divergence to determine the behavior of the original integral.
What is the limit comparison test for improper integrals?
-The limit comparison test for improper integrals is an alternative method used to determine the convergence or divergence of an improper integral. It involves comparing the limit of the ratio of the two functions as one of the variables approaches a particular value (such as infinity) with the limit of the ratio for a known convergent or divergent integral.
Why is it important to practice and discuss the comparison theorem with others?
-Practicing and discussing the comparison theorem with others helps solidify understanding and develop intuition for the concept. It allows for the exploration of different scenarios and functions, enhancing problem-solving skills and the ability to apply the theorem in various contexts.
How does the comparison theorem apply to integrals with discontinuous functions?
-The comparison theorem can also be applied to improper integrals where one of the limits is a discontinuity in the function. The theorem is used in a similar manner, comparing the behavior of the functions to determine the convergence or divergence of the integral, taking into account the nature of the discontinuity.
What is the significance of the example with the arctan function in the script?
-The example with the arctan function demonstrates how to handle integrals where the function's growth rate is not immediately clear. It shows the importance of recognizing bounded functions and understanding the behavior of exponential functions in comparison to polynomial functions, which is crucial for determining the convergence of the improper integral.
Outlines
📚 Introduction to the Comparison Theorem for Improper Integrals
The video begins with an introduction to the comparison theorem for improper integrals. Professor V explains that the theorem helps determine the convergence of improper integrals by comparing two continuous functions, f(x) and g(x), where f(x) is greater than or equal to g(x). The theorem states that if the integral of f(x) from a to infinity converges, then the integral of g(x) also converges, and if the integral of g(x) diverges, then the integral of f(x) also diverges. The emphasis is on understanding the essence of the theorem and the inequality it represents, rather than memorizing the specific functions f and g.
📈 Graphing and Understanding the Comparison Theorem
In this paragraph, Professor V uses a graph to illustrate the comparison theorem. The focus is on the conditions under which the theorem applies: both functions must be non-negative. The explanation continues with the concept of area under the curve, which represents the value of the integral. The idea is that if the area under the larger function (f(x)) converges (doesn't blow up), then the area under the smaller function (g(x)) must also converge. Conversely, if the area under g(x) diverges, then the area under f(x) must also diverge. The importance of the direction of inequality is highlighted, as it affects the conclusion that can be drawn from the theorem.
🤔 Applying the Comparison Theorem to Specific Cases
Professor V discusses how to apply the comparison theorem to specific cases, emphasizing that there are four possible scenarios, only two of which allow for a conclusion. The other two scenarios provide no information about the convergence or divergence of the integrals. The paragraph also introduces the concept of P-series and how it can be used to determine the convergence of improper integrals. The key point is that if the integral of a larger function converges, then the integral of a smaller function must also converge, and vice versa for divergence.
🧠 Mastering the Comparison Theorem Through Practice
The video segment encourages viewers to practice and be patient when learning the comparison theorem. It acknowledges that the theorem is difficult to master and that it requires practice and discussion to solidify understanding. The importance of talking about the concepts with others is highlighted as a way to reinforce learning. The video also mentions that the comparison theorem can be applied to improper integrals with a discontinuity in the function, not just those with an infinite limit.
📝 Working with Improper Integrals Using the Comparison Theorem
This paragraph delves into the process of using the comparison theorem to evaluate specific improper integrals. The video provides examples and walks through the steps of comparing functions and setting up inequalities to determine convergence or divergence. The role of P-series in simplifying the process is emphasized, as it allows for the comparison without the need to evaluate the actual integral. The video also addresses the importance of having a preliminary idea of whether the integral will converge or diverge before applying the theorem.
🌟 Summary and Encouragement for Further Learning
In the concluding paragraph, Professor V summarizes the key points of the video and encourages viewers to continue practicing the comparison theorem. The video acknowledges the challenges of the concept and offers support for students. It also mentions the possibility of creating additional content for further learning and encourages viewers to follow the professor on social media platforms for more mathematical content and assistance.
Mindmap
Keywords
💡Comparison Theorem
💡Improper Integrals
💡Convergence
💡Divergence
💡p-Series
💡Continuous Functions
💡Inequality
💡Graphing
💡Limits
💡Integration Techniques
Highlights
Introduction to the comparison theorem for improper integrals.
Explanation of the theorem's statement involving continuous functions f and g with f(x) ≥ g(x) ≥ Z for x ≥ a.
Illustration of the theorem with a graph to help visualize the concept.
Clarification that memorizing which function is f and which is g is not important, but understanding the essence of the theorem is.
Discussion on the meaning of convergence in the context of improper integrals and how it relates to the accumulation of area under a curve.
Explanation of the four possible cases for applying the comparison theorem and when it can provide information.
Introduction of p-series as a helpful tool in applying the comparison theorem for improper integrals.
Example of using the comparison theorem to determine the convergence of an improper integral involving 1/x^2 from 1 to Infinity.
Demonstration of how the comparison theorem can be used for improper integrals with a discontinuity in the function.
Explanation of the process of determining whether an improper integral converges or diverges and the importance of initial suspicion.
Example of using the comparison theorem to show that an improper integral of x/√(x^2 - 1) from 1 to Infinity diverges.
Illustration of how to handle an improper integral at the lower limit where the function is undefined, using the example of 0 to Infinity of (sin x)/√(x) dx.
Another example demonstrating the process of comparing functions to determine the convergence of an improper integral, this time with x + 1/√(x^4 - x) from 1 to Infinity.
Mention of the Limit Comparison Test as an alternative method for improper integrals, especially useful for infinite series.
Final example involving the improper integral from 0 to Infinity of arctan(x)/(x^2 + e^x) dx, showing the application of comparison theorem with non-polynomial functions.
Summary of the video, emphasizing the importance of practice and patience in mastering the comparison theorem for improper integrals.
Transcripts
Browse More Related Video

Worked example: Integral test | Series | AP Calculus BC | Khan Academy
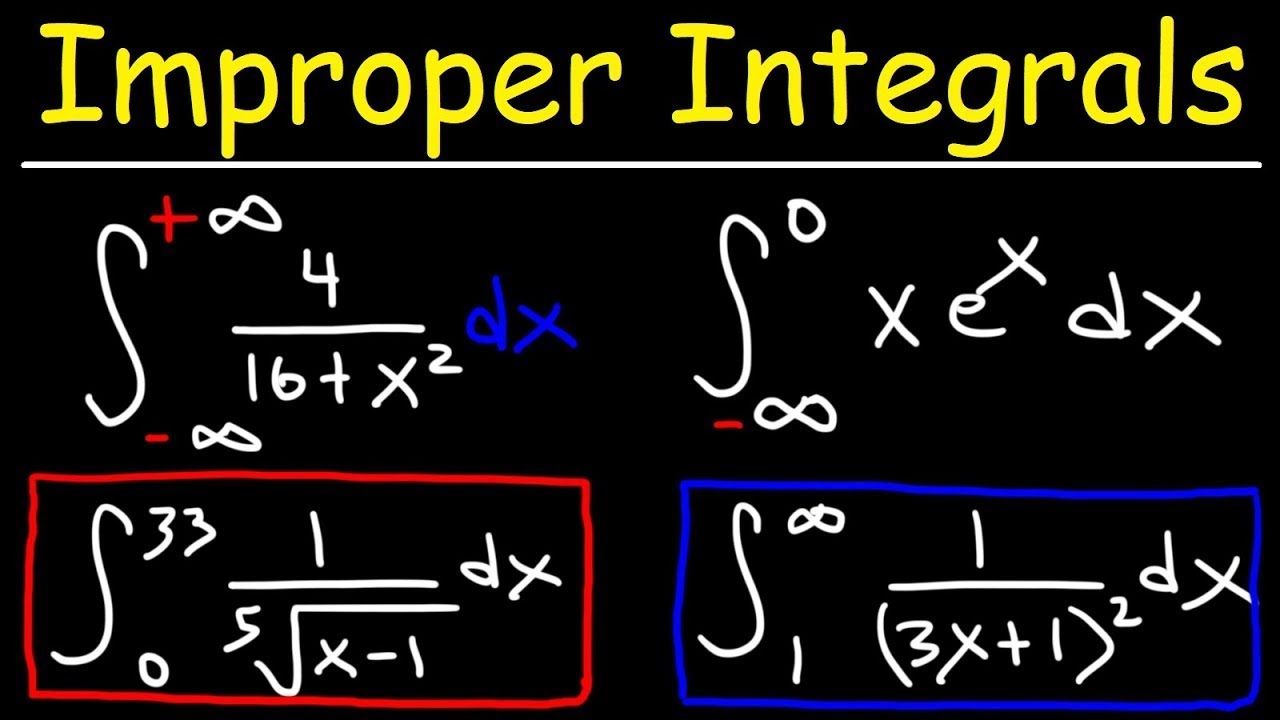
Improper Integrals - Convergence and Divergence - Calculus 2
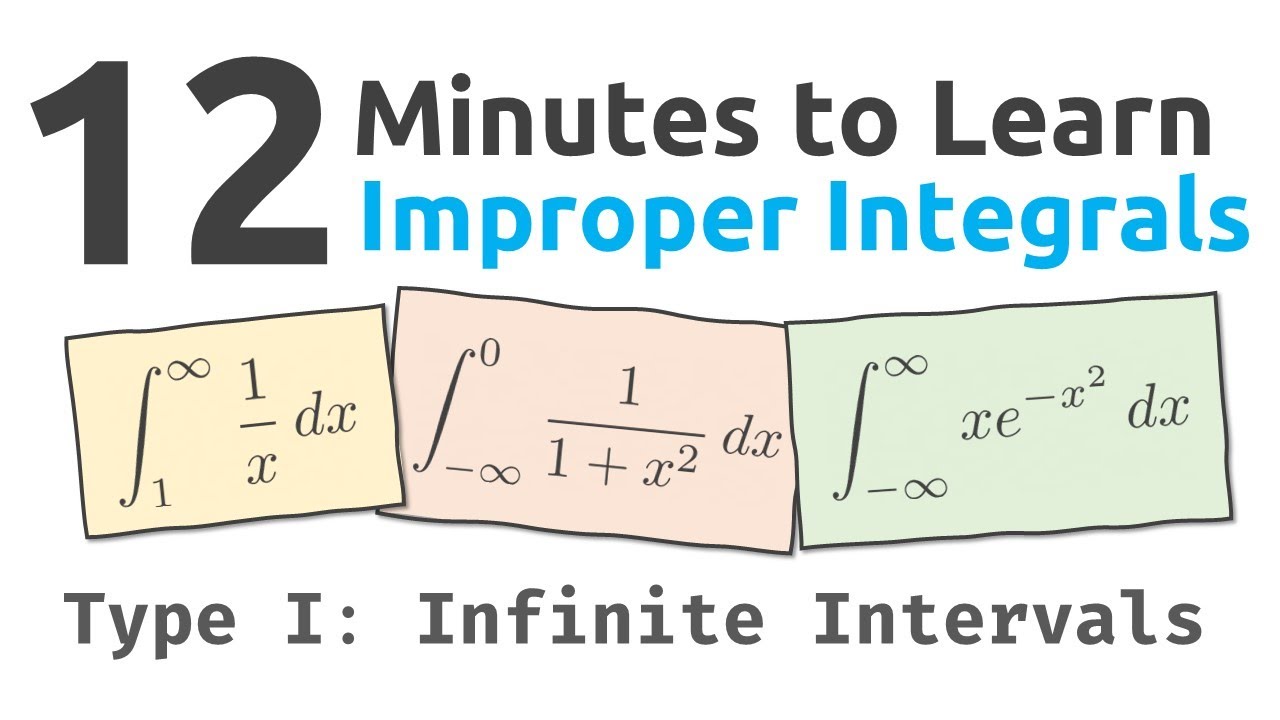
Improper Integrals of Type I (Infinite Intervals) in 12 Minutes

Proof of p-series convergence criteria | Series | AP Calculus BC | Khan Academy

Calculus 2 Lecture 9.3: Using the Integral Test for Convergence/Divergence of Series, P-Series

Improper Integrals - Discontinuity in the Interval
5.0 / 5 (0 votes)
Thanks for rating: