Lec-13 I Schrodinger wave question I Applied Chemistry I Chemical Engineering

Chemical Engineering Department_LJIET
29 Jul 202113:43
EducationalLearning
32 Likes 10 Comments

TLDRIn this video lecture series on applied chemistry, the focus is on quantum theory for chemical systems, introducing the wave mechanical concept of atoms and Heisenberg's uncertainty principle. The lecture delves into Schrödinger's equation, explaining its derivation and significance in determining the position of electrons around an atomic nucleus. It also discusses the relationship between an electron's wave function, its probability distribution, and the potential energy. The session concludes with an exploration of Schrödinger's wave equation in one dimension and its extension to three dimensions, highlighting its importance in quantum chemistry.

Takeaways
  • 📘 The lecture series is on Applied Chemistry with the subject code 3130506.
  • 🌟 The current chapter focuses on Quantum Theory for Chemical Systems, also known as Quantum Chemistry.
  • 🌊 The wave mechanical concept of an atom and Heisenberg's uncertainty principle are foundational topics covered.
  • 📈 The uncertainty principle relates the position (x) and momentum (p) of particles, emphasizing its applicability to atomic systems.
  • 💫 Atoms exhibit both wave-like and particle-like properties, which are central to the study of quantum chemistry.
  • 🥼 Schrödinger's equation is introduced as a method to determine the position of electrons around an atomic nucleus.
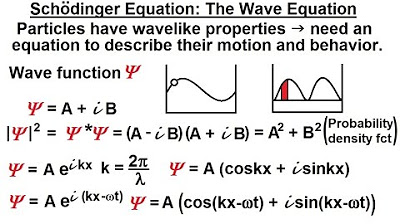
  • 📐 The mathematical representation of the wave function (psi) is key in Schrödinger's equation.
  • 🔄 Differentiation of the wave function is used to derive relationships between the wave properties and the position of electrons.
  • 🔧 The kinetic energy of a particle is related to its mass, velocity, and wavelength through de Broglie's equation.
  • 🌐 Schrödinger's equation can be applied in one dimension and expanded to three dimensions for a comprehensive understanding of particle behavior.
  • 🚀 The total energy of a particle is the sum of its kinetic and potential energy, which is integral in solving the Schrödinger's equation.
Q & A

  • Who is the speaker in the transcript?

    -The speaker in the transcript is Sugruti Yoshi from Algae Institute of Engineering and Technology.

  • What is the subject code for Applied Chemistry?

    -The subject code for Applied Chemistry is 3130506.

  • What chapter of the course are they currently studying?

    -They are currently studying Chapter 4, which focuses on Quantum Theory for Chemical Systems.

  • What is the main concept introduced in the chapter?

    -The main concept introduced in the chapter is the wave mechanical concept of an atom, including Heisenberg's uncertainty principle.

  • What does the wave mechanical theory of an atom involve?

    -The wave mechanical theory of an atom involves the study of atomic systems, recognizing that atoms can exhibit both wave and particle natures.

  • What is Schrödinger's equation and why is it significant?

    -Schrödinger's equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes with time. It is significant because it allows us to calculate the position and momentum of particles, such as electrons, around the atomic nucleus.

  • What is the relationship between the probability of finding an electron and the wave function?

    -The probability of finding an electron at a particular position in an atom is determined by the wave function, which represents the amplitude of the wave associated with the electron.

  • How is the kinetic energy of a particle calculated?

    -The kinetic energy (KE) of a particle is calculated using the formula KE = 1/2 mv^2, where m is the mass of the particle and v is its velocity.

  • What is the relationship between de Broglie's wavelength (λ) and the momentum (p) of a particle?

    -According to de Broglie, the wavelength (λ) is inversely proportional to the momentum (p) of a particle, with the relationship given by λ = h/p, where h is Planck's constant.

  • What is the Schrödinger equation for one dimension?

    -The Schrödinger equation for one dimension is given by -ħ²/8π²m * (d²ψ/dx²) + Vψ = Eψ, where ħ is reduced Planck's constant, m is the mass of the particle, V is the potential energy, E is the total energy, and ψ is the wave function.

  • How can Schrödinger's equation be represented in three dimensions?

    -In three dimensions, Schrödinger's equation is represented as ∇²ψ + 8π²m/ħ² * (E - V)ψ = 0, where ∇² is the Laplacian operator applied in three dimensions (∂²/∂x² + ∂²/∂y² + ∂²/∂z²), and all other terms are as previously defined.

  • What is the significance of solving Schrödinger's equation for a chemical system?

    -Solving Schrödinger's equation for a chemical system allows us to predict the behavior of electrons in atoms and molecules, which is crucial for understanding chemical reactions, bonding, and the properties of materials.

Outlines
00:00
📚 Introduction to Quantum Chemistry and Wave Mechanics

This paragraph introduces the subject of applied chemistry, focusing on quantum theory for chemical systems. It discusses the wave mechanical concept of an atom, Heisenberg's uncertainty principle, and their applications in studying atomic systems and chemical reactions. The speaker explains that these principles apply to smaller objects and are fundamental in understanding quantum chemistry. The lecture then transitions into discussing Schrödinger's equation, which is used to determine the position of an electron around the atomic nucleus, emphasizing the importance of wave functions and their mathematical representation.

05:03
🧪 Differentiation and Application of Schrödinger's Equation

The speaker delves into the process of differentiating Schrödinger's equation to understand the probability of finding an electron at various points within an atom. The paragraph outlines the steps of differentiating the wave function equation, resulting in a series of equations that build upon each other. The speaker also introduces the concept of kinetic energy and its relation to the wavelength, leading to the derivation of a new equation that connects kinetic energy to the properties of the wave function. This section is a deep dive into the mathematical foundations of quantum chemistry.

10:05
🌐 Schrödinger's Equation in Multi-Dimensional Space

In this paragraph, the speaker extends the discussion to Schrödinger's equation in multiple dimensions, starting with the one-dimensional case and then generalizing to three dimensions. The total energy of a particle, a sum of its kinetic and potential energy, is introduced, and the relationship between these energies is explored through the lens of Schrödinger's equation. The speaker also discusses how the wave equation can be applied to particles moving in any direction, providing a comprehensive understanding of how the position of particles can be determined in a quantum system. The lecture concludes with a brief mention of the next session, leaving the audience eager for more.

Mindmap
Keywords
💡Applied Chemistry
Applied Chemistry refers to the practical application of chemistry principles to solve real-world problems. In the context of the video, it is the subject being taught, with a focus on quantum theory for chemical systems, emphasizing the importance of understanding atomic and molecular interactions in chemical reactions.
💡Quantum Theory
Quantum Theory is a fundamental theory in physics that describes the behavior of matter and energy at the atomic and subatomic scales. It introduces concepts such as wave-particle duality and the uncertainty principle, which are crucial for understanding the quantum chemistry discussed in the video.
💡Wave-Mechanical Concept
The wave-mechanical concept, also known as wave mechanics or quantum mechanics, is a principle in quantum chemistry that describes the behavior of particles, such as electrons in atoms, as wave-like entities. This concept is essential for understanding the properties of chemical systems at the quantum level.
💡Heisenberg's Uncertainty Principle
Heisenberg's Uncertainty Principle states that it is impossible to simultaneously know the exact position and momentum of a particle. This principle is a cornerstone of quantum mechanics and has significant implications for the study of atomic systems, as it limits the precision with which we can describe the behavior of particles.
💡Schrodinger's Equation
Schrodinger's Equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes with time. It is used to calculate the wave function of a system, which provides information about the probabilities of finding a particle in different states.
💡Wave Function
A wave function, represented by the Greek letter psi (ψ), is a mathematical function used in quantum mechanics to describe the quantum state of a system. It contains all the information about a particle's probability distribution and is central to solving Schrodinger's Equation.
💡Probability
In the context of quantum chemistry, probability refers to the likelihood of finding a particle, such as an electron, in a particular location or state. It is calculated using the wave function and is a key concept in understanding the behavior of particles at the quantum level.
💡Kinetic Energy
Kinetic energy is the energy of motion possessed by an object due to its velocity. In the video, it is related to the movement of particles, such as electrons, and is used in the derivation of Schrodinger's Equation to describe the energy states of particles in a quantum system.
💡Potential Energy
Potential energy is the stored energy of a system due to its position or configuration. In quantum chemistry, it is associated with the energy states of particles within a system, such as the energy levels of electrons in an atom.
💡One-Dimensional
One-dimensional refers to a system or model that is considered to have only one spatial dimension, such as a straight line. In the video, the one-dimensional aspect is used to simplify the complex equations of quantum mechanics, allowing for easier analysis and understanding of the fundamental concepts.
💡Three-Dimensional
Three-dimensional refers to a system or model that has three spatial dimensions: length, width, and height. In quantum chemistry, the three-dimensional aspect is important for accurately describing the behavior of particles in real-world systems, as it accounts for all possible directions of movement and interaction.
Highlights

Introduction to Applied Chemistry and its subject code 3130506.

Discussion on the quantum theory for chemical systems and its importance in understanding atomic systems and chemical reactions.

Explanation of the wave mechanical concept of an atom, including the Heisenberg uncertainty principle and its application to objects of different sizes.

Description of the dual nature of atoms, exhibiting both wave and particle characteristics.

Introduction to Schrödinger's equation and its significance in determining the position of an electron around the atomic nucleus.

Derivation of the probability of finding an electron at various points in an atom using wave functions.

Explanation of the mathematical representation of wave functions and their relation to the position and amplitude of waves.

First differentiation of the wave function equation with respect to displacement x, leading to the first derivative equation.

Second differentiation of the wave function equation and the resulting second derivative equation.

Substitution of the original wave function into the second derivative equation to obtain a new equation.

Discussion on the kinetic energy of a particle and its relation to mass and velocity.

Derivation of the relationship between wavelength (lambda), Planck's constant (h), and the velocity of the particle (v).

Rearrangement of the kinetic energy equation to express it in terms of lambda and the square of the velocity.

Introduction to the total energy of a particle as the sum of its kinetic and potential energy.

Presentation of the one-dimensional Schrödinger equation and its implications for finding the position of a particle.

Explanation of how to find the position of a particle in any direction using the Schrödinger equation.

Derivation and representation of the three-dimensional Schrödinger wave equation for x, y, and z directions.

Conclusion and preview of the next session's topics.

Transcripts

Browse More Related Video

Lec-14 I Hybridization I Applied Chemistry I Chemical Engineering

Lec-11 I Introduction to quantum chemistry I Applied Chemistry I Chemical Engineering

what is the radius of the hydrogen atom?

Lecture 5: Operators and the Schrödinger Equation

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (1 of 92) The Wave Equation

19. Quantum Mechanics I: The key experiments and wave-particle duality

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Quantum ChemistryWave MechanicsSchrödinger EquationApplied ChemistryAtomic SystemsEducational ContentLecture SeriesInstitute of EngineeringElectron Behavior