High School Physics - Conservation of Energy

Dan Fullerton
29 Nov 201115:25
EducationalLearning
32 Likes 10 Comments

TLDRIn this informative lecture, Mr. Fullerton discusses the principle of conservation of energy, emphasizing its application in analyzing energy transitions and transformations in various physical systems. He illustrates the concept with examples such as an F-18 Hornet's flight dynamics, a falling object's velocity, a toy cart interacting with a spring, and a car's kinetic energy affected by friction. The lecture highlights the importance of understanding energy conservation in problem-solving and its significance in fields like aviation.

Takeaways
  • πŸ“œ The law of conservation of energy states that energy cannot be created or destroyed, only transformed from one form to another.
  • πŸš€ The total mechanical energy (kinetic + potential + spring) is conserved in the absence of friction, but internal energy is considered when friction is present.
  • ✈️ An F-18 Hornet's total energy can be calculated using its mass, velocity, and altitude, and this energy is conserved even as it transitions from high altitude to high velocity.
  • πŸ”Ÿ Drop an object from a height, and its potential energy at the top equals its kinetic energy at the bottom, assuming no friction.
  • 🏎️ In kinematics, the velocity of a falling object can be found using the formula v = √(2gh), where v is the final velocity, g is the acceleration due to gravity, and h is the height.
  • πŸ”„ Conservation of energy can be applied to various scenarios, such as a toy cart compressing a spring and then converting that potential energy back into kinetic energy.
  • πŸ“ˆ When a car brakes, the work done by friction is equal to the change in the car's kinetic energy.
  • πŸ“Š A graph showing the relationship between the work done by friction and the car's kinetic energy would start high for the car's kinetic energy and decrease as friction does more work.
  • πŸ”Œ The work-energy theorem states that work done on an object results in a change in its kinetic energy when moving along a frictionless surface.
  • πŸ”§ The work done by friction on a block sliding down a ramp is the difference between the potential energy at the top and the kinetic energy at the bottom.
Q & A

  • What is the law of conservation of energy?

    -The law of conservation of energy states that energy cannot be created or destroyed, but can only be changed or transformed from one form to another. In the context of this script, it is used to analyze energy transitions and transformations in a system.

  • How is mass related to energy according to the script?

    -The script explains that mass and energy are closely related, and mass is a highly concentrated form of energy. This relationship is encapsulated in the concept that mass-energy cannot be created or destroyed, although conversions of mass into energy are rare in the problems discussed.

  • What is the significance of mechanical energy in the absence of friction?

    -In the absence of friction, mechanical energy, which is the sum of kinetic energy, gravitational potential energy, and spring potential energy, is conserved. This means that the total amount of mechanical energy in a system remains constant.

  • How does the script illustrate the conservation of energy with the example of an F-18 Hornet?

    -The script uses the F-18 Hornet as an example to show how the conservation of energy principle applies to changes in altitude and velocity. It calculates the total energy of the jet at two different altitudes and demonstrates that as the jet dives and loses altitude, it gains velocity, illustrating the trade-off between potential and kinetic energy.

  • What is the formula for gravitational potential energy?

    -The formula for gravitational potential energy is given by PE = mgh, where m is the mass of the object, g is the acceleration due to gravity, and h is the height above a reference point.

  • How does the script explain the conversion of potential energy into kinetic energy in a freely falling object?

    -The script explains that when an object is dropped from a height, its gravitational potential energy at the top is converted into kinetic energy at the bottom. The velocity of the object just before it hits the ground can be calculated using the formula v = sqrt(2gh), where v is the velocity, g is the acceleration due to gravity, and h is the height.

  • What is the relationship between the initial kinetic energy of the cart and the potential energy of the spring in the toy cart example?

    -In the toy cart example, the initial kinetic energy of the cart is equal to the potential energy of the spring once the cart comes to rest. This is another demonstration of the conservation of energy principle, where the total energy in the system remains constant despite the transformation of energy from one form to another.

  • How does the script calculate the spring constant in the toy cart example?

    -The script calculates the spring constant (K) by setting the initial kinetic energy of the cart equal to the potential energy of the spring after the cart comes to rest. Using the potential energy formula (1/2 KX^2) and solving for K, the script finds that K = (2 * initial kinetic energy) / displacement^2.

  • What is the work-energy theorem mentioned in the script?

    -The work-energy theorem states that the work done on an object is equal to the change in its kinetic energy. In the context of the script, when work is done on an object moving on a frictionless horizontal surface, that work is converted into kinetic energy.

  • How does the script demonstrate the relationship between work done by friction and the change in kinetic energy of a car?

    -The script illustrates that as friction does work on a car that is skidding to a stop, the car's kinetic energy decreases. The work done by friction is equal to the change in the car's kinetic energy, which goes from its maximum value (when the car was initially moving) to zero (when the car stops).

  • What is the significance of the conservation of energy principle in the context of the problems discussed in the script?

    -The conservation of energy principle is crucial for understanding and solving the problems discussed in the script. It allows for the analysis of energy transformations and the calculation of various energies in different scenarios, such as the F-18 Hornet's flight, the falling object, the toy cart and spring system, and the block sliding down a ramp.

Outlines
00:00
🌟 Introduction to Energy Conservation

This paragraph introduces the concept of energy conservation, emphasizing its importance in analyzing energy transitions and transformations in various systems. Mr. Fullerton explains the law of conservation of energy, which states that energy cannot be created or destroyed but can only change forms. He also touches upon the relationship between mass and energy, noting that mass is a concentrated form of energy. The focus is on mechanical energy conservation, which involves kinetic, gravitational potential, and spring potential energy. The paragraph presents a problem involving an F-18 Hornet aircraft to demonstrate how total energy remains constant in the absence of friction, and how energy is conserved when the aircraft changes altitude and velocity.

05:01
πŸ“‰ Energy Conservation in Free Fall

In this paragraph, the discussion shifts to applying energy conservation principles to free fall scenarios. It compares two methods of solving for the velocity of a falling object: kinematics and energy conservation. The energy approach is demonstrated by calculating the velocity of an object falling from a height of 10 meters, using the conservation of mechanical energy and the formula for gravitational potential energy. The paragraph also highlights the consistency between the results obtained from both the kinematic and energy approaches, reinforcing the reliability of energy conservation principles in physics problems.

10:02
🌈 Spring Energy Transformation

This section delves into the application of energy conservation toεΌΉη°§ systems. It presents a scenario where a toy cart with kinetic energy compresses a spring on a frictionless surface, and the initial kinetic energy is converted into the spring's potential energy. The spring constant is calculated using the conservation of energy, and another example is provided where a pop-up toy's compressed spring is analyzed for potential energy storage. The transformation of potential energy into gravitational potential energy and kinetic energy as the toy moves is also discussed, along with a problem involving a folder falling from a desk and the speed it attains upon impact.

15:03
πŸš— Work and Energy in Frictional Forces

The final paragraph discusses the work done by frictional forces and how it relates to the change in an object's kinetic energy. A graphical representation is used to illustrate the relationship between the work done by friction and the car's kinetic energy as it slows down from 30 meters per second to a stop. The concept of work-energy theorem is introduced, which states that work done on an object results in a change in its kinetic energy. The paragraph concludes with a problem involving a block sliding down a ramp, where the work done by friction is calculated based on the difference between the potential energy at the top and the kinetic energy at the bottom of the ramp.

Mindmap
Keywords
πŸ’‘Conservation of Energy
The principle stating that the total amount of energy in an isolated system remains constant, though it may change forms. In the video, this concept is central to analyzing energy transitions and transformations in various scenarios, such as the F-18 Hornet jet trading altitude for velocity, demonstrating how energy is conserved in physical systems.
πŸ’‘Mechanical Energy
The sum of kinetic and potential energies in a system. In the context of the video, mechanical energy is highlighted as being conserved in the absence of friction, with examples like the F-18 Hornet's energy conservation illustrating the transformation between potential and kinetic energy without a loss or gain in total mechanical energy.
πŸ’‘Gravitational Potential Energy
The energy an object possesses due to its position in a gravitational field, typically related to its height above a reference point. In the video, the F-18 Hornet's energy calculations and the falling object example both involve gravitational potential energy, which is converted into kinetic energy as the object falls or descends.
πŸ’‘Kinetic Energy
The energy an object has due to its motion, calculated as half the product of its mass and the square of its velocity. The video discusses kinetic energy in relation to the F-18 Hornet's velocity and the falling object, emphasizing how it changes as a result of energy conservation principles.
πŸ’‘Friction
A force that opposes the relative motion or tendency of such motion of two surfaces in contact. In the video, friction is mentioned as a factor that can convert mechanical energy into internal energy, affecting the conservation of mechanical energy but not the total energy of the system.
πŸ’‘Internal Energy
The total energy contained within a system due to the random motion of its molecules and their interactions. While not the primary focus, the video mentions internal energy in the context of friction, where it is generated at the expense of mechanical energy in a non-ideal scenario.
πŸ’‘Spring Constant
A measure of the stiffness of a spring, defined as the force needed to compress or extend the spring by a unit distance. The video explains how the spring constant can be determined using the conservation of energy principle when a cart compresses a spring and comes to rest.
πŸ’‘Potential Energy
The stored energy an object has due to its position or condition. In the video, potential energy is a recurring theme, with examples including gravitational potential energy and elastic potential energy in springs, both of which can be converted into other forms of energy under the right conditions.
πŸ’‘Elastic Potential Energy
The energy stored in a material when it is stretched, compressed, or deformed elastically. In the video, elastic potential energy is discussed in the context of a spring being compressed by a toy cart, where the initial kinetic energy of the cart is converted into the spring's elastic potential energy.
πŸ’‘Work-Energy Theorem
A principle stating that the work done on an object is equal to the change in its kinetic energy. The video touches on this concept when discussing the work done by friction on a car skidding to a stop, highlighting the relationship between work and changes in an object's energy state.
πŸ’‘Energy Transformation
The process by which energy changes from one form to another. The video emphasizes energy transformation through various examples, such as the conversion of gravitational potential energy to kinetic energy in a falling object or the trade-off between altitude (potential energy) and velocity (kinetic energy) in an F-18 Hornet.
Highlights

The law of conservation of energy states that energy cannot be created or destroyed, only changed.

Mass and energy are closely related, with mass being a highly concentrated form of energy.

Mechanical energy is conserved in the absence of friction, meaning the sum of kinetic, gravitational potential, and spring potential energy remains constant.

An F-18 Hornet with a mass of 20,000 kilograms coasting at an altitude of 10,000 meters and a velocity of 250 m/s has a total energy of about 2.59 x 10^9 joules.

When the F-18 dives to 2,000 meters, its velocity increases due to the conservation of energy, trading altitude for velocity.

The conservation of energy concept is crucial for fighter pilots, who often need to make trade-offs between altitude and velocity.

Dropping an object from a height of 10 meters results in a final velocity of approximately 14 m/s, using both energy and kinematic approaches.

A toy cart with 16 joules of kinetic energy compresses a spring by 1 meter, allowing the calculation of the spring constant as 32 N/m.

In a pop-up toy example, the potential energy stored in a compressed spring is converted to gravitational potential energy, with the toy reaching a height of approximately 0.96 meters.

The speed of a folder striking the floor is determined by its initial gravitational potential energy, resulting in a velocity of the square root of 2gh.

A graph showing the relationship between the work done by friction and the kinetic energy of a car reveals a decrease in kinetic energy as friction does more work.

Work done in accelerating an object on a frictionless surface results in a change in kinetic energy, as gravitational potential energy cannot be affected.

A block sliding down a ramp from a height of three meters and reaching the ground with 50 joules of kinetic energy indicates work done by friction can be calculated through energy conservation.

Conservation of energy is a fundamental concept in physics with wide-ranging applications in various real-world scenarios.

The use of energy conservation in problem-solving provides a powerful tool for understanding and analyzing physical systems.

The importance of understanding energy transformations is emphasized, as it is a key aspect of the conservation of energy principle.

The concept of energy conservation is introduced with practical examples to illustrate its application in solving physics problems.

Transcripts

Browse More Related Video

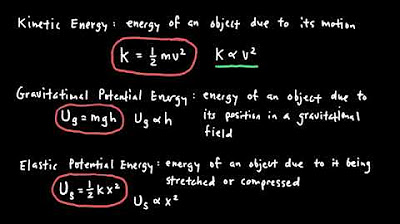
AP Physics 1 Energy Review

Open & Closed Systems in Energy - IB Physics

2022 Live Review 4 | AP Physics 1 | Understanding Work and Energy

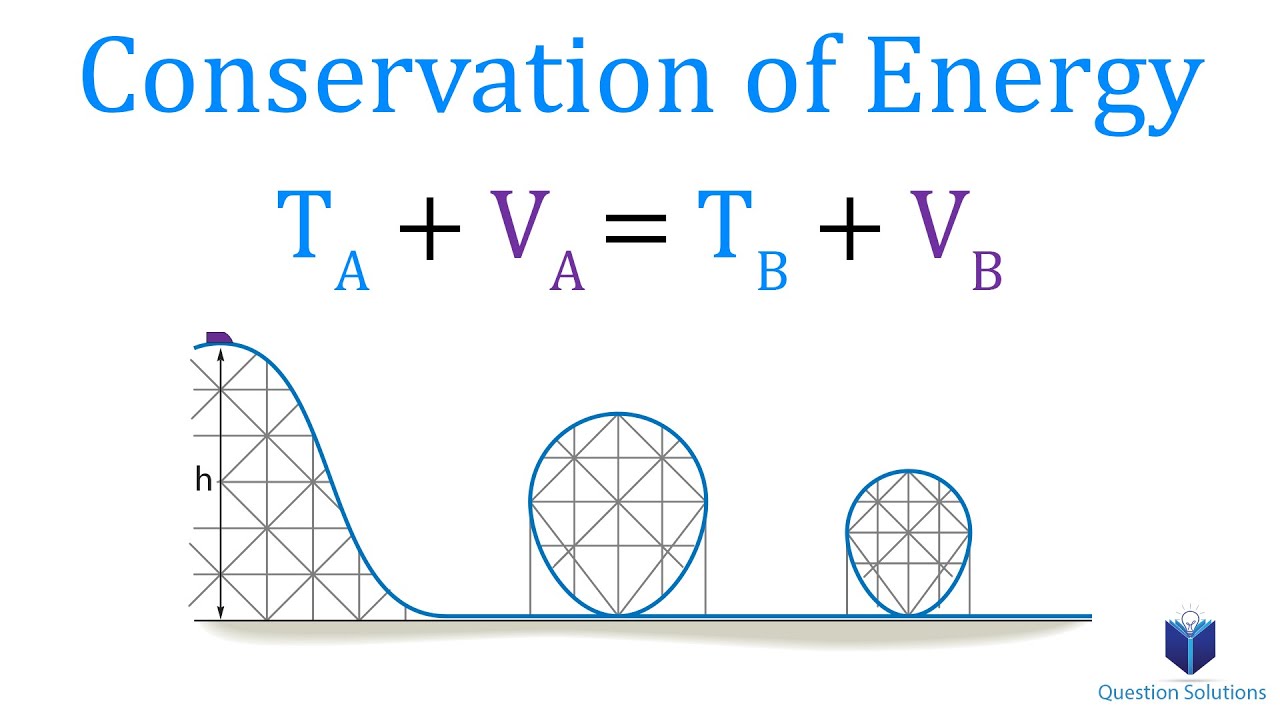
Conservation of Energy (Learn to solve any problem)

2021 Live Review 4 | AP Physics 1 | Understanding Work and Energy

LOL diagrams | Work and energy | Physics | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsPrinciplesConservationOfEnergyMechanicalEnergyFighterPilotsEnergyTransformationsKinematicsSpringMechanicsFrictionWorkPotentialEnergyKineticEnergy