High School Physics - Springs and Hooke's Law

Dan Fullerton
29 Nov 201112:02
EducationalLearning
32 Likes 10 Comments

TLDRIn this informative video, Mr. Fullerton discusses the principles of Hooke's Law and elastic potential energy in springs. He explains the linear relationship between the force exerted by a spring and its displacement from equilibrium, and how to calculate the spring constant. The video also covers how to determine the work done in compressing or stretching a spring, which equates to the stored potential energy. Through examples and graphical analysis, Mr. Fullerton demonstrates how to apply these concepts in practical scenarios, offering insights into the behavior of springs under different forces and displacements.

Takeaways
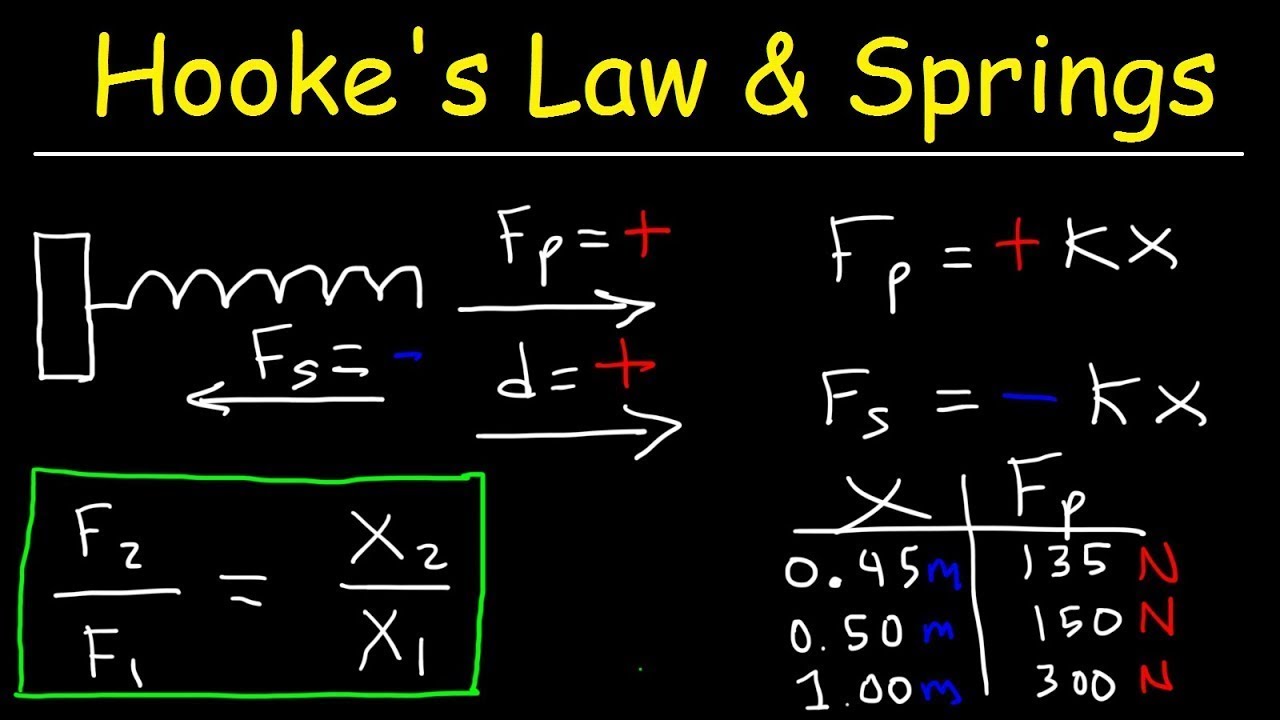
  • πŸ“š Hook's Law describes the linear relationship between the force exerted by a spring and its displacement from the equilibrium position, with the formula FS = -KX, where F is the force, K is the spring constant, and X is the displacement.
  • πŸ” The negative sign in Hook's Law indicates that the force exerted by the spring is a restoring force, aiming to return the spring to its equilibrium position.
  • πŸ”¨ To determine the spring constant (K), one can apply different forces and measure the resulting displacements, then plot a force versus displacement graph and calculate the slope.
  • πŸ“ˆ The slope of the force-displacement graph represents the spring constant (K), with the formula K = Ξ”F/Ξ”X, where Ξ”F is the change in force and Ξ”X is the change in displacement.
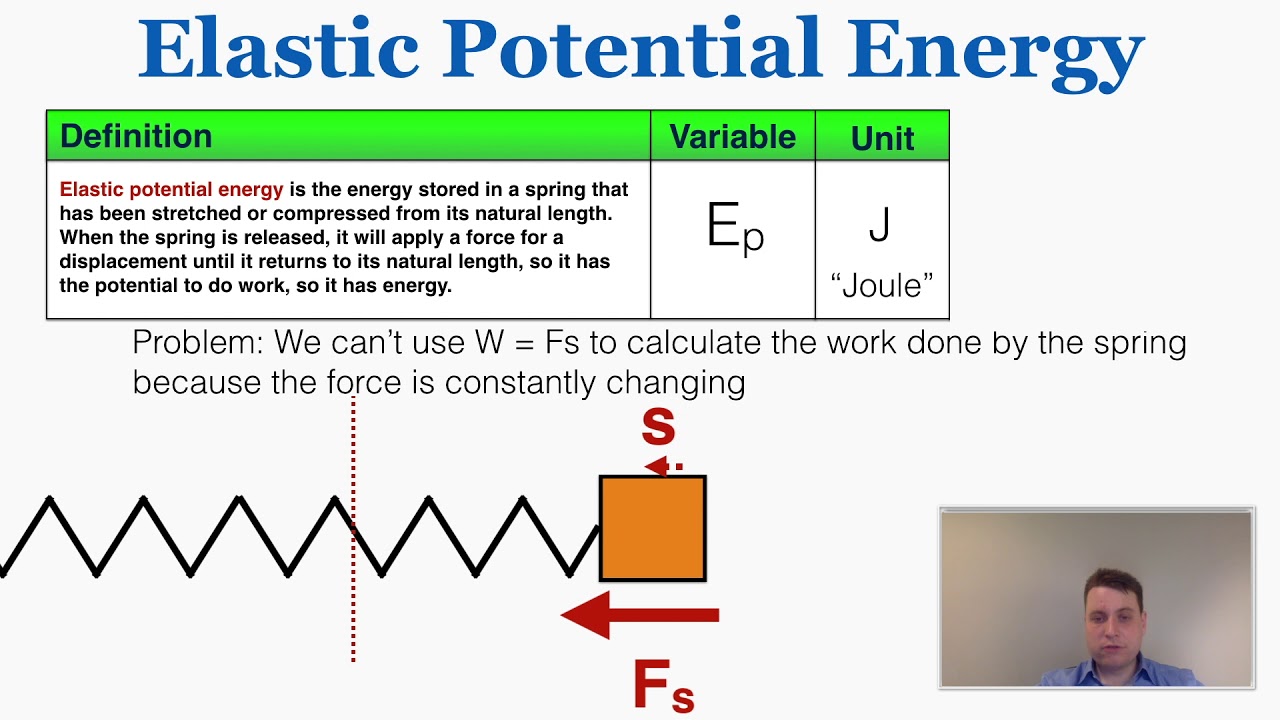
  • 🌟 The work done in compressing or stretching a spring is stored as elastic potential energy in the spring, which can be calculated using the area under the force-displacement graph.
  • πŸ”’ The potential energy (PE) stored in a spring is given by the formula PE = (1/2)KX^2, where K is the spring constant and X is the displacement from the equilibrium position.
  • πŸ“ For a spring with a given spring constant, the stiffer the spring (higher K value), the greater the force exerted for a given displacement.
  • πŸ“ˆ The area of the triangle in the force-displacement graph represents the work done on the spring, calculated as (1/2)base * height, where the base is the displacement and the height is the force.
  • πŸ”§ To find the displacement (X) when given the spring constant (K) and the force (F), use Hook's Law rearranged as X = F/K.
  • 🌐 Hook's Law is an empirical guideline rather than an absolute law of physics, holding true for most springs within a certain range of displacements but not perfectly accurate at all times.
Q & A

  • What is Hooke's Law and how is it related to the force of a spring?

    -Hooke's Law states that the force (F) exerted by a spring is proportional to its displacement (X) from the equilibrium position and is given by the formula F = -kX, where k is the spring constant. The negative sign indicates that the force is a restoring force, attempting to return the spring to its equilibrium position.

  • How do you determine the spring constant (k) using Hooke's Law?

    -To determine the spring constant, you apply a known force to the spring and measure the resulting displacement from its equilibrium position. The spring constant can then be calculated as k = F/X, where F is the applied force and X is the displacement.

  • What is the relationship between the spring constant (k) and the stiffness of a spring?

    -A larger spring constant (k) indicates a stiffer spring. This means that for a given displacement, a stiffer spring will exert a greater force compared to a less stiff spring with a smaller spring constant.

  • How is the elastic potential energy stored in a spring calculated?

    -The elastic potential energy (U) stored in a spring is given by the formula U = (1/2)kX^2, where k is the spring constant and X is the displacement from the equilibrium position.

  • What does the shape of the force-displacement graph represent in terms of work done on a spring?

    -The area under the force-displacement graph represents the work done on the spring. In the case of a linear relationship described by Hooke's Law, the shape is typically a triangle, and the area can be calculated using the formula for the area of a triangle, which is (1/2)base * height.

  • How can you find the displacement (X) if you know the spring constant (k) and the force (F)?

    -Using Hooke's Law, F = kX, you can find the displacement (X) by rearranging the formula to X = F/k. By dividing the known force (F) by the spring constant (k), you can calculate the displacement from the equilibrium position.

  • What is the significance of the work done in compressing or stretching a spring?

    -The work done in compressing or stretching a spring is equal to the potential energy stored in the spring. This energy can be released or used to do work when the spring returns to its equilibrium position.

  • How does the potential energy stored in a spring change with different spring constants and displacements?

    -The potential energy stored in a spring, given by the formula U = (1/2)kX^2, increases with both an increase in the spring constant (k) and the displacement (X) from the equilibrium position. A stiffer spring (larger k) or a greater displacement (larger X) will result in more potential energy being stored.

  • What units are typically used for the spring constant (k) and how does this affect the calculation?

    -The spring constant (k) is typically expressed in Newtons per meter (N/m). However, it can also be given in other units such as Newtons per centimeter (N/cm). The unit used affects the numerical value of k, but the relationship between force, displacement, and potential energy remains consistent.

  • What happens to the force exerted by a spring if you stretch it beyond its elastic limit?

    -Hooke's Law is an empirical relationship that holds true for most springs within their elastic limit. Beyond this point, the relationship between force and displacement becomes non-linear, and Hooke's Law no longer accurately describes the behavior of the spring. The force may continue to increase, but at a decreasing rate, or the spring may undergo permanent deformation.

  • How can you calculate the potential energy stored in a spring if the force-displacement graph is not triangular?

    -If the force-displacement graph is not triangular, you would need to calculate the area under the curve using integration or by approximating the shape with a series of triangles or other geometric shapes, depending on the complexity of the curve.

Outlines
00:00
πŸ“š Introduction to Hook's Law and Elastic Potential Energy

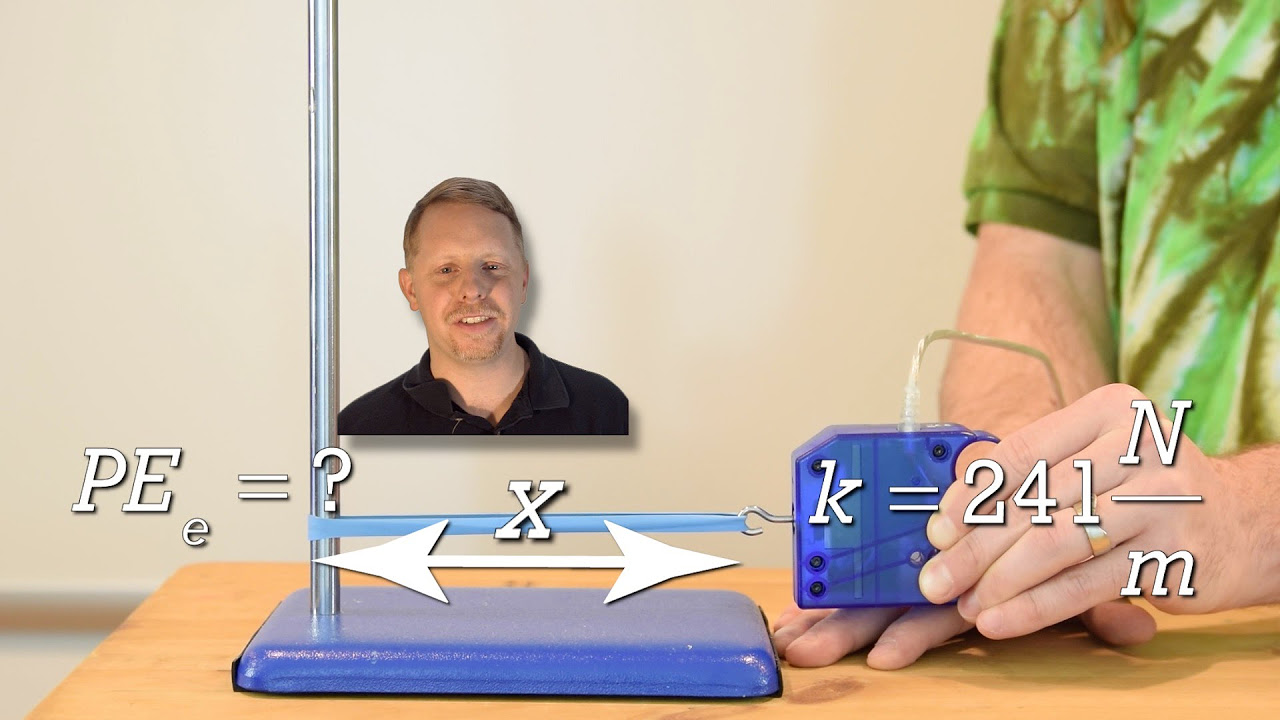
This paragraph introduces the concepts of Hook's Law and elastic potential energy in the context of springs. It outlines the objective to use Hook's Law to determine the elastic force on an object and to calculate a system's elastic potential energy. The paragraph explains that Hook's Law describes a linear relationship between the force exerted by a spring and its displacement from the equilibrium position, represented by the formula FS = -kX. The negative sign indicates that the force is a restoring force, attempting to return the spring to its equilibrium position. The paragraph also clarifies that while Hook's Law is a useful model, it is not a perfect representation of all springs' behavior. It concludes by discussing the method of determining the spring constant through experimentation, involving stretching or compressing the spring and measuring the required force and displacement to create a force-displacement graph.

05:30
πŸ” Calculating Spring Constant and Work Done

This paragraph delves into the process of calculating the spring constant and the work done on a spring. It presents a method for determining the spring constant by applying varying forces and measuring the resulting displacements, then plotting these values on a force-displacement graph. The slope of this graph represents the spring constant (k). The paragraph also explains how to calculate the work done on a spring, which is the potential energy stored within it. This is done by finding the area under the force-displacement graph, which in the case of a triangular shape, is calculated using the formula (1/2) * base * height. The paragraph provides an example where a spring is stretched by 0.1 meters with a force of 20 Newtons, resulting in a spring constant of 200 Newtons per meter and work done equal to 1 Joule.

10:33
πŸ“ˆ Graphical and Analytical Analysis of Springs

This paragraph discusses the graphical and analytical methods for analyzing springs. It explains how to calculate the spring constant and the potential energy stored in a spring using Hook's Law. The paragraph provides examples of how to determine the spring constant from a force-displacement graph and how to calculate the potential energy using the formula (1/2) * k * x^2. It also describes how to find the displacement of a spring when a known force is applied, using the force-spring relationship from Hook's Law. The paragraph concludes with examples of calculating the spring constant and potential energy for different scenarios, emphasizing the practical application of the concepts discussed.

Mindmap
Keywords
πŸ’‘Hooke's Law
Hooke's Law is a fundamental principle in physics that describes the linear relationship between the force exerted by a spring and its displacement from the equilibrium position. It states that the force (F) is equal to the spring constant (k) multiplied by the displacement (x), represented as F = kx. In the video, this law is used to calculate the elastic force on an object and to determine the spring constant. It's important to note that while it's often referred to as a 'law,' it is more of an empirical guideline that holds true for most springs within a certain range of displacement.
πŸ’‘Elastic Potential Energy
Elastic potential energy is the energy stored in a system when it is deformed elastically, such as when a spring is stretched or compressed. This energy is stored due to the potential for the system to do work and is released when the deformation is allowed to return to its equilibrium state. In the context of the video, the elastic potential energy stored in a spring is calculated using the formula PE = 0.5 * k * x^2, where k is the spring constant and x is the displacement from the equilibrium position.
πŸ’‘Spring Constant (k)
The spring constant, denoted as k and measured in Newtons per meter (N/m), is a physical quantity that characterizes the stiffness of a spring. A higher spring constant indicates a stiffer spring, meaning it takes more force to cause the same displacement. The spring constant is determined through experiments where forces and corresponding displacements are measured and used to calculate the slope of the force-displacement graph, according to Hooke's Law.
πŸ’‘Displacement (x)
Displacement, in the context of a spring, refers to the distance the spring is stretched or compressed from its equilibrium position. The equilibrium position is the spring's natural length where it exerts no net force. Displacement is a crucial variable in Hooke's Law, as it directly influences the force exerted by the spring. The negative sign in the formula F = kx indicates that the force exerted by the spring is a restoring force, acting in the opposite direction to return the spring to its equilibrium position.
πŸ’‘Restoring Force
A restoring force is a force that acts to return a system from a displaced position to its equilibrium position. In the case of a spring, the restoring force is the force that tries to bring the spring back to its original, unstretched, or uncompressed state. This force is described by Hooke's Law, where the force exerted by the spring (F) is proportional to the displacement (x) and is directed opposite to the direction of displacement. The negative sign in the formula F = kx indicates the direction of this force.
πŸ’‘Work (W)
In physics, work (W) is a measure of energy transfer that occurs when an object is moved by an external force along a displacement. Work is calculated as the product of the force applied (F) and the displacement (x) in the direction of the force. In the context of springs, work done on the spring (compressing or stretching it) is converted into stored energy within the spring. The work done can be visualized as the area under the force-displacement graph, which in the case of a linear spring, takes the shape of a triangle.
πŸ’‘Force (F)
In the context of the video, force (F) refers to the external force applied to a spring, which causes it to deform, either by stretching or compressing it. According to Hooke's Law, the force exerted by a spring is directly proportional to its displacement from the equilibrium position and is described by the formula F = kx. The force is a key factor in determining the spring constant and in calculating the work done on or by the spring.
πŸ’‘Energy
Energy is the capacity to do work, and in the context of the video, it refers to the potential energy that is stored in a spring when it is deformed. This stored energy can be converted back into mechanical energy when the spring returns to its equilibrium position. The amount of energy stored is directly related to the spring constant and the displacement of the spring from its equilibrium position.
πŸ’‘Equilibrium Position
The equilibrium position of a spring is its natural state when no external forces are acting upon it. In this position, the spring is neither stretched nor compressed, and it exerts no net force. Any displacement from this position results in the spring exerting a restoring force in accordance with Hooke's Law, attempting to return to its equilibrium state.
πŸ’‘Deformation
Deformation refers to the change in shape or size of an object under the influence of an external force. In the case of a spring, deformation occurs when it is either stretched or compressed. Elastic deformation is temporary and reversible, meaning the spring can return to its original shape once the external force is removed. The video discusses how the force exerted by the spring is proportional to the amount of deformation (displacement from the equilibrium position).
πŸ’‘Graph
In the context of the video, a graph is a visual representation used to illustrate the relationship between two variables, such as force and displacement for a spring. The graph of a spring's force-displacement relationship is typically a straight line when the spring obeys Hooke's Law, indicating a linear relationship. The slope of this line represents the spring constant, and the area under the line can be used to calculate the work done or the energy stored in the spring.
Highlights

Introduction to Springs and Hook's Law

Objective to use Hook's Law to determine the elastic force on an object

Objective to calculate a system's elastic potential energy

Explanation of the linear relationship between the force of a spring and its displacement

Definition of the spring constant (K) and its relation to the stiffness of the spring

Discussion on the empirical nature of Hook's Law and its limitations

Method to determine the spring constant through force-displacement graph analysis

Calculation of the work done in compressing or stretching a spring

Derivation of the formula for the potential energy stored in a spring

Practical example of calculating the spring constant from force and displacement

Illustration of calculating the potential energy stored in a compressed spring

Procedure to find the spring constant when given force and change in length

Application of the potential energy formula to a specific spring scenario

Summary of the key formulas for the force on a spring and the potential energy in a spring

Encouragement for further learning and seeking help for understanding Springs

Transcripts

Browse More Related Video

Potential energy stored in a spring | Work and energy | Physics | Khan Academy

(previous version) Introduction to Elastic Potential Energy with Examples

Simple Harmonic Motion, Mass Spring System - Physics Full Topic

Hooke's Law and Elastic Potential Energy

Elastic Potential Energy - IB Physics

GCSE Physics - Elastic Potential Energy and F = ke Equations #45

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsEducationHookesLawElasticPotentialEnergySpringMechanicsMechanicalWorkForceCalculationsSpringConstantEnergyStorageSTEMLearningPracticalPhysics