AP Physics Workbook 6.A Forces in Simple Harmonic Motion
TLDRThis video script discusses the concept of simple harmonic motion in physics, focusing on a scenario where a cart attached to a spring oscillates on a smooth horizontal track. It explains the periodic nature of the motion, the role of the restorative force (as described by Hooke's Law), and how the force and displacement of the cart change throughout its oscillation. The video also includes the creation of free body diagrams to illustrate the forces acting on the cart at different positions and explains how these forces relate to the cart's motion and equilibrium.
Takeaways
- 📚 The concept of simple harmonic motion is introduced, focusing on a scenario involving a cart of mass M attached to an ideal spring on a smooth horizontal track.
- 🏋️♂️ The cart is subject to a restorative force, which acts to return the mass to its equilibrium position when it is displaced, as described by Hooke's law (F = -kx).
- ⏱️ Each oscillation of the mass takes the same amount of time, indicating that the motion is periodic and the equilibrium position is when the displacement x equals zero.
- 🔄 The spring constant (k) is a measure of the spring's stiffness, with higher values indicating a stiffer spring and a greater force needed to cause displacement.
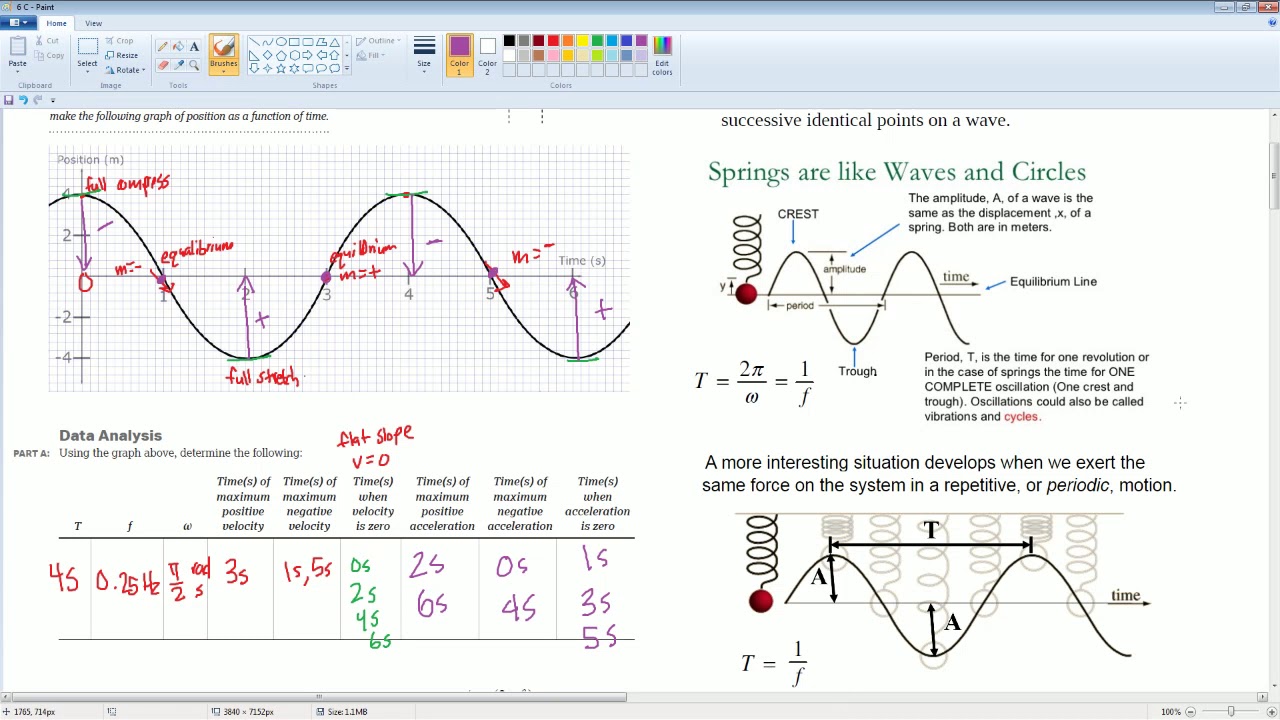
- 📊 A position diagram is used to illustrate the cart's motion between points A and E, showing how the distance from equilibrium varies as the cart moves.
- 🚗 Free body diagrams are drawn to represent the forces acting on the cart at different positions during its motion, both to the right and to the left.
- 🔽 The net force on the cart is indicated by the relative lengths of the force vectors in the diagrams, which reflect the magnitude of the forces.
- 🔄 The motion is symmetrical, meaning the forces acting on the cart are mirrored around the equilibrium position, with the only difference being the direction of the velocity.
- 🔄 At the amplitude points (a and e), the velocity of the cart is zero and the acceleration is at its maximum, indicating a large force from the spring.
- 🎯 The direction of the net force by the spring on the cart is always opposite to the direction of displacement from equilibrium, aiming to restore the cart to its equilibrium position.
- 📈 The cart's velocity is greatest at the equilibrium position (C) and decreases as it moves towards the amplitude points, where the velocity is zero and the force is maximized.
Q & A
What is simple harmonic motion?
-Simple harmonic motion is a type of periodic motion where an object moves back and forth over the same path. It is characterized by a restoring force that brings the object back to its equilibrium position.
What is the significance of the equilibrium position in simple harmonic motion?
-The equilibrium position is the point where the object begins its oscillation and where it has the least potential energy. It is the position where the net force acting on the object is zero, and it serves as the reference point for displacement in the motion.
What is the restorative force in the context of a spring-mass system?
-The restorative force is the force exerted by the spring on the mass, which acts in the direction of returning the mass to its equilibrium position. This force is described by Hooke's Law, where it is proportional to the displacement of the mass from the equilibrium position and is directed opposite to the displacement.
How is Hooke's Law expressed mathematically?
-Hooke's Law is expressed as F = -kx, where F is the force exerted by the spring, k is the spring constant (a measure of the spring's stiffness), and x is the displacement from the equilibrium position. The negative sign indicates that the force is always in the opposite direction to the displacement.
What is the relationship between the spring constant and the force required to stretch the spring?
-The spring constant (k) determines the stiffness of the spring. A larger spring constant means that a greater force is needed to stretch the spring by a given distance. It is directly proportional to the force required for a given displacement.
How does the motion of the cart change as it moves from point A to point E?
-As the cart moves from point A to point E, it experiences a change in velocity and acceleration. It starts at rest at point A, accelerates as it moves away from the equilibrium position, reaches maximum velocity at the amplitude points (E and C), decelerates as it approaches the equilibrium position again, and reverses direction at point E.
What are the forces acting on the cart in the free body diagram?
-In the free body diagram, the forces acting on the cart include the spring force (restorative force), gravitational force, and the normal force. The spring force is horizontal and directed opposite to the displacement, while the gravitational force and normal force are vertical and balanced in the horizontal direction.
How can you determine the direction of the cart's motion from the free body diagram?
-The direction of the cart's motion can be inferred from the direction of the spring force in the free body diagram. If the spring force is to the right, the cart is moving to the left, and vice versa. However, the diagram itself does not directly indicate the motion direction; it must be interpreted based on the context of the problem.
What happens to the cart's acceleration as it approaches the equilibrium position?
-As the cart approaches the equilibrium position, its acceleration decreases. At the equilibrium position, the acceleration is zero because this is the point where the restorative force is balanced by the cart's inertia, and it is not accelerating.
How does the symmetry of the motion affect the forces and displacement?
-The symmetry of the motion means that the forces and displacement are mirrored across the equilibrium position. The magnitude of the forces and the displacement are the same on either side of the equilibrium, but their directions are opposite, reflecting the restoring nature of the spring force.
What is the role of the spring constant in determining the amplitude of the oscillation?
-The spring constant influences the amplitude of the oscillation by affecting the energy transfer within the system. A stiffer spring (higher spring constant) will result in a smaller amplitude of oscillation, while a less stiff spring (lower spring constant) will allow for a larger amplitude.
Outlines
📘 Introduction to Simple Harmonic Motion
This paragraph introduces the concept of simple harmonic motion within the context of a physics workbook. It describes a scenario where a cart of mass M is attached to an ideal spring, resting on a smooth horizontal track. The cart, when pulled to a position 'a' and released, oscillates back and forth. The section emphasizes the periodic nature of simple harmonic motion, the equilibrium position (where x=0), and the concept of the restorative force, which is the force exerted by the spring to return the mass to its equilibrium position. Hooke's law is introduced as F=-kx, highlighting the spring constant (k) and its relationship with the force needed to stretch or compress the spring. The paragraph also discusses the energy dynamics within the system, explaining how external forces and the spring force (kx) interact.
📊 Position Diagrams and Free Body Diagrams
This paragraph delves into the representation of the cart's motion through position diagrams and free body diagrams. It explains how different positions of the cart are labeled and depicted, with a focus on the cart's movement from point A to E. The paragraph outlines the creation of two sets of sketches, one for the cart moving to the right and another for the cart moving to the left. It also touches on the forces acting on the cart at various positions, such as the amplitude of the force at the maximum displacement (point 'a') and the diminishing force as the cart approaches equilibrium. The paragraph further discusses the symmetry of the motion and the implications of the forces on the cart's velocity and acceleration.
🔍 Analyzing the Direction of Forces and Acceleration
This paragraph focuses on the analysis of the direction of forces and acceleration of the cart in simple harmonic motion. It explains how the acceleration changes as the cart moves closer to or further from the equilibrium position, with greater acceleration at points 'a' or 'e' and minimal acceleration at point 'C'. The paragraph also discusses the relationship between the direction of the net force exerted by the spring and the cart's displacement from equilibrium. It clarifies that the direction of the net force is independent of the cart's direction of motion, as evidenced by the identical free body diagrams for the cart moving to the right and left. The explanation emphasizes the restorative force of the spring and how it drives the cart towards equilibrium.
Mindmap
Keywords
💡Simple Harmonic Motion
💡Restorative Force
💡Hooke's Law
💡Spring Constant
💡Equilibrium Position
💡Periodic Motion
💡Displacement
💡Free Body Diagram
💡Amplitude
💡Acceleration
Highlights
The scenario involves a cart of mass M attached to an ideal spring on a smooth horizontal track, exhibiting simple harmonic motion.
In simple harmonic motion, the time taken for each oscillation is constant, making it a periodic motion.
The position of the mass M at equilibrium is denoted as x equals 0, where the spring exerts no force.
The restorative force, or the force exerted by the spring, is directed towards the equilibrium position and is described by Hooke's law, F = -kx.
The negative sign in Hooke's law indicates that the restorative force is always opposite to the displacement of the mass from the equilibrium position.
The spring constant K represents the stiffness of the spring, with units of newtons per meter (N/m).
A greater value of K indicates a stiffer spring, requiring a greater force to stretch the spring by a given distance X.
The position diagram illustrates the cart's motion between points A and E, showing the restorative force's effect on the cart's movement.
At the equilibrium position, the cart is subject to equal distances from labeled points, indicating uniform motion.
As the cart moves further from equilibrium, the restorative force increases, causing the cart to slow down and eventually reverse direction.
The free body diagram represents all forces acting on the cart at different positions during its motion.
The force diagrams for the cart moving to the right and left are symmetrical, reflecting the nature of simple harmonic motion.
The amplitude of the cart's velocity is zero at the maximum displacement (points A and E) and maximum at the equilibrium (point C).
The net force on the cart at any position can be determined by the direction and magnitude of the spring force.
At equilibrium, there is no net horizontal force acting on the cart, as the restorative force is zero.
The direction of the net force by the spring on the cart is opposite to the cart's displacement from equilibrium.
Hooke's law explains the tendency of the cart to move towards equilibrium, with the restorative force acting as the driving mechanism.
Transcripts
Browse More Related Video
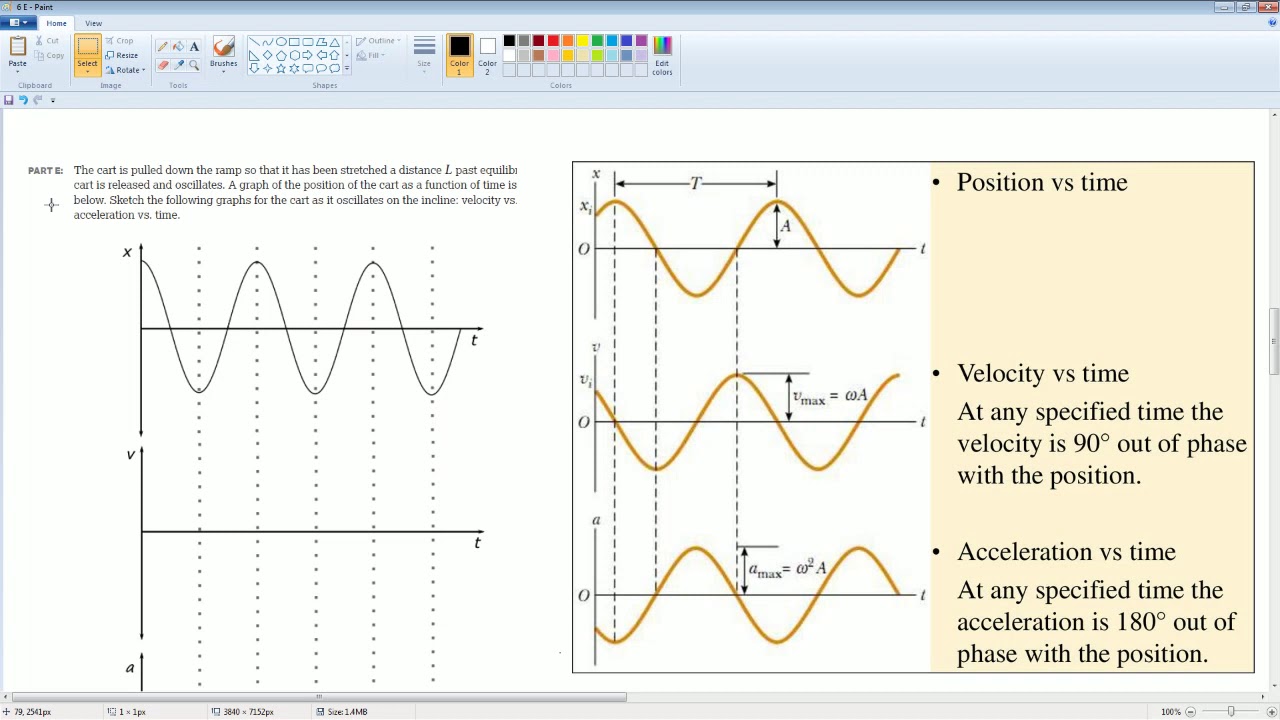
AP Physics Workbook 6.E Equilibrium on an Incline
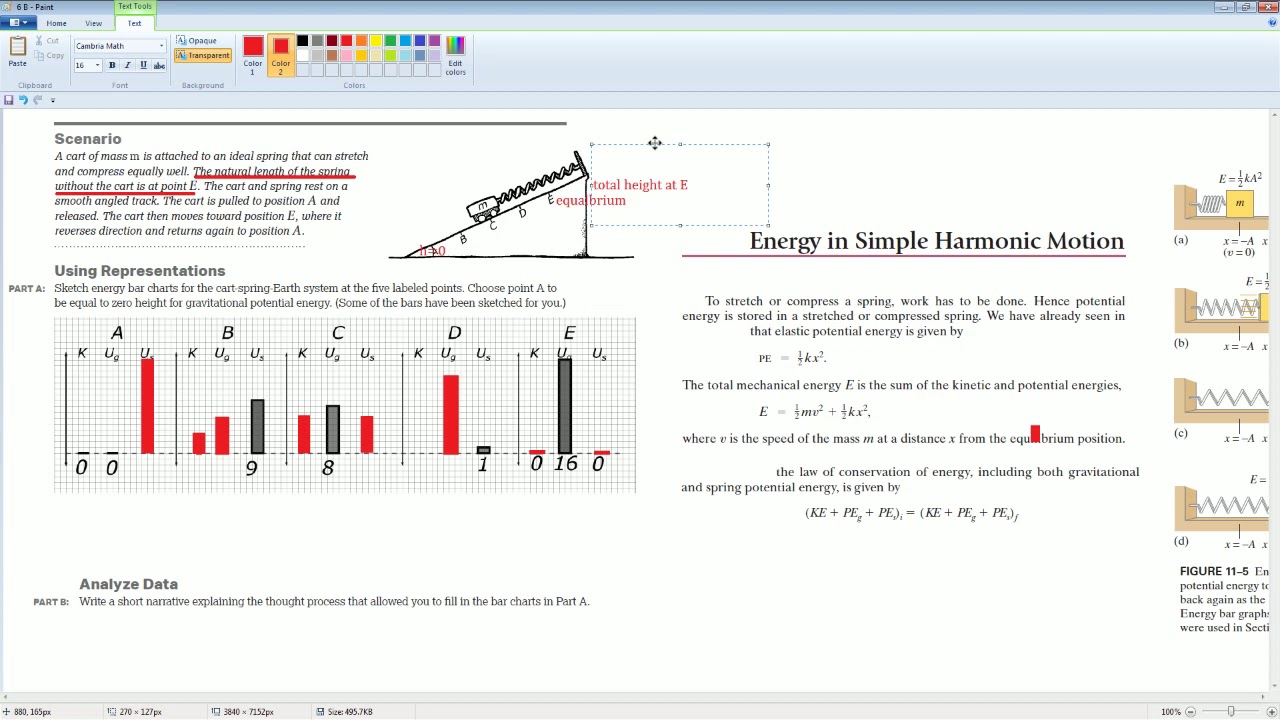
AP Physics Workbook 6.B Simple Harmonic Motion and Energy Review
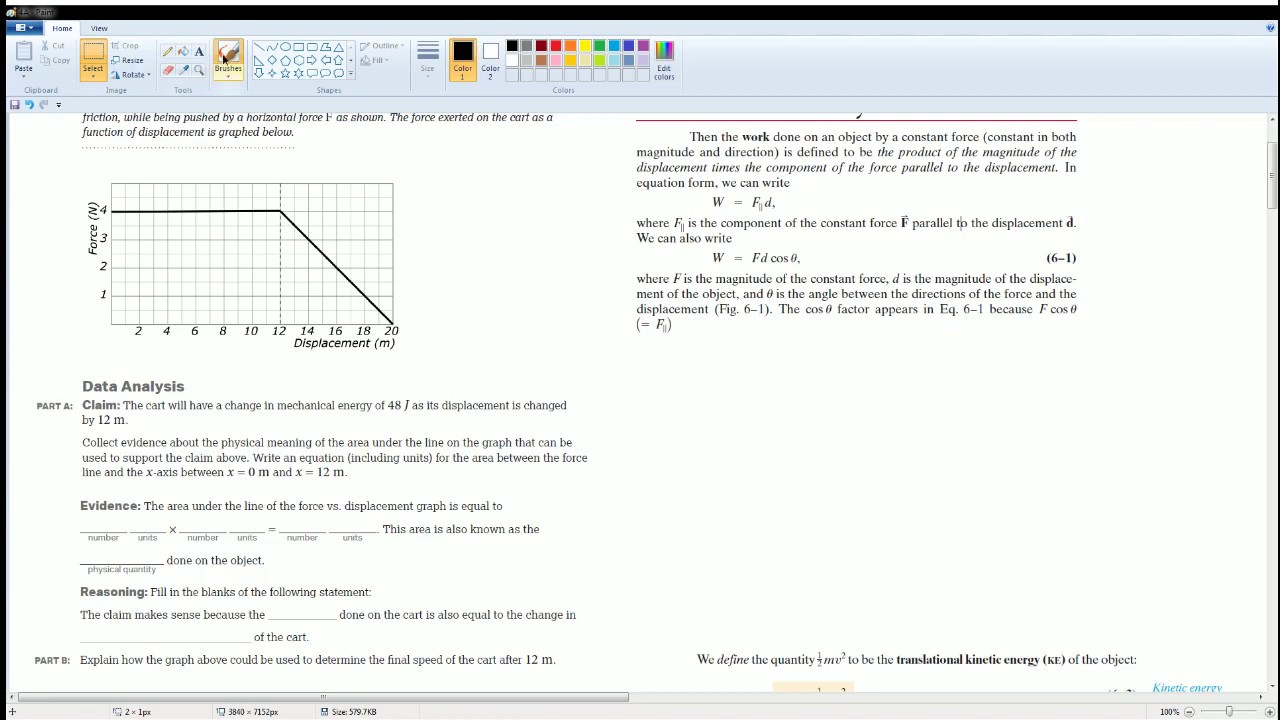
AP Physics Workbook 4.A Work

AP Physics Workbook 4.O Conservation of Energy and Circular Motion
Simple Harmonic Motion, Mass Spring System - Amplitude, Frequency, Velocity - Physics Problems
AP Physics Workbook 6.C Equations of Motion for Simple Harmonic Motion
5.0 / 5 (0 votes)
Thanks for rating: