AP Physics Workbook 3.N Newton's Law of Universal Gravitation
TLDRThe transcript details a lesson on applying Newton's law of universal gravitation to calculate the mass of Jupiter using data about its moons' orbits. The process involves entering and manipulating data in Excel, squaring orbital periods, and deriving equations to find the mass. The lesson also discusses the gravitational forces between two probes sent to study Jupiter's moon, Titan, with one in orbit and the other on the moon's surface, illustrating how gravitational force varies with distance and mass.
Takeaways
- 📚 The lesson focuses on applying Newton's law of universal gravitation to calculate the mass of Jupiter using data about its moons' orbits.
- 🌍 Assumption of circular motion is made for Jupiter's moons' orbits to simplify calculations.
- 🔢 Data entry in Excel is crucial for calculations, with special attention to formatting numbers with exponents correctly.
- 📊 The orbital period and radius of the moons are squared and cubed, respectively, for the calculation process.
- 🎯 The goal is to derive an equation to solve for the mass of Jupiter (M) using the orbital period (T) and radius (R) of the moons' orbits.
- 🔧 The force of gravity between Jupiter and its moons is balanced by the centripetal force required for circular motion.
- 📈 A linear relationship is established by graphing the period squared (T^2) against the radius cubed (R^3) for a straight line.
- 🚀 Two identical probes are sent to study Jupiter's moon, with one in orbit and the other on the moon's surface.
- ⚖️ Gravitational forces are compared between the probe in orbit and the one on the moon's surface, with the understanding that they vary based on distance and mass.
- 📐 The relationship between gravitational force, mass, and distance is key to understanding the differences in forces experienced by the probes.
- 🌌 A practical analogy is used to remember the concept, comparing gravitational attraction to the relationship between people (mass) and their proximity (distance).
Q & A
What is the main topic of the transcript?
-The main topic of the transcript is the explanation of a physics problem related to circular motion and gravitation, specifically using data from Jupiter's moons to determine the mass of Jupiter.
What are the key formulas used in the script to solve the problem?
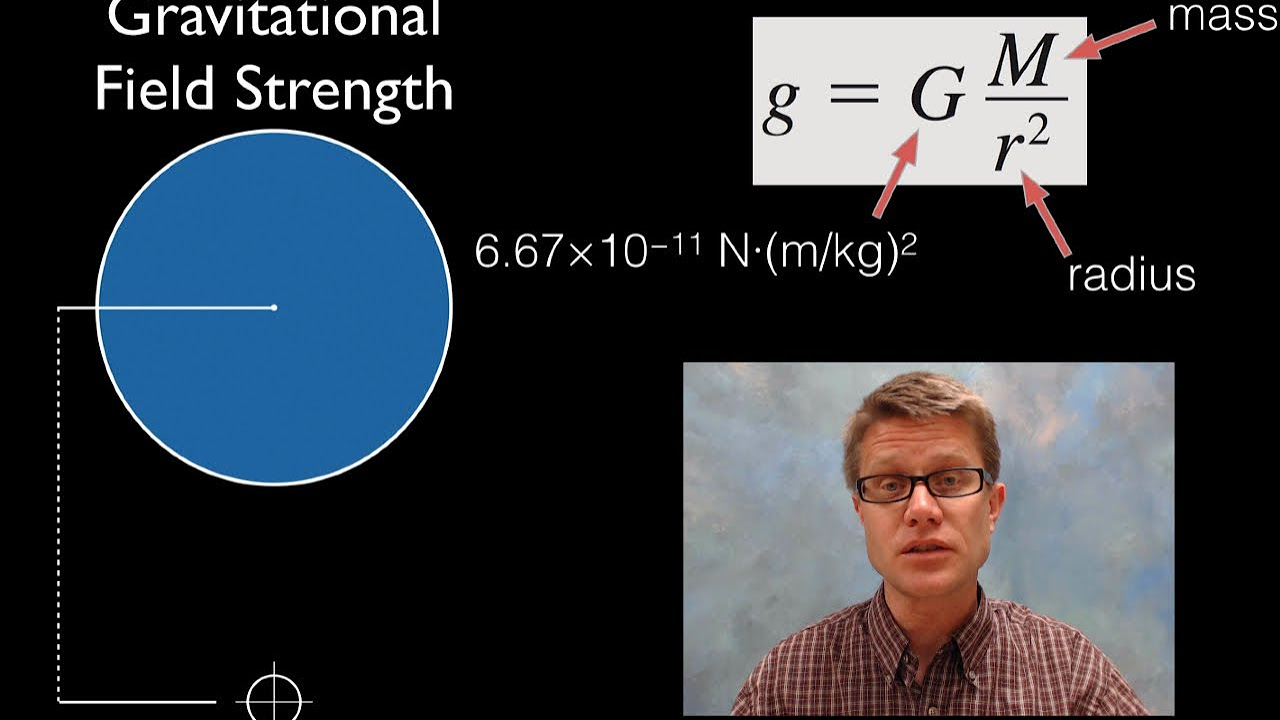
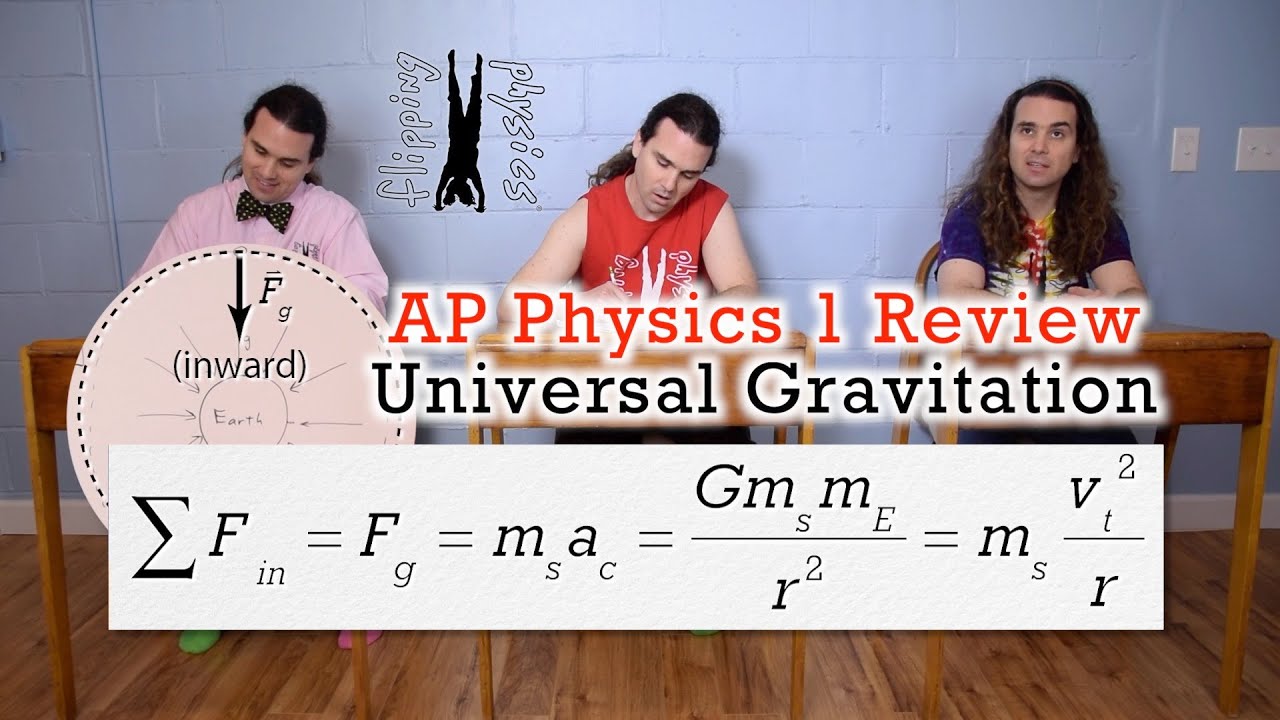
-The key formulas used are Newton's law of universal gravitation (F = G * (m1 * m2) / r^2) and the relationship between orbital period (T), radius (r), and gravitational constant (G) derived from the problem (T^2 = (4 * pi^2 * r^3) / (G * M), where M is the mass of the central body).
How does the speaker suggest entering data into Excel for calculation?
-The speaker suggests entering data into Excel by using columns for different data sets, using the caret symbol (^) for exponentiation, and utilizing Excel's autofill feature to quickly square or cube numbers as needed.
What is the significance of squaring and cubing the orbital radius in the calculations?
-Squaring the orbital radius is part of the process to find the gravitational force acting on the moon, while cubing the radius is necessary to derive the equation for the orbital period in terms of the radius and the mass of Jupiter.
How does the speaker describe the relationship between the gravitational force and the orbital period of Jupiter's moons?
-The speaker describes the relationship by stating that the square of the orbital period is directly proportional to the cube of the orbital radius, as derived from the equation T^2 = (4 * pi^2 * r^3) / (G * M).
What is the purpose of graphing the data as radius cubed vs. period squared in the script?
-The purpose of graphing the data as radius cubed vs. period squared is to obtain a linear relationship, which can be used to determine the mass of Jupiter by fitting a line to the data points and finding the equation of the line.
What are the two different scenarios described for the probes sent to study one of Jupiter's moons?
-The two scenarios are: Probe A is in a geostationary orbit around the moon, and Probe B rests on the surface of the moon and rotates around it.
How does the speaker use Newton's third law to compare the gravitational forces between the moon and the two probes?
-The speaker uses Newton's third law to state that the forces are action-reaction pairs, meaning the force of the moon on Probe A is equal to the force of Probe A on the moon, and similarly for Probe B. This law is used to establish that certain forces are equal in magnitude but opposite in direction.
What is the conclusion about the relative magnitudes of the gravitational forces between the moon and the two probes?
-The conclusion is that the gravitational force when the radius is small (Probe B on the moon's surface) is greater than when the radius is large (Probe A in orbit), due to the inverse square relationship between force and distance.
How does the speaker use an analogy with high school couples to explain the concept of gravitational attraction?
-The speaker uses the analogy of high school couples with different sizes (representing masses) to illustrate how larger masses ('fat couple') have a stronger gravitational attraction (gravitational field) than smaller masses ('skinny couple'), and are more likely to stay together due to this stronger attraction.
What is the practical application of the concepts discussed in the script?
-The practical application is to understand and calculate the gravitational forces acting on celestial bodies, such as Jupiter's moons, which can help in space exploration, understanding planetary dynamics, and determining the mass of celestial bodies like Jupiter.
Outlines
📚 Introduction to AP Physics: Circular Motion and Gravitation
The paragraph introduces an AP Physics workbook solution focusing on Unit 3, specifically circular motion and gravitation. It presents a scenario where data about some of Jupiter's moons is given, and the task is to use this data to calculate the mass of Jupiter, assuming the moons' orbits are circular. The paragraph explains the need to input and manipulate data in Excel, detailing the process of entering numbers, using exponents, and filling in data sheets. It emphasizes the importance of understanding Excel functions for solving such problems.
🧮 Deriving the Equation for Gravitational Force
This paragraph delves into the derivation of the gravitational force equation. It explains the force of gravity as a product of the gravitational constant, the masses of the two objects (in this case, Jupiter and its moon), and the inverse square of the distance between them. The paragraph further discusses the relationship between the moon's velocity and its orbital radius, and how this can be used to cancel out variables and derive an equation for the orbital period. It emphasizes the importance of understanding these relationships to solve for unknown quantities.
📈 Graphing the Relationship between Orbital Period and Radius
The paragraph explains the process of graphing the relationship between the orbital period squared and the radius cubed of Jupiter's moons. It describes how to use Excel to input the data, create a scatter plot, and add a trendline to the graph. The goal is to obtain a linear relationship, which can be used to determine the mass of Jupiter. The paragraph provides a step-by-step guide on how to label the graph, interpret the results, and ensure a proper linear fit by having an equal number of data points above and below the line of best fit.
🚀 Comparing Gravitational Forces in Different Scenarios
This paragraph discusses a hypothetical scenario where two identical probes are sent to study one of Jupiter's moons, with one probe in a geostationary orbit and the other on the moon's surface. It uses Newton's third law to establish that the forces between the moon and the probes are equal and opposite. The paragraph then compares the gravitational forces in these two scenarios, explaining that the force is greater when the radius (distance between the objects) is smaller. It concludes by ranking the gravitational forces from least to greatest based on the mass of the objects and the radius of their orbit or distance from the moon's surface.
💡 Memorizing Gravitational Relationships with Analogies
The final paragraph offers a mnemonic device to remember the relationships between gravitational force, mass, and distance. It uses the analogy of high school couples with varying 'masses' (sizes) to illustrate how the gravitational 'attraction field' changes with distance (how close or far they are from each other). The analogy is extended to compare 'fat' and 'skinny' couples, suggesting that the 'fat' couple (representing larger masses) will have a stronger gravitational attraction and are more likely to 'stay together' than the 'skinny' couple. This creative approach aims to help students visualize and remember the principles of gravitational force.
Mindmap
Keywords
💡Circular Motion
💡Newton's Law of Universal Gravitation
💡Excel
💡Orbital Radius
💡Orbital Period
💡Gravitational Constant
💡Centripetal Force
💡Data Analysis
💡Physics Calculation
💡Excel Functions
Highlights
Introduction to the AP Physics workbook solution for Unit 3: Circular Motion and Gravitation, specifically Section 3.5 on Newton's Law of Universal Gravitation.
Explanation of a scenario involving Jupiter's moons and using their data to determine the mass of Jupiter by assuming circular orbits.
Instructions on how to use Excel for scientific calculations, including entering and manipulating data with exponents.
A step-by-step guide on entering and squaring the orbital period and radius data for Jupiter's moons in Excel.
Derivation of the equation for gravitational force based on the mass of Jupiter, the mass of its moon, and the radius of the moon's orbit.
Introduction of the concept that the force of gravity is equal to the gravitational constant times the masses divided by the radius squared.
Explanation of how to derive the equation for the moon's velocity in orbit using its orbital period and the concept of circular motion.
Derivation of the equation relating the moon's orbital period squared to the radius cubed, which is key for solving the problem.
Discussion on how to graph the relationship between the period squared and the radius cubed to obtain a straight line, which is essential for solving the problem.
Explanation of the practical application of the derived equations in determining the mass of celestial bodies like Jupiter using the motion of its moons.
Illustration of the concept of action and reaction pairs in Newton's third law through the gravitational forces between Jupiter's moon and probes.
Comparison of the magnitude of gravitational forces in different scenarios, such as a probe in orbit versus one on the moon's surface.
Memorization technique using analogies of high school couples to understand the relationship between mass, distance, and gravitational force.
Innovative teaching method that combines physics concepts with practical software tools like Excel to enhance understanding and problem-solving skills.
Detailed walkthrough of scientific data entry and calculation in Excel, which is beneficial for students and professionals alike.
Comprehensive explanation of the mathematical derivations behind the Law of Universal Gravitation, providing a deeper understanding of the principles.
Creative approach to visualizing and graphing data in Excel, which is not only useful for this specific physics problem but also transferable to other scientific disciplines.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: