Rotational kinematic formulas | Moments, torque, and angular momentum | Physics | Khan Academy

Khan Academy Physics
29 Jul 201614:50
EducationalLearning
32 Likes 10 Comments

TLDRThis instructional video delves into the concepts of rotational motion, drawing parallels with linear motion to explain angular displacement, velocity, and acceleration. It emphasizes that the principles and equations derived for linear motion apply to rotational motion as well, with the linear variables replaced by their rotational counterparts. The video also covers the use of graphs in understanding these concepts and provides a step-by-step approach to solving problems using rotational kinematic formulas, with examples involving a bar rotating with constant acceleration and deceleration.

Takeaways
  • 📐 The definitions of rotational motion variables (angular displacement, velocity, and acceleration) mirror those of linear motion, with the linear variable replaced by its rotational counterpart.
  • 📈 The principles and equations derived for linear motion also apply to rotational motion, as long as the linear variables are substituted with their rotational equivalents.
  • 📊 In velocity vs. time graphs, the slope represents acceleration for linear motion and angular acceleration for rotational motion.
  • 🔄 The area under the velocity vs. time curve represents displacement for linear motion and angular displacement for rotational motion.
  • 🌟 To derive rotational kinematic formulas, one can relate areas under velocity graphs to angular velocity and acceleration, resulting in formulas that are the same as linear kinematic formulas but with rotational variables.
  • 🚫 The rotational kinematic formulas only hold true when the angular acceleration is constant.
  • 🔢 To solve problems using rotational kinematic formulas, identify the known variables and the unknown variable, then use the appropriate formula that excludes the unknown.
  • ⏳ For a bar starting from rest and rotating through revolutions with a constant angular acceleration, the time taken can be calculated using the appropriate rotational kinematic formula.
  • 🌀 The final angular velocity of an object can be determined by considering the initial angular velocity, angular acceleration, and time of rotation.
  • 📌 The speed of a point on a rotating object is determined by the distance from the axis of rotation to the point and the angular velocity.
  • 🛑 When an object decelerates to a stop, the angular acceleration is negative and has the opposite sign to the initial angular velocity.
Q & A

  • What is the relationship between linear motion variables and rotational motion variables?

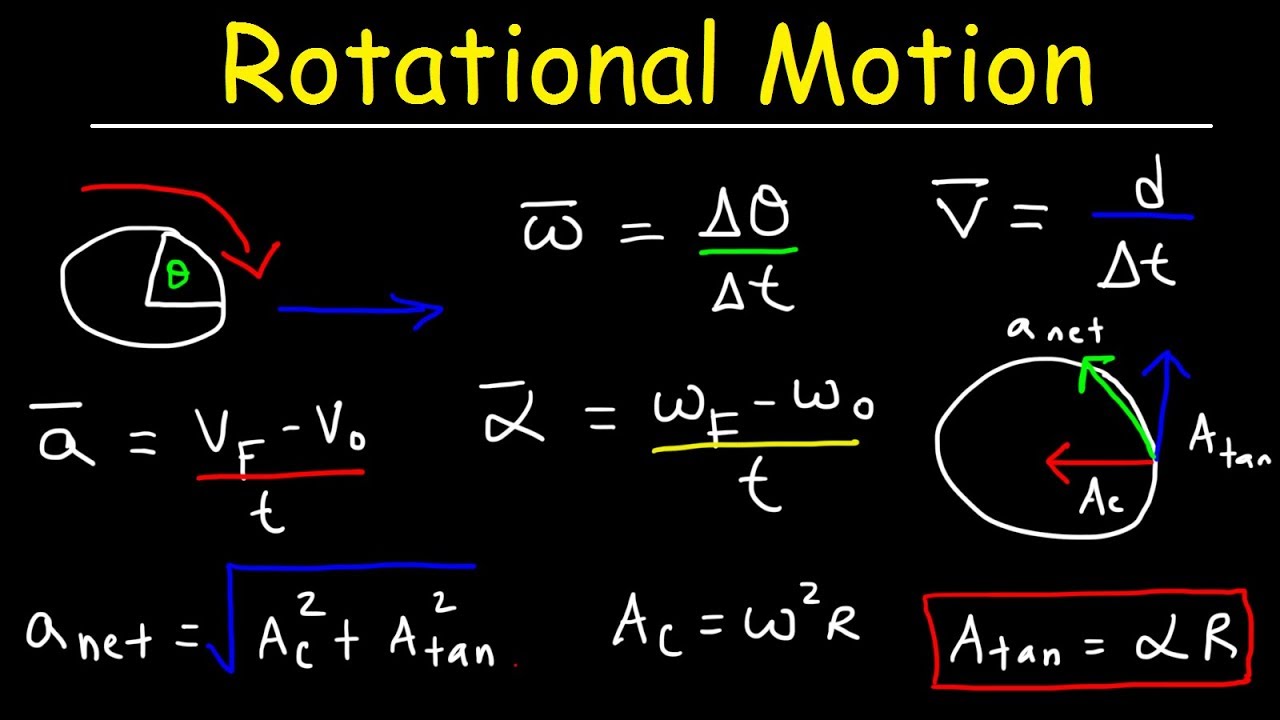
    -Linear motion variables and rotational motion variables are defined in an analogous manner. For instance, linear displacement corresponds to angular displacement, linear velocity corresponds to angular velocity, and linear acceleration corresponds to angular acceleration. The equations and principles derived for linear motion can also be applied to rotational motion by replacing the linear variables with their rotational counterparts.

  • How does the slope of a velocity versus time graph relate to acceleration and angular acceleration?

    -The slope of a velocity versus time graph represents the acceleration in linear motion. Similarly, in rotational motion, the slope of an angular velocity versus time graph represents the angular acceleration. This is because the relationship between velocity (v) and acceleration (a) is analogous to the relationship between angular velocity (ω) and angular acceleration (α).

  • What does the area under a velocity versus time graph represent?

    -The area under a velocity versus time graph represents the total displacement in linear motion. Similarly, the area under an angular velocity versus time graph represents the total angular displacement in rotational motion.

  • How can we derive rotational kinematic formulas?

    -We can derive rotational kinematic formulas by looking at areas under a velocity graph, just as we did for linear kinematic formulas. By relating these areas to angular velocity (ω) and angular acceleration (α), we can derive the rotational kinematic formulas. Alternatively, we can simply replace the linear motion variables in the linear kinematic formulas with their rotational counterparts.

  • Under what conditions do the rotational kinematic formulas hold true?

    -The rotational kinematic formulas are true when the angular acceleration is constant. This is similar to the condition for the linear kinematic formulas, which only hold true when the linear acceleration is constant.

  • What is the significance of the initial and final angular velocities in solving rotational kinematic problems?

    -The initial and final angular velocities (ω_initial and ω_final) are crucial in solving rotational kinematic problems. They are known variables in some cases and the variables we aim to find in others. The choice of which rotational kinematic formula to use depends on which of these variables is not involved in the problem.

  • How do you convert revolutions to radians?

    -One revolution is equivalent to 2π radians. Therefore, to convert revolutions to radians, you multiply the number of revolutions by 2π. For example, 5 revolutions would be 5 * 2π = 10π radians.

  • What is the relationship between the distance from the axis of rotation (r) and the linear speed of a point on a rotating object?

    -The linear speed of a point on a rotating object is directly proportional to the distance from the axis of rotation (r) and the angular velocity (ω). The speed (v) of the point can be calculated using the formula v = r * ω.

  • How do you determine the angular acceleration if an object decelerates to a stop?

    -When an object decelerates to a stop, the final angular velocity (ω_final) is zero. To determine the angular acceleration (α), you can use the rotational kinematic formula that does not involve time. By knowing the initial angular velocity (ω_initial), the angular displacement (Δθ), and the fact that ω_final is zero, you can solve for α.

  • What is the significance of the direction of angular displacement and angular acceleration?

    -The direction of angular displacement and angular acceleration should be consistent with the direction of angular velocity. If an object is speeding up (accelerating), then the direction of angular acceleration should be the same as the direction of angular velocity and angular displacement. Conversely, if an object is slowing down (decelerating), the angular acceleration should be in the opposite direction to the angular velocity and displacement.

  • How can you identify which rotational kinematic formula to use in solving a problem?

    -To identify which rotational kinematic formula to use, you need to look at the known variables and the unknown variable you are trying to find. The formula you choose should not include the variable that is neither given nor asked for. This is because you want to use the formula that allows you to solve for the unknown variable using the known variables.

Outlines
00:00
📚 Introduction to Rotational Motion Variables

This paragraph introduces the concept of rotational motion variables, drawing parallels with linear motion variables. The instructor explains that angular displacement, velocity, and acceleration are defined analogously to their linear counterparts, with the key difference being the replacement of linear motion variables with their rotational equivalents. The paragraph emphasizes that due to these similar definitions, the equations and principles derived for linear motion also apply to rotational motion, provided the linear variables are substituted with their rotational counterparts. The instructor further illustrates this concept using velocity-time graphs, explaining how the slope and area under the curve represent different aspects of motion in both linear and angular contexts.

05:00
🔍 Solving Problems with Rotational Kinematic Formulas

In this paragraph, the instructor guides through the process of solving problems using rotational kinematic formulas. The approach involves identifying known variables, determining the unknown variable to solve for, and selecting the appropriate formula based on which variable is not involved. The instructor uses a specific problem—where a bar rotates through five revolutions with a constant angular acceleration—to demonstrate the steps. By applying the formulas and converting revolutions to radians, the instructor calculates the time taken for the bar to complete the revolutions and the final angular velocity. This section emphasizes the importance of using consistent units and the correct kinematic formula to solve for the unknown variable.

10:03
🌐 Applying Kinematic Formulas to Real-World Scenarios

This paragraph delves into applying rotational kinematic formulas to more complex, real-world scenarios. The instructor presents a scenario where a four-meter long bar starts with an initial angular velocity and decelerates to a stop after a certain number of revolutions. The focus is on calculating the initial edge speed of the bar and the angular acceleration. The explanation involves using the relationship between linear speed and angular velocity, emphasizing how the distance from the axis of rotation affects the linear speed of points on the rotating bar. The paragraph concludes with solving for the angular acceleration using the known initial angular velocity, the number of revolutions, and the final angular velocity. The instructor also highlights the importance of considering the direction of angular displacement and acceleration when solving these problems.

Mindmap
Keywords
💡Rotational Motion
Rotational motion refers to the movement of an object where the direction of motion changes as it moves along a circular path. In the video, this concept is central as it discusses the variables and principles related to rotational motion, such as angular displacement, velocity, and acceleration, which are analogous to their linear counterparts but applied to circular motion. The example of a bar rotating through revolutions illustrates how these concepts are used to analyze and solve problems in rotational motion scenarios.
💡Angular Displacement
Angular displacement is the measure of the angle through which an object in rotational motion has turned. It is analogous to linear displacement but for circular paths. In the video, angular displacement is used to describe the position of an object in rotational motion, such as the number of revolutions a bar has completed. The example given is converting revolutions to radians to find the angular displacement of 10 pi radians after five revolutions.
💡Angular Velocity
Angular velocity is the rate at which an object in rotational motion changes its angular displacement. It is measured in radians per second and is directly analogous to linear velocity, which is the rate of change of position in linear motion. The video explains that angular velocity can be determined by the slope of an angular velocity versus time graph, similar to how the slope of a velocity versus time graph represents linear acceleration.
💡Angular Acceleration
Angular acceleration is the rate of change of angular velocity, indicating how quickly the speed of rotation is changing. It is given in radians per second squared, similar to linear acceleration which is measured in meters per second squared. In the context of the video, angular acceleration is a key variable in the rotational kinematic formulas, used to calculate the time taken for an object to complete a certain number of revolutions with a constant acceleration.
💡Kinematic Formulas
Kinematic formulas are mathematical equations that relate the variables of motion, such as displacement, velocity, acceleration, and time. The video discusses both linear and rotational kinematic formulas, emphasizing that the rotational formulas are derived by replacing linear variables with their rotational counterparts. These formulas are crucial for solving problems involving motion, whether in a straight line or along a circular path.
💡Graphs
Graphs are visual representations used to analyze and solve problems in physics. The video mentions velocity versus time graphs and angular velocity versus time graphs as tools to understand and calculate motion variables. For instance, the slope of a velocity-time graph represents acceleration, while the area under the graph corresponds to displacement in the case of linear motion, and angular displacement in the case of rotational motion.
💡Constant Angular Acceleration
Constant angular acceleration refers to a situation where the rate of change of angular velocity remains the same over time. This is a crucial assumption for the rotational kinematic formulas to be applicable. The video provides an example of a bar rotating with a constant angular acceleration, allowing the use of these formulas to calculate the time taken for the bar to complete five revolutions.
💡Rotational Kinematic Formulas
Rotational kinematic formulas are specific equations that relate the rotational motion variables of an object moving in a circular path. These formulas are derived from their linear counterparts by replacing linear variables with rotational ones. The video explains that these formulas are only valid when the angular acceleration is constant, and they can be used to solve for unknown variables given three known variables in a rotational motion scenario.
💡Initial and Final Conditions
Initial and final conditions refer to the starting and ending values of variables in a motion problem. In the context of the video, initial conditions include the starting angular velocity and angular position, while final conditions include the final angular velocity and angular position after a certain amount of time or rotations. These conditions are essential for applying the kinematic formulas to determine unknown variables, such as the time taken for a bar to rotate through a specific number of revolutions.
💡Deceleration
Deceleration is the decrease in velocity over time, which is the negative counterpart of acceleration. In rotational motion, deceleration is described as a negative angular acceleration. The video discusses an example where a bar starts with an initial angular velocity and decelerates to a stop, requiring the calculation of the angular acceleration, which is found to be negative, indicating a decrease in rotational speed.
Highlights

The rotational motion variables are defined similarly to linear motion variables, with the linear motion variable replaced by its angular counterpart.

Angular displacement is defined as the angular position, analogous to regular (linear) position.

Angular velocity is the angular displacement per unit time, similar to how velocity is the regular displacement over time.

Angular acceleration is the change in angular velocity per unit time, mirroring the definition of regular acceleration.

The equations and principles derived for linear motion also apply to rotational motion by replacing linear variables with their rotational equivalents.

In velocity-time graphs, the slope represents acceleration for linear motion and angular acceleration for rotational motion.

The area under the curve in a velocity-time graph represents displacement, and similarly, the area under an angular velocity-time graph represents angular displacement.

Rotational kinematic formulas can be derived from linear kinematic formulas by replacing linear motion variables with rotational ones.

The rotational kinematic formulas are only valid when the angular acceleration is constant.

The initial angular velocity (omega initial) is zero when the object starts from rest.

To solve rotational kinematic problems, identify three known variables and the one unknown, then use the appropriate formula to find the unknown.

The relationship between angular displacement (theta) and angular acceleration (alpha) is analogous to the relationship between linear displacement (x) and acceleration (a).

When solving problems, ensure that all variables are in consistent units, such as using radians for angular displacement and seconds for time.

The edge velocity of a rotating object can be calculated by multiplying the distance from the axis by the angular velocity.

The angular acceleration can be determined by using the appropriate kinematic formula and the known variables, even if the time is not given or needed.

The rotational kinematic formulas are a powerful tool for solving a wide range of problems involving rotational motion when the angular acceleration is constant.

Transcripts

Browse More Related Video

AP Physics 1 review of Torque and Angular momentum | Physics | Khan Academy

Rotational Kinematics Physics Problems, Basic Introduction, Equations & Formulas

College Physics 1: Lecture 23 - Rotational Motion

AP Physics 1 - Rotational Kinematics

Relating angular and regular motion variables | Physics | Khan Academy

Rotational Motion Physics, Basic Introduction, Angular Velocity & Tangential Acceleration

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsEducationRotationalMotionKinematicFormulasAngularDisplacementAngularVelocityAngularAccelerationConstantAccelerationProblemSolvingMechanicsEducationalContent