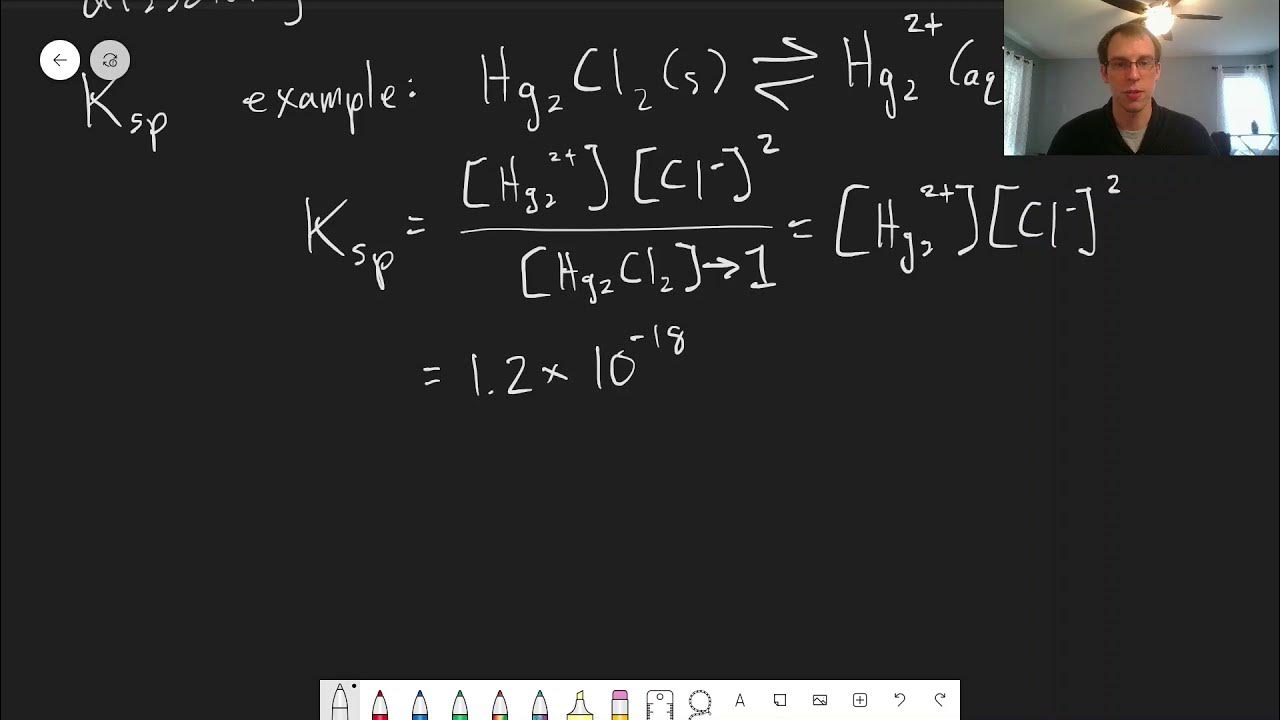Chapter 6: Precipitate Formation | CHM 214 | 056
TLDRThe video script discusses the concept of solubility product (Ksp) and its application in predicting the formation of precipitates in a solution. It uses the example of mercury chloride to illustrate how the addition of chloride ions to a mercury-containing solution can lead to precipitation. The script explains that when the ion product (Q) equals the solubility product (Ksp), solid begins to form. The calculation is demonstrated to determine the concentration of chloride ions at which precipitation starts, resulting in 8.9 x 10^-8 molar. This understanding is crucial for predicting chemical reactions in aqueous solutions.
Takeaways
- 🧪 The script discusses the use of solubility product (Ksp) in determining the formation of precipitates in a solution.
- 🌡️ The example given involves a solution containing mercury ions and the addition of chloride ions to it.
- 🔄 Ksp represents the equilibrium between the solid salt and its aqueous ion forms, which is crucial in understanding precipitate formation.
- 📈 The concentration of mercury is given as 1.5 × 10^(-4) molar, which is used to calculate the necessary chloride concentration for precipitate formation.
- 🔢 The Ksp value for the mercury-chloride system is 1.2 × 10^(-18), which is used in the calculations to find the critical chloride concentration.
- 📊 When the reaction quotient (Q) is less than Ksp, no solid forms, but when Q equals Ksp, precipitate begins to form as the reaction shifts to the left.
- 🧮 The calculation for the critical chloride concentration involves dividing the Ksp value by the mercury concentration and then taking the square root.
- 🌀 The stoichiometric coefficient of chloride in the reaction is considered, which is why its concentration is squared in the calculation.
- 📌 At a chloride concentration of 8.9 × 10^(-8) molar, precipitate formation begins, with mercury ions leaving the solution as chloride concentration increases.
- 📑 The process is an example of how chemical equilibrium concepts can be applied to predict the behavior of substances in solution.
Q & A
What is the significance of solubility product (Ksp) in the context of the given example?
-The solubility product (Ksp) represents the equilibrium constant for the dissolution of a solid salt into its constituent ions in a solution. It is crucial in determining the point at which a precipitate will start to form when additional ions are introduced into the solution.
In the example, why is it important to consider the absence of chloride ions initially?
-Considering the absence of chloride ions is important because it establishes that there is no solid salt form present initially. This sets the stage for understanding at what point the introduction of chloride ions will lead to the formation of a solid precipitate.
What does the concentration of mercury ions being 1.5 times 10 to the minus 4 molar indicate?
-A mercury ion concentration of 1.5 times 10 to the minus 4 molar indicates that the solution has a relatively low but significant amount of mercury ions present. This concentration is above the threshold where precipitate formation would be expected upon the addition of chloride ions.
How does the concept of equilibrium between solid salt and aqueous ions play a role in precipitate formation?
-The equilibrium between solid salt and its aqueous ions is represented by the Ksp value. When the product of the concentrations of the ions (Q) equals the Ksp, the reaction starts to shift towards the left, leading to the formation of the solid precipitate.
What is the stoichiometric coefficient, and why is it relevant in calculating the concentration of chloride ions?
-The stoichiometric coefficient represents the number of moles of a particular ion that react or are produced in a chemical reaction. It is relevant in calculating the concentration of chloride ions because it determines how the concentration of chloride ions must be squared to match the stoichiometry of the reaction.
What is the Ksp value given in the example, and what does it signify?
-The Ksp value given in the example is 1.2 times 10 to the minus 18. This value signifies the point at which the product of the concentrations of mercury and chloride ions will start to form a solid precipitate.
How does the quotient (Q) compare to Ksp in the context of precipitate formation?
-If Q is less than Ksp, no solid will form as the reaction proceeds to the right (more ions in solution). When Q equals Ksp, the reaction is at equilibrium and solid will start to form as the reaction begins to shift to the left (more solid salt form).
What is the calculated concentration of chloride ions that will lead to precipitate formation?
-The calculated concentration of chloride ions that will lead to precipitate formation is 8.9 times 10 to the minus 8 molar.
As the concentration of chloride ions increases beyond the point of precipitate formation, what happens to the amount of solid formed?
-As the concentration of chloride ions increases beyond the point where precipitate formation begins, more solid salt will form because the reaction continues to shift towards the left to re-establish equilibrium as more ions combine to form the solid.
How can the principles discussed in the script be applied to other similar chemical scenarios?
-The principles of solubility product (Ksp) and the equilibrium between solid and aqueous forms can be applied to other systems where precipitate formation is a concern. By identifying the Ksp value and understanding the stoichiometry of the reaction, one can predict and calculate the conditions under which precipitates will form in various chemical scenarios.
What is the unit of measurement for the concentrations discussed in the script?
-The unit of measurement for the concentrations discussed in the script is molarity (M), which expresses the concentration of a substance in terms of moles per liter of solution.
Outlines
🌟 Understanding Solubility Product and Precipitation
This paragraph discusses the application of the solubility product (Ksp) concept to predict the formation of precipitates in a solution. It uses the example of a mercury chloride solution to illustrate how the addition of chloride ions can lead to the formation of solid mercury chloride. The speaker explains that when the ion concentration product (Q) equals the Ksp, precipitation begins. The paragraph provides a step-by-step calculation to determine the concentration of chloride ions at which precipitation starts, using the given Ksp value and the concentration of mercury ions. The calculation involves setting up an equation based on the stoichiometry of the reaction and solving for the unknown chloride concentration, which is found to be 8.9 x 10^-8 Molar.
Mindmap
Keywords
💡Solubility Product (Ksp)
💡Mercury
💡Chloride
💡Precipitation
💡Concentration
💡Aqueous Solutions
💡Equilibrium
💡Reaction Quotient (Q)
💡Stoichiometric Coefficient
💡Molarity
💡Calculation
Highlights
The discussion revolves around the application of solubility product (Ksp) in understanding precipitate formation in solutions.
A hypothetical scenario is presented where mercury is in solution and the introduction of chloride is considered.
The concentration of mercury in the solution is given as 1.5 times 10 to the minus 4 molar.
The absence of chloride in the solution implies no solid prism formation.
The equilibrium between solid salt and aqueous forms of the ion is represented by Ksp.
Two chlorides are present, which is an important consideration in the calculations.
The concept of Q and Ksp is introduced, with Q being the reaction quotient used to predict the direction of the reaction.
Solid formation is expected when Q equals Ksp, indicating a shift in the reaction towards the left.
The Ksp value for the given problem is 1.2 times 10 to the minus 18.
A method to calculate the concentration of chloride needed for precipitate formation is described, involving the square of the chloride concentration.
The equation to find the chloride concentration (x) is outlined, which involves dividing Ksp by the concentration of mercury and then taking the square root.
The calculation results in a chloride concentration of 8.9 times 10 to the minus 8 molar for precipitate formation.
As the concentration of chloride increases beyond this point, more solid will form.
The process emphasizes the practical application of Ksp in predicting precipitate formation in chemical solutions.
The example demonstrates the use of stoichiometric coefficients in the calculation of ion concentrations.
The discussion provides a clear understanding of how ion concentrations interact and affect each other in a solution.
The explanation is didactic, making it accessible for learners to grasp the concept of solubility product and its applications.
The use of step-by-step calculations helps in understanding the relationship between Ksp, ion concentrations, and precipitate formation.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: