Impulse and Momentum Conservation - Inelastic & Elastic Collisions
TLDRThe video script delves into the concept of momentum, a fundamental principle in physics describing mass in motion. It explains momentum as the product of an object's mass and velocity, highlighting its vector nature with both magnitude and direction. The script further discusses the Impulse-Momentum Theorem, linking force, time, and changes in momentum. It also explores collisions, distinguishing between elastic and inelastic types, and the conservation of momentum versus kinetic energy. The video provides equations and examples to illustrate these concepts, emphasizing the importance of understanding momentum in various physical scenarios.
Takeaways
- 🌀 Momentum is the product of an object's mass and its velocity, and it is a vector quantity, meaning it has both magnitude and direction.
- 📈 The impulse-momentum theorem states that the impulse (force multiplied by time) acting on an object is equal to the change in the object's momentum.
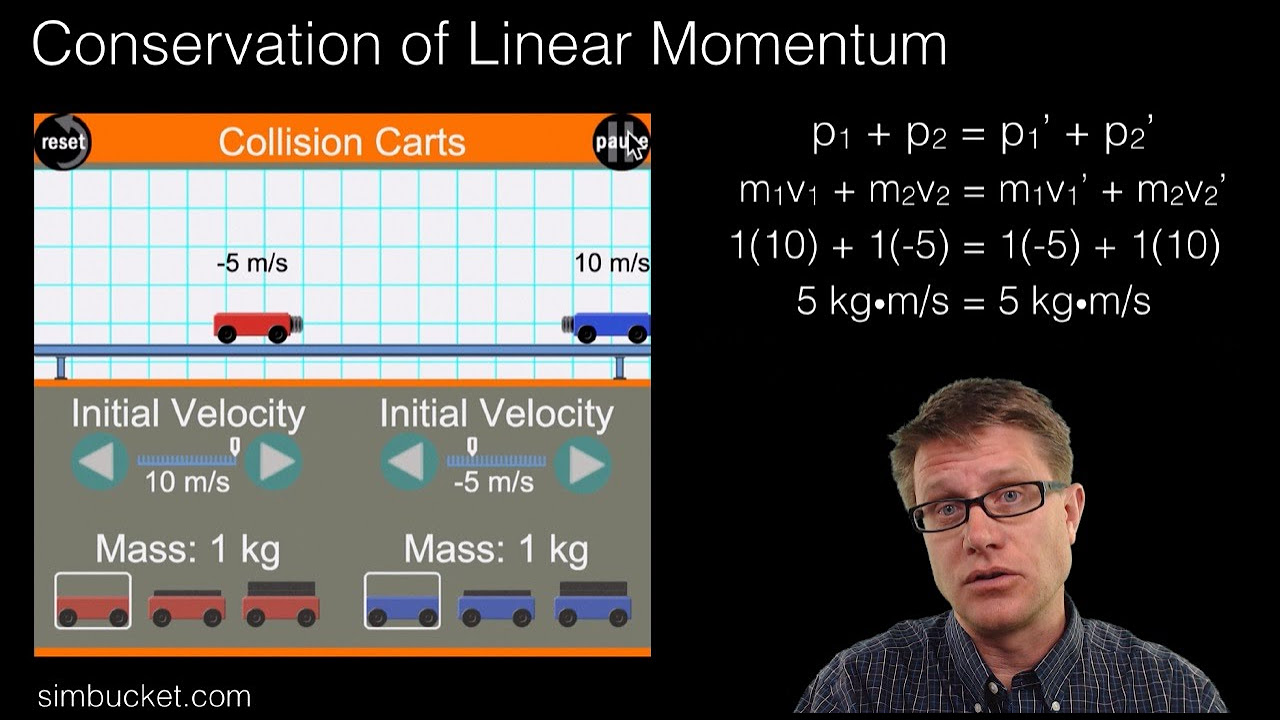
- 🔄 In collisions, whether elastic or inelastic, the total momentum of the system is conserved, though individual momentums of objects within the system may change.
- 🏐 For elastic collisions, both momentum and kinetic energy are conserved, while for inelastic collisions, kinetic energy is not conserved.
- 📊 The force acting on an object can be determined by calculating the derivative of the momentum function with respect to time.
- 🚗 When comparing two objects with different masses and velocities, the one with the greater product of mass and velocity has the greater momentum.
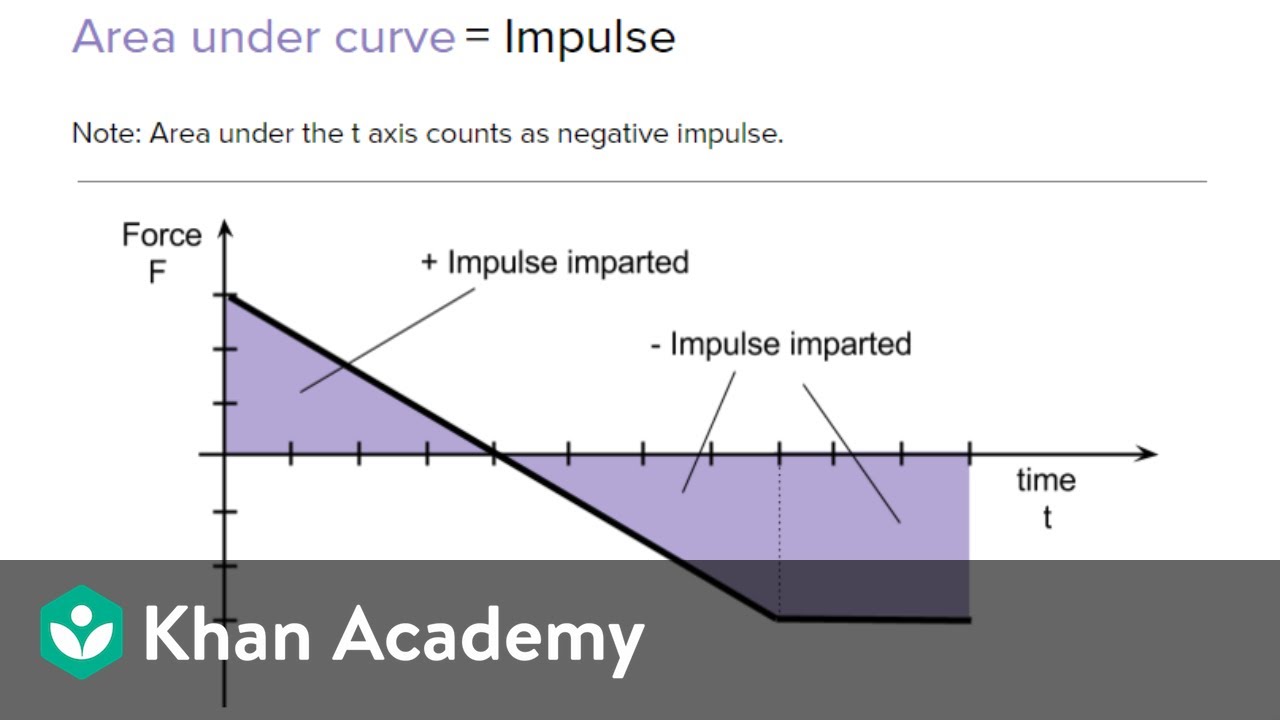
- 🛡 The impulse delivered to an object is equal to the change in its momentum, and can be calculated using the area under a force-time graph.
- 🎾 In calculating the momentum of a system, the individual momenta of all objects are vectorially added to find the net momentum.
- 🚀 The change in momentum of an object is directly proportional to the force applied and the time over which the force is applied.
- 📉 During free fall, the total momentum of a system of two colliding objects is not conserved due to the influence of gravity.
- 🔢 The conservation of momentum and kinetic energy principles are essential in solving problems involving collisions and forces.
Q & A
What is the definition of momentum?
-Momentum is the product of an object's mass and its velocity, representing the quantity of motion. It is a vector quantity, meaning it has both magnitude and direction.
How does momentum relate to velocity?
-Momentum is directly related to velocity as it is the product of mass and velocity. Since velocity is a vector, momentum also has both magnitude and direction, and the two are directly proportional.
What is the Impulse-Momentum Theorem and how is it applied?
-The Impulse-Momentum Theorem states that the impulse acting on an object is equal to the change in the object's momentum. It is used to calculate the effect of a force applied over a period of time on an object's momentum and can be represented by the equation: Impulse (F * Δt) = Change in Momentum (Δp).
How does momentum conservation apply to collisions?
-In collisions, whether elastic or inelastic, the total momentum of the system (all objects involved) is conserved. This means the sum of the momenta before the collision is equal to the sum of the momenta after the collision.
What is the difference between elastic and inelastic collisions in terms of kinetic energy?
-In an elastic collision, both momentum and kinetic energy are conserved. In an inelastic collision, momentum is conserved, but kinetic energy is not conserved, and some of it is transformed into other forms of energy, like heat or sound.
How can you calculate the total momentum of a system of objects?
-To calculate the total momentum of a system, you sum the individual momenta of all the objects in the system. The total momentum is the vector sum of the individual momentum vectors of each object.
What is the relationship between force, time, and impulse?
-Impulse is the product of force and the time over which it acts. A longer duration of force application or a greater force over a given time will result in a larger impulse, which in turn leads to a larger change in momentum of the object.
How can you determine the final velocity of an object after a force has been applied?
-To determine the final velocity of an object after a force has been applied, you can use the impulse-momentum theorem. Knowing the impulse (force multiplied by time) and the mass of the object, you can calculate the change in momentum and then find the final velocity by dividing the final momentum by the object's mass.
What happens to the momentum of an object when an external force acts on it?
-When an external force acts on an object, it can change the object's momentum. The change in momentum is equal to the impulse applied to the object, which is the product of the force and the time over which it acts.
How does gravity affect the conservation of momentum in a free-falling system of two colliding objects?
-In a free-falling system of two colliding objects, the total momentum is not conserved because gravity, an external force, acts on the objects, adding a vertical component to their momentum. This external force increases the overall momentum of the system by imparting a downward momentum component to each object.
Outlines
🚀 Introduction to Momentum
This paragraph introduces the concept of momentum, explaining it as mass in motion. It describes momentum as a vector quantity, having both magnitude and direction, represented by the symbol 'p'. The relationship between mass, velocity, and momentum is discussed through examples involving a three-kilogram ball and a five-kilogram ball, highlighting the vector nature of momentum. The paragraph also connects momentum to Newton's second law and the impulse-momentum theorem, emphasizing the importance of understanding these concepts for further physics study.
📚 Impulse and Momentum in Physics
This section delves deeper into the relationship between impulse and momentum, especially in the context of varying forces over time. It explains that the impulse, represented by 'I', is the product of force and time (F∆t) and is equal to the change in momentum (∆p). The concept is extended to include calculus for physics students, explaining that impulse is the integral of force with respect to time. The paragraph also touches on the derivative relationship between force and the momentum function, and how this relates to the rate of change of momentum. The discussion sets the stage for understanding collisions in terms of momentum conservation.
💥 Elastic and Inelastic Collisions
This paragraph focuses on the conservation of momentum and kinetic energy in elastic and inelastic collisions. It clarifies that while momentum is always conserved in any type of collision, kinetic energy is only conserved in elastic collisions. The difference between inelastic and completely inelastic collisions is also discussed, noting that the latter results in the maximum loss of kinetic energy. The paragraph provides a practical framework for identifying the type of collision based on the conservation of kinetic energy and concludes with equations essential for solving collision problems.
🔢 Equations for Collision Problems
This section presents the fundamental equations used to solve problems involving collisions. It emphasizes the conservation of momentum in isolated systems and introduces the concept of contact forces and reaction forces. The equations are detailed for both inelastic and elastic collisions, highlighting the conditions under which they apply. The paragraph also explains the importance of understanding the internal and external forces in collision scenarios and how they affect the conservation of momentum.
📝 Practice Problems on Momentum
This paragraph presents a series of practice problems to apply the concepts of momentum and impulse. It covers various scenarios, including comparing momentum between different objects, calculating the total momentum of a system, and determining the magnitude and direction of net momentum. The problems are designed to reinforce the understanding of momentum conservation and impulse, and to develop problem-solving skills in the context of physics.
🚗 Impulse and Momentum in Real-World Scenarios
This section explores the application of impulse and momentum in real-world situations, such as the impact of a force on an object over time. It includes examples of calculating the change in momentum when a force is applied, the impulse exerted on an object, and the final momentum and velocity of an object after a collision. The paragraph emphasizes the practical implications of these calculations and their importance in understanding the dynamics of moving objects.
🏌️♂️ Analyzing Force and Contact Time
This paragraph discusses the analysis of force and contact time, particularly in the context of a golf club striking a golf ball. It uses the impulse-momentum theorem to calculate the contact time between the club and the ball, and the average force exerted during the impact. The section provides a clear methodology for solving such problems and illustrates the interplay between force, time, and momentum in dynamic scenarios.
🏐 Calculating Force and Momentum in Sports
This section focuses on calculating the force exerted by a baseball bat on a ball, and the resulting change in the ball's momentum. It provides a detailed explanation of how to use the impulse-momentum theorem to determine the average force and the change in momentum when a ball's direction and speed change upon impact. The paragraph highlights the significant forces involved in sports and the rapid changes in momentum that occur during play.
📈 Impulse and Momentum in Graphical Analysis
This paragraph examines the graphical representation of force over time and how it relates to impulse and momentum. It describes how to calculate the impulse imparted to an object by finding the area under a force-time curve and how this impulse corresponds to the change in the object's momentum. The section provides a method for breaking down complex force-time graphs into simpler shapes to facilitate these calculations.
🤔 Evaluating Statements on Impulse and Momentum
This paragraph involves evaluating statements related to impulse and momentum, discerning truth from falsehood. It covers the equivalence of impulse and change in momentum, the dimensional equivalence of momentum and impulse, and the conditions under which momentum conservation applies. The section clarifies misconceptions and reinforces the principles of momentum and impulse with examples and explanations.
🌌 Conservation of Momentum in Free Fall
The final paragraph discusses the conservation of momentum in a non-isolated system, specifically during free fall. It explains why the total momentum of a system of two colliding objects is not conserved when external forces, such as gravity, are acting on them. The paragraph provides a clear explanation of how gravity affects the momentum of objects and how this leads to an increase in the total momentum of the system, thus challenging the notion of momentum conservation in such scenarios.
Mindmap
Keywords
💡Momentum
💡Mass
💡Velocity
💡Vector
💡Impulse
💡Impulse-Momentum Theorem
💡Force
💡Acceleration
💡Collision
💡Conservation of Momentum
Highlights
Momentum is defined as mass in motion, represented by the symbol p and calculated as mass times velocity.
Momentum is a vector quantity, which means it has both magnitude and direction.
The momentum of an object can be calculated with the formula p = m*v, where p is momentum, m is mass, and v is velocity.
According to Newton's second law, the net force acting on an object is equal to the mass times the acceleration (F = ma).
Impulse is defined as the product of force and time (F*Δt) and is related to the change in momentum of an object.
The impulse-momentum theorem states that the impulse acting on an object is equal to the change in the object's momentum (Impulse = Δp).
In an elastic collision, both momentum and kinetic energy are conserved.
In an inelastic collision, momentum is conserved, but kinetic energy is not conserved.
A completely inelastic collision results in the maximum loss of kinetic energy.
The conservation of momentum can be used to solve for unknown variables in collision problems using the equation Δp = 0.
For elastic collisions, the equations for conservation of momentum and kinetic energy can be combined to solve problems with missing variables.
The force acting on an object can be determined by differentiating the momentum function with respect to time.
In practice, the area under a force-time graph represents the impulse exerted on an object.
The total momentum of an isolated system remains constant during collisions, with the internal forces between colliding objects being equal and opposite.
The change in momentum for objects in a collision is equal in magnitude but opposite in direction, according to Newton's third law.
The conservation of momentum principle is widely applied in physics to analyze collisions and the effects of external forces on objects.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: