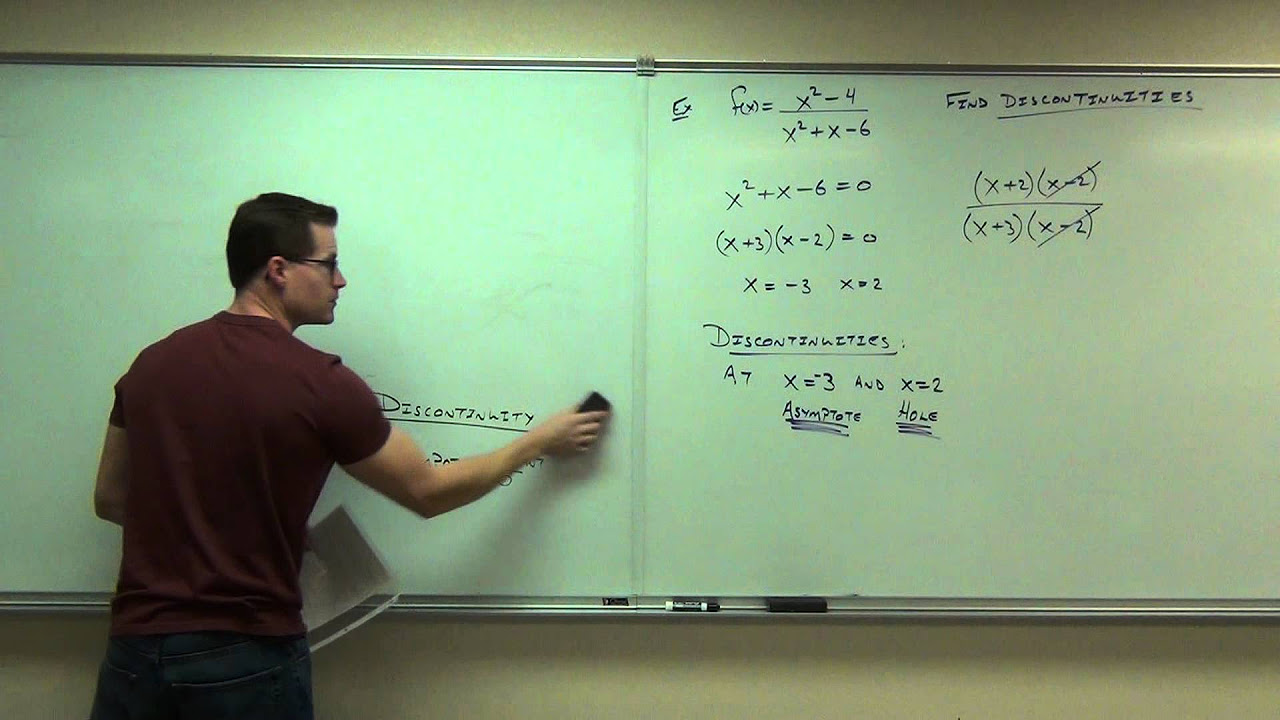Proof: Differentiability implies continuity | Derivative rules | AP Calculus AB | Khan Academy
TLDRThe video script presents a mathematical proof demonstrating the relationship between differentiability and continuity of functions. It begins by defining differentiability as the existence of a limit for the slope of the tangent line at a point C, and continuity as the limit of the function value approaching that point being equal to the function value at that point. Through illustrative examples, the script shows how discontinuities manifest in functions and contrasts them with continuous functions. The proof then leverages the assumption of differentiability at a point to show that the limit of the function minus its value at that point equals zero, thereby establishing that a differentiable function at a point C is also continuous at that point.
Takeaways
- 📌 The main goal of the video is to demonstrate that differentiability of a function at a point C implies continuity at that point.
- 📈 Differentiability is defined by the existence of a limit as X approaches C, where the limit represents the slope of the tangent line at point C.
- 🔍 The process of finding the derivative involves considering a secant line and finding its slope, then taking the limit as X approaches C.
- 🌐 The concept of continuity is introduced as a function being continuous at point C if the limit of the function as X approaches C equals the function's value at C.
- 🚫 Examples of discontinuity, such as point and jump discontinuities, are provided to contrast with continuous functions.
- 📊 The video uses visual examples to illustrate the concepts of differentiability and continuity, emphasizing the importance of understanding these concepts through visualization.
- 🧠 A review of the definitions and properties of limits is provided to establish a solid foundation for understanding differentiability and continuity.
- 🔧 The proof that differentiability implies continuity involves manipulating the limit of the difference quotient and applying properties of limits.
- 🎯 The proof concludes by showing that if a function is differentiable at point C, the limit of the function as X approaches C must equal the function's value at C, confirming continuity.
- 📚 The video script serves as a comprehensive review of the fundamental concepts of differentiability and continuity in calculus, suitable for learners at various levels.
- 💡 The key takeaway is that differentiability is a stronger condition than continuity; a function that is differentiable at a point is also continuous at that point, but not all continuous functions are differentiable.
Q & A
What is the main goal of the video?
-The main goal of the video is to prove that if a function is differentiable at some point C, then it is also continuous at that point C.
How is differentiability defined in the context of the video?
-Differentiability is defined as the existence of a limit as X approaches C of the ratio of the change in Y to the change in X, which is also referred to as the slope of the tangent line at point C.
What is the significance of the slope of the tangent line in the context of differentiability?
-The slope of the tangent line represents the derivative at point C and is a key concept in differentiability. If this limit exists, the function is considered differentiable at point C.
What does it mean for a function to be continuous?
-A function is considered continuous at a point C if the limit as X approaches C of the function F of X is equal to F of C. In other words, the function's value at point C matches the limit of the function as X approaches C.
How does the video illustrate the concept of continuity?
-The video illustrates the concept of continuity by showing that for a continuous function, the limit of F of X as X approaches C from either side equals F of C, indicating no break or jump in the function's value at point C.
What is a point discontinuity and how does it relate to continuity?
-A point discontinuity occurs when there is a gap in the function at point C, causing the limit as X approaches C of F of X to be different from F of C. This indicates that the function is not continuous at point C.
What is a jump discontinuity and how does it differ from a point discontinuity?
-A jump discontinuity occurs when the function has different limit values as X approaches C from the left and right sides. Unlike a point discontinuity, a jump discontinuity does not have a defined value at C, and the limit does not exist, making the function discontinuous at that point.
How does the video prove that differentiability implies continuity?
-The video proves that differentiability implies continuity by assuming the function is differentiable at point C, which means the derivative exists at C. It then shows that the limit of (F of X minus F of C) over X minus C as X approaches C is zero. Using limit properties, it concludes that the limit of F of X as X approaches C equals F of C, which is the definition of continuity.
What is the relationship between the derivative and the slope of the secant line?
-The derivative at a point C is the slope of the tangent line at that point, whereas the slope of the secant line is an approximation of the derivative based on two points on the function. As the points get closer together, the secant line's slope approaches the slope of the tangent line, which is the derivative.
How can you determine if a function is not continuous?
-You can determine if a function is not continuous by looking for points where the limit as X approaches C of F of X does not equal F of C. Discontinuities, such as point or jump discontinuities, indicate that the function is not continuous at those points.
What is the significance of the limit in both differentiability and continuity?
-The limit is crucial in both differentiability and continuity as it provides a way to quantify the behavior of a function at a certain point. For differentiability, the limit of the ratio of changes must exist, and for continuity, the limit of the function as X approaches a point must equal the function's value at that point.
Outlines
📚 Introduction to Differentiability and Continuity
This paragraph introduces the main topic of the video, which is to demonstrate that if a function is differentiable at a point C, it is also continuous at that point. The voiceover begins by revisiting the concepts of differentiability and continuity, explaining that differentiability is related to the existence of a derivative at a certain point, represented by the slope of the tangent line to the function's graph at that point. The concept of continuity is also reviewed, with the definition provided that a function is continuous at a point C if the limit of the function as X approaches C is equal to the function's value at C. The paragraph sets the stage for the upcoming proof by providing a foundational understanding of these two mathematical concepts.
📉 Visualizing Discontinuities and Continuity
In this paragraph, the video script delves into visual representations of discontinuities and continuity to help the viewer understand the concepts more intuitively. It discusses the two types of discontinuities: point discontinuities, where there is a gap in the graph at point C, and jump discontinuities, where the function approaches different values from the left and right sides of point C. The paragraph contrasts these with a truly continuous function, where the limit of the function as X approaches C is equal to the function's value at C. This visual comparison reinforces the definitions and prepares the viewer for the proof that will follow.
🔢 Proof that Differentiability Implies Continuity
The final paragraph presents the proof that differentiability at a point C implies continuity at that point. It begins by setting up the limit expression for the difference of the function's value at X and at C, and then manipulates this expression by factoring in the difference of X and C. Assuming the function is differentiable at C, the derivative (F prime of C) is used to simplify the limit, which ultimately evaluates to zero. This result is then used to show that the limit of the function as X approaches C is equal to the function's value at C, satisfying the definition of continuity. The paragraph concludes by reinforcing the main takeaway: if a function has a derivative at a point, it is also continuous at that point.
Mindmap
Keywords
💡Differentiability
💡Continuity
💡Limit
💡Secant Line
💡Tangent Line
💡Derivative
💡Slope
💡Graph
💡Function
💡Point Discontinuity
💡Jump Discontinuity
Highlights
The video aims to prove that differentiable functions are also continuous at the point of differentiability.
Differentiability is defined by the existence of a limit as X approaches a point C, where the secant line's slope approaches the tangent line's slope.
The slope of the tangent line at a point is represented by the derivative, denoted as F prime of C.
Continuity is defined by the limit of the function F of X as X approaches C being equal to F of C.
A function with a point discontinuity at C will not be continuous, as the limit as X approaches C does not equal F of C.
In the case of a jump discontinuity, the limit as X approaches C does not exist, indicating non-continuity.
A truly continuous function will have the limit as X approaches C of F of X equal to F of C from both sides.
The proof of differentiability implying continuity starts with the assumption that F is differentiable at C.
The limit of the difference (F of X minus F of C) as X approaches C is used to demonstrate continuity.
By manipulating the limit expression and applying limit properties, the proof shows that the limit difference is zero.
If the derivative exists at a point C, the limit of the function F of X as X approaches C minus F of C is zero.
Adding F of C to both sides of the equation derived from the limit properties confirms the function's continuity at point C.
The proof concludes that if a function is differentiable at a point C, it is also continuous at that point.
The video provides a comprehensive review of both differentiability and continuity before presenting the proof.
The visual examples of discontinuities help to clarify the concepts and definitions.
The proof is structured to logically lead the viewer from the assumption of differentiability to the conclusion of continuity.
The use of limit properties is crucial in demonstrating the relationship between differentiability and continuity.
The video's content is valuable for understanding the fundamental concepts of calculus and their interconnections.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: