Worked example: problem involving definite integral (algebraic) | AP Calculus AB | Khan Academy
TLDRThe video script discusses the mathematical concept of population growth modeled by an exponential function. It presents a scenario where a town's population increases at a rate of e^(1.2t) - 2t people per year. The instructor guides through solving two problems: the population growth from year 2 to 5, and the exact population at year 5. Using calculus, specifically the definite integral, the script explains how to find the area under the rate curve to determine the change in population. The solution involves finding the antiderivative of the given function and evaluating it at the given time intervals. The final answer is an approximate population growth of 306 people from year 2 to 5, resulting in a total population of 1,806 at year 5.
Takeaways
- 📈 The town's population grows according to the function e^(1.2t) - 2t, where t represents the number of years.
- 🏠 At t equals two years, the population of the town is 1,500 people.
- 📊 The growth in population between t equals two and t equals five can be calculated by finding the area under the rate curve for that time period.
- 🧮 To find the population growth, integrate the given function from t=2 to t=5, which is ∫(e^(1.2t) - 2t) dt from 2 to 5.
- 🔄 The antiderivative of the function is (5/6)e^(6/5t) - t^2 + C, using u-substitution for the integration.
- 📌 At t=5, the antiderivative evaluates to (5/6)e^6 - 25, and at t=2, it evaluates to (5/6)e^(2.4) - 4.
- ➖ To find the growth, subtract the value at t=2 from the value at t=5, which gives the area under the curve.
- 🔢 The calculation results in an approximate population growth of 306 people between t=2 and t=5.
- 🎉 The town's population at t equals five years is approximately 1,806 people, derived by adding the growth to the initial population at t=2.
- 👉 The key to solving the problem is understanding the concept of rates and using definite integrals to find the change in population over time.
- 📚 This problem demonstrates the application of calculus in modeling and solving real-world scenarios such as population growth.
Q & A
What is the population growth rate of the town?
-The population growth rate of the town is given by the function e to the power of 1.2t minus two t people per year, where t represents the number of years.
How many people did the town have at t equals two years?
-At t equals two years, the town had 1,500 people.
What is the formula to calculate the change in population between two time intervals?
-The change in population between two time intervals can be calculated by finding the area under the rate curve, which is expressed as the definite integral from t equals two to t equals five of the expression e to the 1.2t minus two t dt.
What is the antiderivative of e to the 1.2t?
-The antiderivative of e to the 1.2t is 5/6 times e to the 6/5 t, plus a constant (c), which is used in the indefinite integral.
How can we find the antiderivative of two t?
-The antiderivative of two t is t squared, as it represents the integral of the function with respect to time.
What is the approximate population growth between t equals two and t equals five?
-The approximate population growth between t equals two and t equals five is about 306 people.
What is the town's population at t equals five years?
-The town's population at t equals five years is approximately 1,806 people.
How does the rate curve relate to the population growth?
-The rate curve shows how the rate of change of the population, which is the growth rate, changes as a function of time. It helps to understand the dynamics of the population growth over different time intervals.
What is the significance of the definite integral in this context?
-The definite integral is used to find the accumulated change in population over the given time period (from t equals two to t equals five). It represents the area under the rate curve, which corresponds to the total growth in population during that interval.
How does the concept of u-substitution help in finding the antiderivative?
-U-substitution is a method used in calculus to evaluate integrals by changing the variable of integration. In this context, it helps to simplify the expression and find the antiderivative of the given function more efficiently.
What is the role of constants in the process of integration?
-Constants can be taken in and out of the integral, which is useful when applying methods like u-substitution or when simplifying the antiderivative expression. This property allows for easier computation and manipulation of the integral.
Outlines
📈 Population Growth Analysis
The first paragraph introduces a mathematical problem concerning the population growth of a town. The population is described as growing at a rate of e^(1.2t) - 2t people per year, where t represents the number of years. The problem sets a specific scenario where at t=2 years, the town has a population of 1,500 people. The challenge is to calculate the approximate population growth between t=2 and t=5 years and the population at t=5 years. The explanation begins by emphasizing the importance of understanding the rate of growth represented by the given formula and reviews the concept of a rate curve. It then transitions into a discussion on how to calculate the change in population by finding the area under the rate curve between two times, using the definite integral of the given expression from t=2 to t=5.
🧮 Solution and Calculation
The second paragraph delves into the solution of the problem by working out the antiderivative of the given population growth function. It explains the process of finding the antiderivative of e^(1.2t) and 2t separately, using u-substitution for the exponential part and basic integration for the linear term. The paragraph then evaluates the antiderivative at t=5 and t=2 to determine the change in population over the three-year period. After performing the necessary calculations, it concludes that the population grows by approximately 306 people between t=2 and t=5 years. Finally, by adding this growth to the initial population of 1,500 at t=2 years, the paragraph reveals that the town's population will be approximately 1,806 people at t=5 years.
Mindmap
Keywords
💡population growth
💡exponential function
💡rate of change
💡integral
💡antiderivative
💡u-substitution
💡rate curve
💡definite integral
💡t-squared
💡calculus
💡area under the curve
Highlights
The town's population grows according to a mathematical model involving exponential functions.
The growth rate is described by the function e to the 1.2t power minus two t people per year.
At t equals two years, the town's population is confirmed to be 1,500 people.
The task involves calculating the population growth between t equals two and t equals five years.
The solution requires understanding the concept of a rate curve in calculus.
The rate curve illustrates how the rate of population change varies as a function of time.
The area under the rate curve between two specific times represents the change in population.
The problem can be solved using the definite integral of the given function from t equals two to t equals five.
The antiderivative of e to the 1.2t is calculated using substitution methods.
The antiderivative of two t is found to be t squared.
The population growth is determined by evaluating the antiderivative at t equals five and t equals two.
The calculation involves finding the difference between the evaluated antiderivative results at the two times.
The approximate population growth between t equals two and t equals five is 306 people.
The town's population at t equals five years is calculated to be 1,806 people.
The process demonstrates the practical application of calculus in modeling and predicting population dynamics.
The method used can be applied to other similar growth and decay problems in various fields.
The use of technology, such as a calculator, is integral to solving complex mathematical problems efficiently.
Transcripts
Browse More Related Video
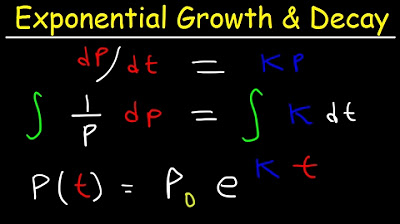
Exponential Growth and Decay Calculus, Relative Growth Rate, Differential Equations, Word Problems
Math 11 - Section 2.4 (previously section 3.3)

Logistic growth versus exponential growth | Ecology | AP Biology | Khan Academy
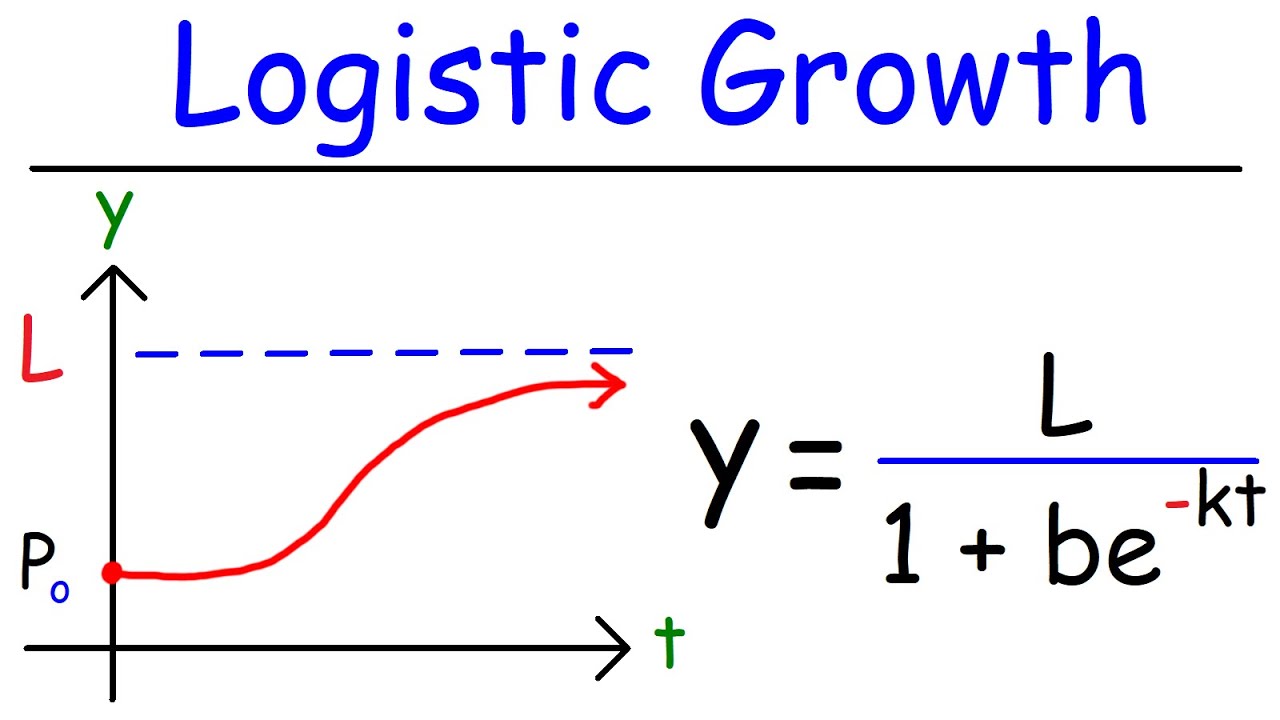
Logistic Growth Function and Differential Equations

Compound Interest & Population Growth Word Problems - Logarithms

Integrals, Population, and Radial Density Functions
5.0 / 5 (0 votes)
Thanks for rating: