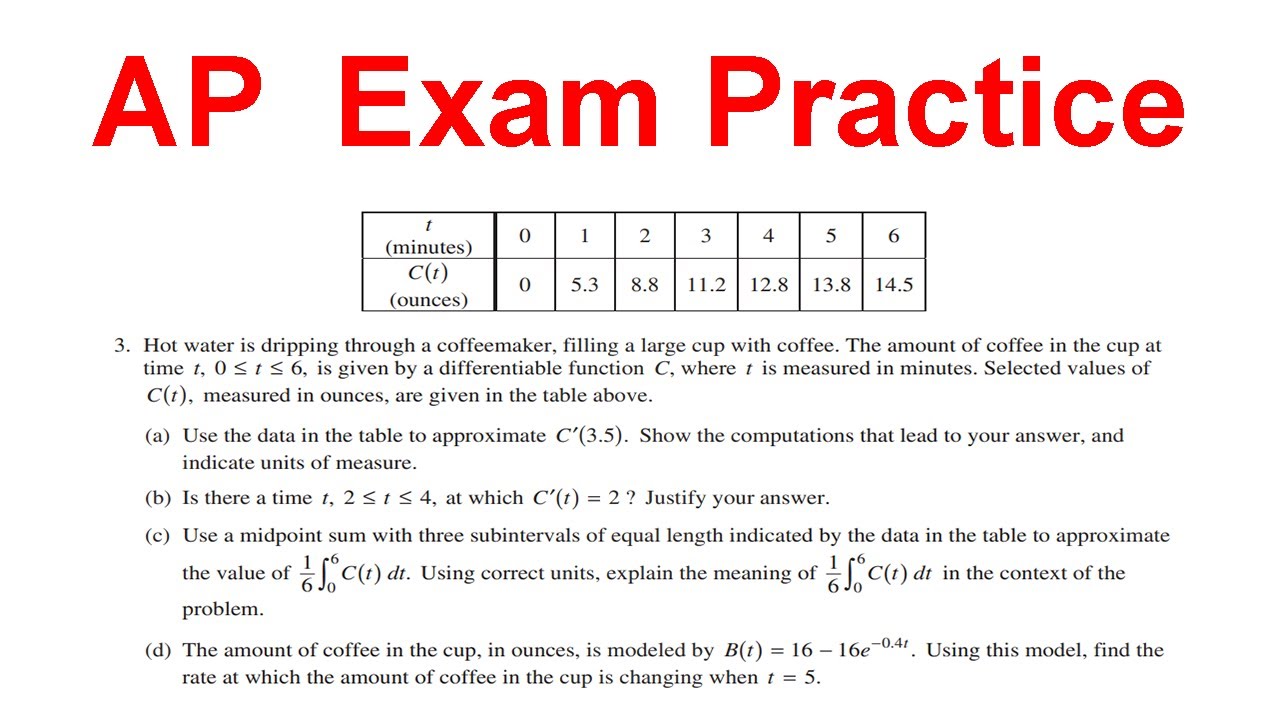
2012 AP Calculus AB Free Response #1

Allen Tsao The STEM Coach
14 Oct 201809:11
EducationalLearning
32 Likes 10 Comments

TLDRIn this engaging video, Alan from Bottle Stem Coach dives into the AP Calculus AB exam, specifically tackling the 2012 free response questions. He begins by using a GeoGebra calculator to estimate the derivative of a function modeling the temperature of water in a tub over time. Alan calculates the rate of temperature change at a specific time and interprets its meaning within the context of the problem. He then uses the left Riemann sum to approximate the average temperature of the water over a 20-minute period, explaining why this method might underestimate the true average due to the function's increasing nature. Finally, Alan addresses a question about the temperature at a later time by integrating the rate of change function and adding it to the initial temperature. The video is a valuable resource for those preparing for AP Calculus exams, offering clear explanations and step-by-step solutions to complex problems.

Takeaways
  • 📈 The temperature of water in a tub is modeled by a strictly increasing, twice differentiable function W(T), where W is in degrees Fahrenheit and T is in minutes.
  • ⏱️ At time T=0, the water temperature is 55 degrees Fahrenheit, and it is heated for 30 minutes.
  • 📊 To estimate the derivative of W with respect to T at T=12, use the secant line slope between T=9 and T=15, resulting in an approximate slope of 1.017 degrees F per minute.
  • 🧮 By applying the fundamental theorem of calculus, the total increase in water temperature from T=0 to T=20 is 16 degrees Fahrenheit.
  • 🌡️ The average temperature of the water over 20 minutes is calculated using the left Riemann sum with four subintervals, yielding an average temperature of 60.79 degrees Fahrenheit.
  • ⬇️ The left Riemann sum provides an underestimate of the average temperature because the function is increasing, and the left endpoint is always lower than the right.
  • 🔢 To find the water temperature at T=25, integrate the first derivative of the function from T=20 to T=25 and add it to the temperature at T=20, resulting in approximately 73.043 degrees Fahrenheit.
  • 📚 The video uses GeoGebra calculator for graphing and visual representation of mathematical functions and data.
  • 🤔 The presenter emphasizes the importance of understanding the context of the problem when interpreting the meaning of derivatives and integrals in the problem.
  • 📐 The secant line method is used for estimating derivatives when exact function values are not available at specific points.
  • 📉 The area under the derivative curve represents the change in the function, which in this context is the change in water temperature over time.
  • 🔁 The presenter also discusses the implications of using different Riemann sums (left, right, midpoint) on the accuracy of the approximation, especially for increasing functions.
Q & A

  • What is the subject of the video Alan is discussing?

    -Alan is discussing the AP Calculus AB exam from 2012, specifically focusing on response questions.

  • What is the context of the water temperature model in the video?

    -The water temperature in a tub is modeled by a strictly increasing, twice differentiable function W(T), where W is measured in degrees Fahrenheit and T is measured in minutes.

  • At what temperature does the water start heating?

    -The water starts heating at an initial temperature of fifty-five degrees Fahrenheit.

  • How is the derivative of W with respect to T at T=12 estimated in the video?

    -The derivative is estimated by calculating the secant line slope between T=9 and T=15, as T=12 lies between these two values.

  • What is the approximate value of the derivative of W at T=12?

    -The approximate value of the derivative of W at T=12 is 1.017 degrees Fahrenheit per minute.

  • How is the total change in water temperature from T=0 to T=20 found?

    -The total change in water temperature is found by evaluating the antiderivative of the derivative function and applying the Fundamental Theorem of Calculus, which results in a change of 16 degrees Fahrenheit.

  • What is the average temperature of the water over the 20-minute period?

    -The average temperature is approximated using the left Riemann sum with four subintervals, resulting in an average temperature of 60.79 degrees Fahrenheit.

  • Why is the left Riemann sum considered an underestimate for the average temperature?

    -The left Riemann sum is an underestimate because it consistently uses the smaller left endpoint value for each subinterval, especially in the context of an increasing function.

  • What is the temperature of the water at T=25, according to the model?

    -The temperature at T=25 is found by integrating the first derivative of the model from T=20 to T=25 and adding it to the temperature at T=20, which results in approximately 73.043 degrees Fahrenheit.

  • What is the significance of the units in the calculation of the average temperature?

    -The units are important as they ensure the calculation reflects the correct physical quantity; in this case, the average temperature is expressed in degrees Fahrenheit.

  • What is the role of the secant line in estimating derivatives in the context of the video?

    -The secant line is used to estimate the instantaneous rate of change, or the derivative, at a point that lies between two given data points by calculating the slope of the line passing through those points.

  • How does Alan suggest viewers can get more help with their calculus homework?

    -Alan offers free homework help on platforms like Twitch and Discord, and encourages viewers to engage with him there for further assistance.

Outlines
00:00
📊 AP Calculus AB 2012 Free Response Question Analysis

In this segment, Alan introduces the AP Calculus AB 2012 free response questions. He uses GeoGebra to graph and analyze the temperature of water in a tub over time, modeled by a strictly increasing, twice differentiable function W(T), where W is in degrees Fahrenheit and T is in minutes. Starting with T=0 at 55 degrees Fahrenheit, the water is heated for 30 minutes. Alan estimates the derivative of W at T=12 by calculating the secant line slope between T=9 and T=15, resulting in approximately 1.017 degrees Fahrenheit per minute. He then calculates the total temperature change over 20 minutes using the antiderivative and the fundamental theorem of calculus, finding a 16-degree Fahrenheit increase. Alan also discusses the average temperature over 20 minutes using the left Riemann sum with four subintervals, concluding that the approximation underestimates the average temperature due to the function's increasing nature. The segment ends with a brief mention of the next steps in the video series.

05:02
🔢 Estimating the Water Temperature at T=25 and the Average Temperature Over 20 Minutes

Alan continues the AP Calculus AB 2012 free response analysis by addressing the temperature of the water at T=25. He integrates the first derivative of the water temperature function from T=20 to T=25 and adds the initial temperature at T=20 to find the temperature at T=25, which is approximately 73.043 degrees Fahrenheit. He also discusses the use of trigonometric functions in radians and the importance of setting the correct unit for the calculations. Alan then evaluates the average temperature over the 20-minute period, noting that the left Riemann sum underestimates the actual average due to the function's increasing trend. The paragraph concludes with a summary of the findings and an invitation for viewers to engage with the content through likes, comments, and subscriptions, and to seek further assistance through offered platforms like Twitch and Discord.

Mindmap
Keywords
💡AP Calculus
AP Calculus is a high school mathematics course that covers topics in calculus, which is a branch of mathematics that deals with rates of change and accumulation. In the video, Alan is discussing the AP Calculus AB exam, which is a standardized test that high school students can take to demonstrate their knowledge of calculus. The theme of the video revolves around solving problems from the 2012 AP Calculus AB free response section.
💡Graphing Calculator
A graphing calculator is a type of electronic device that is used to perform mathematical calculations and plot graphs of functions. In the context of the video, Alan mentions using a GeoGebra calculator, which is a software tool that can be used to visualize mathematical concepts. The graphing calculator is essential for solving the first two questions of the AP Calculus exam, which involve graphing and analyzing mathematical functions.
💡Twice Differentiable Function
A twice differentiable function is a mathematical function that has two derivatives, meaning it has a well-defined rate of change and its rate of change is also changing at a well-defined rate. In the video, Alan discusses a function W(T) that models the temperature of water in a tub as a function of time T. The function being twice differentiable is important because it allows for the calculation of the rate of change (derivative) and the rate at which that rate of change is changing (second derivative).
💡Derivative
The derivative of a function is a measure of the rate at which the function's value changes with respect to its variable. In the video, Alan calculates the derivative of the function W(T) with respect to time T at T=12 minutes by estimating the slope of the secant line between the points (9, W(9)) and (15, W(15)). The derivative in this context is used to determine how quickly the temperature of the water is changing at a specific time.
💡Secant Line
A secant line is a straight line that intersects a curve at two or more points. In the video, Alan uses the concept of a secant line to estimate the derivative of the function W(T) at T=12 by finding the slope of the line between the points at T=9 and T=15. The secant line is a method to approximate the rate of change of a function at a point where the function is not explicitly differentiable.
💡Antiderivative
An antiderivative, also known as an integral, is a function whose derivative is equal to the original function. In the video, Alan uses the concept of antiderivatives to find the change in temperature of the water from T=0 to T=20 by integrating the derivative function and applying the Fundamental Theorem of Calculus, which states that the definite integral of a function can be found by evaluating its antiderivative at the limits of integration.
💡Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus is a foundational theorem that links the concept of differentiation and integration. It states that the definite integral of a function can be computed by finding the antiderivative of the function and evaluating it at the limits of integration. In the video, Alan applies this theorem to calculate the total change in temperature of the water over a 20-minute period.
💡Riemann Sum
A Riemann sum is a method used to approximate the definite integral of a function. It involves dividing the area under the curve of the function into rectangles and summing their areas. In the video, Alan uses the left Riemann sum to approximate the average temperature of the water over a 20-minute period by dividing the interval into subintervals and using the left endpoint of each subinterval to estimate the area of the rectangles.
💡Average Temperature
The average temperature in the context of the video refers to the mean value of the temperature of the water over a given period of time, which is 20 minutes in this case. Alan calculates this by using the left Riemann sum to approximate the total area under the curve of the temperature function and then dividing by the width of the interval (20 minutes) to find the average value.
💡Underestimate
An underestimate occurs when a calculated or approximated value is less than the actual value. In the video, Alan explains that using the left Riemann sum to approximate the average temperature of the water results in an underestimate because the function is increasing, and the left endpoint of each subinterval represents a lower value than the midpoint would.
💡Integration
Integration is the mathematical process of finding an antiderivative or the area under the curve of a function. In the video, Alan performs integration to find the temperature of the water at T=25 by integrating the rate of change function from T=20 to T=25 and adding this to the known temperature at T=20. This process allows him to determine the accumulated change in temperature over the interval.
Highlights

Introduction to AP Calculus AB 2012 free response questions by Alan with Bottle Stem.

Use of GeoGebra calculator for graphing calculator questions.

Modeling the temperature of water in a tub as a strictly increasing, twice differentiable function W(T).

Initial water temperature at time T=0 is 55 degrees Fahrenheit.

Water is heated for 30 minutes from T=0.

Estimating the derivative of W with respect to T at T=12 using secant line slope between T=9 and T=15.

Calculating the secant line slope as approximately 1.017 degrees Fahrenheit per minute.

Interpreting the derivative as the rate of temperature change over time.

Using the fundamental theorem of calculus to find the total temperature change from T=0 to T=20.

The water temperature increases by 16 degrees Fahrenheit over 20 minutes.

Using the left Riemann sum with four subintervals to approximate the average temperature of water over 20 minutes.

The approximation underestimates the average temperature due to the strictly increasing nature of the function.

Calculating the average temperature as 60.79 degrees Fahrenheit.

Determining the temperature of the water at T=25 by integrating the rate of change from T=20 to T=25.

Adding the initial temperature at T=20 to the integrated rate of change to find the temperature at T=25.

Final temperature at T=25 is calculated to be 73.043 degrees Fahrenheit.

Assumption of trigonometric functions being in radians for the integration.

Alan offers free homework help on Twitch and Discord for further assistance.

Invitation for viewers to comment, like, subscribe, and engage with the content for more help.

Transcripts

Browse More Related Video

How to solve Table of Values Questions on the AP Calc AB Exam

2016 AP Calculus AB Free Response #1

2013 AP Calculus AB Free Response #3

2011 AP Calculus AB Free Response #2

2015 AP Calculus AB Free Response #1

2017 AP Calculus AB Free Response #4

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
AP CalculusFree ResponseGraphing CalculatorDerivative EstimationTemperature ModelingIntegral CalculusEducational ContentMath TutorialRiemann SumFundamental TheoremOnline Learning