Equation of a tangent line | Taking derivatives | Differential Calculus | Khan Academy
TLDRIn the video, the presenter tackles the problem of finding the equation of the tangent line to the function f(x) = x * e^x at the point x = 1. Through a step-by-step process, the presenter explains how to calculate the derivative at the given point, which turns out to be 2e, and then uses algebraic methods to determine the y-intercept, resulting in -e. The final equation of the tangent line is y = 2ex - e. The explanation is complemented with a graphical demonstration to visually confirm the solution.
Takeaways
- 📌 The problem involves finding the equation of the tangent line to a given function at a specific point.
- 🔍 The function in question is f(x) = x * e^x, and we are interested in the tangent line at x = 1.
- 📈 To find the tangent line, one must first determine the slope of the tangent line, which is the derivative of the function at the given point.
- 🧮 The derivative of x is 1, and the derivative of e^x is e^x, leading to the use of the product rule for differentiation.
- 🌟 The derivative of the function f(x) = x * e^x is found to be f'(x) = e^x + x * e^x using the product rule.
- 🏷 At x = 1, the derivative (slope of the tangent line) is calculated to be 2e.
- 📝 To find the equation of the tangent line, we use the point-slope form of a line, which is y - y1 = m(x - x1), where m is the slope and (x1, y1) is the point through which the line passes.
- 🔎 By substituting the point (1, e) and the slope 2e into the point-slope form, we can solve for the y-intercept (b).
- 🧱 The y-intercept is found to be -e, leading to the equation of the tangent line: y = 2ex - e.
- 📊 The equation of the tangent line is confirmed by graphing it and visually checking that it touches the curve at the point (1, e).
- 🙏 The video acknowledges the persistence of [? Akosh ?] in prompting the exploration of this mathematical problem.
Q & A
What is the main topic of the video?
-The main topic of the video is finding the equation of the tangent line to a given function at a specific point.
What function is being discussed in the video?
-The function being discussed is f(x) = x * e^x.
What is the point at which the tangent line is to be found?
-The tangent line is to be found at the point where x = 1, which corresponds to the point (1, e) on the curve.
How is the slope of the tangent line determined?
-The slope of the tangent line is determined by finding the derivative of the function at the given point. In this case, the derivative is found using the product rule.
What is the derivative of the function f(x) = x * e^x?
-The derivative of the function f(x) = x * e^x is f'(x) = e^x + x * e^x.
What is the slope of the tangent line at x = 1?
-The slope of the tangent line at x = 1 is 2e, which is found by evaluating the derivative at that point.
How is the y-intercept of the tangent line equation found?
-The y-intercept of the tangent line equation is found by substituting the point (1, e) into the equation y = mx + b, where m is the slope, and solving for b.
What is the final equation of the tangent line?
-The final equation of the tangent line is y = 2ex - e.
How does the video confirm the correctness of the derived tangent line equation?
-The video confirms the correctness of the derived tangent line equation by graphing it on a graphing calculator and visually showing that it touches the curve at the given point (1, e).
What is the significance of the number e in the context of this problem?
-In the context of this problem, the number e is significant because it is the base of the natural logarithm and appears in the derivative of the function e^x. It also shows up in the final equation of the tangent line.
How does the video use algebraic rules to derive the equation of the tangent line?
-The video uses algebraic rules, specifically the product rule for differentiation and algebraic manipulation to solve for the y-intercept, to derive the equation of the tangent line.
Outlines
📚 Introduction to Finding the Tangent Line Equation
The paragraph begins with the speaker acknowledging a gap in their previous explanations about derivatives and tangent lines. They introduce a problem sent by a user, Akosh, which involves finding the equation of the tangent line to a given function at a specific point. The function in question is f(x) = x * e^x, and the point of interest is (1, e). The speaker explains the concept of a tangent line and its slope, which is derived from the function's derivative at the given point. They also discuss the process of graphing the function to gain an intuitive understanding of the problem. The speaker then proceeds to calculate the derivative of the function at x=1, using the product rule and the fact that the derivative of e^x is e^x. The result is 2e, which is the slope of the tangent line at the point (1, e).
🧠 Solving for the Tangent Line Equation
In this paragraph, the speaker continues the process of finding the equation of the tangent line to the function f(x) = x * e^x at the point (1, e). They have already determined the slope of the tangent line to be 2e. The speaker then explains how to find the y-intercept of the tangent line by using the point (1, e) which lies on it. By substituting the point and the slope into the general equation of a line, they solve for the y-intercept, which turns out to be -e. The final equation of the tangent line is y = 2ex - e. The speaker then confirms the solution by graphing the tangent line using a graphing calculator and shows that it indeed touches the curve at the point (1, e). They conclude by acknowledging Akosh's persistence in seeking a solution to the problem and express hope that the explanation was useful to the viewers.
Mindmap
Keywords
💡derivative
💡tangent line
💡slope
💡function
💡graphing calculator
💡product rule
💡point-slope form
💡y-intercept
💡algebra
💡e to the x
💡curve
Highlights
The problem at hand involves finding the equation of the tangent line to a given function at a specific point.
The function in question is f(x) = x * e^x, which is not a trivial function to graph.
The point of interest is (1, e), where f(1) = e, representing the location where the tangent line will be found.
The slope of the tangent line at a point is given by the derivative of the function at that point.
The derivative of x is 1, and the derivative of e^x is e^x, which is a unique property of the exponential function.
The product rule is applied to find the derivative of the function f(x) = x * e^x.
The derivative at x = 1, or the point (1, e), is calculated to be 2e.
The equation of the tangent line is to be found using algebraic techniques learned in algebra 1.
The y-intercept of the tangent line is determined by using the point (1, e) which lies on the tangent line.
The y-intercept is calculated to be -e, completing the equation of the tangent line.
The final equation of the tangent line is y = 2ex - e, which is confirmed visually using a graphing calculator.
The process of finding the tangent line involves both understanding the derivative as the slope and applying algebraic problem-solving skills.
The example demonstrates the practical application of derivatives in determining the equation of a tangent line.
The video content is a result of a persistent inquiry from a viewer named Akosh, highlighting the value of viewer engagement in content creation.
The visual confirmation using a graphing calculator serves as a practical demonstration of the theoretical concepts discussed.
The transcript provides a step-by-step guide that is both educational and accessible, making complex mathematical concepts understandable.
Transcripts
Browse More Related Video
Equation of a tangent line [IB Maths AI SL/HL]

2011 Calculus AB free response #3 (a & b) | AP Calculus AB | Khan Academy
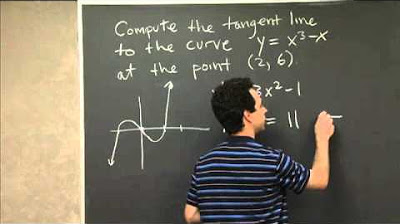
Tangent Line to a Polynomial | MIT 18.01SC Single Variable Calculus, Fall 2010

2010 AP Calculus AB Free Response #6

2022 AP Calculus AB Free Response #5
Equation of a normal line [IB Maths AI SL/HL]
5.0 / 5 (0 votes)
Thanks for rating: