Proof: d/dx(ln x) = 1/x | Taking derivatives | Differential Calculus | Khan Academy

Khan Academy
27 Apr 200809:52
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the concept of derivatives in calculus, highlighting the natural logarithm's derivative as a key focus. It explains the process of finding the derivative using the definition of a derivative and logarithm properties. Through a step-by-step derivation that involves a limit approach and a substitution technique, the video concludes that the derivative of the natural log of x is 1/x. This result is particularly interesting as it contrasts with other derivatives that result in exponents, showcasing the unique and fascinating nature of the natural logarithm's derivative.

Takeaways
  • πŸ“š The natural log (ln) is equivalent to the logarithm with base e.
  • πŸ” The derivative of the natural log of x is explored using the definition of a derivative and limits.
  • πŸ“ˆ The process involves taking the limit of the slope as the difference between two points approaches zero.
  • 🌟 The natural log of x plus delta x is manipulated using logarithm properties to simplify the expression.
  • 🧠 Logarithm properties, such as log(a) - log(b) = log(a/b), are applied to the derivative calculation.
  • 🎨 A substitution is made with u = delta x / x to simplify the expression and to approach the limit as u approaches 0.
  • πŸ“Š The limit of (1 + u)^(1/u) as u approaches 0 is a key step in the calculation, which is related to the number e.
  • πŸ…°οΈ The expression is further simplified using the property a^(bc) = (a^b)^c, leading to ln(1 + u)^(1/u).
  • πŸ‘‰ The final result of the derivative of ln(x) is 1/x, highlighting the unique nature of the natural log function.
  • 🌐 The derivative of the natural log function is significant because it results in a power function, unlike other exponential functions.
  • πŸŽ“ The explanation showcases the interplay between calculus and logarithms, emphasizing the importance of understanding fundamental concepts.
Q & A

  • What is the main topic of the transcript?

    -The main topic of the transcript is the derivative of the natural logarithm function.

  • What is the natural log defined as in the context of the transcript?

    -In the transcript, the natural log of something is defined as the logarithm base e of that something.

  • What is the basic concept of a derivative in calculus?

    -The basic concept of a derivative in calculus, as mentioned in the transcript, is to find the slope at a particular point by taking the limit as the difference between two points approaches zero.

  • How does the speaker approach the calculation of the derivative of the natural log of x?

    -The speaker approaches the calculation by applying the definition of a derivative, using properties of logarithms, and making a substitution to simplify the expression, ultimately leading to the result.

  • What logarithmic property is used to simplify the expression in the calculation?

    -The logarithmic property used is log(a) - log(b) = log(a/b), which helps simplify the expression by allowing the combination of logs into a single log expression.

  • What is the significance of the substitution made in the calculation?

    -The substitution made, where u = delta x / x, simplifies the expression and allows for the application of properties of limits and exponents, leading to the recognition of the definition of e and the final result.

  • What is the limit expression that the speaker recognizes as related to the definition of e?

    -The limit expression recognized is the limit as u approaches 0 of (1 + u)^(1/u), which is related to the definition of e as the limit of (1 + 1/n)^n as n approaches infinity.

  • What is the final result of the derivative of the natural log of x?

    -The final result of the derivative of the natural log of x is 1/x.

  • Why does the speaker find the derivative of the natural log of x to be particularly interesting?

    -The speaker finds it interesting because, unlike other derivatives that result in another exponent, the derivative of the natural log results in a reciprocal function, x to the negative 1 or 1/x, which is a unique outcome.

  • How does the concept of e relate to the derivative of the natural log of x?

    -The concept of e is integral to the calculation as it is the limit of (1 + u)^(1/u) as u approaches 0, which is the same as the limit of (1 + 1/n)^n as n approaches infinity. This limit is used to derive the final result of the derivative, which is 1/x.

  • What is the significance of the final result for the derivative of the natural log of x in calculus?

    -The significance of the final result, 1/x, is that it provides a fundamental relationship between the natural logarithm function and its derivative, which is crucial for various applications in calculus, including optimization and related rates problems.

Outlines
00:00
πŸ“š Introduction to Derivatives and Natural Logarithm

The speaker introduces the concept of derivatives, highlighting two of the coolest derivatives in calculus. The focus then shifts to the natural logarithm (ln), which is equivalent to the logarithm with base e. The speaker explains the process of finding the derivative of the natural log of x, using the definition of a derivative and the limit as the difference between two points approaches zero. The explanation involves taking the derivative of ln(x) and simplifying it using logarithm properties, such as log(a) - log(b) = log(a/b), and the fact that logarithm expressions follow exponent rules. The speaker emphasizes the importance of understanding logarithm properties and previous derivative videos for a better grasp of the concept.

05:05
πŸ”’ Derivative of Natural Logarithm and Limit Properties

Continuing from the previous paragraph, the speaker delves into the calculation of the derivative of the natural logarithm function. A substitution is made, introducing a new variable u, which simplifies the expression. The speaker then uses the properties of exponents and logarithms to further simplify the expression and find the limit as u approaches zero. The process leads to a familiar concept in calculus, the number e, which is derived from the limit of (1 + 1/n)^n as n approaches infinity. The speaker concludes by stating that the derivative of the natural log of x is 1/x, which is a fascinating result given that it does not lead to another exponent but rather to the inverse of x. This result is considered neat because it showcases the unique behavior of the natural logarithm function in calculus.

Mindmap
Keywords
πŸ’‘Derivative
In calculus, the derivative is a fundamental concept that describes the rate of change of a function at a particular point. It is used to analyze the behavior of functions, such as determining the slope of a curve at a given point. In the video, the derivative of the natural log function is being explored, which is a specific application of the derivative concept.
πŸ’‘Natural Log
The natural log, often denoted as ln or log_e, is a logarithm with base e, where e is a mathematical constant approximately equal to 2.71828. It is a crucial concept in calculus, especially in relation to exponential growth and decay processes. The video focuses on understanding and calculating the derivative of the natural log function.
πŸ’‘Logarithm Properties
Logarithm properties are rules that govern the behavior of logarithmic expressions, such as the product and quotient rules, and the change of base formula. These properties are essential for simplifying and manipulating logarithmic expressions, which is a key part of the process shown in the video for finding the derivative of the natural log function.
πŸ’‘Exponential Functions
Exponential functions are mathematical functions of the form f(x) = a^x, where a is a constant and x is the variable. They are closely related to logarithmic functions and are used to model growth and decay processes. The video touches on the connection between exponential functions and the natural log when discussing the derivative.
πŸ’‘Limit
In mathematics, a limit is a value that a function or sequence approaches as the input (or index) approaches some point. Limits are a fundamental concept in calculus, used to define continuity, derivatives, and integrals. The video script discusses taking the limit as the difference between two points approaches zero to find the derivative.
πŸ’‘Slope
Slope is a measure of the steepness of a line, representing the rate of change of the y-values with respect to the x-values. In the context of the video, the slope is used to describe the tangent to a curve at a particular point, which is the geometric interpretation of the derivative.
πŸ’‘Substitution
Substitution is a mathematical technique used to simplify expressions or to solve equations by replacing one or more variables with equivalent expressions. In the video, substitution is used to transform the expression for the derivative of ln(x) into a more manageable form.
πŸ’‘e (Euler's number)
Euler's number, commonly denoted by e, is a significant mathematical constant approximately equal to 2.718281828459045. It is the base of the natural logarithm and has extensive applications in various fields, including calculus, where it appears in formulas for growth and decay, and in the definition of the derivative of the natural log function.
πŸ’‘Logarithmic Functions
Logarithmic functions are a class of mathematical functions that are the inverse of exponential functions. They are used to solve equations involving products of the same base raised to different powers. The video script focuses on the natural logarithm, which is a specific type of logarithmic function with base e.
πŸ’‘Rate of Change
The rate of change is a measure of how quickly a quantity changes with respect to another quantity. It is a core concept in calculus and is used to analyze the behavior of functions, such as the speed of an object or the growth rate of a population. The derivative of a function at a point represents the instantaneous rate of change at that point.
Highlights

The introduction of the two coolest derivatives in calculus.

The natural log is equivalent to the logarithm base e.

Derivative definition involves finding the slope at a point by taking the limit of the difference between two points.

The derivative of natural log of x is explored using logarithm properties.

Logarithm property simplification: log(a) - log(b) = log(a/b).

The substitution method is introduced to simplify the derivative calculation, with u = delta x / x.

The limit of (1 + u)^(1/u) as u approaches 0 is e, a fundamental mathematical constant.

The derivative of the natural log of x is found to be 1/x, which is a surprising result given other derivatives lead to exponents.

The natural log derivative result demonstrates the unique behavior of the natural log function compared to other exponents.

The explanation of how the natural log derivative relates to the concept of e and its significance in mathematics.

The use of logarithm and exponent properties to simplify complex expressions in calculus.

The demonstration of the derivative calculation process, which is both educational and insightful.

The clear and detailed walkthrough of the derivative of the natural log function, making complex calculus concepts accessible.

The connection between the natural log derivative and the concept of compound interest, illustrating the practical applications of mathematical theories.

The comprehensive explanation of the natural log derivative, highlighting the interplay between different branches of mathematics.

The emphasis on the importance of understanding logarithm properties and the concept of limits in calculus.

The final result of the derivative of the natural log of x being 1/x, a fundamental result in calculus.

Transcripts

Browse More Related Video

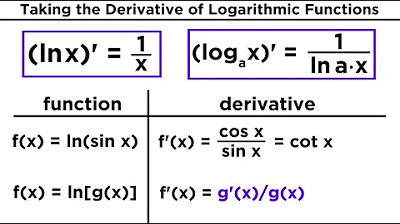
Derivatives of Logarithmic and Exponential Functions

Limits and Derivatives

_-substitution: definite integral of exponential function | AP Calculus AB | Khan Academy

Derivative of log_x (for any positive base a­1) | AP Calculus AB | Khan Academy

Applying the Definition of the Derivative to 1/x

Logarithmic functions differentiation | Advanced derivatives | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusDerivativesNatural LogExponential GrowthMathematicsEducational ContentLogarithm PropertiesLimit Conceptse (Euler's number)Slope of Functions