Even More Chain Rule
TLDRThe video script provides a comprehensive explanation of the chain rule in calculus, demonstrating how it applies to composite functions. It defines the chain rule and illustrates its application through several examples, breaking down complex derivative calculations into understandable steps. The script emphasizes the process of identifying inner and outer functions, calculating their derivatives, and then applying the chain rule to find the derivative of the composite function. It aims to clarify the concept for viewers and encourages practice through additional examples.
Takeaways
- 📚 The chain rule is a fundamental concept in calculus, particularly for understanding the derivatives of composite functions.
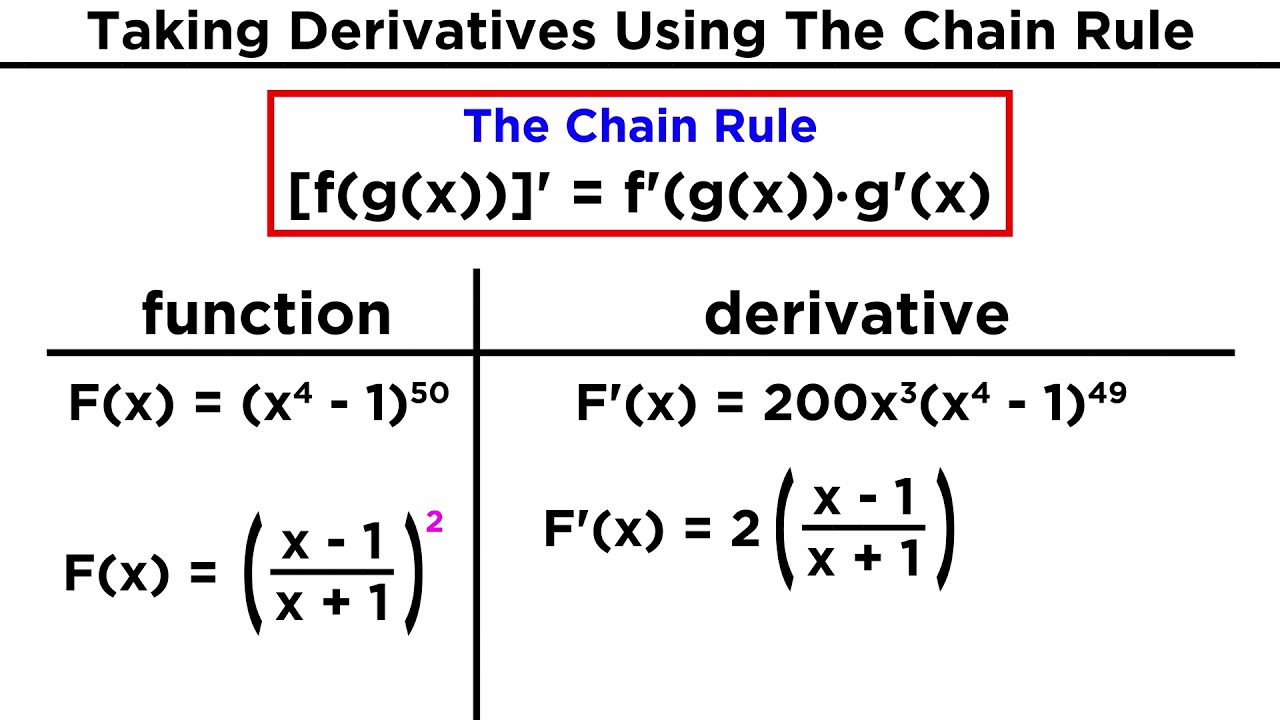
- 🔄 The chain rule states that the derivative of a composite function f(x) = h(g(x)) is f'(x) = h'(g(x)) * g'(x).
- 🌟 When applying the chain rule, it's important to identify the inner function (g(x)) and the outer function (h(x)).
- 📈 To find the derivative of the composite function, first calculate the derivative of the inner function and then multiply it by the derivative of the outer function evaluated at the inner function.
- 🧠 The chain rule helps to break down complex derivative problems into simpler, manageable parts.
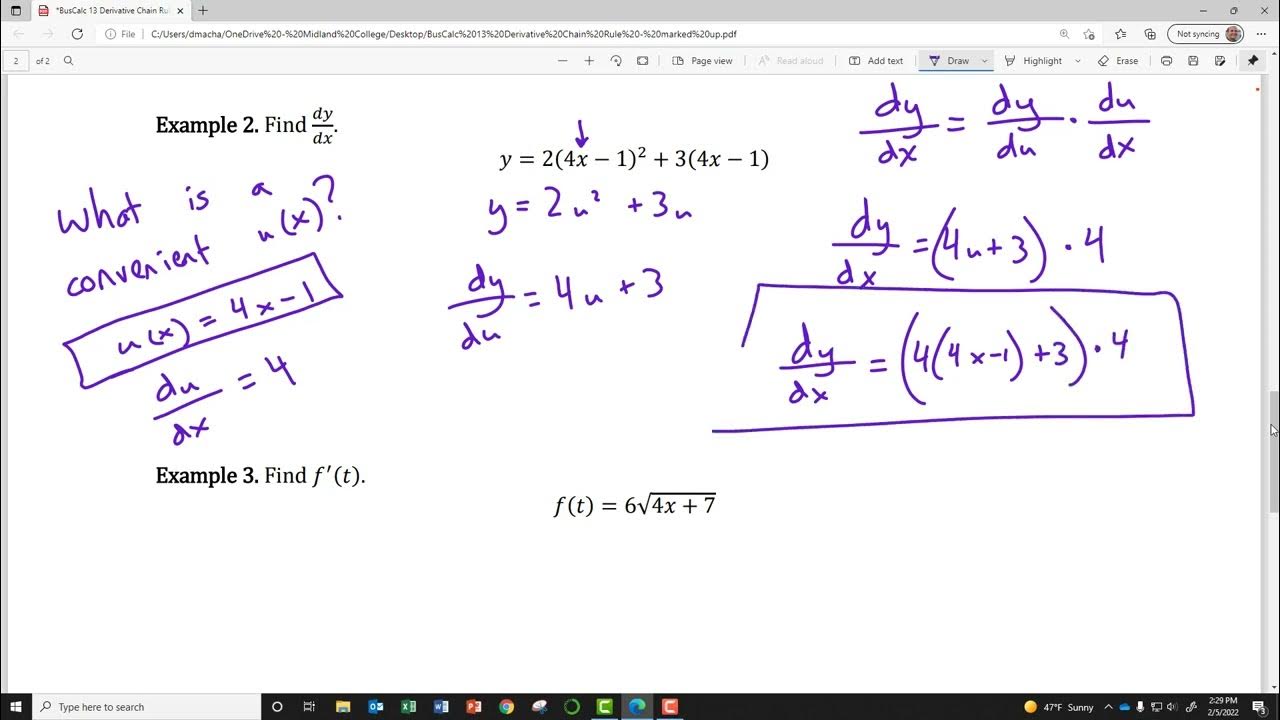
- 📊 Example 1: For f(x) = (x^2 + 5x + 3)^5, the inner function g(x) = x^2 + 5x + 3 and the outer function h(x) = x^5. The derivative f'(x) is found by applying the chain rule.
- 📊 Example 2: For f(x) = (x^7 - 3x^9)^(-10), the inner function g(x) = x^7 - 3x^9 and the outer function h(x) = x^(-10). The derivative is calculated using the chain rule by finding g'(x) and h'(g(x)).
- 📊 Example 3: For f(x) = (5x^(-8) + x^(-8))^(1/5), the chain rule simplifies the process by directly taking the derivative of the inner function and adjusting for the outer function.
- 🔍 The chain rule is not only useful for computation but also for gaining a deeper understanding of the relationship between the functions and their derivatives.
- 📝 Practice is key to mastering the chain rule; re-visiting examples and trying new ones can solidify understanding.
- 🎓 The chain rule is a powerful tool in a mathematician's arsenal, with applications extending beyond basic calculus into more advanced mathematical fields.
Q & A
What is the chain rule in calculus?
-The chain rule is a fundamental rule in calculus that is used to find the derivative of a composite function. It states that the derivative of a function f(x) which is equal to h(g(x)) is the product of the derivative of the outer function h(x) evaluated at g(x), and the derivative of the inner function g(x).
How is the chain rule expressed mathematically?
-Mathematically, the chain rule is expressed as: (f'(x) = h'(g(x)) * g'(x)). Here, f'(x) represents the derivative of the function f(x), h'(x) is the derivative of the outer function h(x), and g'(x) is the derivative of the inner function g(x).
What are the components of a composite function in the context of the chain rule?
-In the context of the chain rule, a composite function is made up of two components: the outer function (f) and the inner function (g). The outer function is the function that is being evaluated last, and the inner function is the function that is being used as an input to the outer function.
What is an example of a composite function?
-An example of a composite function given in the script is f(x) = (x^2 + 5x + 3)^5. Here, the inner function g(x) is x^2 + 5x + 3, and the outer function h(x) is x^5.
How do you find the derivatives of the outer and inner functions in the chain rule?
-To find the derivatives in the chain rule, you first identify the inner function (g(x)) and the outer function (h(x)). Then, you take the derivative of the inner function with respect to x (g'(x)) and the derivative of the outer function evaluated at the inner function (h'(g(x))).
What is the significance of evaluating the outer function derivative at the inner function in the chain rule?
-Evaluating the outer function derivative at the inner function is crucial because it accounts for how the outer function changes as the input (which is the inner function) changes. This is necessary for accurately determining the overall derivative of the composite function.
Can the chain rule be applied to functions with more than two layers of composition?
-Yes, the chain rule can be extended to functions with more than two layers of composition. If a function is composed of multiple layers, you would apply the chain rule iteratively, starting from the innermost function and working your way outward.
What is the derivative of g(x) = x^2 + 5x + 3?
-The derivative of g(x) = x^2 + 5x + 3 is g'(x) = 2x + 5.
What is the derivative of h(x) = x^5?
-The derivative of h(x) = x^5 is h'(x) = 5x^4.
How does the chain rule help in simplifying the process of finding derivatives?
-The chain rule simplifies the process of finding derivatives of composite functions by breaking down the problem into smaller parts. Instead of trying to find the derivative of the entire composite function at once, you find the derivatives of the individual functions and then multiply them according to the chain rule, which makes the process more manageable.
What is the final expression for f'(x) in the example where f(x) = (x^2 + 5x + 3)^5?
-The final expression for f'(x) in the given example is 5(x^2 + 5x + 3)^4 * (2x + 5).
How does the chain rule relate to the concept of functions and their dependencies?
-The chain rule relates to the concept of functions and their dependencies by showing how the rate of change of a function (f'(x)) depends on the rates of change of its constituent functions (g'(x) and h'(x)). It illustrates how the output of one function (g(x)) becomes the input for another (h(x)), and how this relationship affects the overall derivative.
Outlines
📚 Introduction to the Chain Rule
This paragraph introduces the concept of the chain rule in calculus, providing a definition and context for its application. It explains that the chain rule is used when dealing with composite functions, such as f(x) = h(g(x)), and that the derivative of f(x) is the product of the derivative of the inner function (g'(x)) and the derivative of the outer function evaluated at the inner function (h'(g(x))). The explanation is supported by a specific example where f(x) = (x^2 + 5x + 3)^5, and the process of identifying the inner and outer functions, calculating their derivatives, and applying the chain rule to find the derivative of the composite function is detailed. The paragraph aims to make the concept more digestible by walking through the application of the chain rule step by step.
🧠 Further Examples of the Chain Rule
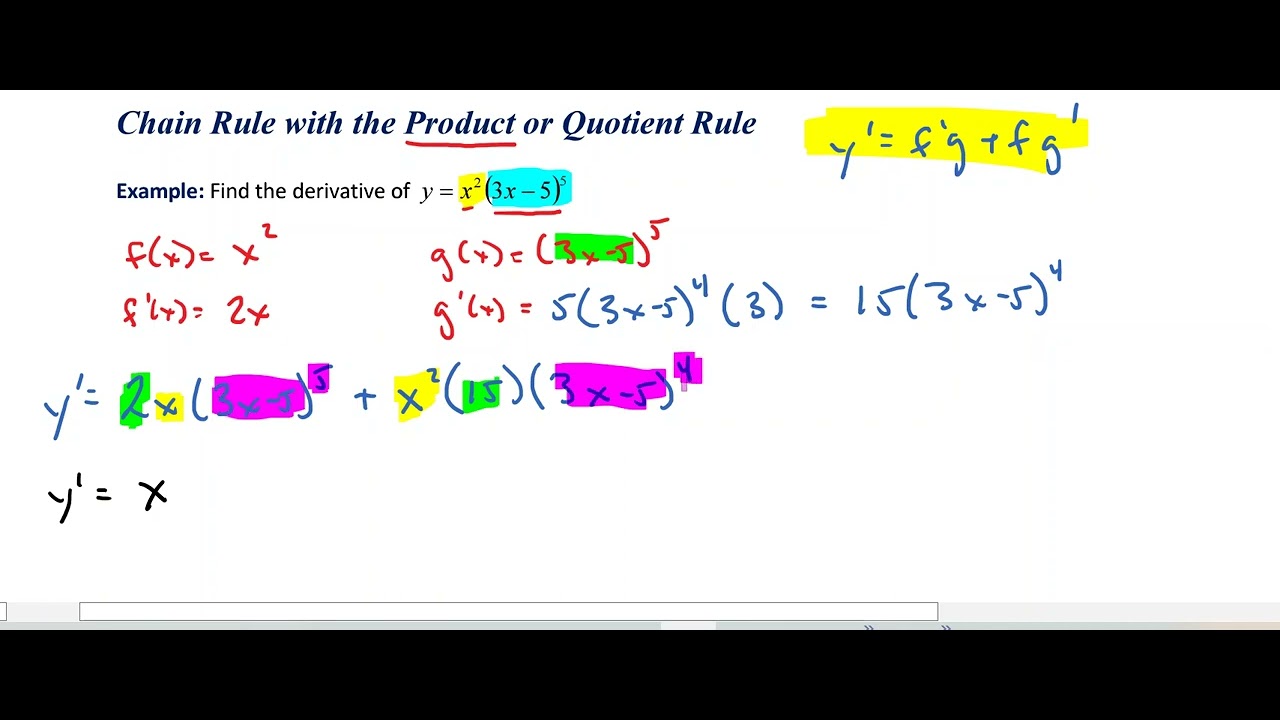
This paragraph continues the discussion on the chain rule by providing additional examples to reinforce understanding. It starts with a new composite function where g(x) = x^7 - 3x^(-9) and h(x) = x^(-10), and then calculates f(x) = h(g(x)). The paragraph walks through the process of finding the derivatives of g(x) and h(x), and then applying the chain rule to find the derivative of the composite function f(x). Another example is given where f(x) = (5x^(-8) + x^(-8))^(5/5), and the chain rule is applied without explicitly writing h(g(x)). The paragraph emphasizes the importance of understanding the chain rule for solving more complex calculus problems and promises more examples in future presentations to further clarify the concept.
Mindmap
Keywords
💡Chain Rule
💡Composite Function
💡Derivative
💡Inner Function
💡Outer Function
💡Function Composition
💡g'(x)
💡h'(x)
💡Differentiation
💡Composite Function Examples
Highlights
The chain rule is introduced as a fundamental concept in calculus, particularly for handling composite functions.
The chain rule states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function, multiplied by the derivative of the inner function.
An example is provided where f(x) = (x^2 + 5x + 3)^5, demonstrating how to identify the inner and outer functions for applying the chain rule.
g(x) is identified as the inner function, x^2 + 5x + 3, and h(x) as the outer function, x^5, in the given example.
The derivatives of g(x) and h(x) are calculated as part of the chain rule application, resulting in g'(x) = 2x + 5 and h'(x) = 5x^4.
The chain rule is applied to find the derivative of the composite function f(x) by multiplying g'(x) by h'(g(x)).
Another example is presented with g(x) = x^7 - 3x^(-3) and h(x) = x^(-10), illustrating the process of applying the chain rule with different functions.
The derivative of f(x) is found by applying the chain rule, multiplying the derivative of g(x) by h'(g(x))
A quick example demonstrates that the chain rule simplifies the process of finding the derivative of composite functions without needing to explicitly calculate h(g(x)) every time.
The chain rule is shown to be a powerful tool for differentiating complex functions, which is crucial for advanced studies in calculus.
The transcript emphasizes the importance of understanding the chain rule for those looking to deepen their knowledge of calculus and its applications.
The chain rule is applicable not only to polynomial functions but also to more complex expressions, as shown in the examples provided.
The process of applying the chain rule is reiterated, emphasizing the need to calculate the derivatives of both the inner and outer functions and then combine them appropriately.
The transcript provides a clear and detailed explanation of the chain rule, making it accessible to learners at various levels of mathematical understanding.
The chain rule is a foundational concept that enables the analysis of rates of change in scenarios where multiple functions are combined.
The transcript encourages learners to practice the chain rule with multiple examples to solidify their understanding and prepare for more complex applications.
The chain rule is a key mathematical tool that has practical applications in various fields, including physics, engineering, and economics, by helping to model dynamic systems.
The transcript concludes with a promise of more examples in future presentations, indicating the ongoing importance and relevance of the chain rule in mathematical education.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: