Taylor series | Chapter 11, Essence of calculus

3Blue1Brown

7 May 201722:19

EducationalLearning

32 Likes 10 Comments

TLDRThe script delves into the significance of Taylor series in approximating functions across various fields, highlighting their utility in simplifying complex problems, such as those in physics involving pendulums. It explains the process of constructing a quadratic approximation for the cosine function near x=0, emphasizing the role of derivatives in shaping the polynomial. The concept of Taylor polynomials and their generalization to different functions and inputs is explored, along with the idea of series convergence and divergence, illustrating the power and limitations of Taylor series in capturing the behavior of functions.

Takeaways

🌟 Taylor series are crucial mathematical tools used in various fields such as math, physics, and engineering for approximating functions.
📚 The concept of Taylor series involves using polynomials to approximate non-polynomial functions near a specific input, making them easier to compute and manipulate.
📈 The approximation process begins with identifying the value of the function and its derivatives at the input point, ensuring the polynomial matches these values.
🔄 Taylor series leverage cyclic patterns of derivatives, such as those of cosine, which repeat in a simple 1, 0, -1, 0 sequence when evaluated at x=0.
🎯 The accuracy of a Taylor polynomial increases with the number of terms included, but this also makes the polynomial more complex.
📊 Geometrically, Taylor series can be understood in terms of approximating the area under a curve, with higher-order terms accounting for the areas of shapes like triangles.
🌐 Taylor series can converge to a specific function for all inputs, as seen with e^x, or only within a certain range, like the natural log of x around x=1.
💡 The radius of convergence defines the interval around the input where the Taylor series provides an accurate approximation.
🚀 Taylor series are not just finite polynomials but can represent infinite series, offering a powerful way to capture the behavior of functions near a point.
📚 The study of Taylor series includes understanding error bounds, convergence tests, and their wide applications in advanced mathematical and scientific problems.

Q & A

What is the significance of Taylor series in various fields?
-Taylor series are crucial in fields like math, physics, and engineering as they provide a powerful tool for approximating functions, which can simplify complex calculations and analyses.
How did the speaker's understanding of Taylor series click during a physics class?
-The speaker realized the importance of Taylor series when studying the potential energy of a pendulum. By approximating the cosine function with a quadratic term, the problem became much simpler and clarified the relationship between pendulums and other oscillating phenomena.
What is the motivation behind using polynomials to approximate non-polynomial functions in Taylor series?
-Polynomials are preferred for approximation because they are easier to compute, take derivatives, integrate, and are generally more manageable than other functions.
How does the Taylor series approximation work for the cosine function near x equals 0?
-The Taylor series approximation for cosine near x=0 involves finding a polynomial that matches the value, first derivative, and second derivative of the cosine function at x=0. This leads to the quadratic approximation of 1 - (theta^2)/2.
What are the roles of c0, c1, and c2 in the quadratic approximation of the cosine function?
-c0 ensures the approximation equals 1 at x=0, matching the cosine value. c1 is set to 0 to ensure a flat tangent line at x=0. c2, with a value of -1/2, ensures the second derivative matches that of the cosine function, controlling the curvature.
Why is the Taylor series for e^x particularly simple?
-The Taylor series for e^x is simple because the derivative of e^x is itself, meaning all higher-order derivatives at x=0 are also 1. This results in a series of terms that are simply 1 times x, x^2/2!, x^3/3!, and so on.
What is the concept of Taylor polynomials?
-Taylor polynomials are the polynomials obtained by truncating the Taylor series at a certain term. They provide an approximation of a function based on its derivatives at a single point.
How does the radius of convergence relate to the Taylor series?
-The radius of convergence is the maximum distance from the point of approximation where the Taylor series still converges to the actual function. Outside of this radius, the series may diverge and not represent the function accurately.
What happens when a Taylor series is summed up infinitely?
-An infinite Taylor series, if it converges, is called a Taylor series. It represents the sum of all terms of the polynomial approximation and can equal the actual function value, depending on the function and the range of convergence.
How does the geometric interpretation of the second-order term in Taylor series relate to the fundamental theorem of calculus?
-The second-order term in Taylor series can be understood geometrically as the area of a small triangle that represents the change in the area under the graph due to a small change in x. This ties back to the fundamental theorem of calculus, which states that the graph itself represents the derivative of the area function.
What is the general process for creating a Taylor series?
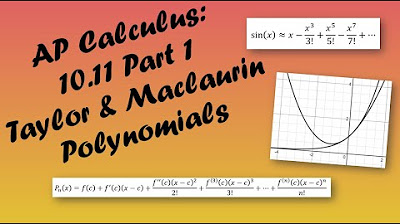
-To create a Taylor series, one calculates the derivatives of the function, evaluates them at a chosen point (usually 0 or another point of interest), and then constructs a polynomial using these derivatives as coefficients divided by the appropriate factorials. This process is repeated to the desired degree of approximation.

Outlines

00:00

📚 Introduction to Taylor Series

The paragraph introduces the concept of Taylor series and their significance in various fields such as math, physics, and engineering. It discusses the author's realization of their importance during a physics class while studying the potential energy of a pendulum. The author explains how approximating the cosine function with a quadratic polynomial simplifies the problem, leading to an exploration of how to construct such an approximation using the principles of Taylor series.

05:05

🔢 Deriving the Quadratic Approximation

This paragraph delves into the process of deriving a quadratic approximation for the cosine function using Taylor series. It explains the step-by-step method of determining the coefficients (c0, c1, and c2) by matching the function's value, first derivative, and second derivative at x=0. The author also discusses the concept of higher-order derivatives and how they can be used to improve the approximation by adding more terms to the polynomial. The paragraph concludes with the best possible quadratic and cubic approximations for the cosine function.

10:06

🌐 Generalizing Taylor Series

The paragraph discusses the general process of creating Taylor series by approximating functions near a given input point using polynomials derived from the function's derivatives. It explains how to construct Taylor polynomials for any function at a specific input (a) by evaluating derivatives at that point and incorporating them into the polynomial with appropriate factorial adjustments. The paragraph also touches on the concept of the radius of convergence, which defines the range within which the Taylor series converges to the actual function.

15:10

📈 Geometric Interpretation of Taylor Series

This paragraph provides a geometric interpretation of the second-order term in Taylor series by relating it to the fundamental theorem of calculus. It describes how the area under a curve can be approximated by considering the sum of rectangles, triangles, and higher-order geometric shapes, each corresponding to a term in the Taylor series. The author illustrates how the shape and size of these shapes are related to the function's derivatives and how they contribute to the overall approximation of the function's area.

20:12

🌟 Infinite Series and Convergence

The final paragraph discusses the concept of infinite series and the convergence of Taylor series. It explains how an infinite sum can be represented as a series that converges to a specific value, allowing for the approximation of functions using their Taylor series. The author provides examples of functions like e^x, sine, and natural log, highlighting how their Taylor series behave differently across various ranges of inputs. The paragraph concludes with a reminder of the fundamental intuition behind Taylor series and a brief mention of upcoming content on probability.

Mindmap

Keywords

💡Taylor series

A Taylor series is a mathematical representation that approximates a function by summing an infinite series of terms calculated from the values of its derivatives at a single point. In the video, Taylor series are highlighted as powerful tools in various fields due to their ability to approximate complex functions with polynomials, which are easier to work with. The Taylor series for a function f(x) near a point a is given by the sum of terms (f^n(a))/(n!) * (x-a)^n, where f^n(a) represents the nth derivative of f evaluated at a.

💡approximation

Approximation in the context of the video refers to the process of simplifying complex functions or values by using simpler expressions that are close in value. The Taylor series is a method of approximation that involves using polynomials to approximate non-polynomial functions, which is particularly useful in fields like math, physics, and engineering where precise calculations are often required but the functions involved are difficult to compute directly.

💡derivative

A derivative in calculus is a measure of how a function changes as its input changes. It is the slope of the function at a particular point and is used to find the rate of change or the tangent line to the function at that point. In the context of the video, derivatives are crucial for constructing Taylor series because they provide the necessary information to determine the coefficients of the polynomial approximation.

💡cosine function

The cosine function is a fundamental trigonometric function that describes periodic changes in various phenomena, such as the position of the pendulum in the video's example. It is defined as the ratio of the adjacent side to the hypotenuse in a right-angled triangle, and its graph is a wave-like curve that oscillates between -1 and 1.

💡pendulum

A pendulum is a weight suspended from a pivot so that it can swing freely back and forth. In physics, pendulums are used to study oscillatory motion and the concept of mechanical energy. The video uses the pendulum as an example to illustrate how the cosine function and its approximation can be applied to understand the potential energy of the pendulum in different positions.

💡potential energy

Potential energy is the stored energy an object possesses due to its position relative to other objects, forces, or fields. In the context of the video, the potential energy of a pendulum is discussed, which depends on its height above the lowest point in its swing and is related to the gravitational force acting on the pendulum.

💡polynomial

A polynomial is an algebraic expression consisting of variables and coefficients, involving only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. Polynomials are used in the Taylor series as they are simpler and more manageable than the functions they approximate, making calculations like differentiation and integration easier.

💡oscillating phenomena

Oscillating phenomena refer to the periodic or repetitive variations in physical quantities such as displacement, pressure, or electric charge. These are common in physical systems like pendulums, springs, and waves. The video uses the pendulum as an example of an oscillating system to demonstrate how mathematical tools like Taylor series can simplify the analysis of such systems.

💡Taylor polynomials

Taylor polynomials are a specific type of polynomial that serves as an approximation of a function near a point by using the function's derivatives at that point. They are a component of Taylor series and provide a way to represent complex functions with simpler polynomial forms, which can be particularly useful for calculations and analysis in various applications.

💡radius of convergence

The radius of convergence is a measure that describes the range of inputs for which a Taylor series converges to the function it represents. Outside of this range, the series may diverge, meaning it does not approach a specific value as more terms are added. The radius of convergence is an important concept in understanding the applicability and limitations of Taylor series approximations.

💡fundamental theorem of calculus

The fundamental theorem of calculus is a key result that connects integration and differentiation. It states that if a function is continuous on a closed interval, then the derivative of the integral of that function from one point to another is the function itself. This theorem is foundational in calculus and has implications for understanding the relationship between the area under a curve and the function's derivative.

Highlights

Taylor series is a powerful tool for approximating functions, crucial in math, physics, and engineering.

The importance of Taylor series was realized not in calculus but in a physics class while studying the potential energy of a pendulum.

The cosine function's awkwardness in pendulum problems can be mitigated by approximating it with a quadratic function.

Taylor series involves taking non-polynomial functions and finding polynomials that approximate them near a given input.

Polynomials are preferred for approximations due to their simplicity in computation, differentiation, and integration.

The process of constructing a quadratic approximation for cosine of x near x=0 involves setting specific conditions based on the function's value and derivatives at x=0.

The constant term c0 in the approximation ensures the polynomial's value matches that of cosine x at x=0.

The coefficient c1 controls the derivative of the approximation to match that of the cosine function at x=0.

The second derivative of the polynomial is exactly 2 times c2, which should be -1/2 for a good approximation of cosine x.

The best possible quadratic and cubic approximation of cosine is 1 - (1/2)x^2, as adding a cubic term does not improve the approximation.

Adding a fourth-order term to the polynomial improves the approximation of cosine x, with the coefficient c4 being 1/24.

The process of creating a Taylor polynomial involves dividing the nth derivative of the function by n! to cancel out the factorial effect.

Each term added to the Taylor polynomial corresponds to a higher order derivative of the function at the approximation point.

Taylor series can be used to approximate the area under a graph by considering the function as the derivative of the area function.

The second-order term in the Taylor polynomial becomes significant when approximating the area function geometrically.

An infinite sum in mathematics, or series, can converge to a specific value, as seen with the Taylor series for e^x.

The Taylor series for e^x converges to e for any input value of x, illustrating the power of Taylor series in function approximation.

The radius of convergence for a Taylor series is the maximum distance from the approximation point where the series still converges.

Taylor series translate derivative information at a single point to approximation information around that point, providing a fundamental intuition for their use.

Transcripts

Browse More Related Video

Finding Taylor Series

2011 Calculus BC free response #6a | AP Calculus BC | Khan Academy

Maclaurin series of cos(x) | Series | AP Calculus BC | Khan Academy

AP Calculus BC Lesson 10.11 Part 1

Taylor Polynomials Day 1

Avon High School - AP Calculus BC - Topic 10.11 - Example 2

Related Tags

Mathematics Physics Engineering Taylor Series Function Approximation Cosine Approximation Polynomials Calculus Concepts Educational Content Math Intuition