Using Partial Fractions in Differential Equations (Differential Equations 34)

Professor Leonard

1 May 201940:11

EducationalLearning

32 Likes 10 Comments

TLDRThis video delves into the application of partial fractions in solving differential equations, particularly those related to population modeling. The presenter shares techniques for handling partial fractions, emphasizing the importance of recognizing linear factors and setting up equations to minimize sign errors. The video serves as a refresher on algebraic techniques, showcasing how to simplify complex equations into manageable parts through the use of partial fractions, and concludes with the application of initial conditions to solve for specific variables.

Takeaways

📚 The video is a refresher on partial fractions, particularly in the context of differential equations related to population modeling.
🔄 The process of partial fraction decomposition involves separating a complex fraction into simpler fractions based on its factors.
🎓 It's important to order and factor the differential equation properly before applying partial fractions to avoid sign errors.
📝 When setting up partial fractions, ensure that the numerators are constants and the degree of the numerator is one less than the denominator.
🌟 Use algebraic techniques, like moving negatives around, to simplify the process of dealing with partial fractions.
📌 Plug in numbers like zero to eliminate one of the terms in the numerator, making it easier to solve for the coefficients.
🔢 For linear factors in population models, partial fractions often result in terms involving natural logarithms.
🧩 When combining logarithms, remember that log(a) + log(b) = log(ab), which simplifies the integration process.
🛠️ Always remember to apply the initial conditions to solve for the arbitrary constant in the final equation.
📈 The video provides three examples to illustrate the process of partial fraction decomposition and its application in differential equations.
🚀 The next video will apply these concepts to real-life population models, building on the techniques learned in this video.

Q & A

What is the main topic of the video?
-The main topic of the video is about partial fractions in differential equations, specifically focusing on algebraic techniques to handle them in population models.
Why does the video suggest setting up the differential equation in a particular order?
-Setting up the differential equation in a specific order can simplify the solution process, making it easier to identify patterns and apply mathematical techniques effectively.

Outlines

00:00

📘 Introduction to Partial Fractions in Differential Equations

The video begins with a brief introduction to the topic of partial fractions in the context of differential equations. The speaker acknowledges the upcoming video on modeling populations, which will involve partial fractions, and offers this video as a refresher for those who might need it. The aim is to make viewers comfortable with partial fractions and provide some algebraic techniques to simplify the process. The speaker plans to cover three examples to illustrate the application of partial fractions in solving differential equations.

05:03

📊 Partial Fractions and Algebraic Techniques

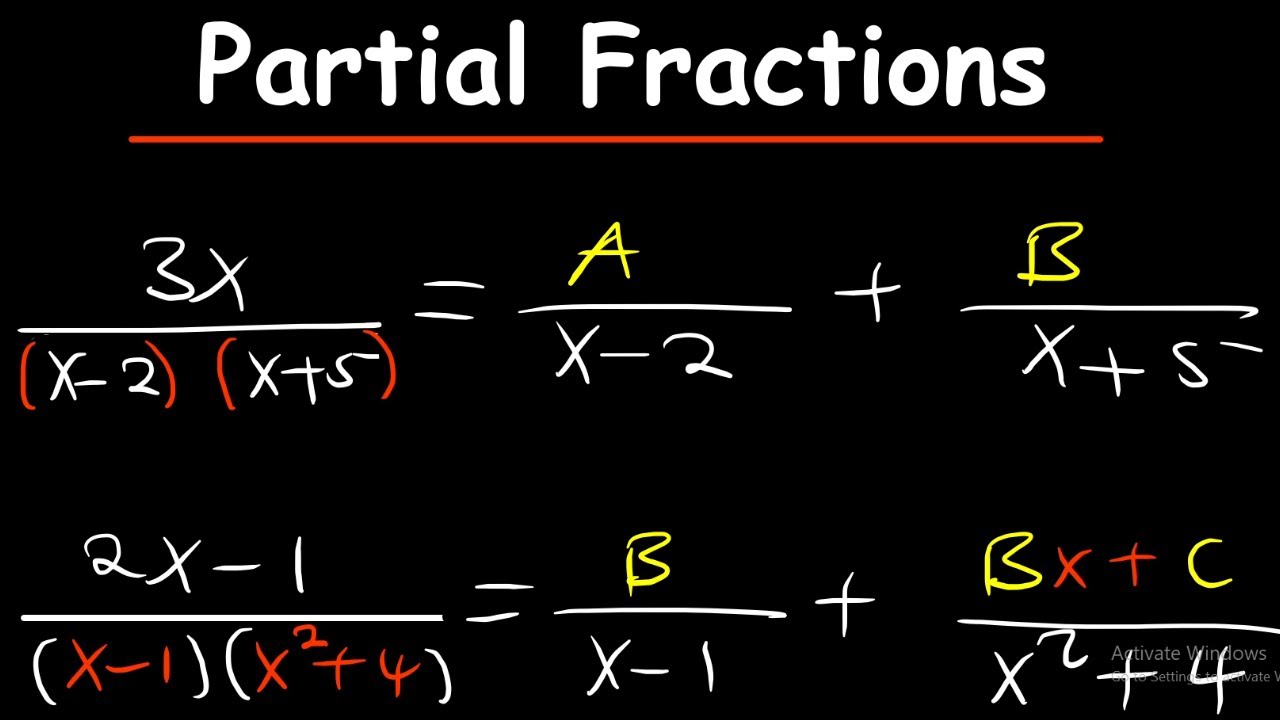
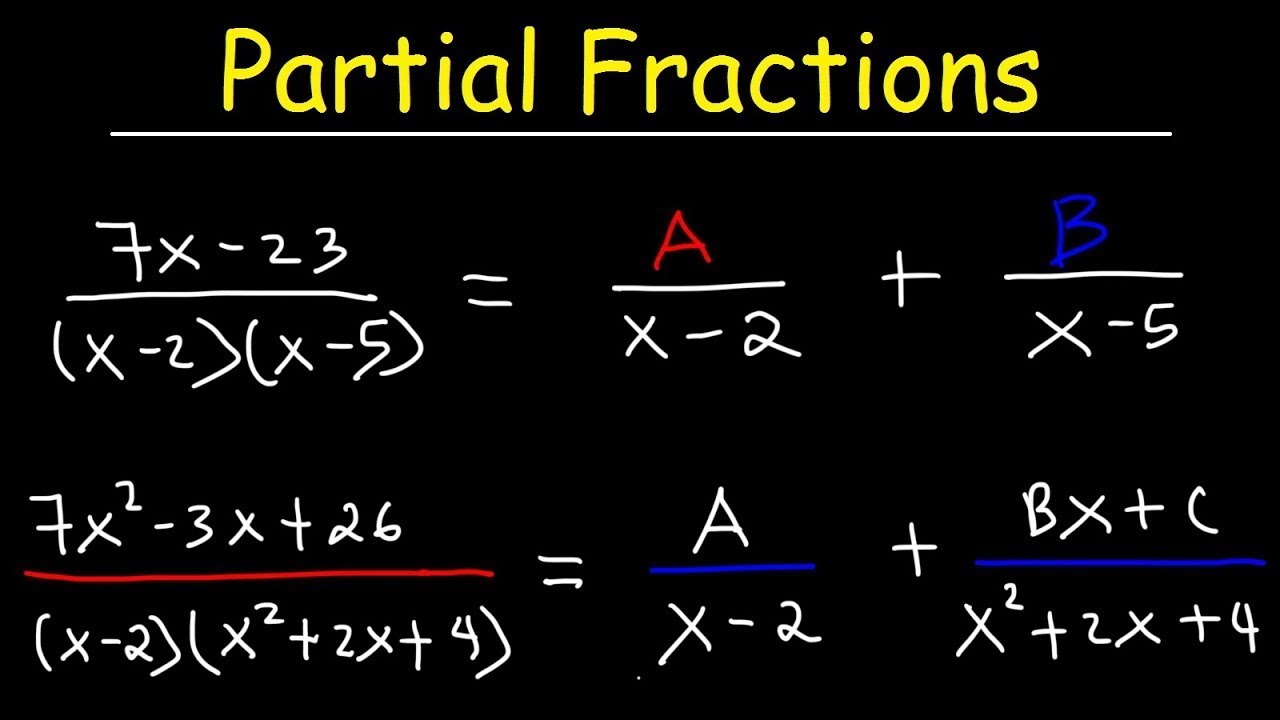
This paragraph delves into the specifics of setting up partial fractions and the algebraic techniques involved. The speaker explains how to deal with linear factors and negative signs to simplify the process. The explanation includes a step-by-step breakdown of how to separate a given differential equation into partial fractions, emphasizing the importance of order and sign to avoid errors. The speaker also introduces a trick for solving coefficients using the method of setting x to specific values to eliminate terms.

10:03

🧠 Simplifying Partial Fractions with Logarithms

The speaker continues the discussion on partial fractions, focusing on how to simplify them using logarithms. The process involves finding a common denominator and setting numerators equal, which leads to natural logarithms when integrated. The speaker provides a detailed example of how to rewrite a complex fraction as a combination of simpler fractions involving logarithms. The explanation also touches on how to handle negative terms and the importance of having a single term in the denominator for ease of computation.

15:05

🔄 Techniques for Dealing with Negative Terms in Partial Fractions

In this paragraph, the speaker shares techniques for dealing with negative terms in partial fractions. The focus is on ensuring that the negative fraction has only one term, which simplifies the process of converting to logarithms and eventually to exponentials. The speaker provides an example to illustrate the method, emphasizing the importance of factoring and grouping terms appropriately to avoid unnecessary complexity. The explanation also includes how to use initial conditions to solve for arbitrary constants in the equations.

20:06

🧩 Applying Partial Fractions to Differential Equations

The speaker applies the concepts of partial fractions to differential equations, providing a step-by-step guide on how to recognize when to use partial fractions and how to set up the equations for decomposition. The explanation includes the process of factoring out constants and dealing with linear factors to prepare for partial fraction decomposition. The speaker also discusses the importance of order and sign in the process and provides an example to demonstrate the method, including how to solve for coefficients using specific values.

25:06

📈 Finalizing Partial Fraction Decomposition in Differential Equations

The speaker concludes the discussion on partial fractions by demonstrating the final steps of decomposition in differential equations. The focus is on simplifying the resulting expressions and solving for the function of the independent variable. The speaker provides a detailed example that includes factoring, distributing, and solving for the arbitrary constant using initial conditions. The explanation emphasizes the importance of the order of terms and the handling of negative signs throughout the process.

Mindmap

Keywords

💡Partial Fractions

Partial fractions is a mathematical technique used to decompose a complex fraction into simpler fractions. In the context of the video, it is a crucial method for solving differential equations, particularly those involving linear factors. The process involves setting up a common denominator and equating the numerators to find the coefficients of the simpler fractions, which can then be integrated separately.

💡Differential Equations

Differential equations are equations that involve an unknown function and its derivatives. They are fundamental in modeling various phenomena in physics, engineering, and other disciplines. In the video, the focus is on first-order linear differential equations, which are often solvable using techniques like separation of variables and partial fractions.

💡Separation of Variables

Separation of variables is a method for solving first-order differential equations by rearranging the equation so that all terms involving the dependent variable are on one side and all terms involving the independent variable are on the other side. This allows the equation to be integrated directly. The video briefly mentions this method in the context of differential equations.

💡Integration

Integration is the mathematical process of finding the function whose derivative is a given function, or the reverse process of differentiation. In the video, integration is used to find the original function from the differential equations after applying techniques like partial fractions.

💡Initial Condition

An initial condition is a specified value of the solution to a differential equation at a given initial time or position. It is essential for finding the unique solution to an otherwise potentially infinite set of solutions. In the video, the initial condition is used to determine the arbitrary constant in the solution after applying partial fractions.

💡Linear Factors

Linear factors refer to terms in a polynomial or a differential equation that are of the first degree, meaning they involve only the first power of the variable. In the video, linear factors are crucial for applying partial fractions, as the process involves breaking down the equation into simpler fractions with linear numerators.

💡Algebraic Techniques

Algebraic techniques are methods used in algebra to solve equations or manipulate expressions. In the context of the video, these techniques are applied to simplify differential equations before solving them, such as factoring out negative terms or rearranging terms to facilitate partial fraction decomposition.

💡Natural Logarithms

Natural logarithms, denoted as ln, are a type of logarithm with base e (approximately 2.71828). They are used in various mathematical and scientific contexts, including solving certain types of differential equations. In the video, natural logarithms appear as part of the integrated form of the differential equation after applying partial fractions.

💡Exponential Functions

Exponential functions are mathematical functions of the form f(x) = a * b^x, where a and b are constants. They are widely used in modeling growth and decay processes, including population dynamics. In the video, exponential functions arise in the solutions of differential equations after applying integration and logarithms.

💡Arbitrary Constant

An arbitrary constant is a constant that is introduced into a mathematical expression or equation to account for unknowns or to allow for a family of solutions. In differential equations, it represents the freedom to adjust the solution to match initial or boundary conditions. The video explains how to determine the value of this constant using initial conditions.

Highlights

The video introduces an optional refresher on partial fractions in the context of differential equations, particularly useful for those less familiar with the concept.

The speaker emphasizes the importance of being comfortable with partial fractions, especially when dealing with population models and linear factors.

A differential equation is presented with an initial condition, highlighting the need to separate variables and deal with partial fractions.

The process of separating the differential equation is discussed, noting that the resulting integrals may not be straightforward and require partial fraction decomposition.

The speaker demonstrates how to rearrange and factor the equation to prepare for partial fractions, emphasizing the importance of order and sign in the process.

The concept of partial fractions is explained, detailing how to break down a complex fraction into simpler components.

The method of finding coefficients for partial fractions is outlined, using the technique of plugging in values to solve for unknowns.

The speaker provides a step-by-step example of how to apply partial fractions to a differential equation, including the integration process.

The importance of ensuring that the negative fraction in a partial fraction has only one term is highlighted, simplifying the subsequent exponential process.

The video presents a second differential equation example, showing how to correctly apply partial fractions and avoid common sign errors.

The speaker discusses the utility of factoring out negative terms and multiplying both sides by a constant to simplify the equation.

The process of solving for the constant term in the differential equation using the initial condition is demonstrated.

The speaker emphasizes the importance of reordering and factoring differential equations to make them more amenable to partial fraction decomposition.

A final example is provided to recap the process of partial fractions in differential equations, focusing on the recognition of linear factors and the setup for decomposition.

The video concludes with a preview of applying these concepts to population models in the next video, promising a deeper exploration of real-life applications.

Transcripts

Browse More Related Video

Calculus Chapter 3 Lecture 24 Partial Fractions

Partial Fractions Decomposition simplified

Partial Fraction Decomposion

Lec 30 | MIT 18.01 Single Variable Calculus, Fall 2007

Population Growth and Decline (Differential Equations 35)

Partial Fractions (Non-Repeating Linear Factors)

Related Tags

Partial Fractions Differential Equations Population Modeling Algebraic Techniques Integral Simplification Educational Content Mathematical Tutorial Error Avoidance Linear Factors Logarithmic Functions