Physics 60 Interference of Light (3 of 8) Path and Phase Difference: General Case

Michel van Biezen
13 May 201308:17
EducationalLearning
32 Likes 10 Comments

TLDRThis online lecture delves into the general case of light interference, where two rays from different sources with identical intensity and wavelength interact. The focus is on the path length difference, or the extra distance one ray travels, creating a phase difference. The lecture explains how to calculate the resulting electric field amplitude and intensity when the waves combine, considering various phase differences from 0 to 180 degrees. The formula derived shows the relationship between the intensity of the combined beams and the cosine squared of the phase difference, offering insight into constructive and destructive interference.

Takeaways
  • 🌟 The lecture focuses on the interference of light, specifically the general case where two rays from different sources with the same intensity and wavelength interact.
  • 🔍 The concept of path length difference is introduced, which is the extra distance one ray travels compared to the other, resulting in a phase difference.
  • 📏 The script uses the letter 'F' to denote the fraction of a wavelength difference between the two rays, which is key to understanding interference patterns.
  • 🌀 The phase difference, represented by the angle beta, is calculated by multiplying the extra distance (a fraction of the wavelength) by 2π/wavelength.
  • 📐 The interference results in constructive, destructive, or a combination of both, depending on the phase difference between the two rays.
  • 📊 The amplitude of the electric field oscillations when the two waves combine is given by 2 * E * cos(beta/2), where E is the amplitude of each individual wave.
  • 🌀 The total intensity of light at the point of interference is derived from the square of the total electric field oscillation, considering the phase difference.
  • 🔢 The intensity of the combined beams is calculated to be 4 * I_sub_nought * cos^2(beta/2), where I_sub_nought is the intensity of a single beam.
  • 📉 The intensity varies based on the phase difference, with constructive interference (beta = 0) resulting in maximum intensity and destructive interference (beta = 180 degrees) resulting in minimum intensity.
  • 📚 The general formula provided allows for calculating the intensity of any two beams of light with a phase difference, assuming they come from similar sources with the same intensity, wavelength, and frequency.
  • 📈 The script concludes with the understanding that the total intensity of two interfering beams is influenced by the cosine squared of half the phase difference between them.
Q & A

  • What is the main topic of the lecture?

    -The main topic of the lecture is the general case of interference of light, specifically focusing on the intensity of light when two rays come together with a path length difference.

  • What is meant by 'path length difference' in this context?

    -'Path length difference' refers to the extra distance traveled by one ray compared to another when two rays of light come together. This difference can result in a phase difference between the rays.

  • What happens when two rays of light with a path length difference come together?

    -When two rays of light with a path length difference come together, they interfere with each other. This interference can be constructive, destructive, or somewhere in between, depending on the phase difference.

  • How is the phase difference represented and calculated?

    -The phase difference is represented by the angle β (beta) and is calculated using the formula: (2π/λ) * (extra distance), where λ is the wavelength. The phase difference can range from 0 to 2π.

  • What happens when the phase difference (β) is zero?

    -When the phase difference (β) is zero, the cosine of β/2 is 1, resulting in the total electric field oscillation amplitude being twice the amplitude of each individual ray.

  • What is the result when the phase difference (β) is 180 degrees?

    -When the phase difference (β) is 180 degrees, the cosine of β/2 is zero, resulting in complete destructive interference where the total amplitude is zero.

  • What formula is used to find the total intensity of the two rays combined?

    -The total intensity of the two rays combined is given by the formula: I_total = 4 * I_0 * cos²(β/2), where I_0 is the intensity of a single ray and β is the phase difference.

  • How is the intensity of a single ray (I_0) defined in terms of the electric field?

    -The intensity of a single ray (I_0) is defined as I_0 = (1/μ₀c) * E_0², where E_0 is the amplitude of the electric field oscillation, μ₀ is the permeability of free space, and c is the speed of light.

  • What is the significance of the cosine squared term in the intensity formula?

    -The cosine squared term in the intensity formula accounts for the phase difference between the two rays. It determines the extent of constructive or destructive interference, thus affecting the total intensity.

  • In this context, what does β represent and how does it relate to wavelength?

    -In this context, β represents the phase difference between the two rays, and it is related to the wavelength by the formula β = (2π/λ) * (extra distance). The phase difference determines the interference pattern observed.

Outlines
00:00
🌟 Introduction to Interference of Light

This lecture introduces the concept of light interference, focusing on the general case where two rays from different sources with the same intensity and wavelength travel different distances. The path length difference causes a phase difference, resulting in constructive or destructive interference. The lecture aims to determine the intensity of light when the two rays converge, using the concept of electric field oscillations and phase angles.

05:01
📏 Calculating Intensity with Phase Difference

The lecture continues by explaining how to calculate the intensity of light at a point where two waves with a phase difference meet. The intensity is given by 1 over the magnetic constant times the speed of light, multiplied by the square of the electric field oscillation. For two waves with equal magnitudes and a phase difference, the total intensity is derived by plugging in the values and considering the cosine of half the phase angle. This detailed calculation demonstrates how the intensity varies with the phase difference between the waves.

Mindmap
Keywords
💡Interference of Light
Interference of light is a phenomenon in physics where two or more waves meet and combine to form a resultant wave. In the video, this concept is central to understanding how light behaves when two rays from different sources but with the same intensity and wavelength interact. The script discusses constructive and destructive interference, which are the two primary outcomes of this phenomenon.
💡General Case
The general case in the context of the video refers to a scenario where two rays of light from different sources meet, and there is a path length difference due to one ray traveling a greater distance. This path length difference is essential for understanding the interference pattern that results from the interaction of these rays.
💡Path Length Difference
Path length difference is the extra distance one ray of light travels compared to another when they meet at a point. In the script, this difference is crucial for determining the phase difference between the two rays, which in turn affects the type of interference that occurs.
💡Fraction of a Wavelength
A fraction of a wavelength is a measure used to describe the path length difference between two rays of light in terms of the wavelength of the light. In the video, the script uses this term to discuss the phase difference that arises due to the path length difference, which is key to calculating the resulting interference pattern.
💡Phase Difference
Phase difference is the difference in phase between two waves, measured in degrees or radians. In the context of the video, the phase difference, denoted as beta, is calculated based on the extra distance traveled by one ray and is critical in determining the intensity of light at the point of interference.
💡Electromagnetic Wave
An electromagnetic wave is a wave that carries energy through space, composed of oscillating electric and magnetic fields. The script mentions that every electromagnetic wave has an electric field oscillation, which is essential for understanding how the interference of light occurs at a point where two rays meet.
💡Vector Sum
Vector sum refers to the result of adding two or more vectors together. In the script, the vector sum is used to describe the combined effect of the electric field oscillations of the two interfering light rays, which is crucial for calculating the resultant amplitude at the point of interference.
💡Cosine
The cosine function is used in the video to relate the phase difference to the amplitude of the resultant electric field oscillation. The script explains that the amplitude of the total electric field oscillation is proportional to the cosine of the phase difference divided by two.
💡Intensity
Intensity, in the context of the video, refers to the amount of energy carried by a wave per unit area. The script discusses how the intensity of light at the point of interference is calculated by considering the amplitude of the combined electric field oscillations and is given by the formula involving the cosine squared of the phase difference.
💡Constructive and Destructive Interference
Constructive and destructive interference are two types of wave interference. Constructive interference occurs when the peaks of two waves align, resulting in a wave with greater amplitude, while destructive interference occurs when the peak of one wave aligns with the trough of another, leading to cancellation and a reduced amplitude. The script explains these concepts in the context of the phase difference between the two interfering light rays.
Highlights

The lecture focuses on the general case of light interference where two rays from different sources with the same intensity and wavelength meet.

A path length difference, or extra distance traveled by one ray compared to the other, leads to a fraction of a wavelength difference.

The phase difference, represented by angle beta, determines the type of interference - constructive, destructive, or a combination.

The phase difference is calculated as a fraction of 2π, derived from the extra distance and wavelength.

The total electric field oscillation when two waves meet is a vector sum, considering their magnitudes and phase difference.

The amplitude of the electric field oscillations is given by 2 times the individual amplitude times the cosine of half the phase difference.

When there is no phase difference, the total amplitude is simply twice the amplitude of each individual wave.

If the phase difference is 180 degrees, the waves completely cancel each other out, resulting in zero amplitude.

The intensity of light at a particular location is calculated using the electric field oscillation magnitude squared.

The total intensity for two interfering waves is 4 times the intensity of a single beam, modified by the cosine squared of half the phase difference.

The general formula for the intensity of two interfering beams with a phase difference is derived, providing a comprehensive understanding of light interference.

The lecture explores the conditions for constructive and destructive interference, highlighting the importance of path length difference and phase difference.

The relationship between the phase difference and the resulting interference pattern is mathematically described, offering a clear explanation.

The lecture demonstrates how to calculate the amplitude and intensity of light when two waves with a phase difference meet.

The impact of the phase difference on the amplitude and intensity of the resulting wave is clearly illustrated through examples.

The lecture provides a step-by-step approach to solving interference problems, making the complex topic more accessible.

The mathematical derivations and examples presented in the lecture help to deepen the understanding of the principles of light interference.

Transcripts

Browse More Related Video

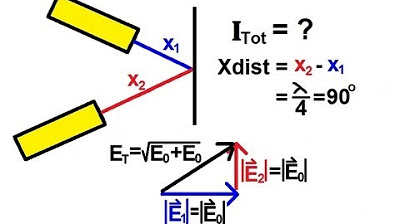
Physics 60 Interference of Light (2 of 8) Path and Phase Difference

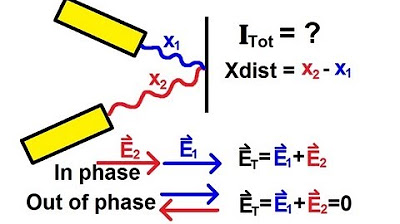
Physics 60 Interference of Light (1 of 8) Path and Phase Difference: Introduction

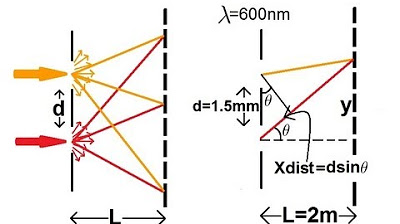
Physics 60 Interference of Light (4 of 8) Young's Double Slit

Waves part 2 (Double Slit, Single Slit, and Standing Waves)

Physics - Diffraction of Light (1 of 4) The Thin Slit

Physics 60 Interference of Light (8 of 8) Glass Plates

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Light InterferenceWavelengthPhase DifferenceConstructiveDestructiveElectromagnetic WavesVector AdditionCosine LawIntensity CalculationOnline LecturePhysics Education