Product and Quotient Rules

RH Mathematics

9 Sept 202121:21

EducationalLearning

32 Likes 10 Comments

TLDRThis video explains the product and quotient rules for derivatives in calculus. It clarifies that the derivative of a product is not simply the product of the derivatives and demonstrates the correct approach with examples. The quotient rule is also detailed, showing how to find derivatives of functions divided by each other. The video includes practice problems and emphasizes the importance of understanding these rules for AP Calculus.

Takeaways

📚 The video introduces the product and quotient rules for differentiation in calculus.
❌ You cannot simply multiply the derivatives of each factor to find the derivative of a product; this misconception is debunked early in the video.
📈 The product rule is demonstrated with an example involving the derivative of a polynomial resulting from the product of two linear terms.
🔄 The product rule states that the derivative of a product of two functions is the derivative of one times the other, plus the other's derivative times the first.
📉 The video explains that the derivative of a quotient is found using a modification of the quotient rule, emphasizing the need to remember it in terms of 'top' and 'bottom' rather than 'f' and 'g'.
📚 Examples are worked through to illustrate the application of the product and quotient rules, including finding the equation of a normal line to a curve at a given point.
📝 The importance of correctly applying the quotient rule is highlighted, with an example from an AP exam where many students struggled.
🔢 The video provides algebraic examples for practice, emphasizing the need to rewrite expressions like square roots and square terms correctly before differentiation.
📉 The derivative of tangent is given as secant squared, and the video offers a proof to validate this rule, encouraging students to understand the reasoning behind formulas.
📈 The derivative of secant is shown to be secant tangent, with an explanation that helps students remember the relationship between these trigonometric functions.
🔍 The video concludes with a set of drill problems for practice, emphasizing the need for repetition to master the product and quotient rules.

Q & A

What is the main topic of the video?
-The main topic of the video is the explanation of the product and quotient rules in calculus, including their applications and examples.
Why is it incorrect to assume that the derivative of a product is the product of the derivatives of each factor?
-It is incorrect because the derivative of a product of two functions involves both the derivative of one function times the other function, and the other function's derivative times the first function, not just the product of their derivatives.
What is the product rule in calculus and how is it applied?
-The product rule states that the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function. It is applied by multiplying and adding the derivative of each function while keeping the other function constant.
Can you provide an example of the product rule from the video?
-An example from the video is the derivative of (2x + 3)(x - 4), which is not simply the product of their derivatives but rather involves applying the product rule to get 4x - 5.
What is the quotient rule in calculus and how is it different from the product rule?
-The quotient rule states that the derivative of a quotient of two functions is the bottom function times the derivative of the top function minus the top function times the derivative of the bottom, all divided by the bottom function squared. It is different from the product rule as it involves a division of functions rather than multiplication.
How does the video explain the process of finding the equation of a normal line?
-The video explains that the slope of the normal line is the negative reciprocal of the derivative of the function at a given point. It then uses this slope and a given point to write the equation of the normal line.
What is the purpose of the table problems presented in the video?
-The table problems are used to demonstrate how to apply the product and quotient rules in practical situations, such as finding the derivative of a function at a specific point.
Can you explain the process of rewriting the square root as a power of one-half when taking derivatives?
-When taking the derivative of a square root, it is rewritten as the power of one-half to apply the power rule. For example, the derivative of √x is (1/2)x^(-1/2).
Why is it important to remember the derivative of tangent x and secant x in AP Calculus?
-It is important because these derivatives come up frequently in AP Calculus problems, and knowing them helps in solving a variety of calculus questions efficiently.
How does the video illustrate the derivative of secant x?
-The video illustrates the derivative of secant x by showing that the derivative is secant x times tangent x, using the quotient rule and trigonometric identities.
What is the significance of practicing the product and quotient rules multiple times as mentioned in the video?
-Practicing the product and quotient rules multiple times helps in mastering these fundamental calculus concepts, making them second nature and improving problem-solving speed and accuracy.

Outlines

00:00

📚 Introduction to Product and Quotient Rules

The video begins by posing a question about the incorrect assumption that the derivative of a product can be found by simply multiplying the derivatives of each factor. The instructor clarifies that this is not true, using the example of the derivative of (2x + 3)(x - 4), which expands to a quadratic polynomial. The product rule is introduced, explaining that it applies to the derivative of two functions multiplied together, not a constant and a function. The rule states that the derivative of a product is the derivative of the first function times the second function, plus the first function times the derivative of the second function. Examples are provided, including finding the equation of a normal line and calculating derivatives at specific points.

05:03

🔍 Working Through Product and Quotient Rule Examples

The script continues with a series of examples to illustrate the application of the product and quotient rules. It includes a table problem where the derivative of a function at a specific point is calculated, as well as algebraic examples that require rewriting expressions and applying the rules correctly. The instructor emphasizes the importance of practice and provides a couple of problems for viewers to try on their own, followed by the solutions.

10:04

📈 Exploring the Quotient Rule and Trigonometric Identities

The video script delves into the quotient rule, explaining its formula and application with examples, including a table problem and an AP exam question. The instructor also introduces new derivative rules for tangent and secant functions, providing a brief explanation of why these rules are true and emphasizing their importance in AP calculus. The script includes a step-by-step demonstration of deriving the formula for the derivative of secant.

15:06

📝 Advanced Quotient Rule Examples and Horizontal Tangent Discussion

This section presents more complex examples of applying the quotient rule, including finding the instantaneous rate of change at a specific point and solving for where the slope of the tangent is zero, indicating a horizontal tangent. The instructor also discusses the importance of correctly applying the quotient rule and the conditions under which the original function is undefined, which is crucial for understanding where horizontal tangents can exist.

20:09

📚 Final Practice Problems and a Note on Exponential Functions

The final part of the script offers a set of practice problems for the viewer to solve, focusing on the application of the quotient rule and the derivatives of exponential functions. The instructor reminds viewers of the importance of distinguishing between e^(x^2) and (e^x)^2, as they are not the same. The emphasis is on the need for repeated practice to internalize the product and quotient rules.

Mindmap

Keywords

💡Derivative

The derivative is a fundamental concept in calculus representing the rate at which a function changes at a given point. It is the slope of the tangent line to the function at that point. In the video, the derivative is used to analyze the behavior of various mathematical functions, such as when finding the slope of a tangent or normal line to a curve.

💡Product Rule

The product rule is a formula in calculus for finding the derivative of a product of two functions. It states that the derivative of two functions multiplied together is the derivative of the first times the second, plus the first times the derivative of the second. The video script explains that simply multiplying the derivatives of each factor does not yield the correct result, as demonstrated with the example of the derivative of (2x + 3)(x - 4).

💡Quotient Rule

The quotient rule is a derivative rule used to find the derivative of a quotient of two functions. It is expressed as the bottom function times the derivative of the top function minus the top function times the derivative of the bottom function, all divided by the bottom function squared. The video emphasizes the importance of this rule and provides examples of its application, such as finding the derivative of a function given in a table.

💡Normal Line

A normal line is a line that is perpendicular to the tangent line of a curve at a particular point. The video discusses the concept of the normal line in the context of finding the slope of such a line, which is the negative reciprocal of the derivative of the function at that point.

💡Tangent Line

A tangent line is a straight line that touches a curve at a single point without crossing it. In the video, the slope of the tangent line is found by taking the derivative of the function at a specific point, which is crucial for understanding the instantaneous rate of change of the function.

💡Power Rule

The power rule is a basic derivative rule in calculus that states the derivative of x raised to the power of n is n times x to the power of n-1. The video mentions the power rule when discussing the derivative of a polynomial function, such as 2x^2 - 5x - 12.

💡Distributive Property

The distributive property is a fundamental algebraic principle that allows for the multiplication of a term by a sum, by multiplying the term by each addend and then summing the results. In the script, the property is used when expanding the expression (2x + 3)(x - 4).

💡Algebraic Examples

Algebraic examples in the context of the video refer to problems that involve applying calculus rules, such as the product and quotient rules, to algebraic expressions. The script provides several algebraic examples to illustrate the application of these rules in finding derivatives.

💡Trigonometric Functions

Trigonometric functions, such as sine and cosine, are periodic functions that relate angles to the ratios of the sides of a right triangle. The video discusses the derivatives of trigonometric functions, particularly in the context of the product rule, where the derivative of sine squared x is explored.

💡Chain Rule

The chain rule is a fundamental theorem in calculus for finding the derivative of a composite function. Although not explicitly detailed in the script, the concept is alluded to when discussing the derivative of a function that involves another function, such as the derivative of sine squared x rewritten as a product of sine x and sine x.

💡Horizontal Tangent

A horizontal tangent to a curve is a tangent line with a slope of zero, indicating that the function has a horizontal line of tangency at that point. The video explains how to find the points of horizontal tangency by setting the derivative of the function equal to zero and solving for the variable.

Highlights

Introduction to the product and quotient rules in calculus.

Debunking the misconception that the derivative of a product can be found by simply multiplying the derivatives of each factor.

Explanation of why the derivative of a product results in a second-degree polynomial, using the example of (2x + 3)(x - 4).

Demonstration of the product rule with the function f(x) = (2x + 3)(x - 4) and its derivative.

Clarification that the product rule applies to the derivative of two functions involving x, not a constant times a function.

Application of the product rule to find the equation of a normal line at a given point.

Derivation of the slope of a normal line using the derivative of y with respect to x.

Example of using the product rule to find h'(x) for h(x) = x^2 * f(x) and calculating h'(5).

Emphasis on practicing algebraic examples to master the product rule.

Instruction to rewrite square roots as one-half powers and apply the product rule.

Explanation of how to handle the derivative of sine squared x by rewriting it as a product of sine x.

Introduction to the quotient rule and its formula.

Advice against memorizing the quotient rule formula in terms of f and g, but rather in terms of top and bottom for better understanding.

Use of the quotient rule to solve a table problem involving p(x) = g(x) / f(x) and finding p'(1).

Discussion of the AP exam and common mistakes made by students when applying the quotient rule.

Derivation of the derivative of tangent x as secant squared x and the importance of this formula in AP calculus.

Demonstration of the process to find the derivative of secant x and its result as secant x times tangent x.

Presentation of additional algebraic quotient rule examples for practice.

Explanation of how to find horizontal tangents by setting the derivative equal to zero and solving for x.

Final set of drill problems for practice with the product and quotient rules.

Note on the difference between e to the x squared and e to the 2x, emphasizing the importance of understanding exponent rules.

Transcripts

Browse More Related Video

AP Calculus AB: Lesson 3.2 The Product Rule

Business Calculus - Math 1329 - Section 2.3 - Product and Quotient Rules; Higher-order Derivatives

Derivatives of Trigonometric Functions

Derivatives of Polynomial Functions: Power Rule, Product Rule, and Quotient Rule

AP Calculus AB and BC Unit 2 Review [Differentiation: Definition and Basic Derivative Rules]

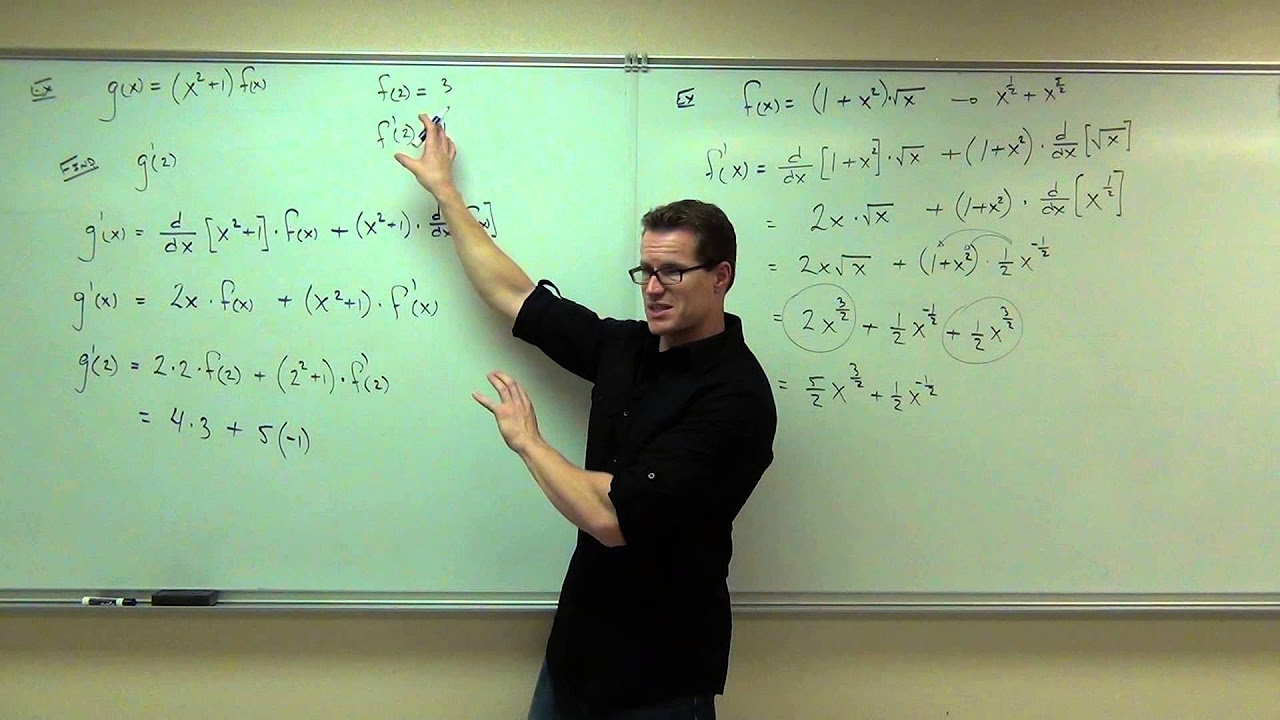
Calculus 1 Lecture 2.3: The Product and Quotient Rules for Derivatives of Functions

Related Tags

Calculus Product Rule Quotient Rule Derivative Trigonometry Tangent Secant Algebra AP Calculus Educational Learning