2019 Multiple choices 10

MATH MATTERS
15 May 202103:42
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers an in-depth review of the 2019 AP Calculus Multiple Choice problems, providing a comprehensive analysis of the questions and strategies for tackling them. It is an essential resource for students preparing for the AP exam, highlighting key concepts and offering insights into problem-solving techniques. The summary aims to engage viewers by distilling the essence of the script, making complex calculus topics accessible and intriguing.

Takeaways
  • 📘 Understand the fundamental theorem of calculus and its applications.
  • 🧮 Master techniques for differentiation, including the chain rule, product rule, and quotient rule.
  • 📈 Practice interpreting and sketching graphs of functions, especially with respect to limits and continuity.
  • 🔢 Get comfortable with various integration techniques, such as substitution and integration by parts.
  • 📊 Analyze and solve problems involving rates of change in the context of real-world applications.
  • ✏️ Review the concepts of sequences and series, focusing on convergence tests.
  • 📝 Be proficient in solving optimization problems using calculus principles.
  • 📐 Ensure understanding of parametric, polar, and vector functions.
  • 🧑‍🏫 Study and practice problems on the application of derivatives to understand motion, including velocity and acceleration.
  • 📚 Familiarize yourself with past AP Calculus multiple-choice questions to identify common themes and problem types.
Q & A

  • What is the structure of the 2019 AP Calculus exam?

    -The 2019 AP Calculus exam consists of two sections: a multiple-choice section and a free-response section. The multiple-choice section has 45 questions to be completed in 105 minutes, divided into two parts: one allowing the use of a calculator and one not.

  • What strategy is recommended for approaching the multiple-choice questions?

    -A recommended strategy is to first quickly go through all the questions, answering the ones you find easiest, and marking the more challenging ones for review. This ensures that you maximize your score by securing all the points you can confidently achieve first.

  • How can you identify and avoid common pitfalls in calculus problems?

    -Common pitfalls include misreading the problem, making algebraic errors, and incorrectly applying calculus principles. To avoid these, carefully read each problem, double-check your calculations, and review fundamental calculus concepts regularly.

  • What is a good approach to solving limits problems on the exam?

    -For limits problems, first try direct substitution. If that doesn’t work, consider factoring, rationalizing, or using L'Hopital's Rule. Always check for continuity and the behavior of the function as it approaches the limit.

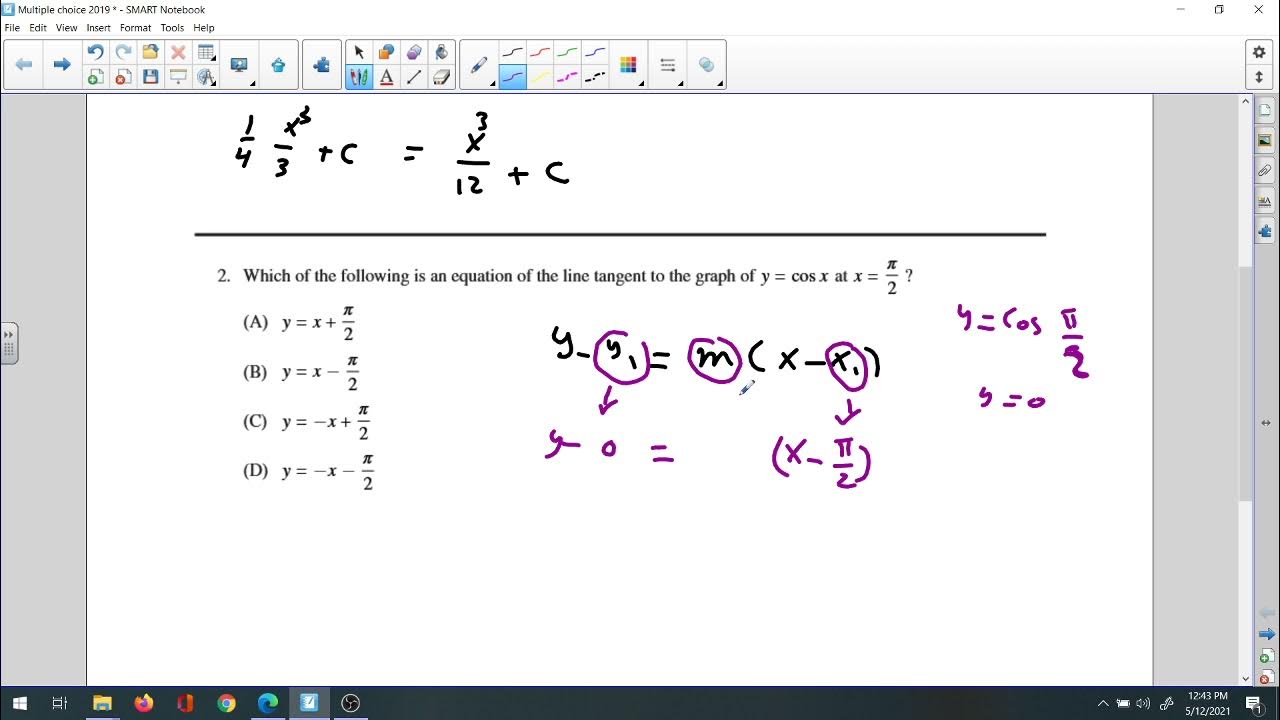
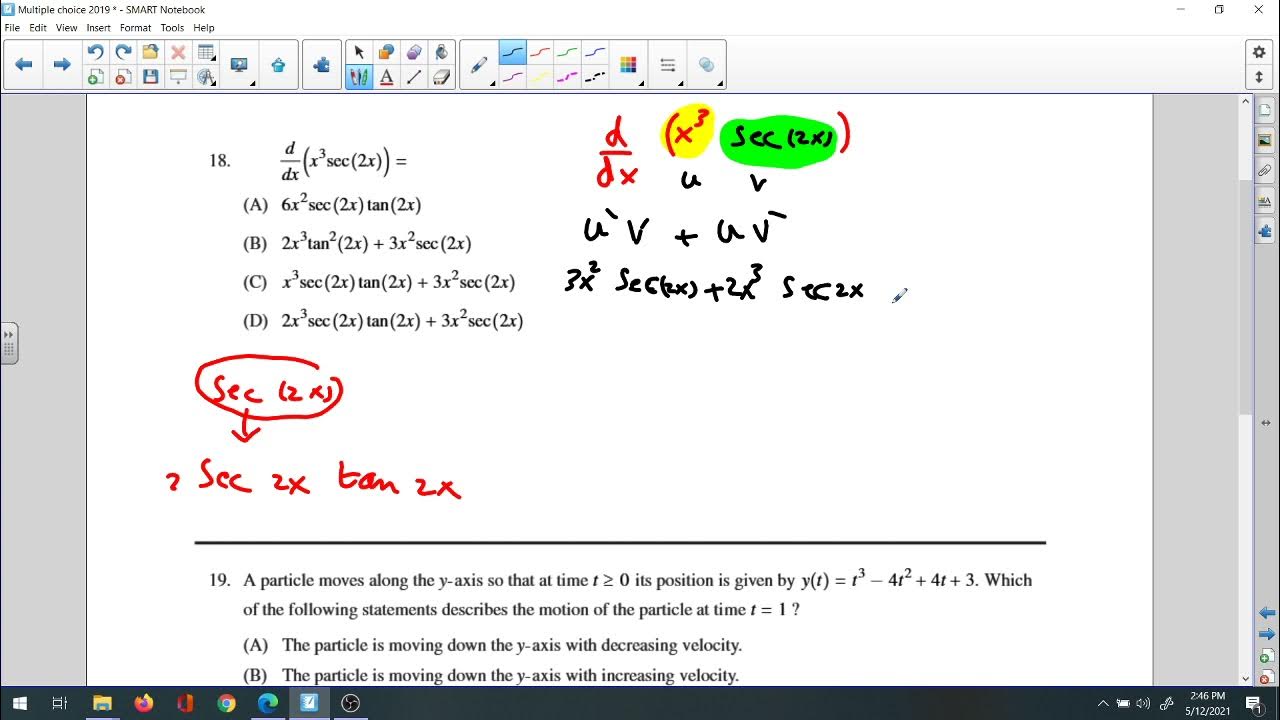
  • How should you handle problems involving derivatives?

    -For derivative problems, start by clearly identifying the function and applying the appropriate differentiation rules (product rule, quotient rule, chain rule). Simplify your result and interpret it within the context of the problem.

  • What techniques are useful for solving integration problems?

    -Useful techniques for integration problems include substitution, integration by parts, and recognizing common integral forms. Practice different techniques to become proficient in identifying the best approach for each problem.

  • Why is time management crucial during the AP Calculus exam?

    -Time management is crucial because it ensures you have enough time to address all questions, review your answers, and make necessary corrections. Proper time management can significantly improve your overall score.

  • What tips can help improve accuracy on the multiple-choice section?

    -To improve accuracy, read each question carefully, avoid rushing, eliminate obviously incorrect answers, and double-check your calculations. Additionally, practice with past exams to become familiar with the question format.

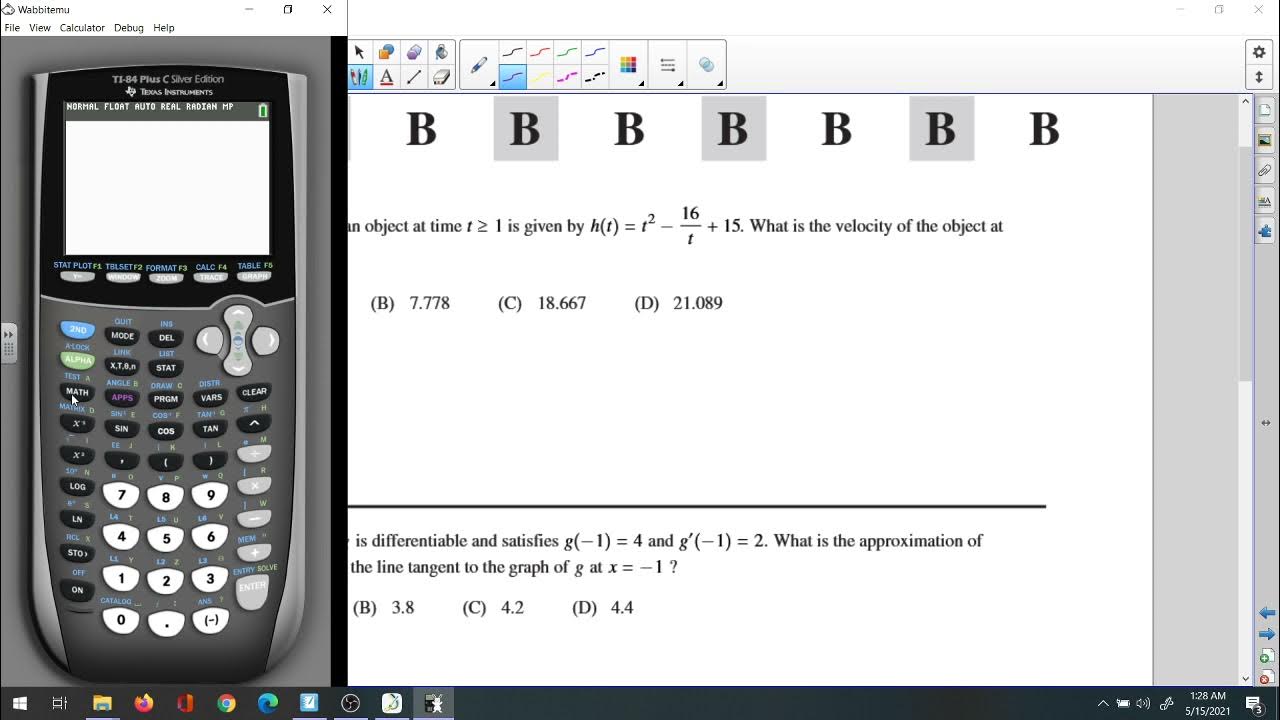
  • How should you prepare for the calculator-allowed section of the exam?

    -For the calculator-allowed section, ensure your calculator is functioning properly and that you are familiar with its features. Practice solving problems that specifically require a calculator to build your proficiency.

  • What are some effective study strategies for the AP Calculus exam?

    -Effective study strategies include practicing with past exam questions, focusing on areas where you struggle, reviewing fundamental concepts, and seeking help from teachers or study groups. Regular, consistent practice is key to success.

Outlines
00:00
📚 Review of 2019 AP Calculus Multiple Choice Questions

This paragraph introduces the topic of reviewing multiple choice questions from the 2019 AP Calculus exam. It sets the stage for a detailed analysis and discussion of the problems, possibly including strategies for tackling similar questions in the future.

Mindmap
Keywords
💡AP Calculus
AP Calculus is an advanced placement course and examination offered by the College Board for high school students. It is designed to be equivalent to a college-level calculus course and covers topics such as limits, derivatives, integrals, and infinite series. In the context of the video, AP Calculus is the main theme, as it discusses multiple-choice problems from the 2019 AP Calculus exam, which are central to the educational content being reviewed.
💡Multiple Choice
Multiple choice is a common format for assessments, where respondents are given a question and a set of possible answers, from which they must select the correct one. In the video, the focus is on multiple-choice problems from the AP Calculus exam, which requires test-takers to have a deep understanding of calculus concepts to make the right selection.
💡Limits
Limits are a fundamental concept in calculus, describing the behavior of a function as its input approaches a certain value. The video likely discusses how to evaluate limits and their significance in solving calculus problems, as they are a core topic in AP Calculus.
💡Derivatives
Derivatives represent the rate at which a function changes. They are central to the study of motion and optimization problems. The video script may include examples of derivative calculations and their applications in solving multiple-choice questions.
💡Integrals
Integrals are used to calculate the accumulated quantity of a variable and are the inverse operation of differentiation. The script likely explains how to compute integrals and their relevance in solving area, volume, and work problems in the context of the AP Calculus exam.
💡Infinite Series
Infinite series are sums of infinitely many terms. They are a key concept in calculus, especially in understanding convergence and divergence. The video might provide insights into how to determine whether a series converges and how to apply this knowledge in exam questions.
💡Function
A function is a mathematical relationship between two sets, where each element from the first set is paired with exactly one element of the second set. In the video, functions are the basis for exploring calculus concepts such as limits, derivatives, and integrals.
💡Continuity
Continuity in calculus refers to a function's property where it is defined at every point in its domain and its graph does not have any breaks or jumps. The video may discuss continuity as it relates to the behavior of functions and solving related problems.
💡Differentiation
Differentiation is the process of finding the derivative of a function, which is a measure of how a function changes as its input changes. The video script probably includes techniques for differentiation and how they are applied to multiple-choice problems.
💡Optimization
Optimization problems involve finding the maximum or minimum values of a function, which is often done using derivatives. The video might explain how to set up and solve optimization problems in the context of the AP Calculus exam.
💡Volume
Calculating volume using integrals is a common application in calculus, especially for solids of revolution. The video script may provide examples of how to use integration to find the volume of various shapes, which is a typical type of question on the AP Calculus exam.
💡Rate of Change
The rate of change is a concept closely tied to derivatives and is used to describe how quickly a quantity is changing at any given moment. The video likely discusses how to calculate rates of change and their importance in understanding the dynamics of various phenomena.
Highlights

Differentiation techniques and their applications

Integration strategies, including substitution and by parts

Fundamental Theorem of Calculus and its practical uses

Limits and continuity problems with step-by-step solutions

Series convergence tests and their applications

Applications of derivatives, including optimization and motion problems

Implicit differentiation and related rates problems

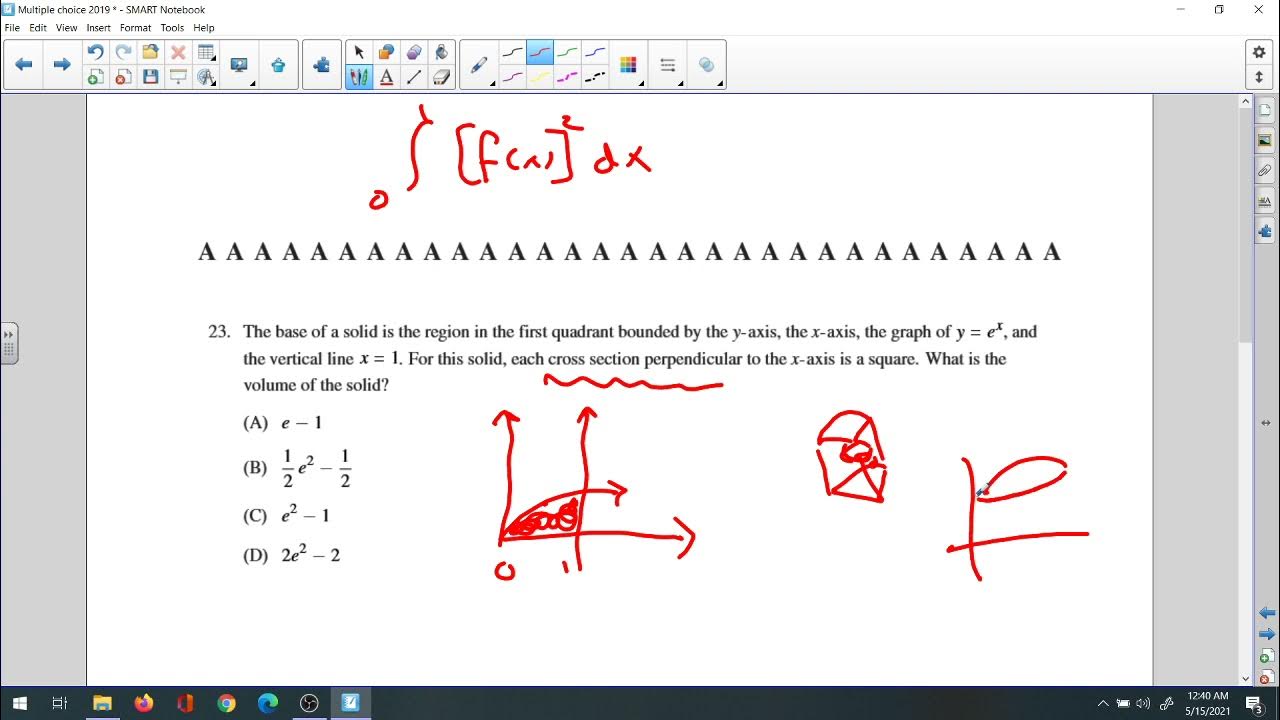
Volume calculations using the disk and washer methods

Understanding and solving problems involving parametric equations

Techniques for solving differential equations

Polar coordinates and their applications in calculus

Multivariable functions and partial derivatives

Application of calculus in real-world contexts

Strategies for multiple-choice problem-solving in AP Calculus

Review of common mistakes and pitfalls in calculus problems

Transcripts

Browse More Related Video

2019 Multiple choices 1,2

2019 Multiple choices 18,19

2019 Multiple choices 87,88

2019 Multiple choices 76,77,78

2019 Multiple choices 81,82,83

2019 Multiple choices 22,23

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
AP CalculusMultiple Choice2019 ExamProblem SolvingEducationalMathematicsExam PrepCollege BoardCritical ThinkingTest Review