2019 Multiple choices 18,19
TLDRThis video script offers an in-depth review of the 2019 AP Calculus Multiple Choice problems, dissecting each question to uncover the underlying concepts and strategies for solving them. It serves as an invaluable resource for students preparing for the AP exam, providing clear explanations and insights into tackling calculus problems efficiently.
Takeaways
- 📚 The 2019 AP Calculus exam focused on a variety of topics including limits, derivatives, and integrals.
- 🔍 Multiple-choice questions tested students' understanding of fundamental calculus concepts and their applications.
- 📉 Free response sections were designed to assess in-depth problem-solving skills and analytical abilities.
- 📈 The exam included questions on optimization problems, which are key in real-world applications of calculus.
- 📝 Students were expected to demonstrate proficiency in both the mechanics and theory of calculus.
- 📊 The use of graphs and visual representations was emphasized to solve calculus problems.
- 🤔 Critical thinking was required for questions involving rate of change and accumulation.
- 📐 Geometry-related problems, such as arc length and surface area, were part of the exam.
- 🧩 The integration of various mathematical tools, like the Fundamental Theorem of Calculus, was tested.
- 📚 Reviewing past AP Calculus exams can help students identify patterns and prepare for future tests.
- 🔑 Memorization of formulas alone was not sufficient; the ability to apply them in different contexts was crucial.
Q & A
What is the primary focus of AP Calculus AB?
-AP Calculus AB focuses on the concepts of differential and integral calculus, with an emphasis on preparing students for college-level mathematics.
How does the AP Calculus exam typically assess students' understanding of derivatives?
-The AP Calculus exam assesses students' understanding of derivatives through multiple-choice questions and free-response questions that require students to find derivatives of functions and apply them to solve problems.
What is the Fundamental Theorem of Calculus and why is it important?
-The Fundamental Theorem of Calculus links the concept of the derivative to the concept of the integral, showing that differentiation and integration are essentially inverse processes. It is important because it provides a foundation for solving many calculus problems.
What is the difference between an open interval and a closed interval on the real number line?
-An open interval includes all the numbers between two given numbers but not the numbers themselves, while a closed interval includes all the numbers between two given numbers including the numbers themselves.
How can you determine the concavity of a function using calculus?
-The concavity of a function can be determined by analyzing the second derivative. If the second derivative is positive, the function is concave up, and if it is negative, the function is concave down.
What is the Chain Rule used for in calculus?
-The Chain Rule is used to find the derivative of a composite function. It allows you to differentiate the outer function and the inner function separately and then combine the results.
What is the limit of a function as x approaches a certain value?
-The limit of a function as x approaches a certain value is the value that the function approaches as x gets arbitrarily close to that value, but does not necessarily equal it.
What is the purpose of the Mean Value Theorem in calculus?
-The Mean Value Theorem states that if a function is continuous on a closed interval and differentiable on the open interval, then there exists at least one point where the derivative of the function equals the average rate of change of the function over that interval.
What is the difference between a continuous function and a discontinuous function?
-A continuous function is one where the limit of the function as x approaches a certain value is the same as the function's value at that point. A discontinuous function has points where the limit does not exist or is not equal to the function's value at that point.
How can you determine if a sequence converges or diverges?
-A sequence converges if the terms of the sequence get arbitrarily close to a specific value as the index goes to infinity. It diverges if the terms do not approach a finite value.
What is the relationship between the slope of the tangent line to a curve at a point and the derivative of the function at that point?
-The slope of the tangent line to a curve at a point is equal to the derivative of the function at that point. The derivative represents the instantaneous rate of change of the function at a given point.
Outlines
📚 AP Calculus Multiple Choice Review 2019
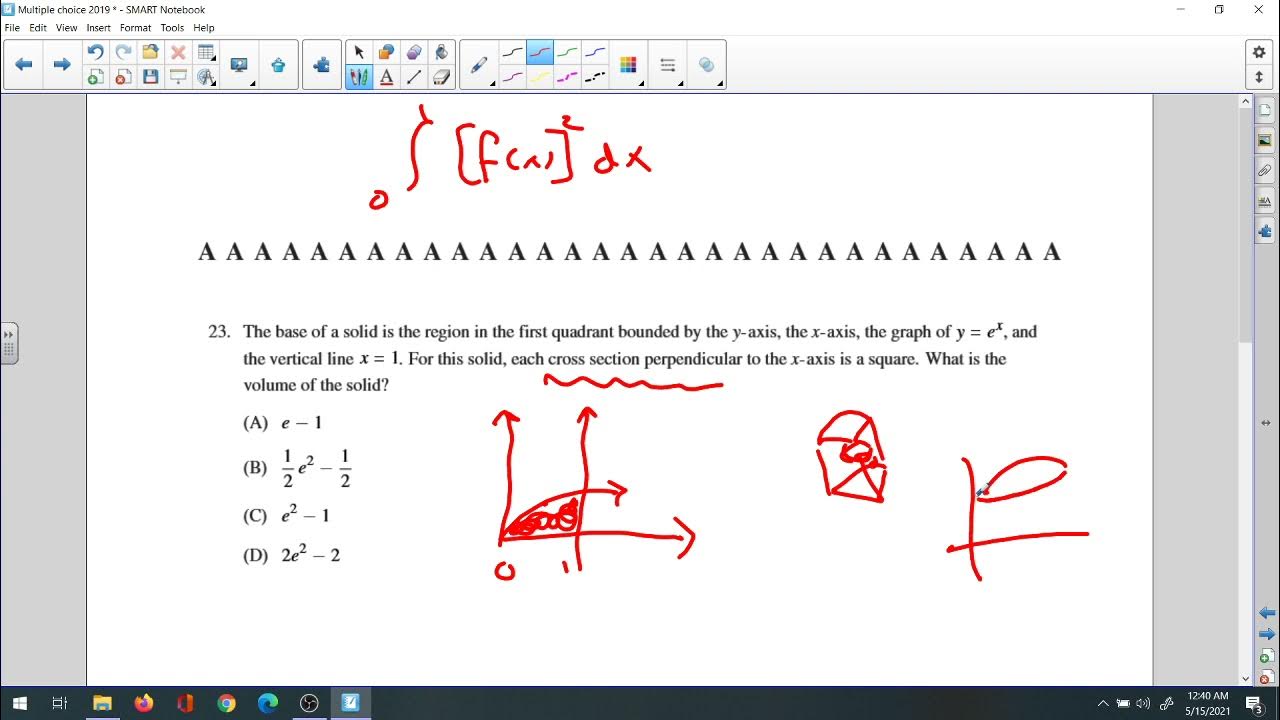
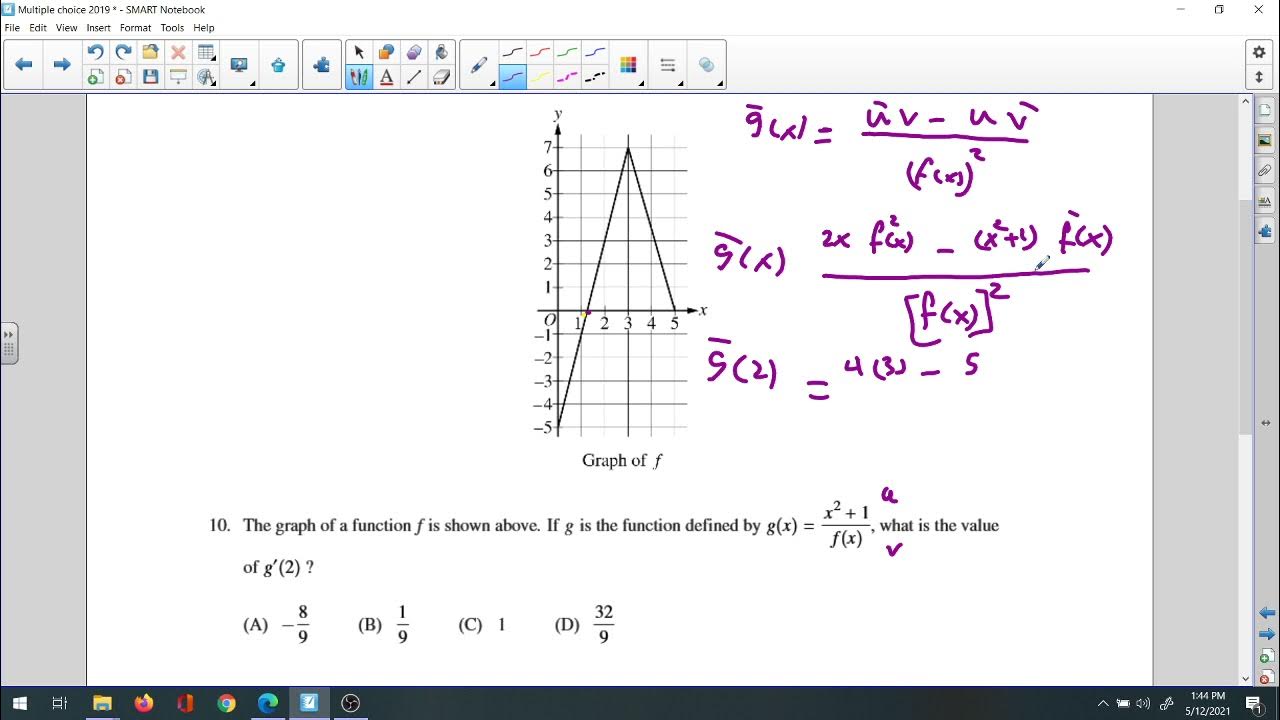
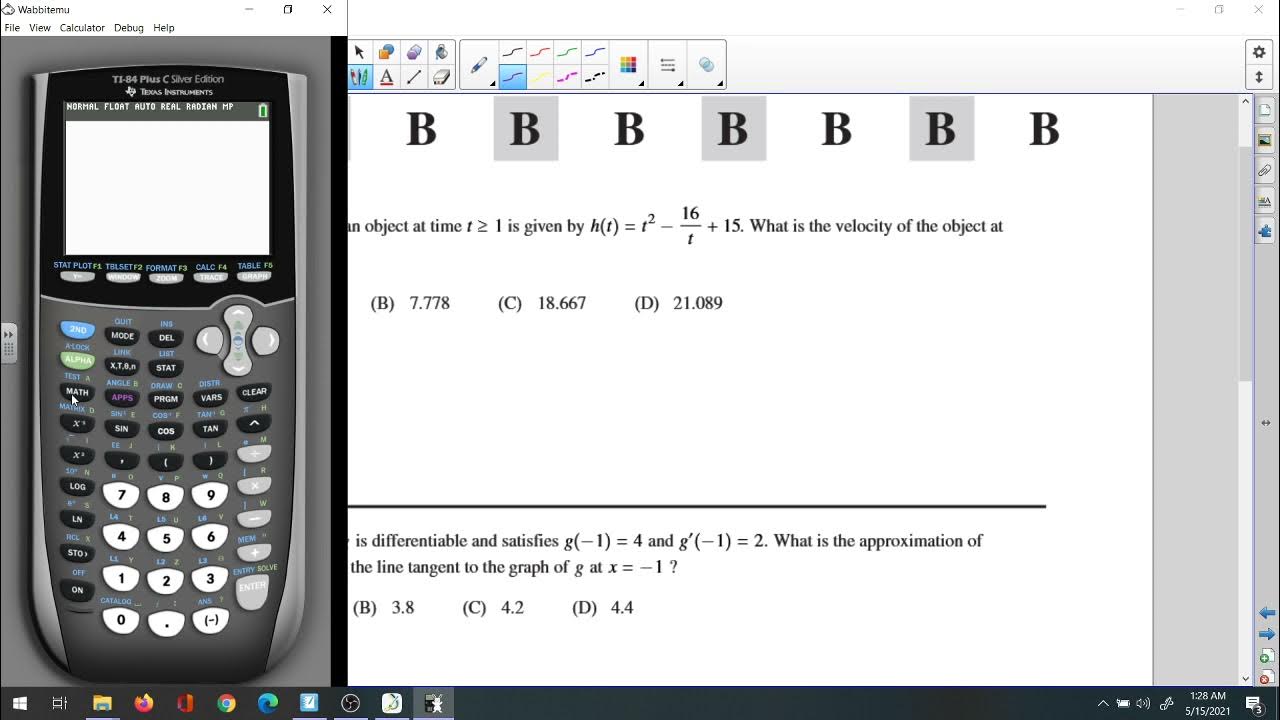
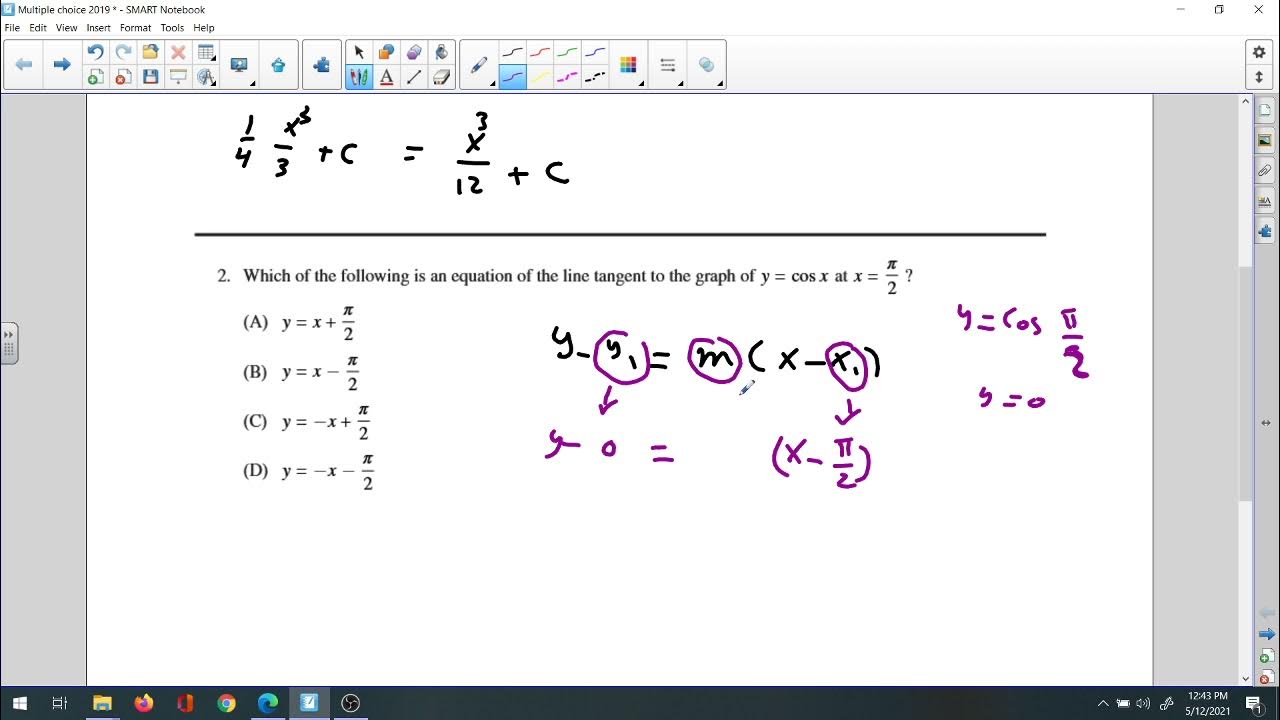
This paragraph introduces a review session focused on the multiple-choice problems from the AP Calculus exam in the year 2019. The review aims to help students understand and solve problems from the past exam, potentially preparing them for similar questions in future tests. The summary would include the purpose of the review, the type of problems covered, and the significance of practicing with past exam questions for better exam performance.
Mindmap
Keywords
💡AP Calculus
💡Multiple Choice
💡2019
💡Review
💡Problems
💡Limits
💡Derivatives
💡Integrals
💡Infinite Series
💡Test-taking Strategies
💡Educational Content
Highlights
Introduction to the 2019 AP Calculus exam structure and format.
Overview of key topics covered in the multiple-choice section.
Analysis of the most challenging problems and common student errors.
Explanation of innovative methods for solving complex integrals.
Discussion on the application of the Fundamental Theorem of Calculus in multiple-choice questions.
Strategies for approaching limit problems effectively.
Techniques for solving derivative-based questions quickly and accurately.
Insights into the use of graphical analysis in multiple-choice questions.
Tips for managing time during the exam to maximize scoring potential.
Comparison of 2019 exam difficulty with previous years.
Detailed walkthrough of a particularly difficult problem involving series and sequences.
Recommendations for additional resources and practice materials.
Discussion on the importance of understanding conceptual versus procedural knowledge.
Analysis of student performance data and common misconceptions.
Conclusion with key takeaways and advice for future test-takers.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: