Calculus BC – 10.2 Working with Geometric Series

The Algebros
31 Dec 202114:33
EducationalLearning
32 Likes 10 Comments

TLDRIn this calculus lesson, Mr. Bean explores geometric sequences and series, focusing on infinite geometric series. He reviews the concept of a geometric sequence, explains how to find the nth term, and introduces the formula for the sum of an infinite geometric series. The lesson demonstrates how to determine if a series converges and how to calculate its sum if it does, using examples and emphasizing the importance of the common ratio's absolute value being less than one for convergence. Mr. Bean also advises on using a calculator for complex series calculations without rounding intermediate steps.

Takeaways
  • 📚 The lesson is focused on geometric series, particularly infinite geometric series, and is a review for some students but may be new to others.
  • 🔢 A geometric sequence is defined by a number that is repeatedly multiplied by another number, known as the common ratio (r).
  • 🌟 The nth term of a geometric sequence can be found using the formula: first term * r^(n-1).
  • 👉 The script provides examples of how to determine the common ratio (r) and the first term of a geometric sequence from a given set of numbers.
  • 🔍 To find the nth term of a sequence, one can start with any term and adjust the exponent of r accordingly to match the position of the term.
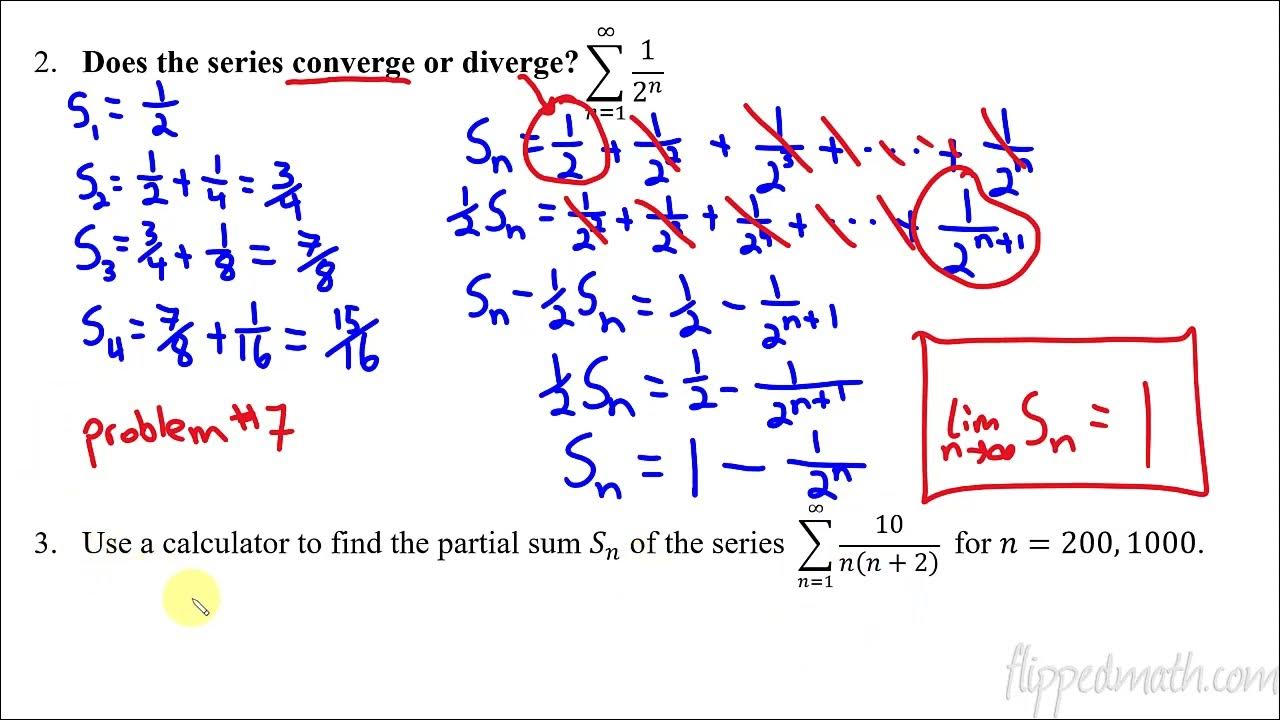
  • ∞ When dealing with infinite geometric series, the series converges if the absolute value of the common ratio (|r|) is less than one and diverges otherwise.
  • 📉 The formula to find the sum of a converging infinite geometric series is: a / (1 - r), where 'a' is the first term.
  • 📝 The script includes examples that demonstrate how to apply the formula for the sum of an infinite geometric series with various values of 'a' and 'r'.
  • 🔧 The importance of not rounding intermediate steps when calculating the sum of an infinite series is emphasized to avoid errors in the final answer.
  • 📱 The use of a calculator for complex calculations involving infinite series is discussed, with a demonstration of how to input and store values for accurate results.
  • ✅ The lesson concludes with a mastery check for students to test their understanding of geometric series and infinite geometric series.
Q & A

  • What is a geometric sequence?

    -A geometric sequence is a sequence of numbers where each term after the first is found by multiplying the previous one by a fixed, non-zero number called the common ratio.

  • How do you find the nth term of a geometric sequence?

    -The nth term of a geometric sequence can be found using the formula a * r^(n-1), where 'a' is the first term and 'r' is the common ratio.

  • What is the common ratio in the example where the sequence starts with 4 and each term is multiplied by 3?

    -In the given example, the common ratio 'r' is 3, as each term is obtained by multiplying the previous term by 3.

  • Can you have a geometric sequence with a common ratio of 1?

    -Yes, a geometric sequence can have a common ratio of 1, but it will result in all terms being the same as the first term, essentially creating a constant sequence.

  • What is an infinite geometric series?

    -An infinite geometric series is the sum of the terms of an infinite geometric sequence, provided that the absolute value of the common ratio is less than 1.

  • Under what condition does an infinite geometric series converge?

    -An infinite geometric series converges if the absolute value of the common ratio 'r' is less than 1.

  • What is the formula to find the sum of an infinite geometric series that has converged?

    -The sum of a converged infinite geometric series can be found using the formula a / (1 - r), where 'a' is the first term and 'r' is the common ratio.

  • How do you determine the common ratio 'r' if you know the sum of an infinite geometric series and the first term?

    -You can set up an equation with the sum of the series equal to the first term divided by (1 - r), then solve for 'r' by isolating it on one side of the equation.

  • Why is it important to avoid rounding intermediate steps when calculating the sum of an infinite geometric series?

    -Rounding intermediate steps can introduce errors that accumulate and affect the final result, so it's crucial to keep values unrounded until the final calculation.

  • Can a calculator be used to find the sum of an infinite geometric series?

    -Yes, a calculator can be used to assist in finding the sum of an infinite geometric series, especially for complex calculations, by inputting the values of 'a' and 'r' and performing the division (1 - r).

Outlines
00:00
📚 Introduction to Geometric Sequences and Series

This paragraph introduces the concept of geometric sequences and series with Mr. Bean as the instructor. It explains that a geometric sequence is a sequence of numbers where each term after the first is found by multiplying the previous one by a fixed, non-zero number called the common ratio (r). The paragraph provides an example starting with the number four and multiplying by three repeatedly to illustrate the concept. It also discusses different ways to represent the nth term of a geometric sequence, emphasizing the most common formula which involves the first term (a) and r raised to the power of (n-1). Additionally, it touches on how to find the nth term if the sequence starts with a term other than the first.

05:01
🔍 Understanding Infinite Geometric Series

The second paragraph delves into infinite geometric series, which are series where the number of terms extends to infinity. It explains that for an infinite geometric series to converge to a specific value, the common ratio (r) must have an absolute value less than one. If the absolute value of r is greater than or equal to one, the series diverges. The paragraph provides a formula for the sum of a convergent infinite geometric series, which is a over (1 - r), where 'a' is the first term and 'r' is the common ratio. It also includes examples of how to apply this formula to find the sum of specific series, emphasizing the importance of ensuring the series converges before using the formula.

10:02
🧮 Calculating Sums of Infinite Geometric Series

This paragraph continues the discussion on infinite geometric series, focusing on the process of calculating their sums. It provides a step-by-step approach to finding the sum of a series by identifying the first term (a) and the common ratio (r), and then applying the formula for the sum of a convergent series. The paragraph includes examples with different series, showing how to rewrite the series for clarity, calculate the first term, and use the formula to find the sum. It also discusses a scenario where the series does not converge due to the common ratio being greater than one, in which case no sum can be found. The paragraph concludes with a note on using a calculator to assist with these calculations, emphasizing the importance of not rounding intermediate steps to avoid errors in the final answer.

Mindmap
Keywords
💡Geometric Sequence
A geometric sequence is a sequence of numbers where each term after the first is found by multiplying the previous one by a fixed, non-zero number called the common ratio. In the video, the concept is introduced with an example starting with the number four and multiplying by three repeatedly to generate the sequence. The geometric sequence is central to understanding the video's theme of series and their sums.
💡Common Ratio (r)
The common ratio, denoted as 'r', is the factor by which we multiply each term in a geometric sequence to get the next term. In the script, Mr. Bean illustrates this with sequences where the common ratio is two or three-fourths, showing how it is used to generate subsequent terms in the sequence.
💡Geometric Series
A geometric series is the sum of the terms in a geometric sequence. The video explains that a geometric series can be finite or infinite, and the focus is on infinite geometric series, which are sums that extend indefinitely and have specific convergence properties.
💡Infinite Geometric Series
An infinite geometric series is a series where the number of terms approaches infinity. The video delves into the conditions under which such a series converges to a sum, highlighting that it converges if the absolute value of the common ratio is less than one.
💡Convergence
Convergence in the context of a series refers to the property of the series approaching a finite sum as the number of terms increases indefinitely. The video explains that an infinite geometric series converges if the absolute value of the common ratio is less than one, which is a key concept for determining the sum of the series.
💡Divergence
Divergence is the opposite of convergence; it occurs when the series does not approach a finite sum as the number of terms increases. In the video, it is mentioned that if the absolute value of the common ratio is greater than or equal to one, the series will diverge, meaning it does not have a sum.
💡nth Term
The nth term of a sequence refers to the term in a specific position 'n' in that sequence. The video provides formulas for finding the nth term of a geometric sequence, such as 'a * r^(n-1)', which is essential for understanding how to calculate any term in the sequence.
💡Sum of a Series
The sum of a series is the result of adding all the terms of that series together. The video discusses how to calculate the sum of an infinite geometric series when it converges, using the formula involving the first term and the common ratio.
💡Absolute Value
The absolute value of a number is its distance from zero on the number line, regardless of direction, and is used in the video to determine the convergence of an infinite geometric series. It is mentioned in the context of the common ratio's absolute value being less than one for the series to converge.
💡Calculator
A calculator is a device or software used to perform mathematical calculations. In the script, the use of a calculator is suggested for finding the sum of more complex infinite geometric series, emphasizing the importance of precision and avoiding rounding errors in intermediate steps.
Highlights

Introduction to the concept of geometric sequences and series by Mr. Bean.

Explanation of a geometric sequence as a sequence where each term is the product of the previous term and a fixed number, called the common ratio.

Illustration of a geometric sequence with an example starting with the number four and multiplying by three repeatedly.

Introduction of the nth term formula for a geometric sequence: a * r^(n-1).

Discussion on different starting points for a geometric sequence and the adjustment of the exponent in the nth term formula.

Demonstration of deriving a rule for a given sequence using the common ratio.

Calculation of the first term of a sequence using the example sequence 3, 6, 12, etc., with a common ratio of 2.

Explanation of how to find the zero term in a geometric sequence by working backwards.

Transition to the calculus portion of the lesson focusing on infinite geometric series.

Definition of an infinite geometric series and the condition for convergence based on the absolute value of the common ratio.

Presentation of the formula for the sum of a converging infinite geometric series: a / (1 - r).

Application of the convergence formula with an example series to find its sum.

Explanation of the process to determine the value of r that makes an infinite series converge to a specific number.

Use of a calculator to solve for r in an example where the series converges to 23.

Emphasis on the importance of not rounding intermediate steps when using a calculator to find the sum of an infinite series.

Conclusion of the lesson with a reminder to practice and master the concepts covered.

Transcripts

Browse More Related Video

Calculus BC – 10.1 Defining Convergent and Divergent Infinite Series

Ch. 12.3 Geometric Sequences

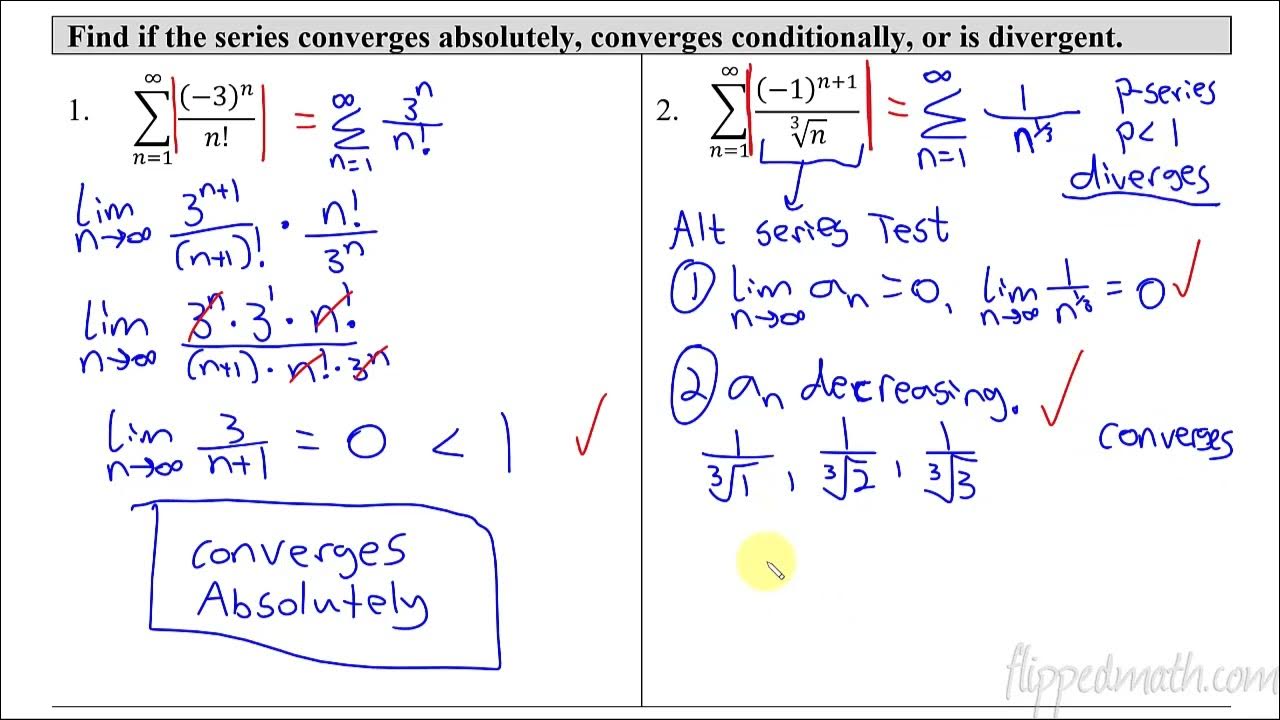
Calculus BC – 10.9 Determining Absolute or Conditional Convergence

Ratio test | Series | AP Calculus BC | Khan Academy

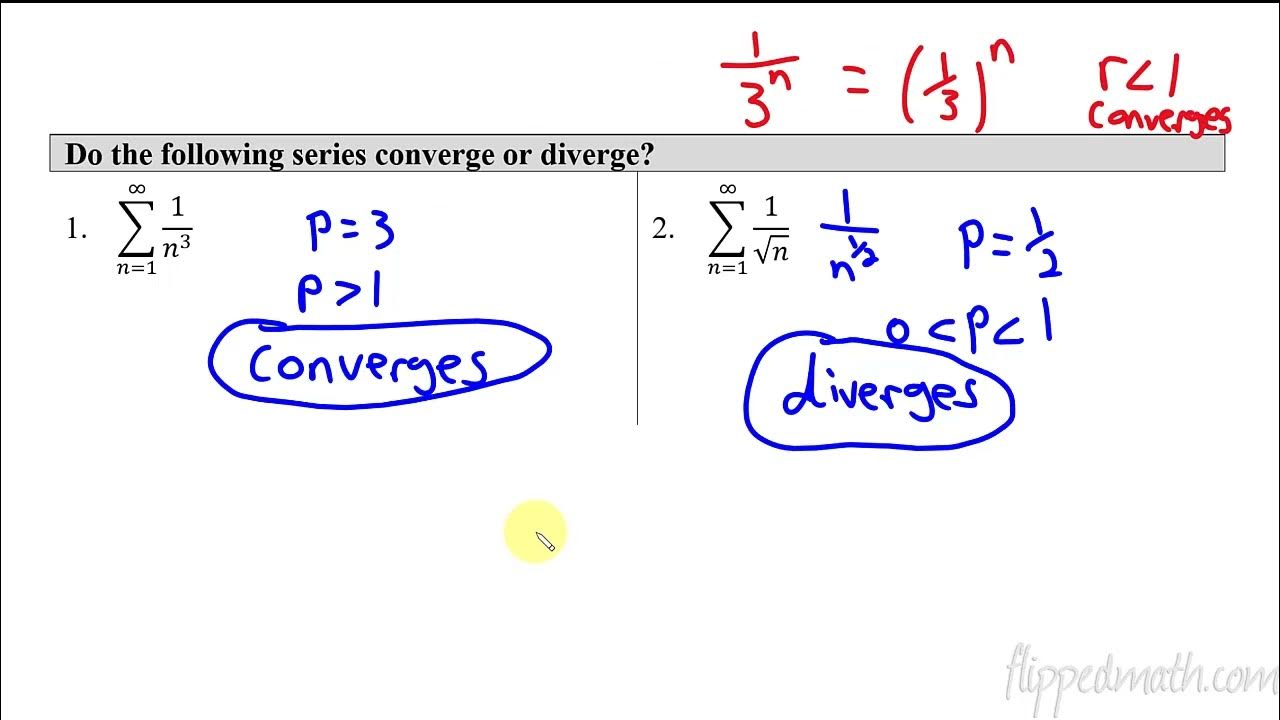
Calculus BC – 10.5 Harmonic Series and p-series

Geometric Series (Precalculus - College Algebra 72)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus LessonsInfinite SeriesGeometric SequencePre-CalculusAlgebra OneEducational ContentMathematics TutorialSeries ConvergenceCommon RatioMr. Bean