Calculus BC – 10.1 Defining Convergent and Divergent Infinite Series

The Algebros
28 Jan 202216:30
EducationalLearning
32 Likes 10 Comments

TLDRIn this calculus lesson, Mr. Bean introduces the concepts of convergent and divergent infinite series, essential for understanding sequences and series. He explains the difference between a sequence and a series, the importance of monotonic and bounded sequences, and how to determine if a series converges to a specific value or diverges. Through examples and a strategy for finding the sum of an infinite geometric series, Mr. Bean helps students grasp these complex topics, setting a solid foundation for further lessons.

Takeaways
  • 📚 The lesson focuses on understanding convergent and divergent infinite series, which are foundational for further calculus topics.
  • 🔍 The concept of a sequence is introduced as a rule for generating terms, with examples provided to illustrate how to calculate specific terms in a sequence.
  • 🔢 A monotonic sequence is defined as one that either consistently increases or decreases without reversal, whereas a bounded sequence has a maximum and/or minimum value.
  • 🌐 The difference between a sequence (a_n) and a series (S_n) is clarified, with a_n representing a single term and S_n representing the sum of the first n terms.
  • ∞ An infinite series is the sum of an infinite sequence of numbers, and its behavior can be diverging (going to infinity) or converging (approaching a specific value).
  • 📈 The method to determine if a series converges or diverges involves examining the partial sums and whether they approach a specific value as n approaches infinity.
  • 📝 A technique is demonstrated for finding the sum of an infinite geometric series by manipulating the series to find a pattern and then taking the limit as n approaches infinity.
  • 📉 The script includes an example of a series that diverges, showing that the partial sums increase without approaching a specific value, indicating the series does not converge.
  • 🧮 The use of a calculator to find the sum of the first n terms of a series is discussed, with a demonstration of how to input the series into a calculator for large n values.
  • 📚 The importance of understanding the concepts of sequences, series, and their convergence or divergence is emphasized for success in further calculus studies.
Q & A

  • What is the main topic of the calculus lesson presented by Mr. Bean?

    -The main topic of the lesson is convergent and divergent infinite series.

  • What does Mr. Bean suggest about the understanding of series and sequences for the lesson?

    -Mr. Bean suggests that having a good grasp of what series and sequences are is important for understanding the rest of the unit.

  • What is a sequence according to the script?

    -A sequence is a collection of numbers that are in one-to-one correspondence with positive integers.

  • What does 'monotonic' mean in the context of sequences?

    -In the context of sequences, 'monotonic' means that the sequence either never decreases or never increases.

  • What is a 'bounded' sequence as described in the script?

    -A 'bounded' sequence is one that has a maximum on the upper bound or a minimum on the lower bound, meaning the whole set of numbers in the sequence is contained within these bounds.

  • What is the difference between an infinite series and a partial sum according to the script?

    -An infinite series is the sum of an infinite sequence of numbers, while a partial sum is the sum of the first n terms of a sequence.

  • How does Mr. Bean explain the relationship between a_n and S_n?

    -Mr. Bean explains that a_n is an expression for the nth term in a sequence, while S_n is the sum of the first n terms of the sequence. The limit of S_n as n approaches infinity is equivalent to the infinite series sum.

  • What is the definition of a convergent series as presented in the script?

    -A convergent series is one where the sequence of partial sums approaches a specific number, meaning the series converges to that number.

  • How does Mr. Bean demonstrate the convergence of a series to the students?

    -Mr. Bean demonstrates the convergence of a series by showing the partial sums and how they approach a specific number, and also by providing a mathematical proof using the formula for S_n.

  • What is the strategy Mr. Bean uses to find the sum of an infinite geometric series?

    -Mr. Bean uses a strategy of writing out the series, multiplying the entire series by a common ratio, and then subtracting the two series to find a formula for S_n, which can then be used to determine the convergence of the series.

  • How does Mr. Bean use a calculator to find the sum of the first 200 terms of a series?

    -Mr. Bean uses the summation function on a calculator, inputs the formula for the nth term, sets the range from 1 to 200, and calculates the sum to estimate the convergence of the series.

  • What conclusion does Mr. Bean draw about the series that starts with 1, then 3, 6, 10, 15, etc.?

    -Mr. Bean concludes that the series is divergent because the partial sums are increasing without approaching a specific number.

Outlines
00:00
📚 Introduction to Convergent and Divergent Infinite Series

This paragraph introduces the topic of the video, which is the study of convergent and divergent infinite series, taught by Mr. Bean. It emphasizes the importance of understanding sequences and series as a foundation for the next 14 lessons in Unit 10 of calculus. The paragraph explains the concept of a sequence as a rule for generating terms associated with positive integers and introduces the idea of monotonic and bounded sequences. It also differentiates between the terms of a sequence (a_n) and the sum of the first n terms (S_n), providing an example to illustrate the difference.

05:01
🔍 Understanding Convergence and Divergence in Series

The second paragraph delves into the definitions of convergent and divergent series. It explains that an infinite series is the sum of an infinite sequence of numbers and that convergence occurs when the partial sums approach a specific number, while divergence happens when the partial sums do not approach any number. The paragraph provides a method to determine convergence or divergence by examining the behavior of partial sums and introduces the concept of finding the sum of an infinite series by taking the limit of S_n as n approaches infinity.

10:02
📘 Techniques for Analyzing Series Convergence

This paragraph demonstrates a technique for analyzing the convergence of a series by examining the partial sums and using a specific strategy to find the sum S_n. It illustrates this with an example series and shows how to manipulate the series to find a pattern or rule for S_n. The technique involves multiplying the series by a factor and subtracting it from the original series to simplify and find a closed-form expression for S_n. This method is particularly useful for series where it's difficult to determine convergence by simply examining the partial sums.

15:03
📱 Utilizing Calculators for Series Summation

The final paragraph discusses the practical application of calculators to determine the sum of a large number of terms in a series, which would be impractical to do by hand. It provides a step-by-step guide on how to use a calculator, specifically a TI-84, to sum the first 200 and then 1000 terms of a given series. The results are used to observe the behavior of the series and to estimate its convergence or divergence. The paragraph also includes an example of a divergent series to illustrate the concept and concludes with encouragement to practice these skills for mastery.

Mindmap
Keywords
💡Infinite Series
An infinite series is the sum of an infinite sequence of numbers. In the context of the video, it is a fundamental concept that the series either converges to a specific value or diverges, meaning it does not approach a finite sum. The script discusses how to determine if a series is convergent or divergent by examining the behavior of its partial sums.
💡Convergent
A convergent series is one where the sum of its terms approaches a finite value as more terms are added. The video explains that if the partial sums of a series get closer and closer to a specific number, the series is said to converge to that number, as illustrated by the example where the series appears to converge to one.
💡Divergent
A divergent series is one where the sum of its terms does not approach a finite value, but instead grows without bound. The script provides an example of a divergent series where the partial sums increase monotonically without converging to a specific number, indicating that the series is diverging.
💡Partial Sum
A partial sum refers to the sum of the first n terms of a sequence. The video emphasizes the importance of partial sums in determining whether an infinite series converges or diverges. The script demonstrates how to calculate partial sums and observe their behavior to make conclusions about the series.
💡Sequence
A sequence is an ordered list of numbers, often defined by a rule or formula. In the video, sequences are used to generate terms for series, and understanding sequences is crucial for studying series. The script provides an example of a sequence defined by a formula and how its terms are calculated.
💡Monotonic Sequence
A monotonic sequence is one that is either entirely non-increasing or non-decreasing. The video explains that if a sequence's terms either consistently get larger or smaller without changing direction, it is considered monotonic. This property is relevant when discussing the convergence of a series.
💡Bounded
A bounded sequence or series has an upper and/or lower limit; it does not grow indefinitely in any direction. The script mentions that a sequence is bounded if it has a maximum and minimum value, which is a property that can be relevant to determining the convergence of a series.
💡nth Term
The nth term of a sequence, denoted as a_n, is the term in the sequence at the nth position. The video script explains how to calculate the nth term using a formula and how it differs from the sum of the first n terms, which is a key distinction in series analysis.
💡Summation
Summation is the mathematical operation of adding a sequence of numbers to find their total. In the video, the concept of summation is used to describe the process of adding the terms of an infinite series. The script also shows how to use the summation formula to find the sum of a series.
💡Limit
In the context of calculus, a limit is the value that a function or sequence approaches as the input approaches some value. The video discusses the concept of limits in relation to infinite series, specifically how the limit of the partial sums as n approaches infinity can determine the convergence of a series.
💡Calculator
The video script mentions the use of a calculator to compute the sum of a large number of terms in a series, which would be impractical to do by hand. It provides an example of how to use a calculator to find the partial sums of a series, demonstrating a practical application of technology in mathematical analysis.
Highlights

Introduction to convergent and divergent infinite series by Mr. Bean.

Explanation of the importance of understanding sequences and series for the next 14 lessons in Unit 10.

Definition of a sequence as a rule for generating terms and its one-to-one correspondence with positive integers.

Illustration of how to determine the nth term of a sequence with a given formula.

Introduction of monotonic sequences that either never decrease or never increase.

Definition of a bounded sequence with a maximum or minimum value.

Concept of an infinite series as the sum of an infinite sequence of numbers.

Explanation of partial sums and their difference from the nth term of a sequence.

Demonstration of calculating the nth term and the sum of the first n terms with an example.

Clarification on the equivalence of summation of all terms of a sequence and the limit of partial sums as n approaches infinity.

Definition and distinction between convergent and divergent series based on the behavior of partial sums.

Method to determine if a series converges or diverges by examining the sequence of partial sums.

Strategy to find the sum of an infinite geometric series by manipulating the series expression.

Use of algebraic manipulation to derive a formula for the sum of the first n terms (s of n).

Proof that a specific series converges to one by taking the limit as n approaches infinity.

Application of a calculator to find the sum of the first 200 and 1000 terms of a series.

Observation that the series appears to be approaching 7.5 based on partial sums calculation.

Identification of a series that diverges by showing that its partial sums keep increasing without converging to a specific number.

Conclusion and encouragement for students to apply the concepts learned in the mastery check.

Transcripts

Browse More Related Video

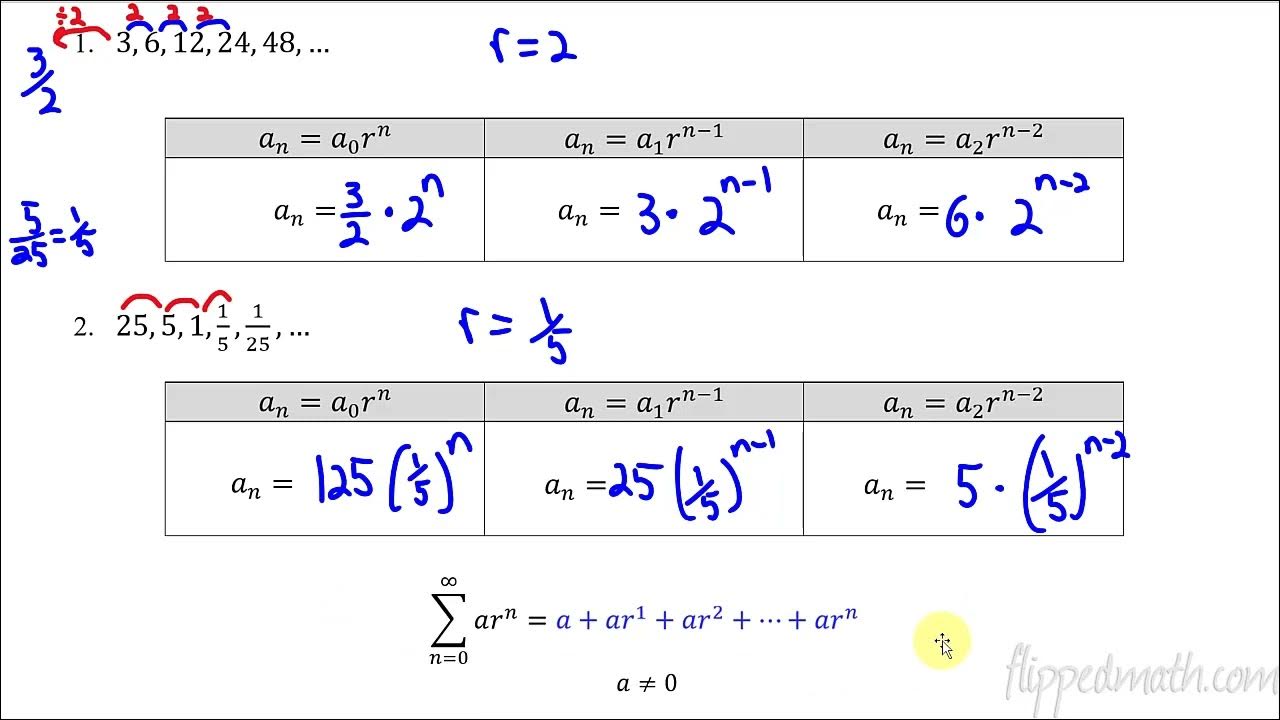
Calculus BC – 10.2 Working with Geometric Series

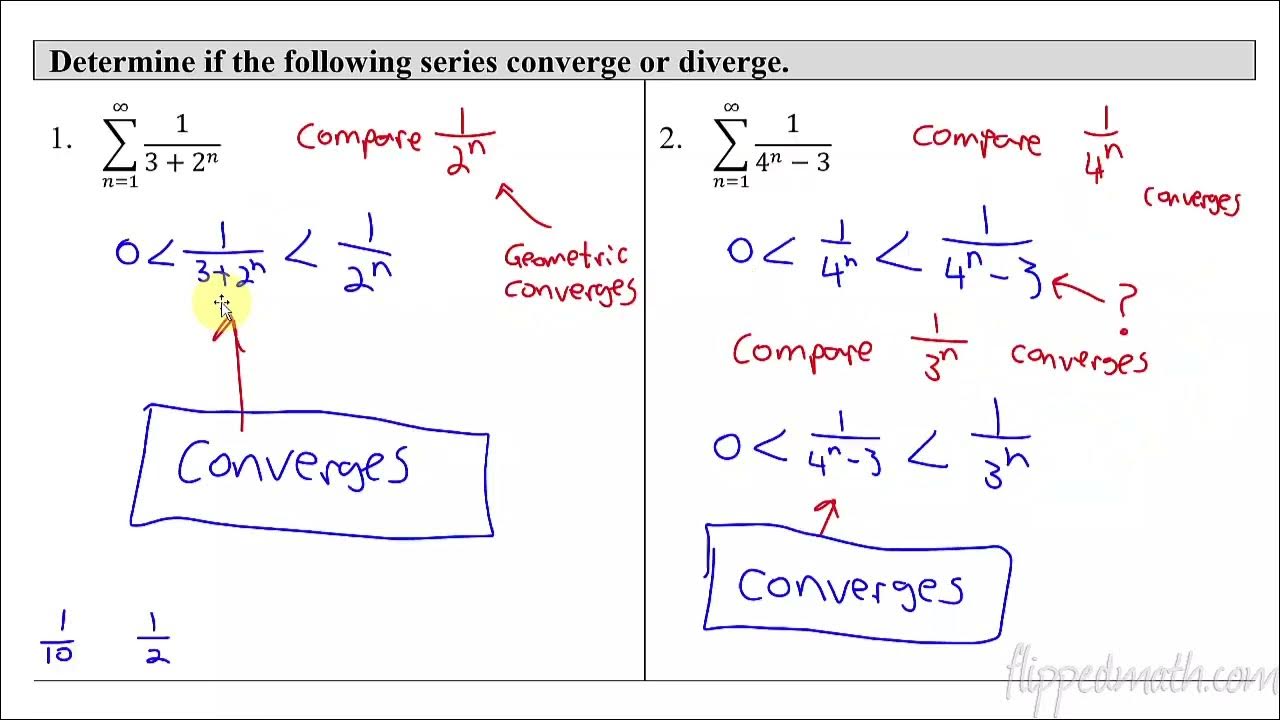
Calculus BC – 10.6 Comparison Tests for Convergence

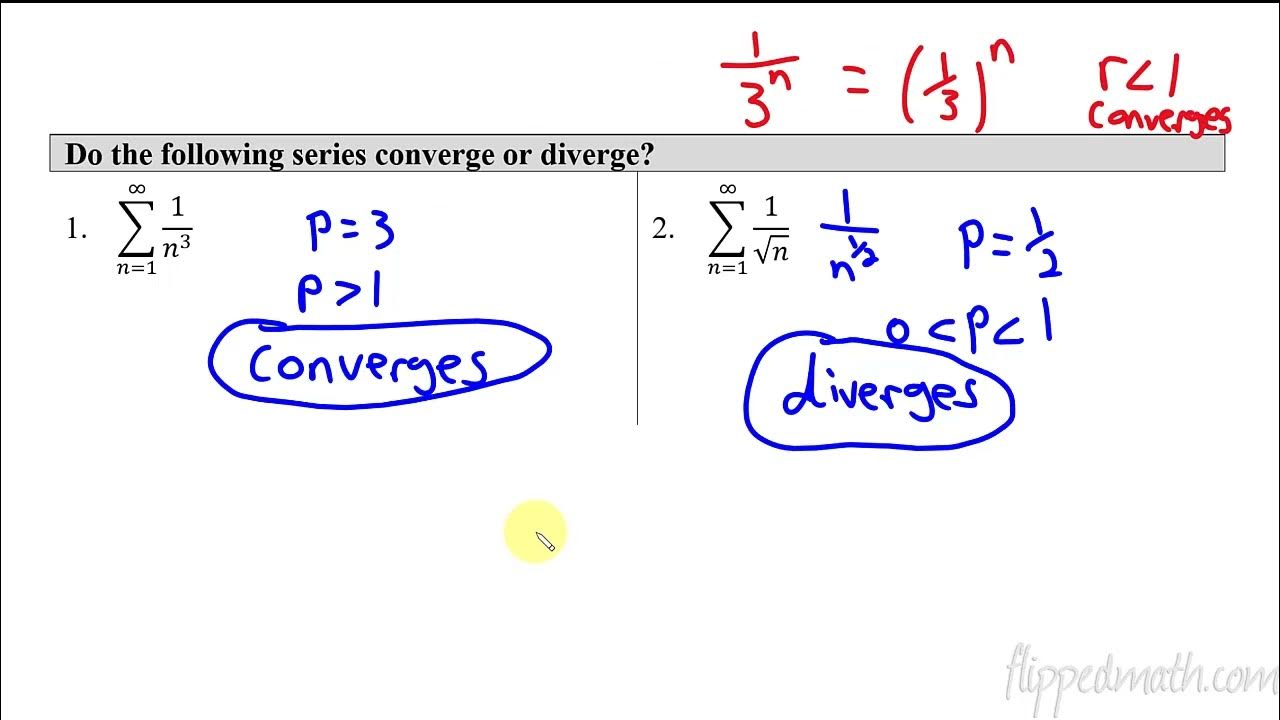
Calculus BC – 10.5 Harmonic Series and p-series

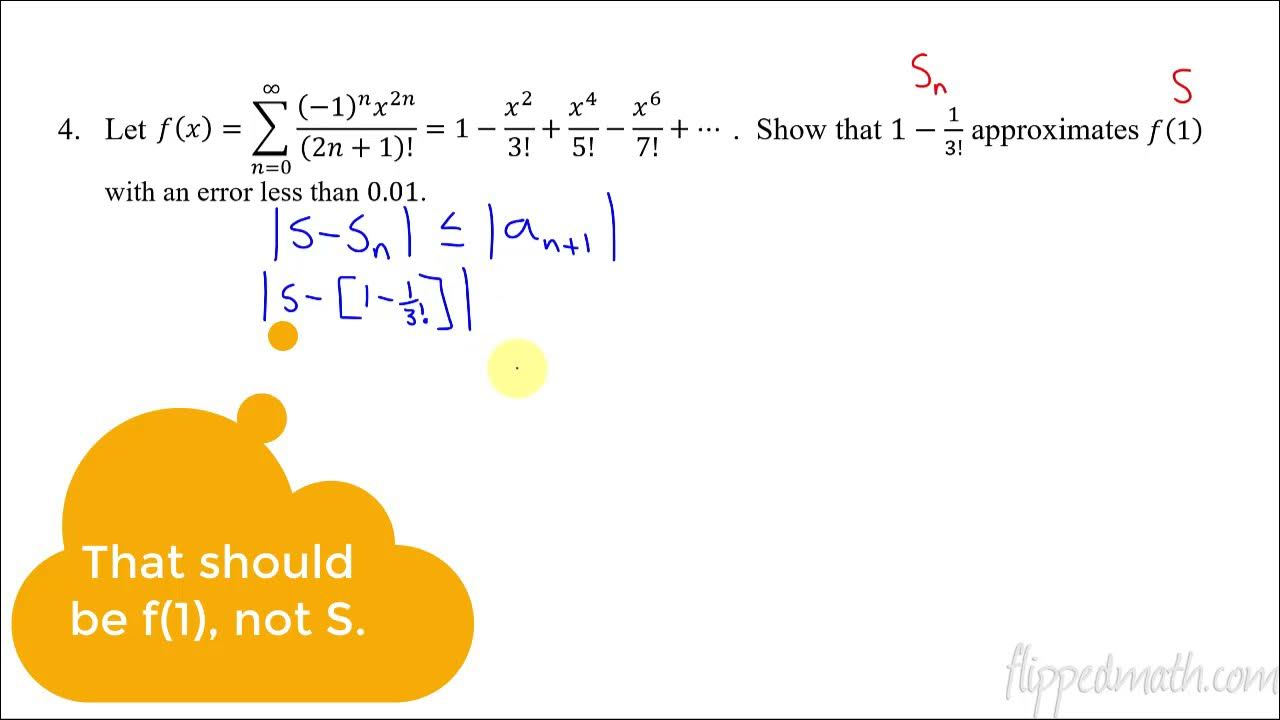
Calculus BC – 10.10 Alternating Series Error Bound

Calculus BC – 10.9 Determining Absolute or Conditional Convergence

Geometric Series (Precalculus - College Algebra 72)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus LessonsInfinite SeriesConvergentDivergentEducationalMathematicsAlgebraPre-CalculusSequencesPartial Sums