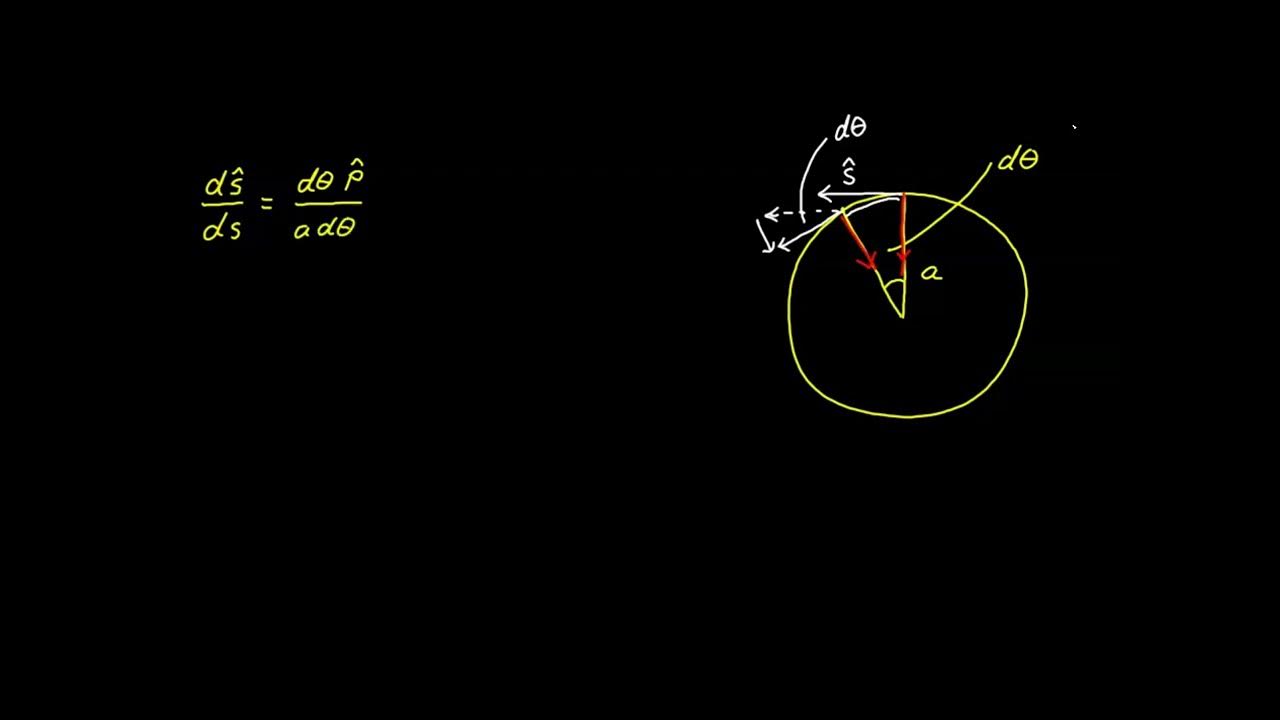Curvature of a Vector Function (Calculus 3)
TLDRIn this Houston Mathprep video, the concept of curvature for vector-valued functions is explored, explaining how it measures the rate of change in direction relative to arc length. The video discusses constant curvature in lines and circles, introduces the curvature formula involving the magnitude of the unit tangent's change over arc length, and uses the Greek letter kappa to denote curvature. It revisits an example from a previous video to demonstrate the calculation of curvature, including finding unit tangent and principal unit normal vectors. The video also works through a new problem involving a helix function, simplifying derivatives and magnitudes to find the curvature, which turns out to be a constant value.
Takeaways
- 📚 Curvature is a measure of how much the direction of a vector-valued function changes with respect to arc length.
- 🔍 Vector-valued functions with constant curvature include lines (curvature is zero) and circles (curvature is constant and depends on the radius).
- 🚗 The curvature of a circle is due to the constant steering required to maintain a circular path, resulting in a constant change in direction.
- 📉 The general formula for curvature is based on the magnitude of the change of the unit tangent vector with respect to arc length, often abbreviated as \( \kappa \).
- 🔄 Finding curvature involves calculating the magnitude of the derivative of the unit tangent vector (\( \hat{t}' \)) and the magnitude of the derivative of the position vector (\( r' \)).
- 📐 For a vector-valued function, the unit tangent vector is found by dividing the derivative of the position vector by its magnitude.
- 🌀 The principal unit normal vector is derived from the derivative of the unit tangent vector, which is part of the process for finding curvature.
- 📈 The magnitude of \( \hat{t}' \) is not necessarily one, unlike the unit tangent vector itself, and is crucial for calculating curvature.
- 📝 An example given in the script involves a vector-valued function of the form \( \langle t\cos(t) - \sin(t), t\sin(t) + \cos(t), 1 \rangle \), where the curvature is calculated step by step.
- 🧩 Simplification of derivatives in the example leads to a cleaner expression for \( r'(t) \), which is essential for finding the magnitude needed for curvature.
- 🔢 The final curvature calculation in the example results in a value of \( \frac{1}{t} \) (or \( \frac{1}{|t|} \) if considering negative \( t \)), highlighting the dependency of curvature on the parameter \( t \).
Q & A
What is the concept of curvature in the context of vector-valued functions?
-Curvature is a measure of how much the direction of a vector-valued function changes with respect to arc length. It indicates the rate of change in the tangent vector to the curve as one moves along the curve.
Why do lines have constant curvature zero?
-Lines have constant curvature zero because their tangent vector always points in the same direction, resulting in no change in the unit tangent vector, hence no curvature.
How is the curvature of a circle characterized?
-A circle has constant curvature because, regardless of where one starts on the circle, the unit tangent direction changes by a constant amount (2π) over the entire circumference (2πr).
What is the general formula for calculating the curvature of a vector-valued function?
-The general formula for curvature is based on the magnitude of the change of the unit tangent vector with respect to arc length, often represented as |t̂'| divided by |r'|.
What is the significance of the unit tangent vector in finding curvature?
-The unit tangent vector is crucial in finding curvature as it represents the direction of the curve at a given point, and its rate of change with respect to arc length directly influences the curvature.
What is the difference between t̂ and t̂' in the context of curvature?
-t̂ represents the unit tangent vector, which is the direction of the curve at a given point. t̂' is the derivative of the unit tangent vector, indicating how the direction of the curve is changing with respect to arc length.
Why is the magnitude of t̂' not always one?
-The magnitude of t̂' is not always one because t̂' represents the rate of change of the unit tangent vector, which can vary depending on the curve's geometry, whereas t̂ is always a unit vector (magnitude of one) by definition.
Can you provide an example of a vector-valued function with non-constant curvature?
-An example of a vector-valued function with non-constant curvature is given in the script: t*cos(t) - sin(t), t*sin(t) + cos(t), 1. The curvature of this function changes depending on the value of 't'.
What is the process for finding the principal unit normal vector in the context of curvature?
-The process involves finding the derivative of the unit tangent vector (t̂') and then calculating its magnitude. This magnitude is then used in the curvature formula to determine how the direction of the curve is changing.
How does the curvature formula relate to the unit tangent and principal unit normal vectors?
-The curvature formula directly uses the magnitude of the derivative of the unit tangent vector (t̂') and the magnitude of the derivative of the position vector (r'). These quantities are also involved in finding the unit tangent and principal unit normal vectors.
What is the curvature of the helix function given in the script?
-The curvature of the helix function is 3/10, as calculated by dividing the magnitude of t̂' (which is 3/root(10)) by the magnitude of r' (which is root(10)).
Outlines
📚 Introduction to Curvature of Vector-Valued Functions
This paragraph introduces the concept of curvature for vector-valued functions, explaining it as a measure of directional change with respect to arc length. It distinguishes between vector-valued functions with constant and variable curvature, providing examples such as lines (curvature zero) and circles (constant non-zero curvature). The paragraph also introduces the formula for curvature, denoted by the Greek letter kappa (𝜅), and relates it to the magnitude of the change in the unit tangent vector with respect to arc length. It mentions that finding curvature often involves calculating unit tangent and principal unit normal vectors, referencing previous work for these calculations.
🔍 Calculating Curvature: A Detailed Walkthrough
The second paragraph delves into the process of calculating curvature by revisiting a previous example where unit tangent and principal unit normal vectors were determined. It explains how to use the magnitude of the derivative of the unit tangent vector (t hat prime) and the magnitude of the derivative of the position vector (r prime) to find curvature. The example provided involves a vector-valued function representing a helix, and the paragraph guides through simplifying the function's derivative, calculating the magnitude of r prime, and eventually determining the curvature by dividing the magnitude of t hat prime by the magnitude of r prime, resulting in a curvature value of 3/10 for the helix.
Mindmap
Keywords
💡Curvature
💡Vector-valued function
💡Arc length
💡Unit tangent vector
💡Principal unit normal vector
💡Tangent vector
💡Magnitude
💡Product rule
💡Derivative
💡Helix
💡Pythagorean identity
Highlights
Curvature of a vector-valued function measures the change in direction with respect to arc length.
Lines have constant curvature of zero as they travel in the same direction.
Circles have constant curvature, with the unit tangent changing uniformly around the circumference.
The curvature formula is based on the magnitude of the change of the unit tangent with respect to arc length.
Curvature is often abbreviated with the symbol 'kappa' (κ) from the Greek alphabet.
Finding curvature involves calculating the magnitude of the derivative of the unit tangent vector.
Unit tangent and principal unit normal vectors are integral to curvature calculation.
The magnitude of the derivative of the unit tangent vector (t hat prime) is not necessarily one.
An example calculation of curvature for a helix is provided, with a simplified expression for r prime of t.
The curvature of the helix is calculated to be 3 over 10, demonstrating a non-constant curvature.
The process of simplifying r prime of t and finding its magnitude is explained in detail.
The unit tangent vector is derived by dividing r prime by its magnitude.
The principal unit normal vector (t hat prime) is found by differentiating the unit tangent vector.
The magnitude of t hat prime is crucial for determining the curvature.
An example vector-valued function is given, and its curvature is calculated step by step.
The curvature of the example function is found to be one over the magnitude of t, assuming t is not negative.
The video concludes with a summary of the curvature calculation process and its significance.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: