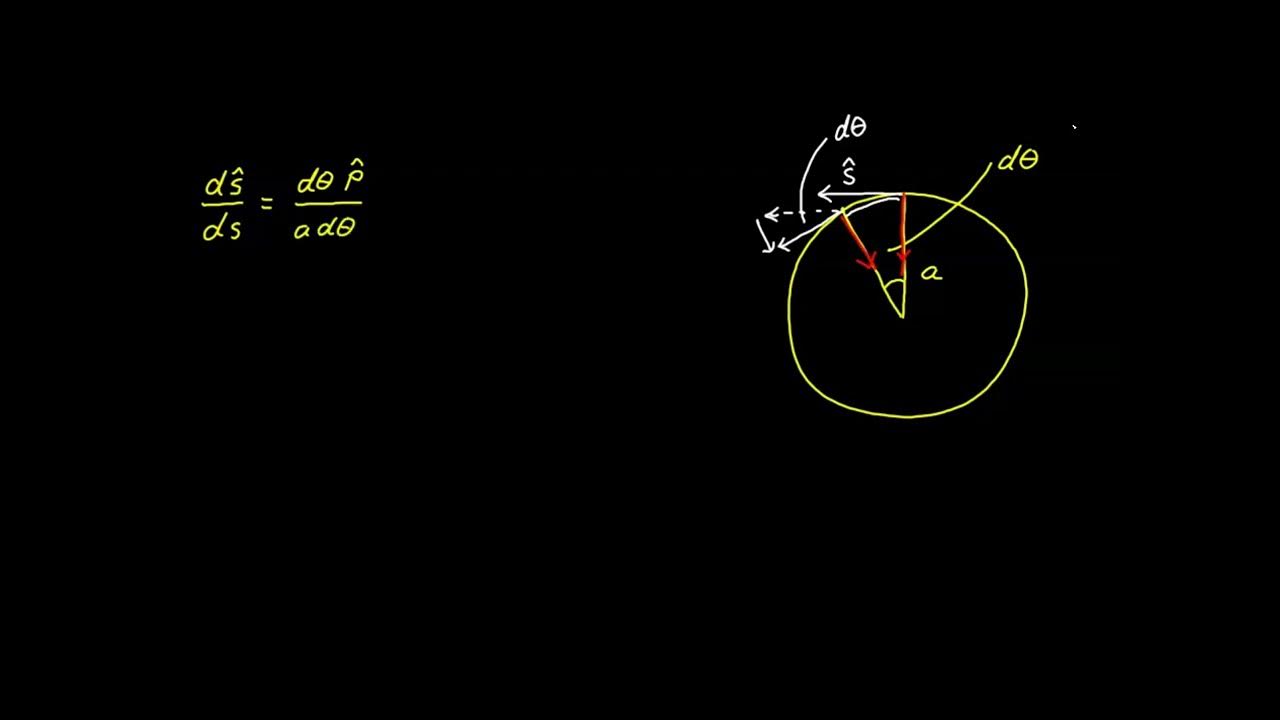Curvature formula, part 5
TLDRThis educational video script delves into the concept of curvature in mathematics, explaining how the formula for curvature measures the degree to which a curve bends. It discusses the significance of the cross-product between the first and second derivatives of a function that parameterizes the curve, and the importance of normalizing these derivatives to ensure the measurement of curvature is independent of the speed at which one travels along the curve. The script also clarifies the role of arc length in calculating curvature, providing a deeper understanding of the mathematical tools used to analyze the bending of curves.
Takeaways
- 🔍 The script discusses the concept of curvature in the context of a mathematical formula, aiming to explain why the formula represents how much a curve actually curves.
- 📏 The numerator of the formula is related to a cross-product between the first and second derivatives of a function that parameterizes the curve.
- 📚 The first derivative of the function gives the direction of the curve's tangent vectors, which are crucial for understanding the curve's orientation at any given point.
- 🔄 The second derivative is analogous to the first derivative but describes how the tangent vectors themselves change direction, indicating the curve's rate of turning.
- ⊥ The cross-product is a measure of how perpendicular the first and second derivative vectors are, which is indicative of the curve's curvature.
- 🚀 Curvature is independent of the parameterization speed of the function; it should remain constant whether the curve is traversed quickly or slowly.
- 📉 To normalize the formula, the first derivative vector is divided by its magnitude, ensuring that the measurement of curvature is consistent regardless of traversal speed.
- 📐 The normalization process adjusts the second derivative vector by the same scaling factor as the first derivative, maintaining the relationship between the two in the cross-product.
- 📈 The curvature formula involves taking the cross-product of the normalized first derivative and the scaled second derivative, then dividing by the magnitude of the first derivative cubed.
- 🧠 The script emphasizes that understanding the curvature formula requires grasping the relationship between the unit tangent vector and its derivative with respect to the parameter.
- 📝 The final formula for curvature is presented as a derivative of the unit tangent vector with respect to the parameter, adjusted for arc length rather than the parameter itself.
Q & A
What is the main topic of the video script?
-The main topic of the video script is the concept of curvature in mathematics, specifically how to understand and calculate the curvature of a curve using a formula involving the first and second derivatives of a function parameterizing the curve.
What is the significance of the numerator in the curvature formula?
-The numerator in the curvature formula corresponds to a cross-product between the first derivative and the second derivative of the function. This cross-product measures how perpendicular the second derivative vector is to the first, which is indicative of the curve's curving behavior.
Why are the first and second derivatives important in understanding curvature?
-The first derivative gives the direction of the curve at a point, represented by tangent vectors, while the second derivative indicates how the direction changes, or how the tangent vectors turn. The relationship between these derivatives is crucial for measuring the curvature of the curve.
What is the role of the parameter 't' in the context of the script?
-The parameter 't' is used to parameterize the curve. It is the variable with respect to which the function is defined and the derivatives are taken. The curvature, however, should be independent of the parameterization speed, which is why the formula is adjusted with respect to arc length.
Why is it necessary to normalize the first derivative vector in the curvature formula?
-Normalizing the first derivative vector ensures that the measurement of curvature is independent of the speed at which one travels along the curve. It allows for a pure measurement of how perpendicular the second derivative vector is to the first, without being affected by the length of the tangent vectors.
What does the term 'arc length' represent in the curvature formula?
-Arc length represents the actual length of the curve between two points. The curvature formula is adjusted with respect to arc length to ensure that the curvature value remains consistent regardless of the speed of traversal along the curve.
How does the magnitude of the first derivative vector affect the curvature calculation?
-The magnitude of the first derivative vector is used to normalize the tangent vectors and to scale down the second derivative vector in the cross-product. This scaling ensures that the curvature calculation is based on a unit tangent vector and is consistent with respect to arc length.
What is the significance of the cross-product in the curvature formula?
-The cross-product between the normalized first derivative and the scaled second derivative vector measures the area of the parallelogram formed by these vectors. This area is a measure of the 'turning' of the curve, which is directly related to its curvature.
Why is the second derivative vector not normalized in the curvature formula?
-The second derivative vector is not normalized because its magnitude indicates the rate of change of the tangent vector's direction. A larger magnitude suggests a more rapid turn, which corresponds to higher curvature, and thus should be reflected in the curvature value.
What is the final expression for curvature given in the script?
-The final expression for curvature in the script is the magnitude of the cross-product between the first derivative vector and the scaled second derivative vector, divided by the cube of the magnitude of the first derivative vector.
How does the script connect the curvature formula to the derivative of the unit tangent vector?
-The script explains that the curvature formula is essentially the derivative of the unit tangent vector with respect to the parameter 't', divided by the magnitude of the first derivative vector, which corrects for the parameterization speed and aligns the curvature calculation with arc length.
Outlines
📚 Understanding Curvature Formula
The first paragraph delves into the concept of curvature in mathematical terms, explaining how the formula for curvature is derived from the cross-product of the first and second derivatives of a function that parameterizes a curve. It emphasizes the importance of normalizing these derivatives to unit vectors to measure the perpendicularity between them, which is indicative of the curve's curvature. The paragraph also discusses the significance of considering curvature with respect to arc length rather than the parameter 't', to ensure that the measure of curvature is consistent regardless of the speed at which one traverses the curve.
🔍 Deriving Curvature from Tangent Vector Derivatives
The second paragraph continues the discussion on curvature by focusing on the derivative of the tangent vector with respect to the parameter 't'. It explains the process of deriving curvature by first taking the derivative of the tangent vector in terms of 't' and then normalizing it by dividing by the magnitude of the first derivative, which represents the change in arc length. The summary clarifies the formula for curvature, highlighting the cross-product of the first derivative vector and the scaled second derivative vector, and emphasizes the importance of the magnitude of the first derivative vector being cubed in the denominator to correct for potential discrepancies when measuring curvature with respect to 't' instead of arc length 's'.
Mindmap
Keywords
💡Curvature
💡Cross-product
💡First derivative
💡Second derivative
💡Tangent vector
💡Parameterization
💡Arc length
💡Normalization
💡Magnitude
💡Unit tangent vector
💡Perpendicular
Highlights
The formula for curvature is explained, illustrating how it measures the degree to which a curve bends.
The numerator of the formula corresponds to a cross-product, which is between the first and second derivatives of the function parameterizing the curve.
The first derivative vector provides the direction of movement from one point on the curve to the next.
Tangent vectors along the curve are derived from the first derivative of a parametric function.
The second derivative vector represents the change in direction of the first derivative, contributing to the curve's bending.
When a curve is purely turning, the second derivative vector is perpendicular to the first, indicating curving.
Curvature is independent of the parameterization speed of the function, maintaining a consistent measure regardless of traversal speed.
The cross-product's magnitude is affected by the speed of traversal, necessitating normalization to measure pure perpendicularity.
Normalization involves adjusting vectors to unit length to accurately measure the perpendicular relationship between derivatives.
The curvature formula accounts for the magnitude of the first derivative when scaling down the second derivative.
Curvature is calculated with respect to arc length rather than the parameter to ensure consistency across different speeds.
The formula for curvature involves the cross-product of the normalized first derivative and the scaled second derivative.
The resulting expression for curvature is the derivative of the unit tangent vector with respect to the parameter.
The curvature formula is derived by dividing the cross-product expression by the magnitude of the first derivative cubed.
The final curvature formula is presented, emphasizing its conceptual understanding and practical application.
The video concludes with a plan to demonstrate the computation of curvature through specific examples in upcoming videos.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: