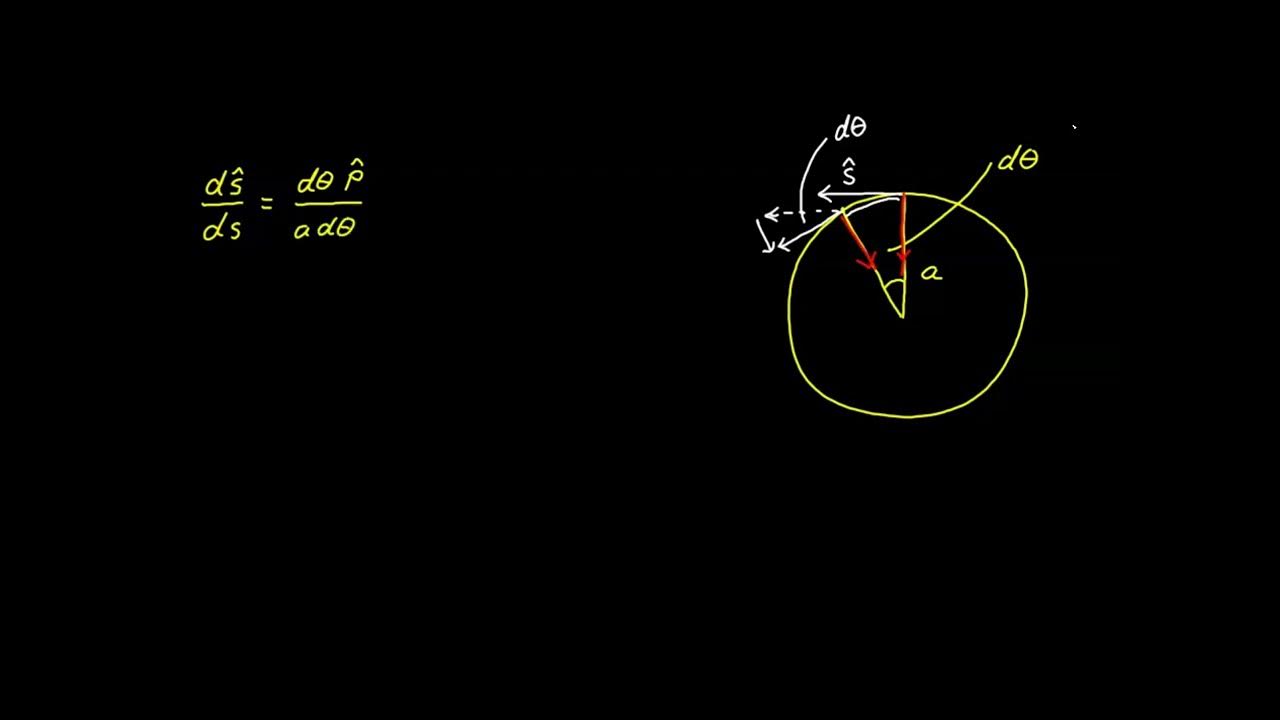Curvature intuition
TLDRThe video script introduces the concept of curvature by using the analogy of driving on a road. It explains how the steering wheel's position relates to the curve's radius of curvature, 'R'. The script then delves into the mathematical representation of curvature, which is the reciprocal of 'R', symbolized by the Greek letter Kappa. It highlights the importance of curvature as a measure of sharpness in turns, with a larger curvature indicating a tighter curve. The video promises a deeper mathematical exploration in subsequent episodes.
Takeaways
- 🚗 The video discusses the concept of curvature in the context of driving on a road that bends.
- 📍 The X and Y axes are introduced as a reference for the curve drawn on the plane.
- 🛣️ The speaker uses the analogy of a road to explain the experience of driving on a curve and the need to steer.
- 🚙 The steering wheel's position is likened to the curvature at a certain point on the road.
- 🔄 If the steering wheel is stuck at a particular angle, the car will trace out a circle, illustrating the concept of radius of curvature.
- 🔽 The radius of curvature (R) is defined as the radius of the circle a car would trace if the steering wheel is locked at a given point.
- 🔄 The curvature of a curve is the reciprocal of the radius of curvature, represented by the Greek letter Kappa (𝜅).
- ↔️ Curvature is a measure of how much a curve bends; a smaller radius of curvature indicates a sharper turn.
- 🔢 The curvature is inversely related to the radius of curvature; a larger curvature value means a more pronounced bend.
- 📚 The video promises to delve into the mathematical description of curvature in upcoming videos, including the use of vector-valued functions.
- 📝 The concept of curvature is introduced as a fundamental measure in understanding the nature of bends and turns in a curve.
Q & A
What is the main topic discussed in the video script?
-The main topic discussed in the video script is the concept of curvature, particularly as it relates to a curve on the XY plane and the idea of a road that a driver would navigate.
What analogy does the script use to explain the feeling of driving on a curve?
-The script uses the analogy of driving a car on a curve, where the steering wheel needs to be turned to follow the curve, to explain the feeling of driving on a curve.
What happens if the steering wheel is stuck while driving on a curve?
-If the steering wheel is stuck, the car will trace out a circle, with the size of the circle depending on the point on the curve where the steering wheel is locked.
What is the term for the circle that a car would trace out if the steering wheel is locked?
-The term for the circle that a car would trace out if the steering wheel is locked is the 'Radius of Curvature'.
How does the radius of curvature relate to the sharpness of a curve?
-The radius of curvature is inversely related to the sharpness of a curve. A smaller radius indicates a sharper turn, while a larger radius indicates a more gentle curve.
What is the mathematical measure used to describe how much a curve curves?
-The mathematical measure used to describe how much a curve curves is the reciprocal of the radius of curvature, denoted by the Greek letter Kappa (κ).
Why is the reciprocal of the radius of curvature used instead of the radius itself?
-The reciprocal of the radius of curvature is used because it provides a measure where more sharp turns result in a higher number, which is a more intuitive way to describe the degree of curvature.
What is the significance of the Greek letter Kappa (κ) in the context of the script?
-In the context of the script, the Greek letter Kappa (κ) represents curvature, which is the reciprocal of the radius of curvature.
How does the curvature change as you move along different parts of a curve?
-As you move along different parts of a curve, the curvature changes, resulting in different radii of curvature. Sharper turns have smaller radii and thus higher curvature values.
What will be covered in the next video according to the script?
-In the next video, the script promises to describe more mathematically how to capture the value of curvature, including the use of parametric equations and vector-valued functions.
What is the purpose of the video script in terms of the explanation of curvature?
-The purpose of the video script is to provide an intuitive understanding of curvature through the analogy of driving a car, and to introduce the concept of the radius of curvature and its mathematical representation.
Outlines
🚗 Introduction to Curvature and Driving Analogy
The video script begins with an introduction to the concept of curvature using a driving analogy. The speaker draws a curve on the X-Y plane and invites the audience to imagine driving on it. They describe the experience of driving on a gentle curve, where the steering wheel is slightly turned to the right, and then imagine the scenario where the steering wheel is stuck, leading to the car tracing out a circle. This circle is dependent on the point on the curve, with tighter curves resulting in smaller circles. The speaker introduces the 'Radius of Curvature' (R) as a measure of how much the curve turns, and explains that curvature itself is the reciprocal of this radius, symbolized by the Greek letter Kappa (𝜅). The script sets the stage for a more mathematical explanation in subsequent videos.
Mindmap
Keywords
💡Curvature
💡X-Y plane
💡Steering wheel
💡Radius of Curvature
💡Circle
💡Turning radius
💡Reciprocal
💡Kappa (Greek letter)
💡Vector-valued function
💡Parametric description
💡Mathematical capture
Highlights
Introduction to the concept of curvature and its analogy to driving on a curved road.
Explanation of how the steering wheel position relates to the curve's gentleness or sharpness.
Illustration of the scenario where the steering wheel is stuck, resulting in a circular path.
The dependency of the traced circle's size on the point on the curve and the steering wheel's angle.
Introduction of the term 'Radius of Curvature' (R) as a measure of how much a curve turns.
Description of the turning radius in cars and its relation to the radius of curvature.
Clarification that curvature is the reciprocal of the radius of curvature, represented by the Greek letter Kappa.
Reasoning behind using the reciprocal of the radius of curvature to measure sharpness of turns.
The significance of a smaller radius of curvature indicating a sharper turn.
The inverse relationship between the radius of curvature and the road's straightness.
Upcoming mathematical description of capturing the curvature value.
Introduction of parametric representation and vector-valued functions for curves.
Anticipation of future videos explaining the mathematical formula for curvature.
The importance of understanding curvature for accurately describing the shape and turns of a curve.
The practical application of curvature in real-life scenarios such as driving and road design.
The analogy's effectiveness in making the abstract concept of curvature more tangible and understandable.
The educational approach of using everyday experiences to explain complex mathematical ideas.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: