A Secret Weapon for Predicting Outcomes: The Binomial Distribution
TLDRThis video script explores the binomial distribution formula, applicable to processes with two outcomes, like a 60% free throw shooter making 7 out of 10 shots. It explains the concept through visual models, introduces Pascal's Triangle for binomial coefficients, and delves into factorials for calculating these coefficients. The script emphasizes the importance of independence between events and concludes with a practical simulation to validate the formula's accuracy, highlighting the foundational role of combinatorics in probability.
Takeaways
- 🏀 The script discusses the probability of a 60% free throw shooter making exactly 7 out of 10 shots using the binomial distribution formula.
- 📊 The binomial distribution is applicable to any situation with a repeating process that can be categorized into two outcomes, such as yes/no questions.
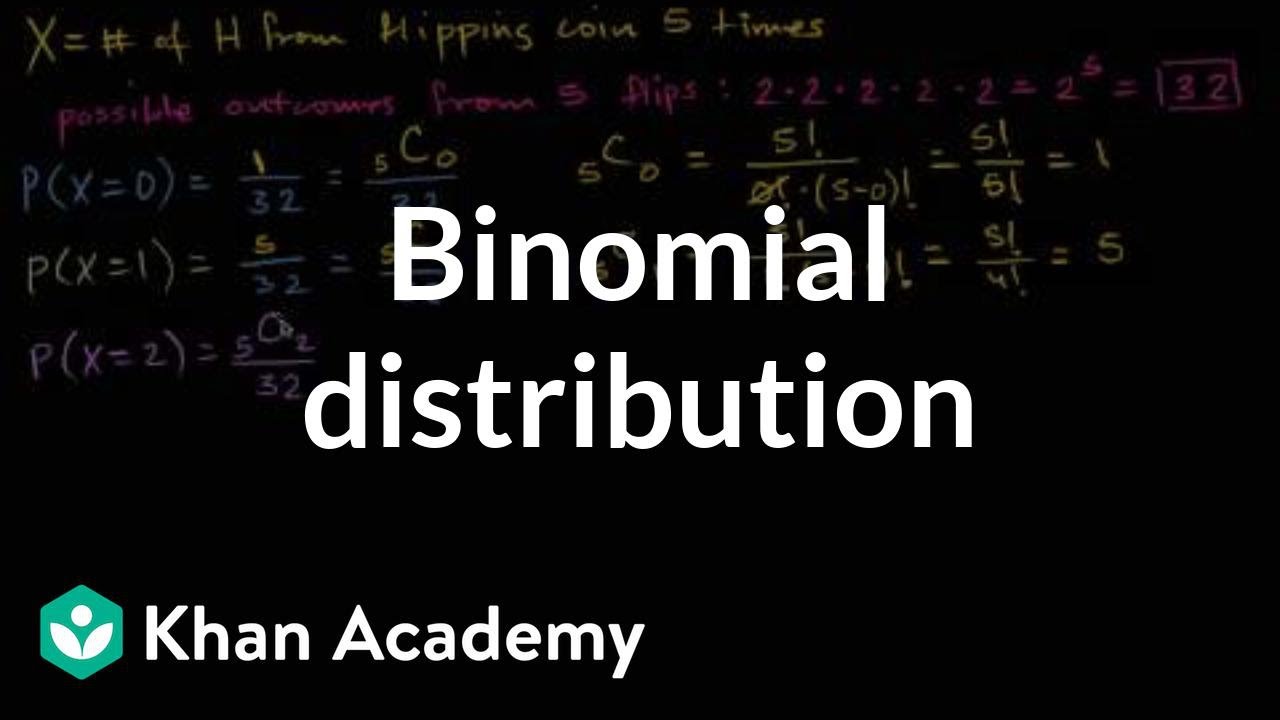
- 🔢 The script introduces the concept of binomial coefficient, which represents the number of ways to get a certain number of successes (makes) out of total trials (shots).
- 🎯 The probability of a specific outcome (e.g., making 7 out of 10 shots) is calculated by multiplying the binomial coefficient with the individual probabilities of success and failure raised to their respective powers.
- 📚 The script explains that the binomial distribution assumes independence between events, meaning the outcome of one event does not affect the others.
- 📉 The video uses a visual approach with 'blobs' to illustrate the concept of binomial distribution and the calculation of probabilities.
- 📏 The script introduces Pascal's Triangle as a pattern for counting the number of ways to achieve a certain number of successes in a series of trials.
- 🔑 A formula for calculating the binomial coefficient is provided, involving factorials, which is crucial for understanding how to compute probabilities for larger numbers of trials and successes.
- 🧩 The importance of combinatorics, the branch of mathematics dealing with counting and arranging groups of items, is highlighted in understanding the binomial distribution.
- 🔄 The script emphasizes the iterative process of adding shots and calculating probabilities, showing how the pattern of probabilities develops with each additional shot.
- 🤖 The video concludes with a practical application, simulating 10,000 'blobs' to test the accuracy of the binomial distribution formula against randomized results.
Q & A
What is the binomial distribution used for?
-The binomial distribution is used to calculate the probability of a given number of successes in a fixed number of independent Bernoulli trials, where each trial has two possible outcomes: success or failure.
What does the term 'binomial' imply about the type of events being analyzed?
-The term 'binomial' implies that there are two categories of outcomes for each trial, which is why it's called 'bi'nomial', and it's used for yes-or-no type questions or events.
What is the probability of a 60% free throw shooter making exactly 7 out of 10 shots?
-The exact probability is calculated using the binomial distribution formula and is approximately 21.5%, as explained in the script.
How is the binomial coefficient represented in the formula for binomial distribution?
-The binomial coefficient is represented as 'n choose k', which is the number of ways to choose k successes out of n trials, and is calculated using factorials.
What is Pascal's Triangle and how is it related to the binomial distribution?
-Pascal's Triangle is a triangular array of numbers where each number is the sum of the two numbers directly above it. It is related to the binomial distribution as the entries in each row of Pascal's Triangle represent the binomial coefficients for a given row.
What is a factorial and how is it used in the binomial coefficient formula?
-A factorial, denoted by an exclamation point (e.g., n!), is the product of an integer and all the positive integers below it down to 1. It is used in the binomial coefficient formula to calculate the number of ways to arrange n unique objects and to account for the arrangements of identical objects in each category.
Why is it important to consider the independence of trials when using the binomial distribution?
-The binomial distribution assumes that the trials are independent, meaning the outcome of one trial does not affect the outcome of another. This is important because if the trials are not independent, the probabilities calculated using the binomial distribution may not be accurate.
What does the script suggest as a starting point for understanding the binomial distribution?
-The script suggests starting with simple scenarios, such as a single shot with a 60% success rate, to build an understanding of the binomial distribution before moving on to more complex situations.
How can one visualize the probabilities of making or missing shots in the script's example?
-The script suggests visualizing the probabilities by looking at a square or 3D grid of 'blobs', where each blob represents a shot and its color represents whether it was a make or miss.
What is the significance of the script's mention of the 'blobs' taking multiple shots?
-The mention of 'blobs' taking multiple shots is a way to visually represent the repeated trials in the binomial distribution, helping to illustrate the concept of multiple independent events and their outcomes.
Outlines
🏀 Understanding Binomial Distribution in Basketball Free Throws
This paragraph introduces the concept of binomial distribution, using the example of a 60% free throw shooter making exactly 7 out of 10 shots. It explains that the binomial distribution is applicable to any process with two possible outcomes, like yes or no questions, and is not limited to sports. The paragraph simplifies the complex formula by breaking it down and building upon basic probabilities, illustrating how to calculate the likelihood of different outcomes using a visual 'blob' representation. It emphasizes that this method can be used to predict the probability of making a certain number of attempts in any repetitive process with binary outcomes.
📊 Deep Dive into the Binomial Coefficient and Pascal's Triangle
The second paragraph delves deeper into the binomial coefficient, which is a key component of the binomial distribution formula. It explains how the coefficient represents the number of ways to achieve a certain number of successes in a given number of trials. The paragraph introduces Pascal's Triangle as a visual tool to determine the binomial coefficients and discusses the pattern observed in the triangle. It also mentions the formula for calculating the binomial coefficient using factorials, which may seem complex at first but is essential for understanding the distribution, especially when dealing with larger numbers of trials and successes.
🧩 Factorials and Combinatorics: The Mathematics of Arranging Outcomes
This paragraph explores the concept of factorials and their role in the binomial coefficient formula. It explains factorials through examples and relates them to the number of ways to arrange objects, which is fundamental to combinatorics. The paragraph uses the analogy of arranging blobs with hats to illustrate how factorials in the denominator of the binomial coefficient formula account for the arrangements of identical objects within categories. It emphasizes the importance of understanding factorials to grasp the binomial distribution formula and its application in calculating probabilities for various outcomes.
🤖 Simulation and Validation of the Binomial Distribution Model
The final paragraph discusses the practical application and validation of the binomial distribution model through simulation. It mentions testing the formula against randomized results with a large number of trials to ensure the model's accuracy. The paragraph acknowledges that while the results may not be exact, they should be close, highlighting the importance of simulation in verifying mathematical models. It concludes by emphasizing the value of the binomial distribution in analyzing repeatable, independent events with binary outcomes and invites viewers to support the creation of educational content on Patreon.
Mindmap
Keywords
💡Binomial Distribution
💡Probability
💡Bernoulli Trials
💡Binomial Coefficient
💡Pascal's Triangle
💡Factorials
💡Independence
💡Combinatorics
💡Randomized Results
💡Success Rate
💡Visualization
Highlights
The binomial distribution formula is introduced to calculate the probability of a certain number of successes in a series of independent trials.
The concept of 'bi' in binomial signifies a two-category outcome, applicable to yes-or-no questions.
A visual representation using 'blobs' is introduced to simplify understanding of binomial probabilities.
The probability of a 60% free throw shooter making 1 out of 1 shots is straightforwardly 60%.
The binomial distribution is applicable to any repeating process with binary outcomes.
The probability of missing both shots is calculated by multiplying the probabilities of individual shot outcomes.
A pattern is observed where the number of ways to make a certain number of shots follows a 1-2-1 sequence.
The binomial coefficient is explained as the number of ways to get a certain number of successes out of total trials.
Pascal's Triangle is introduced as a method to determine the binomial coefficient.
The formula for the binomial coefficient using factorials is presented.
Zero factorial is defined as one to accommodate cases with zero successes or failures.
The importance of combinatorics in understanding the arrangement and grouping of objects is highlighted.
A practical application of the binomial distribution is demonstrated with a simulated test using 10,000 blobs.
The assumption of independence between shots is discussed, acknowledging potential real-world deviations.
The process of counting arrangements is simplified by using a table instead of a full tree diagram.
The video concludes with a summary of the binomial distribution's utility in calculating probabilities for binary outcomes.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: