Position, Distance & Displacement in Physics - [1-2-1]
TLDRThe lesson focuses on clarifying the concepts of position, distance, and displacement in the context of one-dimensional motion. It emphasizes the importance of understanding these terms to grasp further physics concepts such as velocity and acceleration. The key difference highlighted is that position denotes a location with coordinates, distance measures the total path length traveled (always positive), and displacement represents the net change in position from start to finish (which can be positive or negative). The lesson also introduces the idea of vectors, emphasizing that displacement is a vector quantity with both magnitude and direction.
Takeaways
- 📍 The lesson focuses on understanding the concepts of position, distance, and displacement in one-dimensional motion.
- 📏 Position is a coordinate that locates the location of an object along a reference line (e.g., x-axis).
- 🛣️ Distance is the total length of the path traveled by an object, regardless of direction, and is always a positive value.
- 🔄 Displacement, unlike distance, is concerned only with the initial and final positions of an object and can be a negative value, indicating direction.
- 🔢 The calculation of displacement is given by the formula Δx = x₂ - x₁, where x₂ is the final position and x₁ is the initial position.
- 🎯 Negative and positive signs in displacement indicate direction: negative means left (or backwards) and positive means right (or forwards).
- 🔍 The difference between distance and displacement is crucial for further studies in physics, especially when calculating velocity and acceleration.
- 📈 The concept of vectors is introduced, where displacement is the simplest form of a vector because it has both magnitude and direction.
- 🌟 Understanding vectors is essential as many physical quantities, like velocity and acceleration, are vector quantities with both magnitude and direction.
- 📊 In two-dimensional motion, the concepts of position, distance, and displacement extend to an x-y coordinate plane, with displacement calculated considering changes in both axes.
- 📚 The lesson emphasizes the importance of grasping these fundamental concepts to build a strong foundation for advanced topics in physics.
Q & A
What is the main difference between position and displacement?
-Position refers to the specific location of an object along a reference line, represented by a coordinate. Displacement, on the other hand, is the straight-line distance and direction from the initial to the final position, independent of the actual path taken.
How is distance different from displacement in one-dimensional motion?
-Distance is the total length of the path traveled by an object, including all the back and forth movements, and is always a positive value. Displacement only considers the net change in position from the start to the end point and can be negative, indicating the direction of movement.
What is the significance of the sign in displacement?
-The sign in displacement indicates the direction of movement. A positive sign means the object has moved to the right (along the positive direction of the reference line), while a negative sign indicates movement to the left (negative direction).
What is the relationship between displacement and vectors?
-Displacement is a vector quantity because it has both magnitude (the straight-line distance between the initial and final positions) and direction (indicated by the sign). This makes displacement a fundamental concept in physics when dealing with motion and forces.
How do you calculate the displacement in one-dimensional motion?
-Displacement is calculated using the formula Δx = x2 - x1, where x2 is the final position coordinate and x1 is the initial position coordinate. The result gives you the magnitude and the sign indicates the direction of displacement.
What is the difference between distance and displacement when an object returns to its starting point?
-If an object returns to its starting point, the distance traveled would be the total length of all paths taken (including returns), which could be a large number. However, the displacement would be zero because the initial and final positions are the same point.
Why is understanding the difference between position, distance, and displacement important in physics?
-Understanding these concepts is crucial because they form the basis for calculating other physical quantities like velocity and acceleration. Accurate analysis of motion, forces, and energy requires a clear grasp of position, distance, and displacement.
What is the role of the number line in representing position in one-dimensional motion?
-The number line provides a reference for representing positions along a single dimension. It allows us to assign positive and negative values to positions based on their direction relative to a chosen origin point, facilitating the calculation of distances and displacements.
How does the concept of one-dimensional motion simplify the study of physics?
-One-dimensional motion simplifies the study of physics by focusing only on movement along a single axis, which makes it easier to understand and visualize. This foundational understanding is essential before progressing to more complex, multi-dimensional motions.
What is the significance of the Greek letter delta (Δ) in physics?
-The Greek letter delta (Δ) is used to denote change in a quantity. In physics, it signifies the difference between the final and initial values of a variable, such as position (Δx), time (Δt), or temperature (ΔT), which is crucial for calculating displacement, velocity, and other dynamic quantities.
Outlines
📚 Introduction to Position, Distance, and Displacement
This paragraph introduces the fundamental concepts of position, distance, and displacement in the context of one-dimensional motion. It emphasizes the importance of understanding these terms to grasp more complex topics in physics, such as velocity and acceleration. The lesson begins with clarifying potential confusion between these terms and sets the stage for learning about average velocity, average speed, and instantaneous measurements. The concept of one-dimensional motion is introduced, using an imaginary string with a bead moving back and forth as an analogy. The lesson highlights that all motion in this context is relative to a reference point and that negative and positive values represent movement in opposite directions from this point.
📐 Explaining Position and Reference Points
The paragraph delves into the concept of position, explaining that it is a coordinate that locates the place of an object. It uses the analogy of a number line to illustrate how positions are measured in relation to a reference point. The discussion extends to how negative and positive positions represent locations to the left and right of the reference point. The explanation serves to establish a framework for understanding how positions are defined and how they relate to one-dimensional motion. The paragraph also reassures that negative positions are not mystical but simply indicate a direction relative to the reference point.
🛣️ Understanding Distance Traveled
This section clarifies the concept of distance traveled, contrasting it with the concept of displacement. Distance traveled is the total length of the path taken by an object, regardless of direction. It explains that distance is always a positive value because it accumulates the entirety of the path, including any backtracking or zigzagging. The paragraph uses a journey along an x-axis to illustrate how distance is calculated, emphasizing that the sign (positive or negative) of individual segments does not affect the total distance traveled.
🔄 Differentiating Distance from Displacement
The paragraph introduces displacement as a distinct concept from distance. Displacement is defined as the change in position from the initial to the final point and is represented by the vector delta x. It is calculated by subtracting the initial position coordinate from the final position coordinate. The explanation highlights that displacement only considers the endpoints of a journey and ignores any intermediate zigzagging or backtracking. The paragraph also points out that displacement can be a negative value, which indicates the direction of the net movement, unlike distance, which is always positive.
🔽 Negative Displacement and Vector Quantities
This part of the script explores the concept of negative displacement and its implications. It explains that a negative displacement indicates a net movement to the left on the coordinate system, while a positive displacement indicates a movement to the right. The paragraph further discusses the idea of vector quantities, which have both magnitude and direction. Displacement is identified as the simplest form of a vector in physics, with the magnitude representing the distance moved and the sign indicating the direction of movement. The explanation sets the stage for a deeper understanding of vectors and their importance in physical concepts like velocity and acceleration.
📈 Two-Dimensional Motion and Displacement
The final paragraph extends the discussion to two-dimensional motion, emphasizing the difference between distance and displacement in a 2D context. It uses the analogy of walking in a city, moving north and then east, to illustrate that while the total distance traveled would be the sum of all movements, the displacement would be zero since the starting and ending points are the same. This analogy helps to reinforce the concept that displacement is concerned only with the initial and final positions, regardless of the path taken. The paragraph concludes by reiterating the importance of understanding displacement as a vector quantity, which will be a recurring theme in the study of physics.
Mindmap
Keywords
💡Position
💡Distance
💡Displacement
💡One-dimensional motion
💡Reference point
💡Average velocity
💡Instantaneous velocity
💡Vector
💡Scalar
💡Number line
💡Delta notation
Highlights
The lesson focuses on clarifying the concepts of position, distance, and displacement in physics, emphasizing their differences and importance in understanding motion.
One-dimensional motion is used as a starting point to explain these concepts, using an imaginary string and bead as an analogy for movement along the x-axis.
The number line is introduced as a framework for measuring position, with positive and negative values indicating direction relative to a reference point.
Distance is defined as the total length of the path traveled, regardless of direction, and is always a positive value.
Displacement, on the other hand, is the straight-line change in position from the initial to the final point and can be a negative value, indicating direction.
The concept of vectors is introduced, with displacement being the simplest form of a vector because it has both magnitude and direction.
The lesson explains the importance of understanding the difference between distance and displacement for further studies in physics, such as velocity and acceleration.
The use of delta notation (Δ) is explained to represent change in a quantity, such as position or temperature.
A practical example is given where an object moves along a path and the total distance traveled is calculated, highlighting the difference between distance and displacement.
The lesson emphasizes that negative numbers in physics do not represent mystical concepts but rather direction and relative position.
The concept of two-dimensional motion is briefly introduced, with the x-y coordinate plane used to illustrate how distance and displacement are calculated in such scenarios.
The lesson concludes by reinforcing the importance of understanding displacement as a vector quantity, with its magnitude and direction being crucial for further studies in physics.
The lesson provides a foundation for understanding more complex motion and introduces the idea of combining mathematical concepts with physical phenomena.
The use of examples and analogies, such as the string and bead, helps to clarify abstract concepts and make them more accessible to learners.
The lesson emphasizes the importance of the reference point in all measurements and calculations in physics, as it provides the basis for determining position and displacement.
The lesson explains how the sign of a displacement value indicates the direction of movement, with positive values indicating rightward movement and negative values indicating leftward movement.
Transcripts
Browse More Related Video
AP Physics 1 review of 1D motion

What Are Distance and Displacement? | Physics in Motion
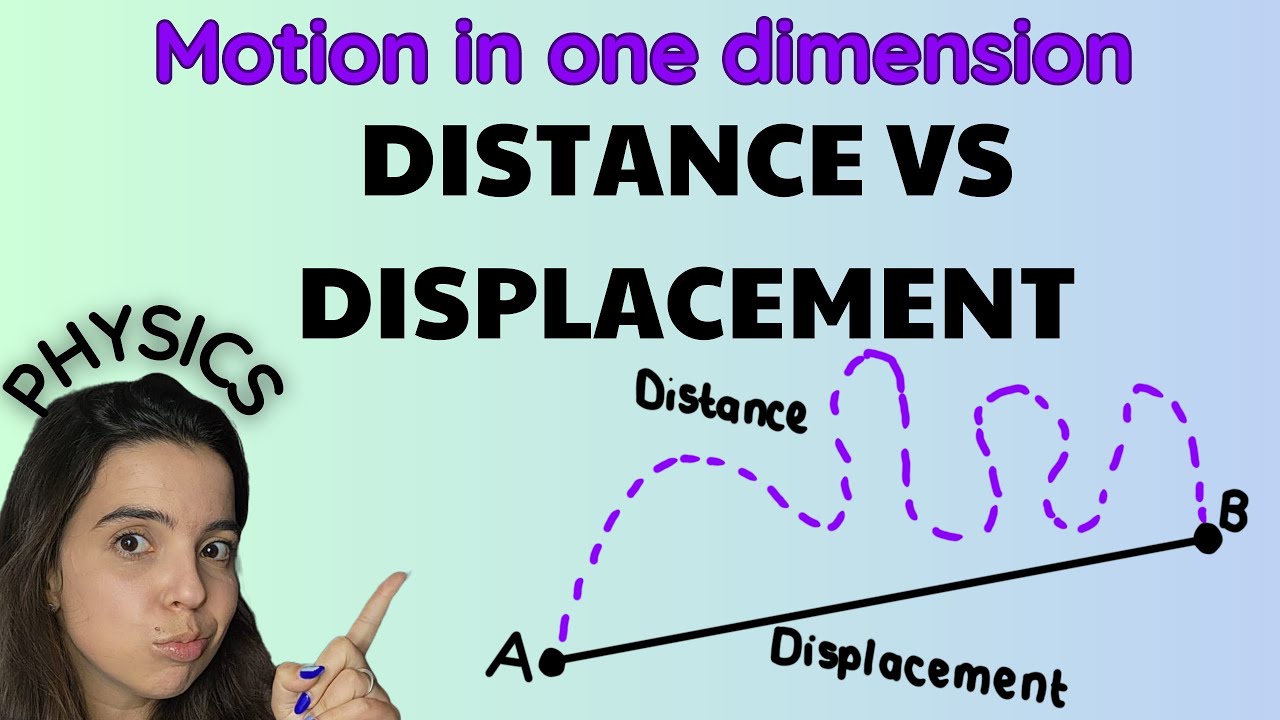
Displacement and Distance: Motion in One Dimension

Motion problems with integrals: displacement vs. distance | AP Calculus AB | Khan Academy

Distance vs. Displacement & Speed vs. Velocity | Kinematics Explained

Distance and displacement in one dimension | One-dimensional motion | AP Physics 1 | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: