How To Use Slater's Rule to Estimate The Effective Nuclear Charge

The Organic Chemistry Tutor
2 Feb 201912:29
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video explores Slater's Rule, a method for estimating the effective nuclear charge on electrons in various elements. By using specific shielding constants, the video demonstrates calculations for elements like beryllium, fluorine, and titanium, comparing estimated values to actual data. It also highlights the accuracy of Slater's Rule for lighter elements and its deviation with heavier ones, providing a practical guide for understanding atomic structure.

Takeaways
  • 📚 Slater's Rule is a method used to estimate the effective nuclear charge (ENC) experienced by electrons in an atom.
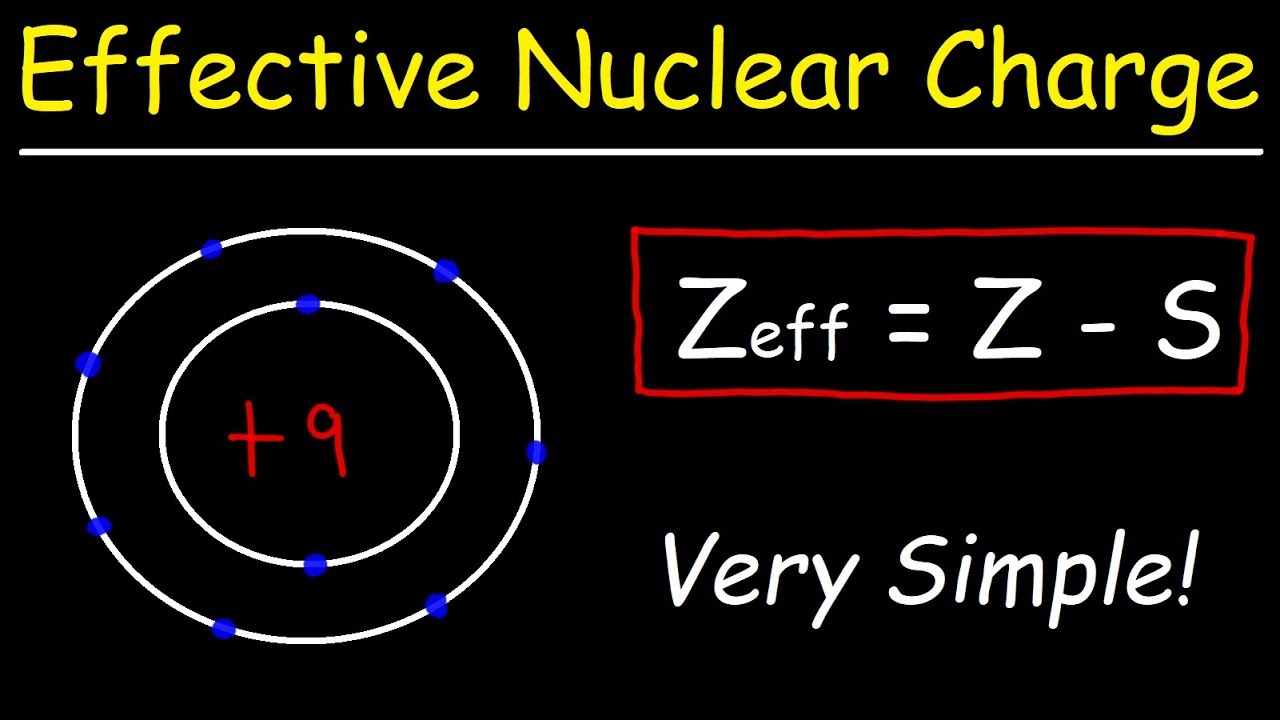
  • 🔍 The formula for calculating ENC is Z - S, where Z is the atomic number and S is the shielding constant.
  • 🌟 Beryllium's electron configuration is 1s² 2s², and its atomic number (Z) is 4.
  • 📉 For the 2s electron in beryllium, the calculated S value using Slater's Rule is 2.05, resulting in an ENC of 1.95.
  • 📈 The estimated ENC for a 2s electron in beryllium is close to the actual value reported on Wikipedia, 1.912.
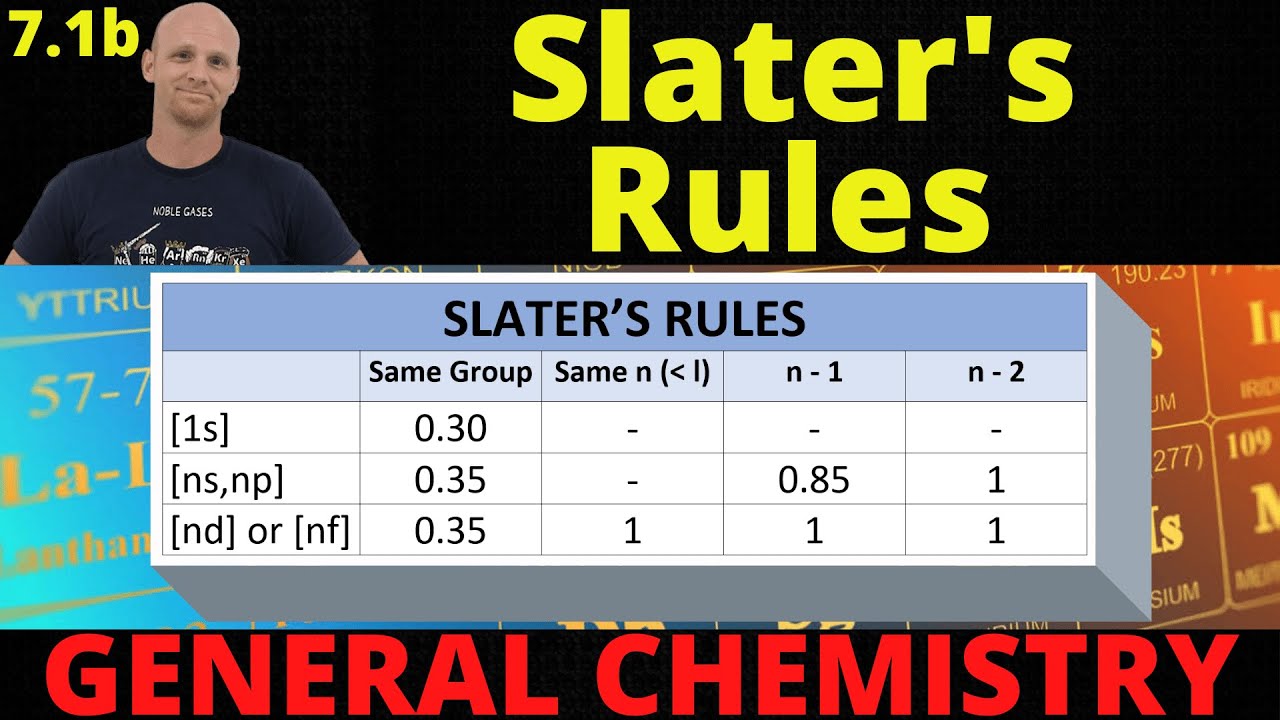
  • 🌐 For s and p electrons, the shielding values differ based on the energy level difference between the electron being analyzed and other electrons.
  • 🍎 Fluorine's electron configuration is 1s² 2s² 2p⁵, and its atomic number is 9.
  • 🔢 For 2p electrons in fluorine, the calculated S value is 3.8, leading to an ENC of 5.2, close to the actual value of 5.1.
  • 🛠 The rules for calculating S values change for d and f electrons, with higher energy level differences receiving a shielding value of 1.
  • 💡 The estimated ENC for a 3d electron in titanium is 9.5, which deviates more from the actual value of 8.983 due to its higher atomic number.
  • 🚀 For 1s electrons in lighter elements like lithium, magnesium, and potassium, the S value is 0.3, providing a more accurate estimation for lighter elements.
Q & A

  • What is Slater's rule used for?

    -Slater's rule is used to estimate the effective nuclear charge (ENC) experienced by an electron in an atom. It helps to understand how the presence of other electrons in the atom shields the electron from the full charge of the nucleus.

  • What is the formula for calculating the effective nuclear charge using Slater's rule?

    -The formula for calculating the effective nuclear charge using Slater's rule is ENC = Z - S, where Z is the atomic number of the element and S is the shielding constant calculated based on the electron configuration of the atom.

  • What is the atomic number of Beryllium and how does it relate to calculating the effective nuclear charge for its 2s electron?

    -The atomic number of Beryllium is 4. It is used in the formula for calculating the effective nuclear charge (ENC = Z - S) where Z represents the atomic number, and in the case of Beryllium's 2s electron, it is subtracted by the shielding constant (S) to estimate the ENC.

  • How is the shielding constant (S) calculated for the 2s electron in Beryllium according to the video?

    -The shielding constant (S) for the 2s electron in Beryllium is calculated by considering the shielding effect of other electrons in the same energy level and those in lower energy levels. It is calculated as 2 * 0.85 (for the 1s electrons) + 1 * 0.35 (for the other 2s electron), resulting in an S value of 2.05.

  • What is the estimated effective nuclear charge for a 2s electron in Beryllium using Slater's rule, and how does it compare to the actual value?

    -The estimated effective nuclear charge for a 2s electron in Beryllium using Slater's rule is 1.95. This is close to the actual value reported on Wikipedia, which is 1.912, indicating that Slater's rule provides a good estimate.

  • What is the electron configuration of Fluorine and how does it affect the calculation of the effective nuclear charge for its 2p electron?

    -The electron configuration of Fluorine is 1s² 2s² 2p⁵. To calculate the effective nuclear charge for its 2p electron, the shielding constant (S) is determined by considering the shielding effect of the 1s and 2s electrons, as well as the other 2p electrons, resulting in an S value of 3.8.

  • What is the atomic number of Fluorine and how is it used in the calculation of the effective nuclear charge for its 2p electron?

    -The atomic number of Fluorine is 9. It is used in the formula for calculating the effective nuclear charge (ENC = Z - S) where Z is the atomic number, and in the case of Fluorine's 2p electron, it is subtracted by the shielding constant (S) to estimate the ENC.

  • How does the shielding rule differ for s and p electrons compared to d and f electrons when using Slater's rule?

    -For s and p electrons, the shielding values are 0.35 for the same group, 0.85 for one group lower, and 1 for two or more groups lower. For d and f electrons, the rule is simplified to 0.35 for the same group and 1 for any group lower.

  • What is the electron configuration of Titanium and how does it influence the calculation of the effective nuclear charge for its 3d electron?

    -The electron configuration of Titanium is 1s² 2s² 2p⁶ 3s² 3p⁶ 4s² 3d³. The calculation of the effective nuclear charge for its 3d electron involves considering the shielding effect of electrons in the second energy level and those in the same energy level as the 3d electrons, resulting in an S value of 13.5.

  • What is the atomic number of Titanium and how is it used in the calculation of the effective nuclear charge for its 3d electron?

    -The atomic number of Titanium is 23. It is used in the formula for calculating the effective nuclear charge (ENC = Z - S) where Z is the atomic number, and in the case of Titanium's 3d electron, it is subtracted by the shielding constant (S) to estimate the ENC.

  • How does the estimation of the effective nuclear charge for the 1s electron in light elements compare to that in heavier elements?

    -For light elements like Lithium, the estimation using Slater's rule (Z - 0.3) is quite accurate. However, for heavier elements, the estimation tends to deviate more from the actual value due to increased electron-electron interactions and shielding effects.

  • What is the significance of the shielding constant (S) value for the 1s electron in estimating the effective nuclear charge for light elements?

    -For light elements, the shielding constant (S) value for the 1s electron is 0.3, which provides a reasonably accurate estimation of the effective nuclear charge. This is because the electron-electron interactions and shielding effects are less complex in lighter atoms.

Outlines
00:00
🔬 Slater's Rule for Effective Nuclear Charge Calculation

This paragraph introduces Slater's Rule and demonstrates its application to calculate the effective nuclear charge of a 2s electron in beryllium. The atomic number 'z' is identified as 4 for beryllium, and the shielding constant 's' is calculated using specific values for different electron groups, resulting in an 's' value of 2.05. Applying the formula z - s, the effective nuclear charge is estimated to be 1.95, which is compared to the actual value of 1.912 found on Wikipedia, indicating a good approximation. The paragraph also outlines the process for estimating the effective nuclear charge for 2p electrons in fluorine.

05:02
📚 Advanced Slater's Rule Application for d Electrons

The second paragraph delves into using Slater's Rule for estimating the effective nuclear charge of a 3d electron in titanium. It begins with the electron configuration and explains the modified rules for calculating 's' when dealing with d and f electrons. The shielding effect is calculated by considering electrons in different energy levels, resulting in an 's' value of 13.5. Using the atomic number of titanium (23), the effective nuclear charge is estimated to be 9.5, which is then compared to the reported value of 8.983 on Wikipedia, showing a reasonable approximation despite some deviation.

10:03
🌟 Effective Nuclear Charge Estimation for 1s Electrons in Light Elements

The final paragraph focuses on estimating the effective nuclear charge for 1s electrons in lithium, magnesium, and potassium. It explains that for 1s electrons, the shielding constant 's' is fixed at 0.3, which provides accurate results for lighter elements. The paragraph provides calculations for each element, showing a close approximation to actual values, although the deviation increases with heavier elements. This illustrates the limitations of Slater's Rule for heavier elements and concludes the tutorial on estimating effective nuclear charge.

Mindmap
Keywords
💡Slater's Rule
Slater's Rule is a method used in chemistry to estimate the effective nuclear charge (ENC) experienced by a particular electron in an atom. It's crucial for understanding atomic structure and the behavior of electrons. In the video, Slater's Rule is applied to various elements to estimate the ENC for different types of electrons, such as 2s in beryllium, 2p in fluorine, and 3d in titanium.
💡Effective Nuclear Charge (ENC)
Effective Nuclear Charge is the net positive charge experienced by an electron in an atom due to the nucleus and the shielding effect of other electrons. It's a key concept in the video, as it's the main value being estimated using Slater's Rule. For example, the video calculates the ENC for a 2s electron in beryllium as 1.95 using this concept.
💡Atomic Number (Z)
The atomic number of an element, denoted by 'Z', is the number of protons in the nucleus of an atom. It's used in the formula for calculating ENC. In the script, the atomic number is used to determine the starting point for the ENC calculation, such as for beryllium (Z=4) and fluorine (Z=9).
💡Electron Configuration
Electron configuration describes the distribution of electrons in an atom's orbitals. It's essential for applying Slater's Rule, as the configuration determines the shielding effects on the electron of interest. The video script provides examples of electron configurations for beryllium, fluorine, and titanium.
💡Shielding Effect
The shielding effect refers to the reduction of the nuclear charge experienced by an electron due to the presence of other electrons in the same or lower energy levels. It's a fundamental concept in the video, as it affects the value of 's' in Slater's Rule and thus the ENC estimate.
💡Inner Shell Electrons
Inner shell electrons are those found in lower energy levels than the electron being analyzed. In the context of Slater's Rule, the script mentions calculating 's' by considering the shielding effect of these electrons, such as the 1s and 2s electrons in the case of the 2p electron in fluorine.
💡Energy Levels
Energy levels in an atom are the regions within which electrons are likely to be found. The script discusses electrons in different energy levels, such as the 2s and 2p levels in beryllium and fluorine, and the 3d level in titanium, when calculating the ENC.
💡Periodic Table
The periodic table is a tabular arrangement of the chemical elements, ordered by their atomic number, electron configuration, and recurring chemical properties. It's referenced in the video to find the atomic number of elements, which is necessary for using Slater's Rule.
💡Fluorine
Fluorine is an element used as an example in the video to demonstrate the application of Slater's Rule. Its electron configuration and the calculation of the ENC for its 2p electron illustrate the process of estimating the charge experienced by electrons in heavier elements.
💡Titanium
Titanium is another element featured in the script, where its 3d electron's ENC is calculated using Slater's Rule. The video explains the specific adjustments to the rule when dealing with d and f electrons, highlighting the differences in shielding values.
💡Light and Heavy Elements
The terms 'light' and 'heavy' elements in the script refer to the atomic mass and the number of protons. The video notes that the accuracy of Slater's Rule for estimating ENC can vary, with lighter elements like lithium providing more accurate estimates compared to heavier elements like potassium.
Highlights

Introduction to Slater's Rule and its use in estimating the effective nuclear charge.

Example of calculating the effective nuclear charge for a 2s electron in beryllium.

Explanation of the formula for effective nuclear charge calculation: Z - S.

Determination of atomic number Z for beryllium as 4.

Slater's Rule values for inner shell electrons based on energy levels.

Calculation of S value for beryllium's 2s electron using Slater's Rule.

Estimation of effective nuclear charge for beryllium's 2s electron as 1.95.

Comparison of estimated value to the actual value reported on Wikipedia.

Second example: estimating the effective nuclear charge for a 2p electron in fluorine.

Electron configuration of fluorine and application of Slater's Rule for s and p electrons.

Calculation of S value for fluorine's 2p electron and its effective nuclear charge.

Third example: estimating the effective nuclear charge for a 3d electron in titanium.

Electron configuration of titanium and rules for calculating S value for d electrons.

Estimation of effective nuclear charge for titanium's 3d electron and its comparison to the reported value.

Final example: estimating the effective nuclear charge for 1s electrons in lithium, magnesium, and potassium.

Use of a simplified S value for 1s electrons and its impact on estimation accuracy.

Demonstration of the increasing deviation in estimations for heavier elements.

Conclusion summarizing the use of Slater's Rule for estimating effective nuclear charge.

Transcripts

Browse More Related Video

7.1b Slater's Rules | General Chemistry

Trick for Slater's Rule, calculation of screening constant and effective nuclear charge.

Using Slater's Rules: 3 Examples

How To Calculate The Effective Nuclear Charge of an Electron

[H2 Chemistry] 2023 Topic 1 Atomic Structure & Physical Periodicity 2

Atomic Radius - Basic Introduction - Periodic Table Trends, Chemistry

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Slater's RuleNuclear ChargeElectron ConfigurationBeryllium 2sFluorine 2pTitanium 3dChemical EstimationQuantum MechanicsEducational VideoAtomic PhysicsElectron Shielding